
A geometric sequence is a sequence of numbers in which each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio. Geometric sequences can be found in various mathematical and real-world contexts, such as financial growth, population growth, and exponential decay.
The answer key for a geometric sequence provides the values of each term in the sequence based on the given parameters, such as the first term and the common ratio. The answer key allows students and learners to easily check their work and verify the correctness of their calculations.
By using the answer key, students can quickly compare their answers with the correct solutions, identify any errors or misconceptions, and make corrections if necessary. This helps in reinforcing the understanding of geometric sequences and their properties, and helps students improve their problem-solving skills.
Furthermore, the answer key serves as a valuable resource for teachers and instructors. It allows them to easily assess and evaluate the progress and understanding of their students. They can use the answer key to identify any common mistakes or misconceptions among the students, and provide targeted feedback and guidance to address those issues. The answer key also enables teachers to determine the effectiveness of their instructional strategies and materials in teaching geometric sequences.
What is a Geometric Sequence?
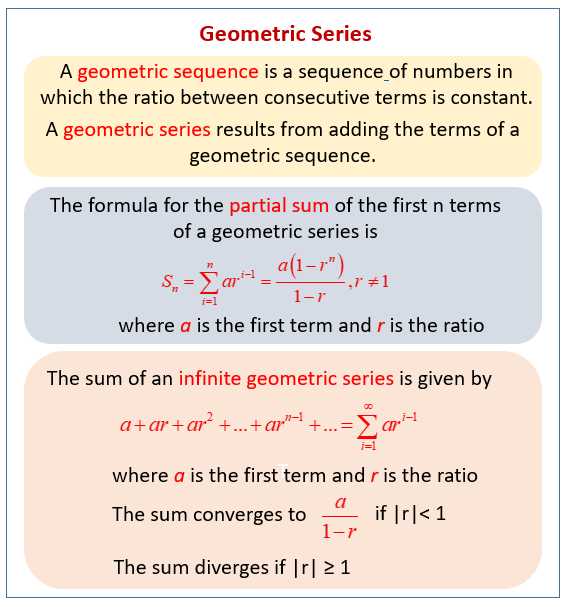
A geometric sequence, also known as a geometric progression, is a sequence of numbers in which each term is found by multiplying the previous term by a fixed, nonzero number called the common ratio. The general form of a geometric sequence can be represented as:
a, ar, ar^2, ar^3, …
Here, a is the first term of the sequence and r is the common ratio. Each term after the first term is obtained by multiplying the previous term by the common ratio. For example, if the first term is 2 and the common ratio is 3, then the sequence would be:
- 2
- 2 * 3 = 6
- 6 * 3 = 18
- 18 * 3 = 54
- …
Geometric sequences can have both finite and infinite terms. If the common ratio is between -1 and 1, the terms of the sequence approach 0 as we move further along the sequence, resulting in an infinite number of terms. On the other hand, if the common ratio is greater than 1, the terms will increase indefinitely.
Geometric sequences are used in various fields such as finance, physics, and computer science. They are particularly useful for modeling exponential growth and decay, as well as calculating compound interest and population growth. Understanding geometric sequences is essential for solving problems involving exponential relationships and understanding patterns in mathematical sequences.
How to Find the nth Term of a Geometric Sequence
Geometric sequences are a type of mathematical sequence where each term is found by multiplying the previous term by a constant. To find the nth term of a geometric sequence, you can use a formula that involves the first term, the common ratio, and the value of n.
The formula to find the nth term of a geometric sequence is given by:
nth term = a * r^(n-1)
Where “a” represents the first term of the sequence, “r” represents the common ratio, and “n” represents the position of the term you are trying to find.
Let’s take an example to understand how this formula works. Consider the geometric sequence: 2, 4, 8, 16, 32, … In this sequence, the first term (a) is 2 and the common ratio (r) is 2. If we want to find the 5th term (n = 5), we can plug in these values into the formula as follows:
5th term = 2 * 2^(5-1)
5th term = 2 * 2^4
5th term = 2 * 16
5th term = 32
Therefore, the 5th term of the geometric sequence 2, 4, 8, 16, 32 is 32.
This formula can be used to find the nth term of any geometric sequence as long as you know the values of the first term, the common ratio, and the position of the term you want to find.
Using the Formula
In mathematics, a geometric sequence is a sequence of numbers in which each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio. In other words, to find any term in a geometric sequence, you can use the formula:
an = a1 * r(n-1)
Where an is the value of the term you want to find, a1 is the value of the first term, r is the common ratio, and n is the position of the term in the sequence.
Using this formula, you can easily find any term in a geometric sequence without having to manually calculate each term. Simply plug in the values of a1, r, and n into the formula and solve for an.
For example, let’s say we have a geometric sequence where the first term (a1) is 2 and the common ratio (r) is 3. We want to find the value of the 5th term (a5). Using the formula, we have:
a5 = 2 * 3(5-1) = 2 * 34 = 2 * 81 = 162
So, the value of the 5th term in this geometric sequence is 162.
This formula is especially useful when dealing with large sequences, as it allows for quick and accurate calculations without having to manually calculate each term. It is an essential tool in the study of geometric sequences and their properties.
Using the Common Ratio and the First Term
The common ratio and the first term are two important components in determining the terms of a geometric sequence. The common ratio, denoted as ‘r’, is the constant ratio between consecutive terms in the sequence. This means that every term in the sequence can be found by multiplying the previous term by the common ratio. The first term, denoted as ‘a₁’, is the initial value of the sequence.
To find any term in a geometric sequence, one can use the formula:
an = a1 * r(n-1)
where ‘aₙ’ is the term to be found, ‘a₁’ is the first term, ‘r’ is the common ratio, and ‘n’ is the position of the term in the sequence.
By knowing the common ratio and the first term, one can easily calculate any term in the sequence. For example, if the common ratio is 2 and the first term is 3, we can find the 5th term by substituting the values into the formula:
a5 = 3 * 2(5-1) = 3 * 24 = 3 * 16 = 48
By understanding the relationship between the common ratio and the first term, one can find or predict any term in a geometric sequence. This knowledge is crucial in various mathematical and real-world applications, such as financial calculations, population growth models, and scientific analyses.
How to Find the Sum of a Geometric Sequence
A geometric sequence is a sequence of numbers in which each term is found by multiplying the previous term by a common ratio. Finding the sum of a geometric sequence can be done using a formula that takes into account the first term, the common ratio, and the number of terms in the sequence.
To find the sum of a geometric sequence, you can use the formula:
Sn = a(1 – r^n) / (1 – r)
Where:
- Sn is the sum of the first n terms
- a is the first term in the sequence
- r is the common ratio
- n is the number of terms in the sequence
By plugging in the appropriate values into the formula, you can easily find the sum of a geometric sequence. It is important to note that this formula only works when the absolute value of the common ratio is less than 1. If the common ratio is greater than 1 or less than -1, the sum of the geometric sequence will be infinite.
For example, let’s say we have a geometric sequence with a first term of 3, a common ratio of 2, and 5 terms. Plugging these values into the formula, we get:
| n | Sn |
|---|---|
| 1 | 3 |
| 2 | 9 |
| 3 | 21 |
| 4 | 45 |
| 5 | 93 |
Therefore, the sum of this geometric sequence is 93.
In conclusion, finding the sum of a geometric sequence involves using a formula that takes into account the first term, the common ratio, and the number of terms. By plugging in the appropriate values, you can easily calculate the sum of the sequence. However, it is important to note that this formula only works when the absolute value of the common ratio is less than 1.
Using the Formula
The formula for finding any term of a geometric sequence is given by an = a1 * r^(n-1). This formula allows us to calculate the value of any term in the sequence without having to manually calculate each term.
To use the formula, we need to know the value of the first term (a1) and the common ratio (r). Once we have these values, we can substitute them into the formula to find any specific term in the sequence.
For example, let’s say we have a geometric sequence with a first term of 3 and a common ratio of 2. To find the fifth term in the sequence, we can use the formula: a5 = 3 * 2^(5-1). Simplifying this equation gives us a5 = 3 * 2^4, which equals 48. Therefore, the fifth term in this sequence is 48.
The formula for a geometric sequence is very useful in solving various problems involving patterns, growth, and decay. It allows us to quickly find any term in the sequence without having to calculate each term individually. This can be especially helpful when dealing with large sequences or complex patterns.
Using the Common Ratio and the First Term
The common ratio is an important concept in geometric sequences. It represents the ratio between any term and the previous term in the sequence. To find the common ratio, you can divide any term by its previous term. For example, in the sequence 2, 4, 8, 16, the common ratio is 2, because each term is twice the previous term. The first term, denoted as ‘a’, is the starting point of the sequence. It is usually given, but it can also be calculated if the common ratio and any term in the sequence are known.
To calculate the first term, you can use the formula a = t / r^(n-1), where a is the first term, t is any term in the sequence, r is the common ratio, and n is the position of the term in the sequence. By rearranging the formula, you can solve for a. For example, if you know the common ratio is 2, and the third term in the sequence is 8, you can substitute these values into the formula and solve for a. In this case, a = 8 / 2^(3-1) = 8 / 4 = 2.
Knowing the common ratio and the first term is useful for finding specific terms in a geometric sequence. Once you have the first term, you can use the formula t = a * r^(n-1) to find any term in the sequence. Here, t represents the term you want to find, a is the first term, r is the common ratio, and n is the position of the term in the sequence. This formula allows you to find terms without having to generate the entire sequence.
In summary, the common ratio and the first term are essential concepts in geometric sequences. The common ratio represents the ratio between any term and the previous term, while the first term is the starting point of the sequence. By using formulas involving the common ratio and the first term, you can calculate specific terms in the sequence and find the first term when the common ratio and another term are known.
Examples of Geometric Sequences
Geometric sequences are sequences of numbers where each term is found by multiplying the previous term by a constant ratio. Here are some examples of geometric sequences:
Example 1:
In the sequence 2, 4, 8, 16, 32, each term is obtained by multiplying the previous term by 2. The common ratio in this sequence is 2.
Example 2:
The sequence 3, 9, 27, 81, 243 is a geometric sequence with a common ratio of 3. Each term is obtained by multiplying the previous term by 3.
Example 3:
Consider the sequence 1, -3, 9, -27, 81. Each term in this sequence is obtained by multiplying the previous term by -3. The common ratio in this case is -3.
Example 4:
In the sequence 1/2, 1/4, 1/8, 1/16, the common ratio is 1/2. Each term is obtained by dividing the previous term by 2.
These examples demonstrate the concept of geometric sequences and how each term is related to the previous term by a constant ratio. Geometric sequences can be found in various mathematical and real-world contexts, and understanding their properties can be useful in solving problems involving growth, decay, or exponential functions.
Increasing Geometric Sequence
An increasing geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a constant called the common ratio. The common ratio can be any non-zero number. In an increasing geometric sequence, the terms get progressively larger as the sequence continues.
To find the next term in an increasing geometric sequence, you need to multiply the previous term by the common ratio. For example, if the first term is 2 and the common ratio is 3, the second term would be 2 * 3 = 6, the third term would be 6 * 3 = 18, and so on.
An increasing geometric sequence can be represented by the formula:
an = a1 * r(n-1)
where an is the nth term, a1 is the first term, r is the common ratio, and n is the position of the term in the sequence. This formula allows you to find any term in the sequence without calculating all the previous terms.
For example, if the first term is 2 and the common ratio is 3, you can find the fifth term:
a5 = 2 * 3(5-1) = 2 * 34 = 2 * 81 = 162
So, the fifth term in this increasing geometric sequence is 162. The sequence continues to grow exponentially with each subsequent term.