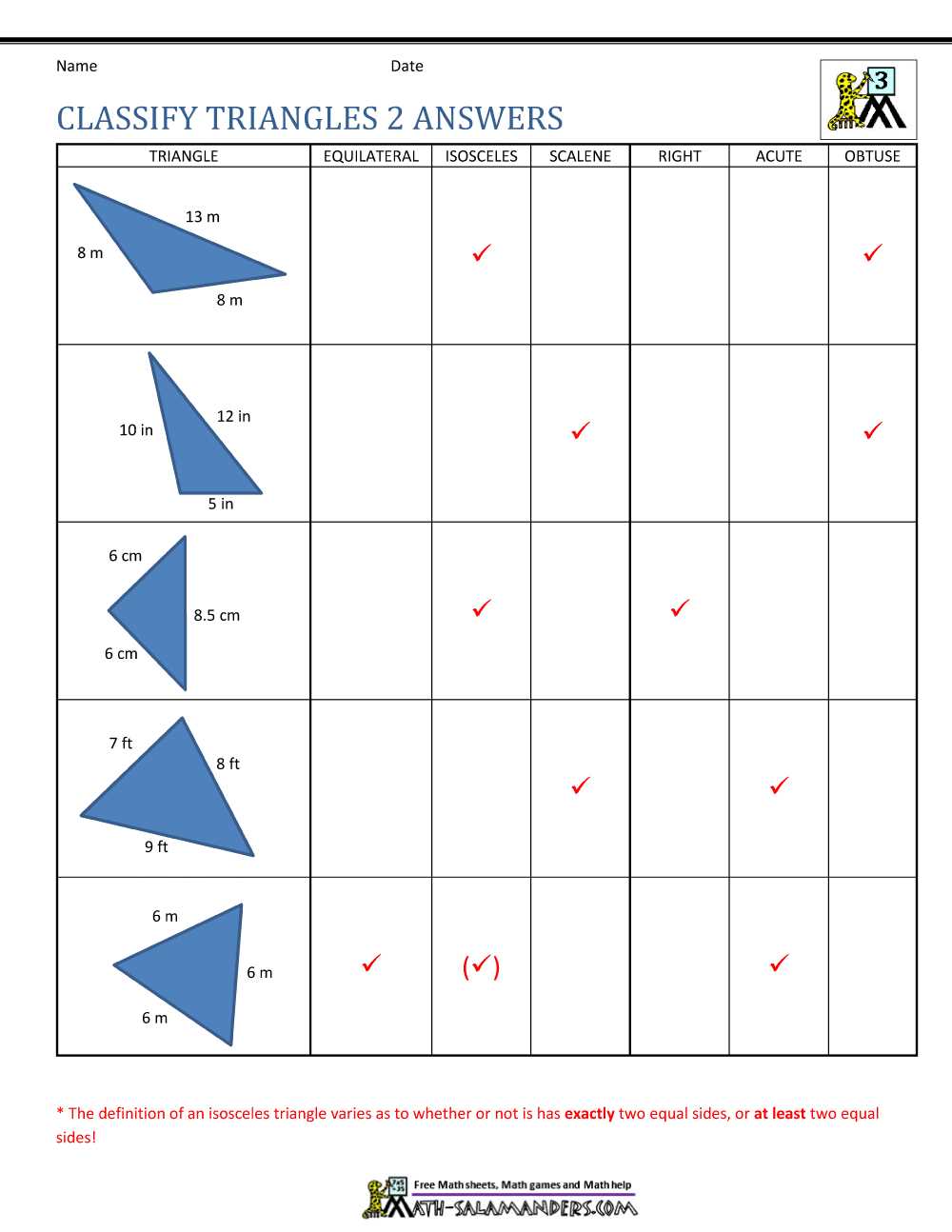
Geometry is a branch of mathematics that deals with shapes, sizes, and properties of figures and spaces. It is a fundamental subject that plays a crucial role in various branches of science and engineering. In geometry, students often have to solve problems and answer questions related to angles, lines, triangles, polygons, and other geometric figures.
Worksheet assignments are a common way for students to practice their geometry skills and apply the concepts they have learned in class. Geometry 3 3 worksheet is one such assignment that focuses on a specific topic in geometry. These worksheets typically contain a set of questions that require students to find the answers using their knowledge of geometric principles and formulas.
When solving a Geometry 3 3 worksheet, students may encounter questions about different types of angles, such as acute, right, obtuse, or straight angles. They may also need to identify and calculate the measures of angles formed by intersecting lines or parallel lines cut by a transversal. Additionally, these worksheets may include questions related to the properties and classifications of triangles and quadrilaterals.
Having access to the answers for Geometry 3 3 worksheets can be immensely helpful for students. It allows them to check their work, verify their solutions, and learn from any mistakes they may have made. Moreover, having the answers readily available can also serve as a study guide and provide additional practice for future exams or assessments.
Geometry 3 3 Worksheet Answers

In geometry, worksheets are commonly used to practice and reinforce concepts learned in class. They provide students with an opportunity to apply their knowledge and skills to various problems and exercises. One common topic in geometry is angles, and in this worksheet, students are likely required to solve problems related to angle relationships.
The geometry 3 3 worksheet answers would typically include solutions to problems involving parallel lines and transversals. Students would need to identify corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles. They would also need to use properties of these angle relationships to find missing angle measures.
For example, one question on the worksheet might ask students to find the measure of a specific angle given that it is a corresponding angle to another angle whose measure is provided. The answer would involve applying the property of corresponding angles, stating that when a transversal intersects two parallel lines, the corresponding angles are congruent.
Another question might involve finding the measure of an alternate interior angle given that the measure of one of the corresponding alternate exterior angles is provided. The answer would require using the property that when a transversal intersects two parallel lines, the alternate interior angles are congruent.
In summary, the geometry 3 3 worksheet answers would involve applying angle relationships involving parallel lines and transversals, and using the properties of corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles to find missing angle measures.
Key Concepts in Geometry 3 3
In Geometry 3 3, students learn about several key concepts related to angles and intersecting lines. These concepts include alternate interior angles, alternate exterior angles, corresponding angles, and consecutive interior angles.
Alternate interior angles: When two parallel lines are intersected by a transversal, alternate interior angles are the pair of angles that are on opposite sides of the transversal and inside the two parallel lines.
Alternate exterior angles: Similar to alternate interior angles, alternate exterior angles are the pair of angles that are on opposite sides of the transversal and outside the two parallel lines.
Corresponding angles: Corresponding angles are the pair of angles that are in the same position relative to the two parallel lines and the transversal. In other words, they are located on the same side of the transversal and in the same relative position with respect to the two parallel lines.
Consecutive interior angles: Consecutive interior angles are the pair of angles that are on the same side of the transversal and inside the two parallel lines.
Understanding these key concepts is crucial in solving various geometry problems and proving geometric theorems. By recognizing and applying the properties of these angles, students can determine relationships between different angles and lines, ultimately leading to a deeper understanding of geometric principles and the ability to solve complex problems.
Understanding the Worksheet Questions
When working on a Geometry 3 3 worksheet, it is important to have a clear understanding of the questions being asked. This will not only help you solve the problems correctly, but it will also allow you to approach each question with the appropriate mathematical concepts and tools.
The first step in understanding the worksheet questions is to carefully read each question and identify the key information and requirements. This may involve analyzing given measurements, identifying geometric shapes or figures, or recognizing specific geometric properties or formulas that are relevant to the problem.
For example:
- Question 1: Find the area of a rectangle with length 10 meters and width 5 meters.
- Question 2: Determine the perimeter of a square with a side length of 8 centimeters.
In question 1, the key information is the length and width of the rectangle. To find the area, you would use the formula: A = length × width. In question 2, the key information is the side length of the square. To find the perimeter, you would use the formula: P = 4 × side length.
Once you have identified the key information, you can use your knowledge of geometric concepts and formulas to solve the problem. It may be helpful to make a plan or outline of the steps you will take to solve the problem before starting your calculations.
By understanding the worksheet questions and applying the appropriate mathematical concepts, you will be able to successfully solve the problems and reinforce your understanding of geometry concepts.
Step-by-Step Solutions for Question 1
In question 1, we are given a triangle ABC with coordinates A(-3, -1), B(4,5), and C(2,-3). The first step is to find the lengths of the sides of the triangle using the distance formula. The distance formula is given by:
d = √((x2 – x1)^2 + (y2 – y1)^2)
Using this formula, we can find the length of side AB:
d(AB) = √((4 – (-3))^2 + (5 – (-1))^2)
d(AB) = √((7)^2 + (6)^2)
d(AB) = √(49 + 36)
d(AB) = √85
Similarly, we can find the lengths of sides BC and CA:
d(BC) = √((2 – 4)^2 + (-3 – 5)^2)
d(BC) = √((-2)^2 + (-8)^2)
d(BC) = √(4 + 64)
d(BC) = √68
d(CA) = √((-3 – 2)^2 + (-1 – (-3))^2)
d(CA) = √((-5)^2 + (2)^2)
d(CA) = √(25 + 4)
d(CA) = √29
Now, we can calculate the perimeter of the triangle by adding the lengths of all three sides:
Perimeter = √85 + √68 + √29
Therefore, the step-by-step solution for question 1 involves finding the lengths of the sides of the triangle using the distance formula, and then calculating the perimeter by adding the lengths of all three sides.
Step-by-Step Solutions for Question 2
In question 2, we are given a triangle ABC with vertices A(3, 4), B(7, 8), and C(2, 6). The question asks us to find the length of side AC. To find the length of a line segment, we can use the distance formula:
Distance formula: The distance between two points (x1, y1) and (x2, y2) is given by the formula: d = √((x2 – x1)^2 + (y2 – y1)^2).
To find the length of side AC, we will use the distance formula with points A(3, 4) and C(2, 6):
- Step 1: Find the difference in x-coordinates: (x2 – x1) = (2 – 3) = -1.
- Step 2: Find the difference in y-coordinates: (y2 – y1) = (6 – 4) = 2.
- Step 3: Square the differences: (-1)^2 = 1 and (2)^2 = 4.
- Step 4: Add the squared differences: 1 + 4 = 5.
- Step 5: Take the square root of the sum: √5.
Therefore, the length of side AC is √5 units.
Step-by-Step Solutions for Question 3
Question 3 in the Geometry 3 worksheet involves finding the measure of an angle in a triangle given two side lengths. Here’s how you can solve it step by step:
Step 1: Start by labeling the triangle. Let’s call the vertices A, B, and C, and the sides a, b, and c, respectively. Also, label the given side lengths as follows: a = 6 cm and b = 9 cm.
Step 2: Use the Law of Cosines to find the measure of angle C. The Law of Cosines states that c^2 = a^2 + b^2 – 2ab*cos(C). Plug in the known values into the equation: c^2 = 6^2 + 9^2 – 2(6)(9)*cos(C).
Step 3: Simplify the equation and solve for cos(C). In this example, c^2 = 36 + 81 – 108*cos(C).
Step 4: Once you have found cos(C), use the inverse cosine function (cos^-1) to find the measure of angle C. Calculate cos^-1 of the value you obtained in the previous step.
Step 5: Finally, you have found the measure of angle C. Round the result to the nearest degree or decimal place, depending on the instructions given in the worksheet.
By following these step-by-step instructions, you can find the measure of angle C in question 3 of the Geometry 3 worksheet. Remember to use the Law of Cosines and trigonometric functions to solve the problem accurately.
Step-by-Step Solutions for Question 4
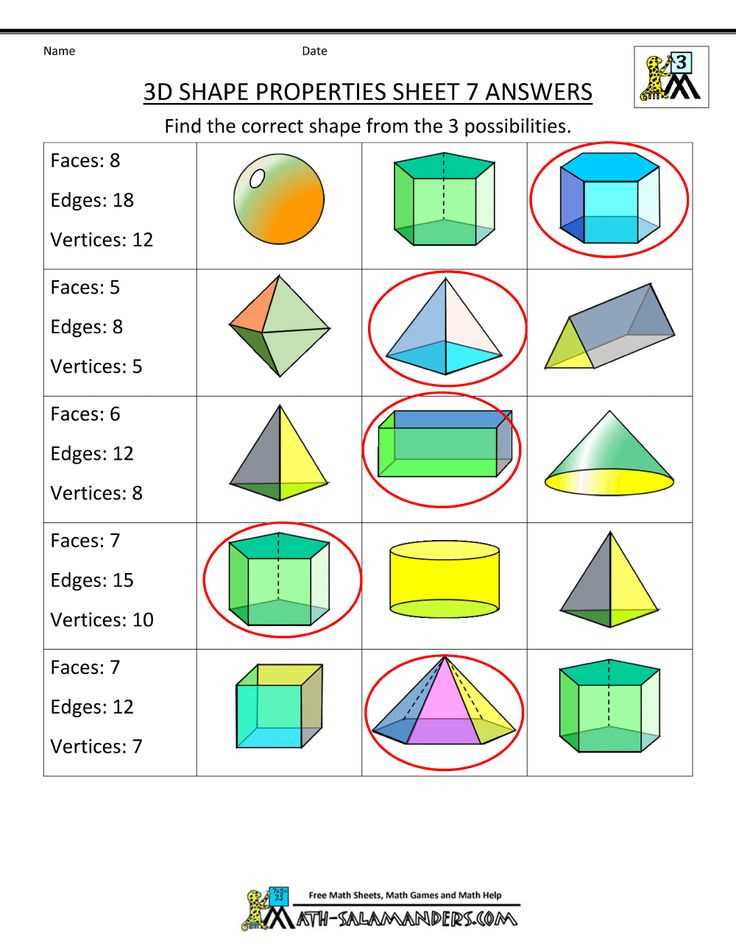
In question 4 of the Geometry 3 3 worksheet, we are given a figure with three lines intersecting at a point. Our task is to find the value of a missing angle based on the given information.
Let’s analyze the figure and the given information. We are told that one of the angles formed by the intersecting lines measures 85 degrees. Additionally, another angle is marked as 45 degrees. Our goal is to find the measure of the angle represented by ‘x’ in the figure.
To find the value of ‘x’, we can use the fact that the sum of angles around a point is equal to 360 degrees. We already know that one angle measures 85 degrees, and another angle measures 45 degrees. Therefore, the third angle, represented by ‘x’, can be found by subtracting the sum of the known angles from 360 degrees. Mathematically, we can express this as:
x = 360 – (85 + 45)
By evaluating the expression, we get:
x = 360 – 130 = 230
Therefore, the missing angle ‘x’ in the figure has a measure of 230 degrees.
Common Mistakes to Avoid
When working on geometry 3 3 worksheets, there are several common mistakes that students often make. By being aware of these mistakes, you can avoid making them yourself and improve your understanding of the topic.
1. Incorrectly identifying angles: One common mistake is misidentifying angles in a given geometric figure. It is important to carefully analyze the figure and correctly label the angles. Pay close attention to angle measures and relationships between angles.
2. Misusing angle properties: Another common mistake is misusing angle properties, such as the vertical angles or the alternate interior angles theorem. It is crucial to understand and apply these properties correctly to solve geometry problems. Take your time to review these properties and practice applying them in different contexts.
3. Failing to show all steps: Showing all the steps in your solution is essential in geometry. Skipping steps or not clearly explaining your reasoning can lead to mistakes and make it difficult for others to follow your work. Make sure to write out all the steps clearly, including any theorems or postulates that you use.
4. Not reviewing previous topics: Geometry concepts are often interconnected, and it is important to continually review and reinforce previous topics. Failing to do so can lead to gaps in your understanding and make it harder to solve more complex problems. Take the time to review previous concepts and ensure you have a strong foundation.
5. Relying solely on memorization: Memorizing formulas and theorems can be helpful but relying solely on memorization can limit your understanding. Instead, focus on understanding the underlying concepts and how different ideas relate to each other. This will allow you to apply your knowledge in a more flexible manner and solve a wider range of problems.
In conclusion,
- Take the time to carefully analyze geometric figures and correctly identify angles.
- Understand and apply angle properties correctly.
- Show all steps in your solution, explaining your reasoning clearly.
- Continually review and reinforce previous topics to build a stronger foundation.
- Focus on understanding concepts rather than solely relying on memorization.
By avoiding these common mistakes and practicing regularly, you can improve your skills in geometry 3 3 and achieve better results in your worksheets and exams.
Further Practice and Resources

Once you have completed the Geometry 3 3 worksheet, it is important to further practice your skills and reinforce the concepts you have learned. Here are some resources and activities that can help you do just that:
- Online Practice: There are several websites that offer free geometry practice questions and quizzes. These can be a great way to test your knowledge and identify areas where you may need additional practice.
- Textbook Exercises: If you have a geometry textbook, make use of the exercises and problems provided. Try to solve as many problems as you can, paying attention to the steps and strategies used to arrive at the correct answer.
- Work with a Study Group: Collaborating with classmates who are studying the same topic can be beneficial. Work together on challenging problems, discuss concepts, and share resources. This can help reinforce your understanding and provide an opportunity for peer learning.
- Tutoring or Extra Help: If you are struggling with certain concepts or need additional guidance, consider seeking extra help from a teacher, tutor, or classmate who excels in geometry. They can provide you with personalized instruction and assistance.
Remember, practice is key when it comes to mastering geometry. Make use of these resources and activities to continue honing your skills and building a solid foundation in geometry.