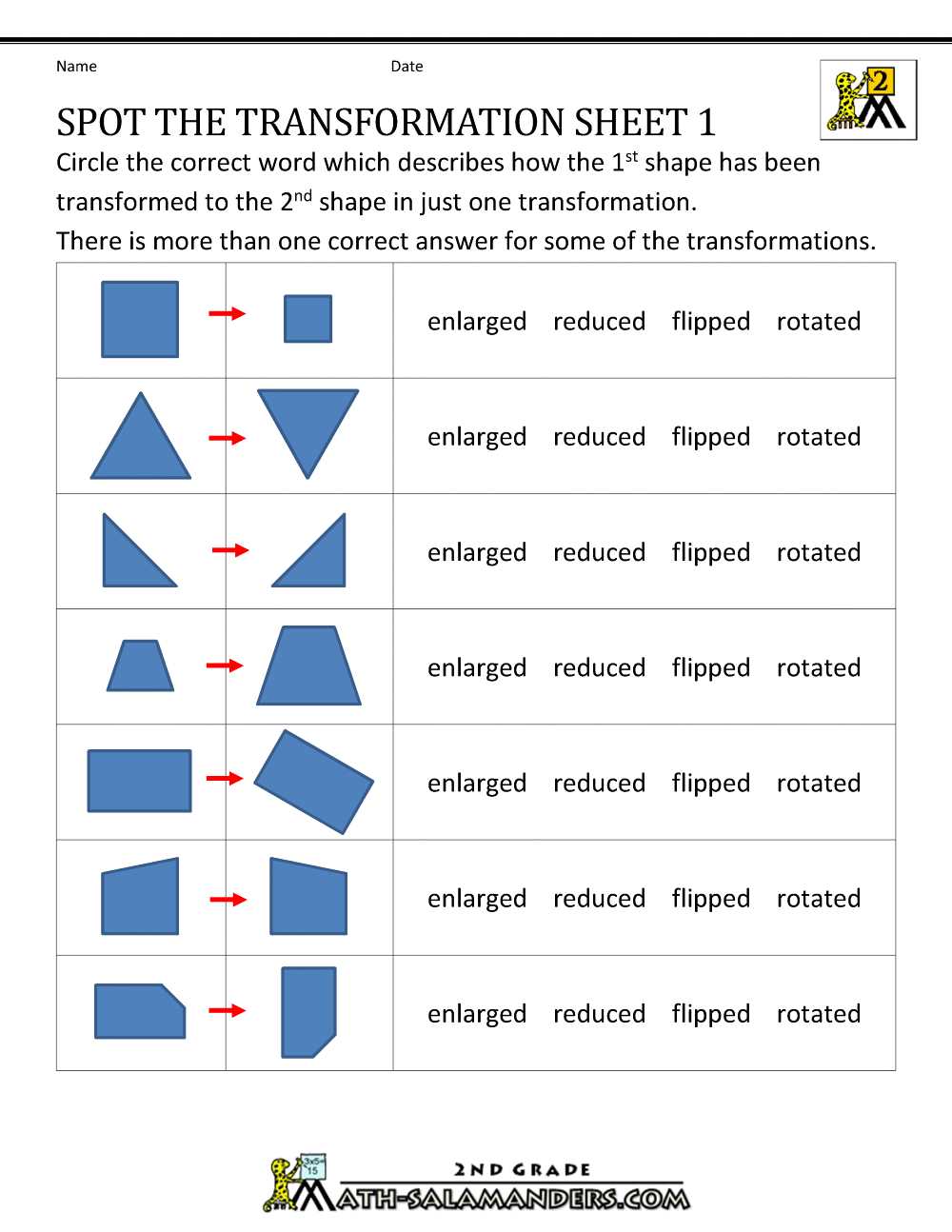
Geometry can be a challenging subject for many students, especially when it comes to solving equations and finding answers on worksheets. In the case of worksheet 8 5, it is important to understand the concepts and formulas needed to successfully complete the problems. This article will dive into the details of geometry 8 5 worksheet answers, explaining the steps and reasoning behind each solution.
One of the key aspects of worksheet 8 5 is understanding the different types of shapes and their properties. Students will need to have a solid grasp of concepts such as angles, triangles, quadrilaterals, and circles. By knowing the definitions and characteristics of each shape, students can easily identify the correct formulas and procedures to use when solving problems.
Another important skill needed to tackle this worksheet is the ability to apply geometric reasoning. Students will encounter problems that require them to use their knowledge of congruent triangles, similar figures, and basic trigonometric ratios. By analyzing the given information and applying the appropriate reasoning, students can determine the correct answers.
Finally, attention to detail is crucial when solving worksheet 8 5. It is important to carefully read each problem and understand what is being asked. Students should make sure to label diagrams correctly, use the appropriate units, and show all necessary work to support their answers. By double-checking their work and paying attention to small details, students can ensure they are providing accurate and complete answers.
Geometry 8 5 Worksheet Answers: Essential Tips and Solutions
Geometry 8 5 worksheet answers can be challenging to find if you’re struggling with this particular topic. However, with the right tips and solutions, you can effectively navigate through the problems and arrive at the correct answers.
When approaching a geometry 8 5 worksheet, it’s crucial to first understand the underlying concepts and definitions. Familiarize yourself with the relevant theorems, postulates, and formulas to have a solid foundation for solving the problems.
One key tip is to carefully read and analyze the given information in each problem. Clearly identify the given angles, lines, and shapes, and make note of any specific relationships or properties mentioned. This will help you determine the appropriate approach to finding the answer.
Another important strategy is to draw accurate diagrams or figures to visualize the problem. Geometry problems often require visual representation, so sketching out the given information can provide valuable insight into the solution. Ensure your diagrams accurately reflect the given information and adhere to the necessary geometric principles.
To solve each problem, apply the relevant theorems and properties. Use logical reasoning and deductive thinking to derive conclusions about the relationships between angles, lines, and shapes. Don’t be afraid to break down complex problems into smaller, more manageable parts before tackling the entire question.
Remember to double-check your work and verify that your answer is supported by the given information and satisfies all the required conditions. Precision is vital in geometry, so pay attention to details and be mindful of any potential errors in interpretation or calculations.
In conclusion, mastering geometry 8 5 worksheet answers requires a strong understanding of the concepts, attention to detail, and logical problem-solving skills. With these essential tips and solutions, you can confidently approach and excel in your geometry assignments.
Understanding Geometry 8 5 Worksheet: An Overview
Geometry 8 5 Worksheet is designed to help students understand and practice concepts related to geometry, specifically focusing on the properties of parallelograms. This worksheet provides a variety of problems that require students to apply their knowledge of parallelogram properties, such as angle measures, side lengths, and diagonals.
One of the main objectives of this worksheet is to help students recognize the different properties and characteristics of parallelograms. By working through various examples and exercises, students will develop their ability to identify parallelograms based on their properties, such as opposite sides being parallel and congruent opposite angles.
The worksheet also includes problems that require students to use the properties of parallelograms to find missing angle measures or side lengths. This type of problem helps students apply their knowledge in a real-world context and develop problem-solving skills.
The Geometry 8 5 Worksheet also covers the concept of using diagonals in parallelograms. Students will learn how to find the length and midpoint of diagonals, as well as determine if diagonals bisect each other. These skills are important in understanding the geometric properties and relationships within parallelograms.
In conclusion, the Geometry 8 5 Worksheet provides a comprehensive overview of parallelogram properties and challenges students to apply their knowledge in solving various problems. By working through this worksheet, students will deepen their understanding of geometry and develop important skills for future mathematical studies.
Importance of Geometry 8 5 Worksheet in Mathematics Curriculum
Geometry is an essential branch of mathematics that deals with the study of shapes, sizes, and properties of figures and spaces. It plays a crucial role in developing spatial reasoning skills and analytical abilities, making it an integral part of the mathematics curriculum. One specific worksheet that holds significance in this curriculum is the Geometry 8 5 worksheet.
The Geometry 8 5 worksheet focuses on the topic of right triangles and trigonometric ratios. It provides students with a structured set of problems and exercises that allow them to apply their knowledge of trigonometry concepts. By solving problems related to right triangles, students develop a deep understanding of the relationships between angles, sides, and ratios in geometry.
Through the Geometry 8 5 worksheet, students learn how to calculate the values of sine, cosine, and tangent for given angles in right triangles. They also practice solving problems involving side lengths and angle measures using trigonometric ratios. These skills are not only essential for geometry but also have applications in various fields such as physics, engineering, and architecture.
The Geometry 8 5 worksheet provides an organized and systematic approach to learning trigonometry concepts. It allows students to reinforce their understanding of important geometric principles and apply them to real-world scenarios. By practicing with the worksheet, students become proficient in solving trigonometry problems, which in turn enhances their problem-solving abilities and logical thinking skills.
In conclusion, the Geometry 8 5 worksheet is a valuable resource in the mathematics curriculum as it helps students master the concepts of right triangles and trigonometry. It enables them to develop their spatial reasoning skills, analytical abilities, and problem-solving skills. By engaging with the worksheet, students can gain a solid foundation in geometry and apply their knowledge to real-life situations.
Common Challenges Faced by Students in Geometry 8 5 Worksheet
Geometry 8 5 worksheet deals with various concepts related to geometry, including angles, triangles, and the Pythagorean theorem. While some students may find the concepts and problems in this worksheet relatively easy to grasp, others may face certain challenges. Here are some common challenges faced by students in Geometry 8 5 worksheet.
1. Understanding Angle Properties:
One of the challenges students face is a lack of understanding of angle properties. This includes identifying vertical angles, adjacent angles, and corresponding angles. Without a clear understanding of these properties, solving problems involving angles can become difficult.
2. Applying the Pythagorean Theorem:
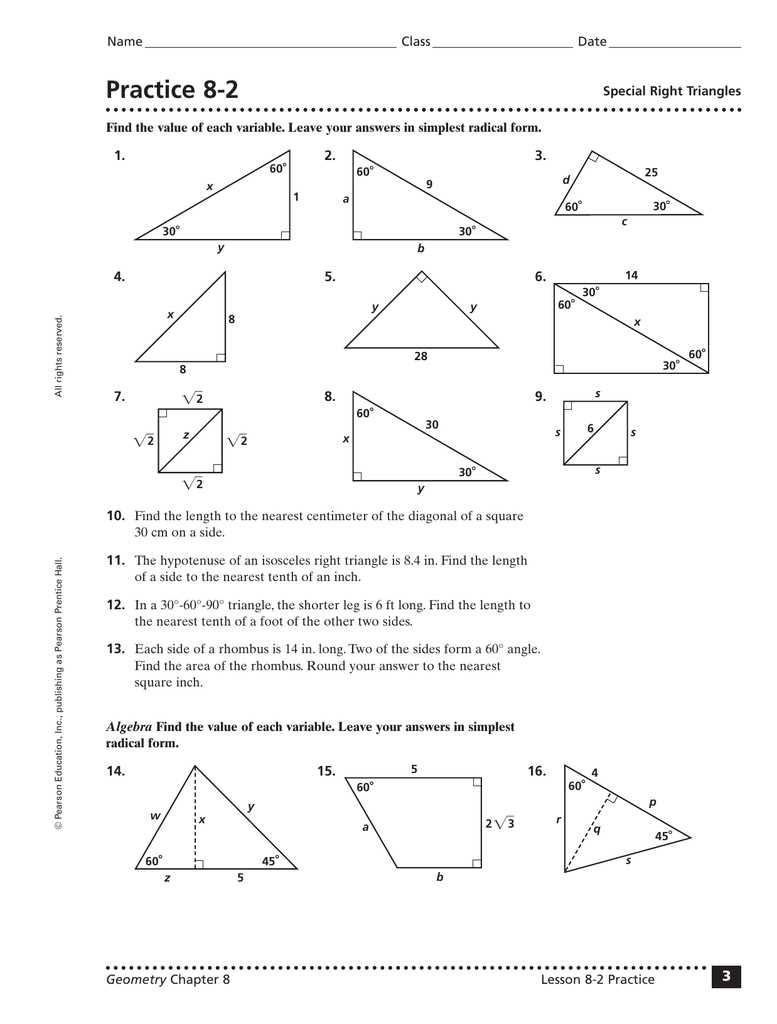
The Pythagorean theorem is a key concept in geometry, and students may struggle to apply it correctly in their calculations. The theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. Students may find it challenging to identify the hypotenuse and correctly set up the equation needed to solve the problem.
3. Working with Triangles:
Geometry 8 5 worksheet often includes problems involving triangles, such as finding missing side lengths or angles. Students may struggle to apply the different formulas and concepts related to triangles, including the Pythagorean theorem, the sum of angles in a triangle, and the properties of similar triangles. This can make solving triangle-related problems challenging.
4. Visualization and Spatial Reasoning:

Geometry heavily relies on visualizing shapes and solving spatial problems. Some students may find it challenging to visualize how different shapes fit together or to understand the spatial relationships between angles, lines, and shapes. This can make it difficult to solve problems involving angles, side lengths, and geometric constructions.
5. Logical Reasoning in Proofs:
Geometry involves logical reasoning and proofs, which can be challenging for students. Understanding how to construct a logical argument and provide supporting evidence can be difficult, especially when faced with complex geometric problems that require multiple steps to prove a statement or theorem.
In conclusion, students in Geometry 8 5 worksheet may face various challenges, including understanding angle properties, applying the Pythagorean theorem, working with triangles, visualizing shapes, and using logical reasoning in proofs. It is essential for students to seek additional help or practice to overcome these challenges and improve their understanding of geometry concepts.
Tips for Solving Geometry 8 5 Worksheet Problems
Geometry 8 5 worksheet problems can sometimes be challenging, but with the right approach, you can find solutions efficiently. Here are some tips to help you navigate these types of problems:
- Read the problem carefully: Before attempting to solve the problem, make sure you understand what is being asked. Pay attention to any given information and any specific conditions mentioned.
- Draw a diagram: Geometry problems often involve shapes and angles, so it can be helpful to visualize the situation by creating a clear and accurate diagram. Label the given information and any unknowns.
- Use theorems and formulas: Geometry is based on a set of theorems and formulas. Familiarize yourself with these and use them as appropriate to solve the given problem. Remember to state which theorem or formula you are using and why.
- Break the problem down: If a problem seems overwhelming, break it down into smaller, more manageable steps. Consider the relationships between different parts of the problem and solve each step methodically.
- Check your work: Once you have found a solution, go back and double-check your work. Make sure your calculations are accurate and that your answer makes sense in the context of the problem. If possible, try to verify your solution using a different method.
By following these tips and practicing regularly, you can improve your skills in solving geometry 8 5 worksheet problems. Remember to stay patient and focused, and don’t hesitate to seek help or clarification if needed.
Step-by-Step Solutions to Selected Geometry 8 5 Worksheet Questions
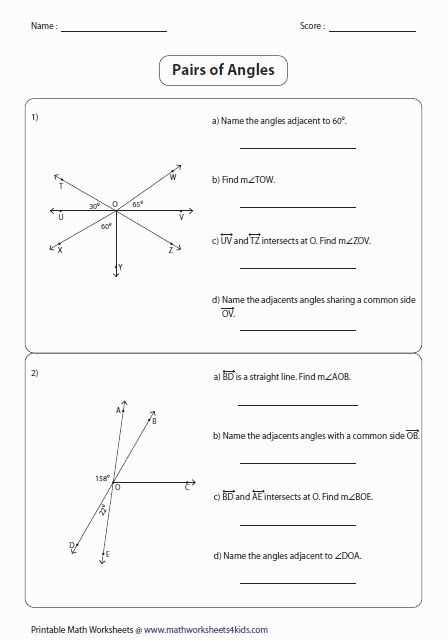
The Geometry 8 5 worksheet contains a variety of challenging questions that test students’ understanding of geometric concepts. In this article, we will provide step-by-step solutions to selected questions from the worksheet, helping students gain a deeper understanding of the topics covered.
Question 1:
* Include the specific question from the worksheet *
In this question, we are asked to find the area of a triangle with a base of 8 units and a height of 5 units. To solve this problem, we can use the formula for the area of a triangle, which is 1/2 times base times height. Plugging in the given values, we have 1/2 times 8 times 5, which simplifies to 20 square units. Therefore, the area of the triangle is 20 square units.
Question 2:
* Include the specific question from the worksheet *
In this question, we are given a polygon and asked to identify its type. To do this, we need to examine the number of sides and angles of the polygon. By counting, we find that the polygon has 6 sides and 6 angles. Since a polygon with 6 sides is called a hexagon, we can conclude that the given polygon is a hexagon.
Question 3:
* Include the specific question from the worksheet *
In this question, we are asked to find the volume of a rectangular prism with dimensions 5 units, 2 units, and 3 units. To find the volume, we multiply the length, width, and height of the prism. Plugging in the given values, we have 5 times 2 times 3, which simplifies to 30 cubic units. Therefore, the volume of the rectangular prism is 30 cubic units.
Question 4:
* Include the specific question from the worksheet *
In this question, we are given the measures of two angles of a triangle and asked to find the measure of the third angle. To solve this problem, we can use the fact that the sum of the measures of the angles in a triangle is always 180 degrees. Let’s say the measures of the given angles are A and B. We can subtract the sum of A and B from 180 to find the measure of the third angle. Therefore, the measure of the third angle is 180 – (A + B) degrees.
By providing step-by-step solutions to selected questions from the Geometry 8 5 worksheet, students can improve their problem-solving skills and deepen their understanding of geometric concepts. It is important to practice these types of problems regularly to excel in geometry.
Key Concepts and Formulas Related to Geometry 8 5
In Geometry 8 5, there are several key concepts and formulas that are important to understand. These concepts and formulas can help solve problems and determine various properties of geometric shapes and figures.
1. Pythagorean theorem: The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. This theorem is often used to find the length of one side in a right triangle when the lengths of the other two sides are known.
2. Similarity: Two geometric figures are considered similar if their corresponding angles are congruent and the ratios of their corresponding side lengths are equal. Similarity is an important concept when dealing with scaling or enlarging geometric figures.
3. Area formulas: There are specific formulas for calculating the area of different geometric shapes, such as triangles, rectangles, squares, circles, and trapezoids. These formulas involve multiplying the lengths of certain sides or using the value of pi for circles.
4. Volume formulas: Similar to area formulas, there are specific formulas for calculating the volume of different three-dimensional geometric shapes, such as cubes, rectangular prisms, cylinders, and spheres. These formulas involve multiplying the lengths of certain sides or using the value of pi for cylinders and spheres.
5. Congruence: Two geometric figures are considered congruent if they have the same shape and size. Congruence is often determined by comparing the lengths of corresponding sides and the measures of corresponding angles.
6. Distance formula: The distance formula is used to find the distance between two points in a coordinate plane. This formula involves finding the difference between the x-coordinates and the y-coordinates of the points and then using the Pythagorean theorem to calculate the distance.
These are just a few of the key concepts and formulas related to Geometry 8 5. By understanding and applying these concepts and formulas, you can solve various geometric problems and gain a deeper understanding of the properties of different shapes and figures.