
Geometry is a fundamental branch of mathematics that deals with the study of shapes, sizes, and properties of objects in space. It plays a crucial role in various fields, including physics, engineering, and architecture. Understanding geometry and mastering its concepts is essential for students, especially when it comes to facing a final exam.
Preparing for a geometry final exam can be challenging, as it requires a thorough understanding of the different geometric principles, theorems, and formulas. Students are often expected to demonstrate their knowledge by solving complex problems, drawing accurate diagrams, and providing clear explanations. Achieving success in a geometry final exam requires not only memorization but also the ability to apply mathematical reasoning and critical thinking skills.
When it comes to studying for a geometry final exam, it is essential to review all the key topics covered throughout the course. This includes concepts such as points, lines, angles, polygons, congruence, similarity, and transformations. In addition to reviewing the theoretical aspects, it is also crucial to practice solving various types of problems, including calculating areas, volumes, and angles in different geometric figures.
In conclusion, a geometry final exam requires a comprehensive understanding of the subject, along with the ability to apply mathematical concepts and problem-solving skills. By reviewing and practicing key concepts, students can increase their chances of success and confidently approach the exam. Geometry is not only a subject in itself but also serves as a foundation for many other mathematical and scientific disciplines. Mastering geometry can open doors to a wide range of career opportunities and pave the way for a deeper understanding of the world around us.
Geometry a Final Exam Answers
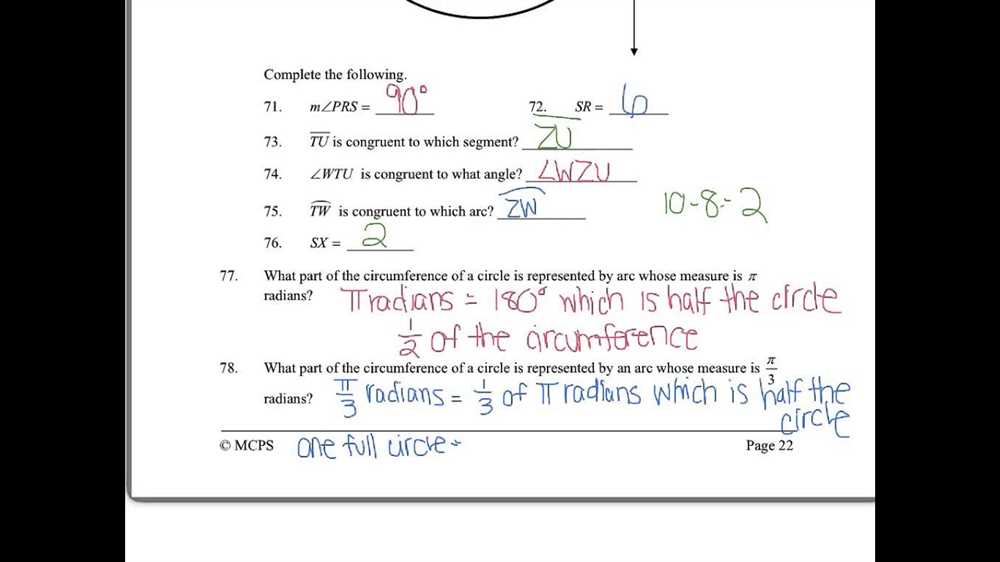
The final exam in geometry is an important assessment that evaluates a student’s understanding of the various concepts and principles learned throughout the course. It challenges students to apply their knowledge and problem-solving skills to solve complex geometric problems.
In order to excel in the final exam, it is crucial for students to have a strong grasp of key geometric concepts such as angles, lines, triangles, quadrilaterals, and circles. They should be able to identify different types of angles, understand the properties of lines and angles, apply the Pythagorean theorem to solve problems involving triangles, and calculate the area and circumference of circles.
Here are some possible answers to geometry final exam questions:

- Question 1: Calculate the measure of angle A in the triangle below.
- Answer: To find the measure of angle A, we can use the fact that the sum of the angles in a triangle is 180 degrees. Given that angles B and C are 45 degrees each, we can subtract their sum from 180 to find angle A: A = 180 – (45 + 45) = 90 degrees.
- Question 2: Find the perimeter of a rectangle with sides measuring 5 cm and 8 cm.
- Answer: The perimeter of a rectangle is found by adding the lengths of all its sides. In this case, the rectangle has two sides measuring 5 cm and two sides measuring 8 cm. Therefore, the perimeter is calculated as follows: P = 2(5 cm) + 2(8 cm) = 10 cm + 16 cm = 26 cm.
- Question 3: Calculate the area of a circle with a radius of 6 cm.
- Answer: The formula to calculate the area of a circle is A = πr^2, where π is a constant approximately equal to 3.14 and r is the radius. Plugging in the values, we have: A = 3.14 × (6 cm)^2 = 3.14 × 36 cm^2 ≈ 113.04 cm^2.
By mastering these key concepts and practicing similar problems, students can feel confident and prepared for their geometry final exam. It is important to study diligently, review class notes and textbook materials, and seek clarification from teachers or classmates if any topics are unclear. With the right preparation and understanding, students can achieve success in their geometry final exam.
Understanding the Basics of Geometry
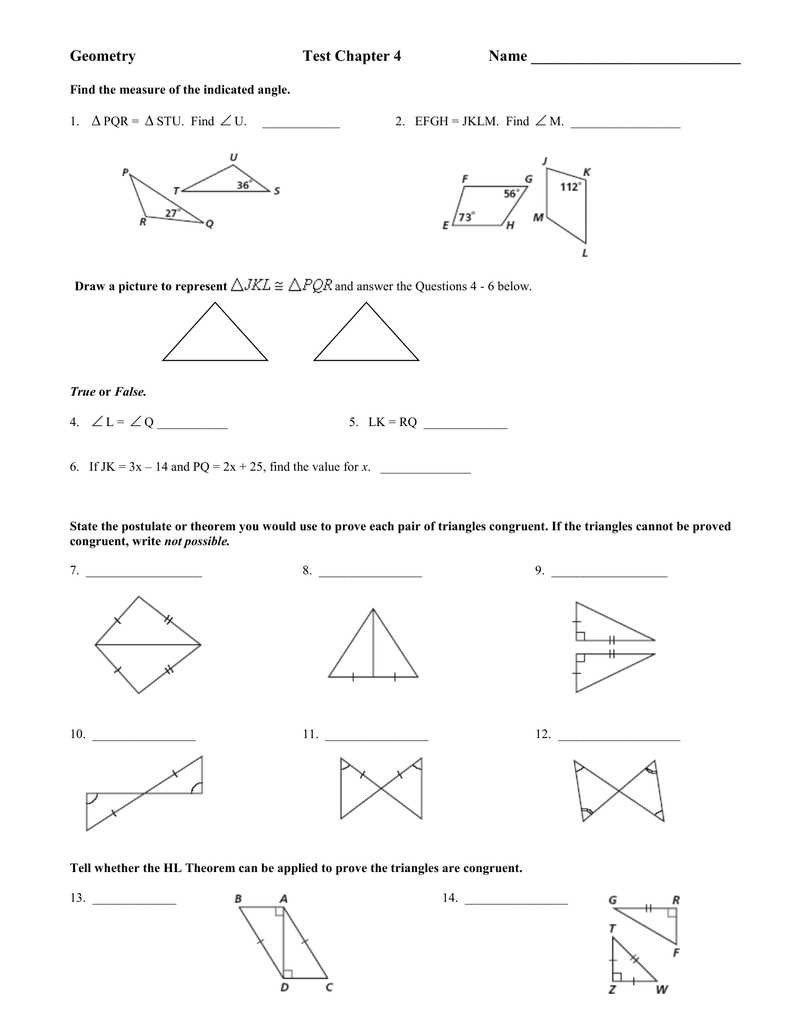
Geometry is a branch of mathematics that deals with the shapes, sizes, and properties of figures and spaces. It is a fundamental subject that is essential for understanding the world around us. In geometry, we study different types of shapes such as points, lines, angles, triangles, polygons, and circles, as well as their properties and relationships.
One of the key concepts in geometry is the notion of a point. A point is a specific location in space and is represented by a dot. In geometry, we use points to define other geometric objects. For example, a line is a straight path that extends indefinitely in both directions. It is made up of an infinite number of points. Another fundamental concept is an angle, which is formed by two rays that share a common endpoint. Angles can be classified as acute, obtuse, right, or straight, depending on their measures.
In addition to points, lines, and angles, triangles are also important in geometry. A triangle is a polygon with three sides and three angles. Triangles can be classified based on the lengths of their sides (equilateral, isosceles, or scalene) or the measures of their angles (acute, obtuse, or right). Another important topic in geometry is circles. A circle is a curve in which all points are equidistant from a fixed center point. Circles have unique properties such as diameter, radius, circumference, and area.
Overall, understanding the basics of geometry is crucial for various fields such as architecture, engineering, and physics. It provides us with the tools to analyze and solve real-world problems involving shapes and spaces. Whether you’re building a bridge or studying the motion of planets, geometry plays a significant role in our daily lives. By studying and mastering the concepts of geometry, you will develop invaluable critical thinking and problem-solving skills that can be applied in numerous areas of study and profession.
Key Concepts in Geometric Shapes

In geometry, there are several key concepts that are fundamental to understanding geometric shapes. These concepts provide the foundation for exploring and analyzing various geometric figures and their properties.
1. Points: A point is a specific location in space, represented by a dot. It has no size or dimensions. Points are often used to define other geometric figures.
2. Lines: A line is a straight path that extends infinitely in both directions. It is made up of an endless number of points. Lines can be defined by two points or by a point and a direction, and they have no thickness or width.
3. Line segments: A line segment is a part of a line with two endpoints. It has a definite length and can be measured. Line segments are often used to construct and analyze larger geometric figures.
4. Angles: An angle is formed by two rays with a common endpoint, called the vertex. Angles can be measured in degrees or radians and are used to describe the amount of rotation between two intersecting lines or line segments.
5. Triangles: A triangle is a polygon with three sides and three angles. It is one of the most basic geometric shapes. Triangles can be classified based on the lengths of their sides (equilateral, isosceles, or scalene) or the measures of their angles (acute, obtuse, or right).
6. Circles: A circle is a closed curve consisting of all points in a plane that are equidistant from a fixed center point. Circles have a radius (the distance from the center to any point on the circle) and a diameter (twice the radius). They are used in many geometric constructions and calculations.
7. Polygons: A polygon is a closed figure with straight sides. It can have any number of sides, but it must have at least three. Polygons are classified based on the number of sides they have (triangle, quadrilateral, pentagon, hexagon, etc.) and their angles (regular or irregular).
These key concepts form the building blocks for understanding geometric shapes and their properties. By mastering these concepts, students can solve problems involving geometric figures, perform calculations, and make connections to other areas of mathematics and real-world applications.
Solving Geometric Problems using Formulas
In the field of geometry, formulas play a crucial role in solving various problems. These formulas provide us with a systematic approach to finding the solutions we need. By understanding and applying the appropriate formulas, we can determine the measurements of angles, sides, areas, and volumes of different geometric figures.
One commonly used formula in geometry is the Pythagorean theorem. This theorem helps us find the length of one side of a right triangle when the lengths of the other two sides are known. The formula states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. By rearranging this formula, we can solve for the missing side length.
Example: Given a right triangle with side lengths of 3 units and 4 units, we can use the Pythagorean theorem to find the length of the hypotenuse. Applying the formula, we have (3^2) + (4^2) = (c^2), where c represents the length of the hypotenuse. Simplifying the equation, we get 9 + 16 = c^2, or 25 = c^2. Taking the square root of both sides, we find that c = 5 units. Therefore, the length of the hypotenuse is 5 units.
Another important formula in geometry is the formula for finding the area of a rectangle. This formula states that the area is equal to the product of the length and width of the rectangle. By substituting the given values into this formula, we can easily calculate the area.
- Example: If the length of a rectangle is 6 units and the width is 4 units, we can find the area by using the formula A = length × width. Plugging in the values, we get A = 6 units × 4 units = 24 square units. Therefore, the area of the rectangle is 24 square units.
By familiarizing ourselves with these and other formulas, we can confidently approach geometric problems and arrive at accurate solutions. It is important to remember that using the correct formula for the given problem is essential for obtaining the correct answer. Through practice and understanding, we can become proficient in using formulas to solve various geometric problems.
Exploring Relationships between Angles
In geometry, angles play a crucial role in understanding the relationships between different lines, rays, and segments. By examining the measures and positions of angles, we can uncover important properties and connections that exist within geometric figures.
Adjacent angles: When two angles share a common vertex and a common side, they are called adjacent angles. The sum of their measures is equal to the measure of the larger angle formed by their non-shared sides.
Example: If angle A and angle B are adjacent angles, and angle A measures 40 degrees while angle B measures 60 degrees, then the larger angle formed by their non-shared sides measures 100 degrees (40 + 60 = 100).
Vertical angles: Vertical angles are formed by two intersecting lines and are opposite to each other. They have equal measures, meaning that if angle A measures x degrees, then the vertical angle opposite to it, angle B, will also measure x degrees.
Example: If angle A and angle B are vertical angles, and angle A measures 70 degrees, then angle B will also measure 70 degrees.
Complementary angles: When the sum of the measures of two angles is equal to 90 degrees, they are called complementary angles. Each angle is said to be the complement of the other.
Example: If angle A and angle B are complementary angles, and angle A measures 40 degrees, then angle B will measure 50 degrees (90 – 40 = 50).
Supplementary angles: If the sum of the measures of two angles is equal to 180 degrees, they are referred to as supplementary angles. Each angle is considered the supplement of the other.
Example: If angle A and angle B are supplementary angles, and angle A measures 100 degrees, then angle B will measure 80 degrees (180 – 100 = 80).
By understanding these relationships between angles, we can solve various geometry problems, determine unknown angles, and analyze the properties of geometric shapes more effectively.
Properties of Triangles and Quadrilaterals
In geometry, triangles and quadrilaterals are two-dimensional shapes with certain properties. These properties help us classify and analyze these shapes, and they also allow us to solve various mathematical problems and prove geometric theorems.
Triangles: Triangles are three-sided polygons. They have several important properties that can be used to solve problems involving angles, sides, and areas. Some key properties of triangles include:
- Interior Angles: The sum of the interior angles of a triangle is always 180 degrees.
- Exterior Angles: The sum of the exterior angles of a triangle is always 360 degrees.
- Pythagorean Theorem: In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
- Triangle Inequality: The sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
Quadrilaterals: Quadrilaterals are four-sided polygons. They have their own set of properties that can be used to classify and analyze them. Some important properties of quadrilaterals include:
- Interior Angles: The sum of the interior angles of a quadrilateral is always 360 degrees.
- Parallelogram Properties: Opposite sides of a parallelogram are equal in length and parallel to each other. Opposite angles are also equal in measure.
- Rectangle Properties: A rectangle is a special type of parallelogram where all angles are right angles and opposite sides are equal in length.
- Square Properties: A square is a special type of rectangle where all sides are equal in length.
- Rhombus Properties: A rhombus is a special type of parallelogram where all sides are equal in length.
Understanding the properties of triangles and quadrilaterals is essential in geometry as it lays the foundation for more advanced concepts. These properties provide us with the necessary tools to solve problems, make deductions, and explore the fascinating world of shapes and their mathematical relationships.