
Are you currently studying geometry and preparing for an upcoming test? Are you looking for a way to assess your understanding of the material covered in Chapter 1? Look no further! In this article, we will provide you with a practice test designed to test your knowledge of the fundamental concepts taught in the first chapter of geometry.
Geometry is a branch of mathematics that deals with the properties, measurement, and relationships of points, lines, angles, surfaces, and solids. In Chapter 1, you would have learned about the basic building blocks of geometry, such as points, lines, and planes, as well as concepts like angles, segments, and rays.
This practice test will include a variety of multiple-choice and short-answer questions that cover different topics from Chapter 1. By taking this test, you will be able to gauge your comprehension of the material and identify any areas that may require further review or study. So grab a pencil and a piece of paper, as we dive into this Geometry Chapter 1 Practice Test!
Chapter 1: Geometry Practice Test

Welcome to the practice test for Chapter 1 of Geometry! This test will assess your understanding of the fundamental concepts and principles covered in this chapter. It is designed to prepare you for the upcoming assessments and help you reinforce your knowledge of the subject.
Before you begin the test, make sure you have reviewed the material thoroughly and taken the time to practice solving problems related to the topics discussed in Chapter 1. This will ensure that you are well-prepared and confident in your abilities to answer the questions correctly.
Test Format
The practice test consists of multiple-choice questions, true/false statements, and problem-solving exercises. It covers various topics such as points, lines, planes, angles, and geometric proofs. You will be asked to identify geometric figures, calculate measurements, and apply theorems and postulates to solve problems.
- Multiple-choice questions: Choose the correct answer from the given options.
- True/false statements: Determine whether the statement is true or false.
- Problem-solving exercises: Apply your knowledge and problem-solving skills to solve geometry-related problems.
To maximize your learning experience, it is recommended to attempt all the questions on your own before referring to the answer key. Take your time, read each question carefully, and think critically before choosing your answer. Remember to show your work for problem-solving exercises to receive partial credit, if applicable.
Once you have completed the practice test, compare your answers with the provided answer key to assess your performance and identify areas for further improvement. Take note of any mistakes or misconceptions and revisit the corresponding topics for clarification.
Good luck with your practice test! Remember to stay focused, utilize your problem-solving skills, and apply the concepts you have learned in Chapter 1 of Geometry. With sufficient practice and understanding, you will be well-prepared for success in this subject.
Basic Geometric Concepts
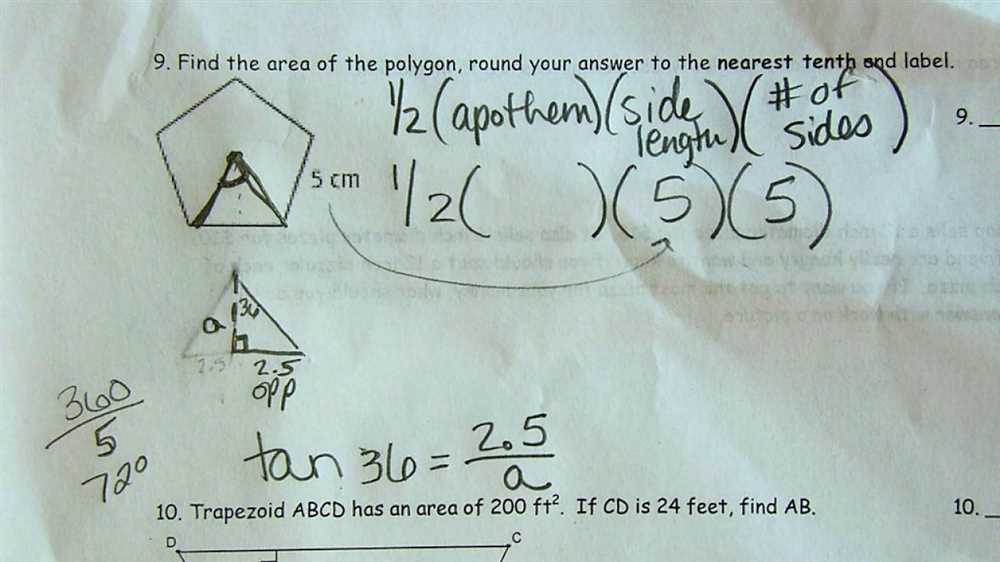
Understanding basic geometric concepts is essential for studying and applying geometry. These concepts serve as building blocks for more advanced topics in the field. By familiarizing ourselves with these fundamental ideas, we can develop a solid foundation in geometry and enhance our problem-solving skills.
One of the first concepts in geometry is that of a point. A point is a location in space that has no size or dimension. It is usually represented by a small dot. Points are important because they serve as the starting point for constructing other geometric objects such as lines and shapes.
Lines are another crucial concept in geometry. A line is defined as a straight path that extends infinitely in both directions. It has no endpoints and can be represented with a straight arrow or by specifying two points on the line. Lines are used to establish relationships between points and are the basis for many geometric theorems and formulas.
Line segments are portions of a line that consist of two endpoints and all the points in between. They have a specific length and can be measured. Line segments are often used to define the sides of polygons and are the building blocks for other geometric shapes.
- Rays are similar to lines but have only one endpoint and extend infinitely in one direction.
- Angles are formed by two rays that share a common endpoint called the vertex. Angles are measured in degrees and can range from 0 to 360.
- Circles are a set of points equidistant from a fixed center point. They are often used in geometry to represent curves and can be described by their radius or diameter.
Understanding these basic geometric concepts allows us to accurately describe and analyze the properties and relationships of geometric objects. By applying these concepts, we can solve problems, prove theorems, and explore the fascinating world of geometry.
Lines and Angles
In geometry, lines and angles are fundamental concepts that help us understand the properties and relationships between different geometric figures.
A line is a straight, infinitely long set of points that extends in both directions. It has no thickness or width. Lines are represented by a straight line with two arrowheads on each end, indicating that it extends infinitely.
Angles, on the other hand, are formed by two rays that share a common endpoint, called the vertex. The rays are the two sides of the angle. Angles are measured in degrees and can range from 0 to 360 degrees. A right angle measures exactly 90 degrees.
Lines and angles have several important properties and relationships. Two lines that never intersect are called parallel lines. Parallel lines have the same slope but different y-intercepts. If two lines intersect, the angles opposite each other, called vertical angles, are congruent or equal in measure.
Angles can also be classified based on their measure. An acute angle measures less than 90 degrees, while an obtuse angle measures more than 90 degrees but less than 180 degrees. A straight angle measures exactly 180 degrees, and a reflex angle measures more than 180 degrees but less than 360 degrees.
Key Terms:

- Line
- Angle
- Vertex
- Parallel lines
- Vertical angles
- Acute angle
- Obtuse angle
- Straight angle
- Reflex angle
Triangles
A triangle is a polygon with three sides and three angles. It is one of the most basic and fundamental shapes in geometry. Triangles are used extensively in various fields such as architecture, engineering, and physics due to their simplicity and versatility.
Types of Triangles:
- Equilateral Triangle: An equilateral triangle has three equal sides and three equal angles. Each angle measures 60 degrees.
- Isosceles Triangle: An isosceles triangle has two equal sides and two equal angles. The remaining angle is different and is known as the base angle.
- Scalene Triangle: A scalene triangle has no equal sides or angles. All three sides and angles are different.
- Right Triangle: A right triangle has one angle measuring 90 degrees. The side opposite the right angle is called the hypotenuse.
- Obtuse Triangle: An obtuse triangle has one angle greater than 90 degrees.
- Acute Triangle: An acute triangle has all three angles measuring less than 90 degrees.
Triangles have various properties and formulas associated with them, such as the Pythagorean theorem, which relates the sides of a right triangle. They also have unique properties when it comes to angles, diagonals, and area calculations.
Understanding the different types of triangles and their properties is essential in geometry as it serves as the foundation for more complex concepts and calculations involving polygons and 3D shapes.
Congruence and Similarity
In geometry, congruence and similarity are two important concepts that deal with comparing and classifying geometric figures. These concepts allow us to determine the relationships between different figures and understand their properties.
Congruence: Two figures are congruent if they have the same shape and size. In other words, all corresponding angles are equal, and all corresponding sides have the same length. Congruence is denoted by the symbol ≅. When we say that two triangles are congruent, for example, it means that all corresponding angles and sides of the triangles are equal.
Similarity: Two figures are similar if they have the same shape but not necessarily the same size. Similar figures have proportional corresponding sides, meaning that the ratio of the lengths of corresponding sides is the same. Similarity is denoted by the symbol ~. When we say that two triangles are similar, it means that the corresponding angles of the triangles are equal and the corresponding sides are in proportion.
In order to determine whether two figures are congruent or similar, we can use different methods such as angle-angle-angle (AAA), side-angle-side (SAS), or side-side-side (SSS) congruence criteria. For similarity, we can use the angle-angle (AA) or side-side-side (SSS) similarity criteria.
Properties of Congruent and Similar Figures:
- Congruent figures have equal area and perimeter.
- Corresponding angles and sides of congruent figures are equal.
- Similar figures have proportional corresponding sides.
- Corresponding angles of similar figures are equal.
- The ratio of the areas of similar figures is equal to the square of the ratio of their corresponding side lengths.
Understanding congruence and similarity is essential in geometry as it helps us solve various problems and make accurate measurements. These concepts form the foundation for many geometric proofs and can be applied to real-life situations such as scaling models, map projections, and architectural designs.
Quadrilaterals
Quadrilaterals are polygons with four sides. They are a fundamental concept in geometry and are used to classify and study different shapes. Understanding the properties and characteristics of quadrilaterals is essential in solving geometry problems and real-life applications.
There are various types of quadrilaterals, each with its own unique properties. Some common types of quadrilaterals include:
- Rectangle: A rectangle is a quadrilateral with four right angles. Its opposite sides are equal in length.
- Square: A square is a special type of rectangle where all four sides are equal in length. It also has four right angles.
- Parallelogram: A parallelogram is a quadrilateral with opposite sides that are parallel and equal in length.
- Rhombus: A rhombus is a parallelogram with all sides equal in length.
- Trapezoid: A trapezoid is a quadrilateral with one pair of parallel sides. The other two sides are not parallel.
Each type of quadrilateral has its own set of properties and formulas that can be used to calculate various measurements such as angles, side lengths, and area. Understanding these properties and formulas is essential in solving geometry problems involving quadrilaterals.
In addition to the basic types of quadrilaterals mentioned above, there are also more complex types such as kites and cyclic quadrilaterals. These shapes have their own unique properties and relationships between their angles and side lengths.
Circles
A circle is a two-dimensional figure that is perfectly round and is formed by all the points that are equidistant from a fixed point called the center. The distance from the center of the circle to any point on the circle is called the radius, and the longest distance across the circle passing through the center is called the diameter.
Circles have many important properties and characteristics. One of them is the circumference, which is the distance around the circle. The formula for the circumference of a circle is C = 2πr, where r is the radius. Another important property is the area, which is the amount of space inside the circle. The formula for the area of a circle is A = πr^2.
Circles can also be used to solve various mathematical problems and equations. For example, they are often used in geometry to find the measurements of angles, lengths of line segments, and areas of other shapes. They can also be used in real-life situations, such as calculating the area of a circular garden or the circumference of a tire.
In conclusion, circles are a fundamental concept in geometry and have many important properties and applications. Understanding circles and their properties is essential for solving mathematical problems and analyzing real-world situations.
Geometric Transformations
Geometric transformations are fundamental concepts in the study of geometry. They involve changing the position, orientation, and/or size of a shape without altering its underlying properties. These transformations are often used to explore and analyze the relationships between different geometric figures.
There are several types of geometric transformations, including translations, rotations, reflections, and dilations. Each type of transformation has its own distinct characteristics and rules. Understanding these transformations is crucial for solving geometric problems and proving geometric theorems.
Translations involve moving a shape along a straight line without changing its orientation or size. In other words, every point of the shape is shifted by the same amount and in the same direction. Translations are often described using coordinates and vectors.
Rotations involve spinning a shape around a fixed point called the center of rotation. Each point of the shape is rotated by the same angle in the same direction. Rotations can be clockwise or counterclockwise and are usually described using angles and trigonometric functions.
Reflections involve flipping a shape across a line of reflection, also known as the mirror line. Every point of the shape is reflected across the line, resulting in a mirror image. Reflections can be described using reflection matrices or geometric constructions.
Dilations involve enlarging or shrinking a shape by a scale factor, which determines the change in size. The center of dilation is a fixed point, and each point of the shape is scaled by the same factor. Dilations can be described using ratios or transformation matrices.
By understanding and applying these geometric transformations, mathematicians and scientists can solve challenging problems and make important discoveries in fields such as architecture, engineering, computer graphics, and physics.