
Geometry Chapter 7 focuses on the properties and relationships of polygons. In this review, we will cover the key concepts and formulas that will be tested in the upcoming exam. By understanding the properties of polygons, you will be able to solve problems involving angles, sides, and diagonals.
One of the main topics of this chapter is the classification of polygons by the number of sides they have. We will discuss the properties of triangles, quadrilaterals, pentagons, hexagons, and other polygons. Understanding these properties will help you determine the type of polygon and its characteristics.
Another important concept in this chapter is the calculation of interior and exterior angles of polygons. We will review the formulas for finding the sum of interior angles, as well as the measurement of each interior angle for different polygons. Additionally, we will explore how to find the measure of exterior angles and the relationship between interior and exterior angles.
In addition to angles, we will also discuss the properties of sides and diagonals. By understanding the relationships between the sides and diagonals of polygons, you will be able to determine their lengths and solve various geometric problems. We will review formulas for finding the length of a side, as well as the relationship between the sides and the diagonals of different polygons.
This Geometry Chapter 7 test review will provide you with a comprehensive overview of the key concepts and formulas covered in the chapter. By reviewing these concepts, you will be well-prepared for the upcoming exam and have a solid understanding of polygons and their properties.
Understanding Congruent Triangles
Congruent triangles are an important concept in geometry. When two triangles are congruent, it means that they have the same size and shape. In other words, all corresponding angles and sides of the triangles are equal. Understanding congruent triangles and the properties that determine congruence is crucial for solving geometric problems and proofs.
One property that determines congruence is angle-angle-side (AAS). If two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the triangles are congruent. This property can be used to prove the congruence of triangles in various geometric problems.
Another property that determines congruence is side-angle-side (SAS). If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the triangles are congruent. This property is useful for proving congruence when the angles are given.
Additionally, the side-side-side (SSS) property states that if all three sides of one triangle are congruent to all three sides of another triangle, then the triangles are congruent. This property is often used in geometric proofs to demonstrate congruence.
In conclusion, understanding congruent triangles and the properties that determine congruence is essential for solving geometry problems. Whether using the angle-angle-side, side-angle-side, or side-side-side property, recognizing congruent triangles and their corresponding angles and sides is a fundamental skill in geometry.
Definition of Congruent Triangles
In geometry, two triangles are said to be congruent if their corresponding sides and angles are equal. This means that if all the sides and angles of one triangle are congruent to the corresponding sides and angles of another triangle, then the two triangles are congruent. In other words, if triangle ABC is congruent to triangle DEF, it means that side AB is equal to side DE, side AC is equal to side DF, side BC is equal to side EF, angle A is equal to angle D, angle B is equal to angle E, and angle C is equal to angle F.
There are several ways to prove that two triangles are congruent. The most common methods of proving congruence are side-angle-side (SAS), side-side-side (SSS), angle-side-angle (ASA), and angle-angle-side (AAS). The SAS congruence theorem states that if two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the triangles are congruent. The SSS congruence theorem states that if all three sides of one triangle are congruent to the corresponding sides of another triangle, then the triangles are congruent. The ASA congruence theorem states that if two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the triangles are congruent. The AAS congruence theorem states that if two angles and a non-included side of one triangle are congruent to two angles and the corresponding non-included side of another triangle, then the triangles are congruent.
Knowing the definition and methods of proving congruent triangles is essential in geometry, as congruence is a fundamental concept that allows for the comparison and classification of triangles. By using congruence, we can determine if two triangles are identical in shape and size, and apply various geometric properties and theorems to solve problems involving congruent triangles.
SSS Postulate
The SSS Postulate, also known as Side-Side-Side Postulate, is one of the fundamental postulates used in geometry to determine congruence between two triangles. According to this postulate, if three sides of one triangle are congruent respectively to three sides of another triangle, then the two triangles are congruent.
The SSS Postulate can be used to prove congruence by comparing the lengths of the sides of the two triangles. In order to apply the SSS Postulate, it is necessary to identify the corresponding sides of the triangles and show that they are congruent. Once this is established, the congruence of the two triangles can be concluded.
In mathematical terms, the SSS Postulate is expressed as follows:
- If triangle ABC is congruent to triangle XYZ, and AB = XY, BC = YZ, and AC = XZ, then triangle ABC and triangle XYZ are congruent.
The SSS Postulate is based on the idea that if all three sides of a triangle are congruent to the corresponding sides of another triangle, then all corresponding angles will also be congruent. This postulate is a crucial tool in proving congruence in various geometric constructions and calculations.
SAS Postulate
The SAS Postulate, also known as the Side-Angle-Side Postulate, is a fundamental concept in geometry. It is used to prove that two triangles are congruent. In order for two triangles to be congruent, they must have exactly the same size and shape.
The SAS Postulate states that if two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the two triangles are congruent. In other words, if we have Triangle ABC and Triangle DEF, and we know that AB ≅ DE, BC ≅ EF, and ∠A ≅ ∠D, then we can conclude that Triangle ABC ≅ Triangle DEF.
This postulate is based on the fact that once two sides and the included angle of a triangle are known, the other angles and side lengths can be determined. It is a powerful tool that allows us to prove congruence between triangles and make deductions about their properties.
By using the SAS Postulate, we can solve various geometric problems, such as finding missing angles or side lengths in triangles. It is an important concept to understand and apply in geometry, as it forms the basis for many other postulates and theorems.
Overall, the SAS Postulate provides a systematic and logical approach to proving congruence between triangles. It allows us to make accurate conclusions about the size and shape of triangles based on the information given. Understanding and applying the SAS Postulate is crucial in the study of geometry.
ASA Postulate
The ASA postulate, also known as “angle-side-angle”, is a fundamental concept in geometry that allows us to determine if two triangles are congruent. Congruent triangles have exactly the same size and shape. The ASA postulate states that if two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the two triangles are congruent.
To apply the ASA postulate, we need to ensure that the two given angles and the included side form a unique triangle. This means that the included side must be between the two given angles. If the side is not included between the angles, then the ASA postulate cannot be used to prove congruence between the triangles.
When using the ASA postulate, it is important to pay attention to the order in which the angles and sides are given. The angle-side-angle order is essential for determining congruence. If the angles and sides are not in the correct order, the ASA postulate cannot be applied.
The ASA postulate is just one of several congruence postulates and theorems used in geometry. It provides a powerful tool for identifying congruent triangles and proving geometric relationships. By understanding the ASA postulate and its application, we can solve various problems and explore the fascinating world of geometry.
Applying Congruent Triangles
When studying geometry, one of the main concepts to understand is congruent triangles. Congruent triangles are two triangles that have the same shape and size. In other words, all corresponding sides and angles of congruent triangles are equal. This concept is crucial in solving various geometric problems and proofs.
One way to apply congruent triangles is through a method called side-angle-side (SAS) congruence. This method states that if two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the two triangles are congruent. This can be useful when proving that two triangles are congruent or when using congruent triangles to find unknown side lengths or angles.
To better understand the application of congruent triangles, let’s consider an example. Suppose we have triangle ABC and triangle DEF. If we know that side AB is congruent to side DE, side BC is congruent to side EF, and angle ABC is congruent to angle DEF, then we can conclude that triangle ABC is congruent to triangle DEF using SAS congruence.
By proving the congruence of two triangles, we can make certain deductions about their corresponding parts. For example, if we know that two triangles are congruent, we can conclude that all corresponding angles and sides are equal. This allows us to solve various geometric problems by applying the properties of congruent triangles.
In conclusion, understanding and applying congruent triangles is essential in geometry. It allows us to prove the congruence of triangles, find unknown side lengths or angles, and make deductions about corresponding parts. By mastering this concept, we can confidently solve geometry problems and further explore the fascinating world of geometry.
Using Congruent Triangles to Prove Congruent Parts
When studying geometry, one powerful tool that we have at our disposal is the use of congruent triangles to prove congruent parts. Congruent triangles are triangles that have the same shape and size, meaning that their corresponding sides and angles are equal. By proving that two triangles are congruent, we can then conclude that their corresponding parts (sides, angles, or both) are also congruent.
To prove congruent triangles, we can use different methods, such as side-side-side (SSS), side-angle-side (SAS), angle-side-angle (ASA), or angle-angle-side (AAS). These methods allow us to identify the congruent parts of two triangles by establishing relationships between their sides and angles.
For example, if we have two triangles ABC and DEF, and we know that AB is congruent to DE, BC is congruent to EF, and angle B is congruent to angle E, then we can use the SAS method to prove that the triangles are congruent. Once we establish the congruence of the triangles, we can conclude that angle A is congruent to angle D, angle C is congruent to angle F, and sides AC and DF are congruent as well.
Using congruent triangles to prove congruent parts is a valuable tool in geometry, as it allows us to make logical deductions based on established congruence. By understanding the properties of congruent triangles and utilizing different congruence criteria, we can confidently prove that corresponding parts of triangles are congruent, further expanding our knowledge of geometric relationships.
Solving Problems with Congruent Triangles
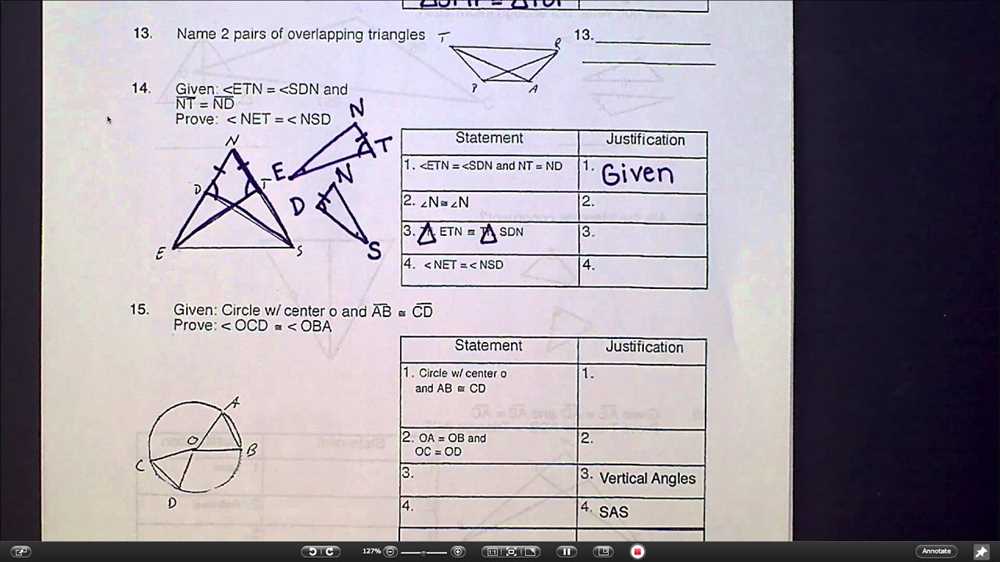
In geometry, congruent triangles have corresponding sides and angles that are equal in measure. This means that if two triangles are congruent, you can use their congruence to solve various types of problems. Whether you need to find missing side lengths, determine angles, or prove statements about geometric figures, congruent triangles can provide you with the necessary information.
One way to solve problems with congruent triangles is by using the Side-Side-Side (SSS) congruence criterion. If you know that all three pairs of corresponding sides in two triangles are equal in length, then you can conclude that the triangles are congruent. This allows you to use the measurements of one triangle to determine the measurements of the other. Additionally, the angles of congruent triangles are also equal in measure, so you can determine angle measurements using congruent triangles as well.
Another method for solving problems with congruent triangles is by using the Side-Angle-Side (SAS) congruence criterion. This criterion states that if two pairs of corresponding sides and the included angle in two triangles are equal in measure, then the triangles are congruent. This is useful for proving statements about triangles and determining missing side lengths or angle measures.
When solving problems with congruent triangles, it is important to carefully analyze the given information and use the appropriate congruence criterion. By applying the principles of congruent triangles, you can confidently solve a variety of geometry problems and make connections between different parts of a geometric figure.
Proving Triangles Similar
The concept of proving triangles similar is an important topic in geometry. This process involves demonstrating that two triangles have certain proportional relationships. By establishing these relationships, we can make conclusions about the corresponding angles and sides of the triangles.
One method of proving triangles similar is through the Angle-Angle (AA) Postulate. This postulate states that if two angles of one triangle are congruent to two angles of another triangle, then the triangles are similar. Using this postulate, we can compare the measurements of the angles in the two triangles and determine if they are congruent.
Another way to prove triangles similar is through the Side-Angle-Side (SAS) Congruence Theorem. This theorem states that if two sides of one triangle are proportional to two sides of another triangle and the included angle is congruent, then the triangles are similar. By comparing the lengths of the sides and the measurements of the included angle, we can determine if the triangles are similar.
In addition to these methods, there are several other theorems and postulates that can be used to prove triangles similar. These include the Side-Side-Side (SSS) Similarity Theorem, the Side-Angle-Side (SAS) Similarity Theorem, and the Angle-Side-Angle (ASA) Similarity Theorem. Each of these theorems and postulates provides a different set of conditions to establish the similarity of two triangles.
Proving triangles similar is a valuable tool in geometry, as it allows us to make deductions and draw conclusions about the relationships between angles and sides in different triangles. By understanding these methods and theorems, we can solve various geometry problems and explore the fascinating world of triangle similarity.