
Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles. One important concept in trigonometry is the law of sines, which allows us to find missing angles or sides in non-right triangles. To help students test their understanding of this fundamental law, teachers often use engaging activities like the Law of Sines Maze.
The Law of Sines Maze is a fun and challenging puzzle that requires students to apply the law of sines to find missing angles or sides and navigate through a maze. However, the maze can be quite daunting without a solution guide. That’s where the Law of Sines Maze Answer Key comes in handy. It provides step-by-step solutions to lead students through the maze, ensuring they practice their trigonometry skills and successfully reach the end.
With the Law of Sines Maze Answer Key, students can check their work, identify any mistakes, and gain a deeper understanding of how the law of sines applies to different scenarios. This answer key acts as a valuable learning tool, reinforcing the concepts and principles behind the law of sines while providing immediate feedback.
Law of Sines Maze Answer Key: A Comprehensive Guide
The Law of Sines is a crucial mathematical concept that helps solve problems involving triangles. The Law of Sines Maze Answer Key is a comprehensive guide that provides step-by-step solutions to various problems related to the Law of Sines. This guide aims to assist students and learners in understanding and applying the Law of Sines effectively.
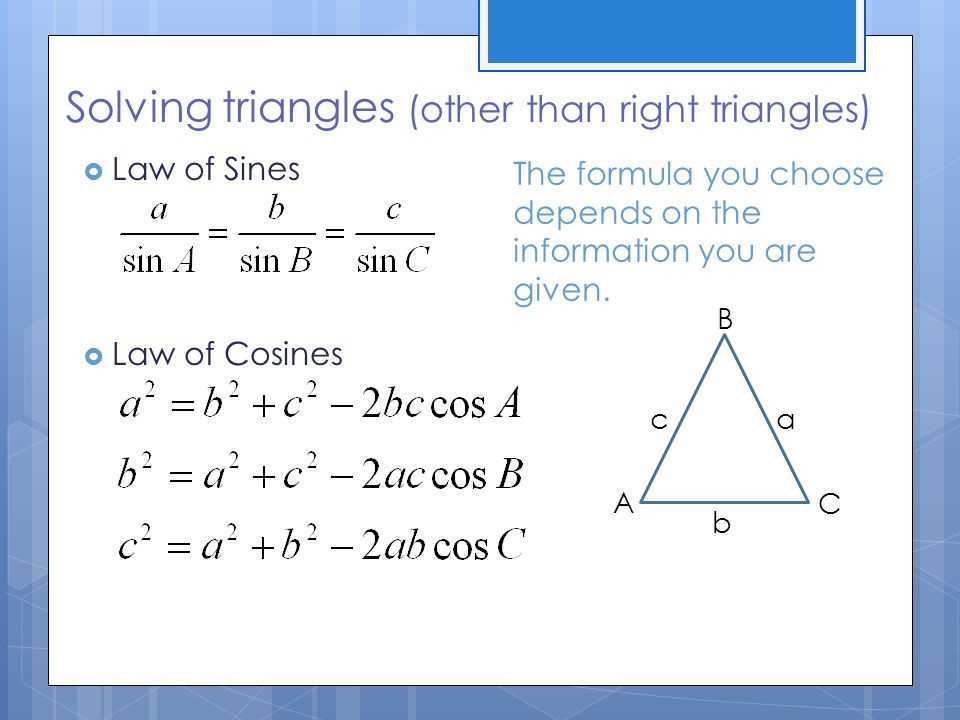
The Law of Sines states that the ratio of the length of a side of a triangle to the sine of its opposite angle is constant. In other words, it provides a relationship between the angles and sides of a triangle. This relationship can be used to find missing angles or sides in a triangle when certain other values are known.
In the Law of Sines Maze Answer Key, you will find detailed explanations for each step involved in solving problems. It begins with identifying the given values, such as angles or sides, and their respective measurements. Next, it demonstrates how to use the Law of Sines formula to set up an equation and solve for the unknown variable.
The answer key also includes examples of different types of problems, such as finding the lengths of sides, finding the measures of angles, or solving for unknown variables in complex triangles. It provides additional tips and tricks for tackling various scenarios and gives insight into common mistakes to avoid.
Overall, the Law of Sines Maze Answer Key serves as a valuable resource for students, educators, and anyone interested in mastering the Law of Sines. It offers a clear and concise guide to understanding the principles behind the Law of Sines and applying them to solve triangle-related problems successfully.
Understanding the Law of Sines
The Law of Sines is a fundamental concept in trigonometry that helps us solve triangles. It states that the ratio of the length of a side of a triangle to the sine of the opposite angle is constant for all three sides and their corresponding angles. This means that if we know the lengths of two sides and the measure of the included angle, we can find the length of the third side and the measures of the remaining angles.
The formula for the Law of Sines is written as follows:
a/sinA = b/sinB = c/sinC
where ‘a’, ‘b’, and ‘c’ are the lengths of the sides of the triangle, and ‘A’, ‘B’, and ‘C’ are the angles opposite to those sides, respectively.
By rearranging the formula, we can solve for different unknowns. For example, if we know the lengths of sides ‘a’ and ‘b’, and the measure of angle ‘A’, we can find the length of side ‘c’ by using the formula:
c = (a/sinA) * sinB
This formula allows us to find missing side lengths and angles in triangles, making it a valuable tool in solving trigonometric problems. The Law of Sines is especially useful when dealing with non-right triangles, as it can be used to solve for missing information without relying on the Pythagorean Theorem. By understanding and applying the Law of Sines, we can confidently solve a variety of trigonometric problems and navigate through the maze of triangle calculations.
Exploring the Law of Sines Maze
The Law of Sines is a trigonometric formula that relates the side lengths of a triangle to the sines of its angles. Understanding and applying this law is crucial in solving various types of problems in trigonometry. To help students grasp this concept, the Law of Sines Maze is a fun and interactive way to practice their skills and deepen their understanding.
The Law of Sines Maze is a worksheet or activity where students navigate through a maze by solving trigonometric equations using the Law of Sines. The maze is designed in such a way that the correct answers lead the students to the next step or the exit, while the wrong answers send them back or in the wrong direction.
Why is the Law of Sines Maze beneficial?
- Engaging: The maze format makes the learning experience more engaging and enjoyable for students. It adds an element of fun and challenge to the practice of solving trigonometric equations.
- Application: The maze allows students to apply the Law of Sines in a practical context. By solving equations to find the correct path, they develop problem-solving skills and reinforce their understanding of the law.
- Visual Representation: The maze provides a visual representation of how the Law of Sines can be applied to solve triangle-related problems. This visual aid helps students visualize the concepts and the relationships between angles and side lengths.
- Self-Assessment: As students navigate through the maze, they can self-assess their progress and understanding. If they reach the correct destination or exit, they know they have solved the equations correctly. If they encounter obstacles or dead ends, they can reevaluate their answers and try again.
The Law of Sines Maze is a valuable tool for educators to reinforce the concept of the Law of Sines and for students to practice and test their understanding of the law in a fun and interactive way.
Importance of the Answer Key
When it comes to solving complex mathematical problems, having the answer key can be of utmost importance. The answer key provides students with a step-by-step guide to solving the problem correctly, ensuring that they are on the right track. It allows them to check their work and identify any mistakes or misconceptions they may have had during the process. Additionally, the answer key serves as a valuable learning tool, as students can compare their answers with the correct ones and understand the reasoning behind each step. It helps them develop a deeper understanding of the concepts and strengthens their problem-solving skills.
The answer key also plays a crucial role in assessing student performance. Teachers can use it to grade assignments and provide feedback to students. It allows them to quickly identify areas where students may be struggling and provide targeted support. Moreover, the answer key can be used as a reference for future assessments, helping teachers identify common misconceptions among students and tailor their instruction accordingly. It ensures that students are learning the material effectively and enables teachers to make informed decisions about their teaching strategies.
In addition to its educational benefits, the answer key can also serve as a time-saving tool. Students can use it to quickly check their answers and move on to the next question without wasting time on unnecessary calculations. Furthermore, teachers can use the answer key to streamline the grading process, especially for large classes. It reduces the time required for manual grading and allows teachers to focus on providing meaningful feedback to their students.
In conclusion, the answer key plays a vital role in mathematics education. It promotes student learning, aids in assessing performance, saves time, and enables teachers to provide effective instruction. Whether it’s solving a maze with the Law of Sines or any other mathematical problem, the answer key serves as an invaluable resource for students and teachers alike.
Solving Trigonometric Problems with the Law of Sines
The Law of Sines is a valuable tool in solving trigonometric problems involving triangles. It allows us to find missing side lengths and angles in non-right triangles by using the relationship between the lengths of the sides and the sines of the opposite angles. This law is particularly useful when we know the lengths of two sides and the measure of one angle, or if we know the lengths of all three sides.
When using the Law of Sines, there are a few steps to follow. First, identify the information that is given in the problem, such as the lengths of sides or the measures of angles. Then, decide which formula to use based on the information given. If we know the lengths of two sides and the measure of one angle, we can use the formula a/sin(A) = b/sin(B) = c/sin(C), where a, b, and c are the lengths of the sides opposite angles A, B, and C, respectively. If we know the lengths of all three sides, we can use a/sin(A) = b/sin(B) = c/sin(C) = 2R, where R is the radius of the circumcircle of the triangle.
Once we have chosen the appropriate formula, we can use it to solve for the unknown quantities. To find a missing side length, we can rearrange the formula to isolate the desired side length. To find a missing angle, we can rearrange the formula to solve for the sine of the angle and then use inverse sine function to find the angle itself. It is important to be mindful of the units being used for angles, as the sine function is typically calculated using radians.
The Law of Sines provides a powerful tool for solving trigonometric problems involving triangles. By understanding and applying this law, we can confidently find missing side lengths and angles, allowing us to fully analyze and understand the properties of non-right triangles.
Step-by-Step Approach to Using the Law of Sines
When solving triangles and dealing with angles and sides, the Law of Sines is a useful tool. This law relates the ratio of the length of a side of a triangle to the sine of its opposite angle. Understanding how to use the Law of Sines can help you solve problems involving triangle trigonometry.
Here is a step-by-step approach to using the Law of Sines:
- Identify the given information: Before applying the Law of Sines, it is important to identify what information is given in the problem. This will typically include the measure of an angle and the length of its opposite side, or the measures of two angles and the length of a side.
- Determine the unknown: Next, determine what information is missing and needs to be solved for. This could be the measure of an angle, the length of a side, or both.
- Use the Law of Sines: Once the given information and unknowns are identified, you can apply the Law of Sines. The law states that the ratio of the length of a side to the sine of its opposite angle is constant. This can be written as: a/sin(A) = b/sin(B) = c/sin(C), where a, b, and c are the lengths of the sides and A, B, and C are the measures of the angles.
- Set up and solve an equation: To use the Law of Sines, set up an equation using the given information and the unknown. Cross-multiply and solve for the unknown.
- Check for ambiguous solutions: When solving for an angle using the Law of Sines, there can sometimes be two possible solutions. Make sure to check if both solutions are valid and make sense in the context of the problem.
- Compute the remaining unknowns: Once one unknown is solved for, you can use the Law of Sines again or apply other trigonometric relationships to find the remaining unknowns if needed.
By following this step-by-step approach, you can effectively use the Law of Sines to solve problems involving triangles and trigonometry. Practice and familiarity with the concept will make it easier to apply in various situations.
Common Mistakes and Solutions
When solving problems involving the law of sines in a maze format, many students make common mistakes that can hinder their progress. It is important to be aware of these mistakes and know how to overcome them. Below are some common mistakes and their solutions:
1. Incorrect application of the law of sines formula:
One common mistake is using the law of sines formula incorrectly. The formula states that the ratio of a side length to the sine of its opposite angle is constant. Make sure to correctly identify the sides and angles in the given problem and apply the formula accordingly. An incorrect application of the formula can lead to wrong answers.
2. Not considering the ambiguous case:

Another mistake is not considering the ambiguous case when there are multiple possible solutions. The law of sines can have two possible solutions for a given angle, depending on the given information. It is important to consider all possible solutions and choose the correct one based on the context of the problem. Ignoring the ambiguous case can result in an incomplete or incorrect solution.
3. Failing to convert angles to radians:
Many students forget to convert angles from degrees to radians when using the law of sines formula. The formula requires angles to be in radians, so it is crucial to convert them before applying the formula. Failing to do so can lead to significant errors in the calculations and ultimately the wrong answer.
4. Rounding errors:
Rounding errors can also cause mistakes in the final answer. The law of sines involves trigonometric calculations, which often require decimal answers. Make sure to round your answers appropriately and carry enough decimal places throughout the calculations to minimize rounding errors. Double-checking the accuracy of your rounded answers can help avoid unnecessary mistakes.
5. Incorrect unit conversions:
If the problem involves different units of measurement, such as converting from feet to meters or vice versa, it is important to perform the necessary unit conversions. Failing to convert units correctly can result in inconsistent or incorrect answers. Pay attention to the given units and carry out the appropriate conversions to ensure accurate solutions.
Tips and Tricks for Mastering the Law of Sines
When studying the Law of Sines, it is important to understand the fundamental concepts and principles behind this mathematical theorem. Here are some tips and tricks to help you master this topic:
1. Understand the Law of Sines: The Law of Sines states that in any triangle, the ratio of the length of a side to the sine of its opposite angle is constant. This can be written as: a/sin A = b/sin B = c/sin C, where a, b, and c are the lengths of the sides, and A, B, and C are the measures of the angles.
2. Memorize the Law of Sines formula: Being able to recall the formula is crucial when applying the Law of Sines. Make sure to memorize it and practice using it in various triangle scenarios.
3. Take note of the ambiguous case: The Law of Sines can have multiple solutions or no solution in certain cases. This occurs when the given information is not sufficient to uniquely determine a triangle. Pay attention to situations where there may be more than one possible triangle that satisfies the given conditions.
4. Use the Law of Sines in conjunction with other trigonometric identities: The Law of Sines is a powerful tool, but it can be even more effective when used in combination with other trigonometric identities, such as the Law of Cosines or the Pythagorean Identity. Understanding how these identities relate to each other can help you solve more complex triangle problems.
5. Practice solving a variety of triangle problems: The best way to master the Law of Sines is through practice. Solve a wide range of triangle problems using this theorem to enhance your understanding and problem-solving skills.
6. Don’t forget to convert angles to radians: When using the Law of Sines, make sure to convert any angle measurements given in degrees to radians. This will ensure accurate calculations and prevent errors.
7. Use real-life examples to apply the Law of Sines: Explore real-life situations where the Law of Sines can be applied, such as calculating distances or angles in navigation or surveying. This can help you understand the practical applications of this theorem.
By following these tips and tricks, you can develop a strong understanding of the Law of Sines and confidently solve various triangle problems.