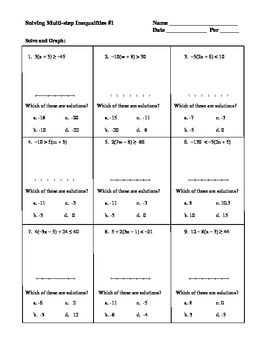
In Lesson 4, we focused on algebraic expressions and how to write them. Algebraic expressions are mathematical phrases that include one or more variables. They can also contain numbers, operations, and even exponents.
The purpose of this homework practice is to reinforce your understanding of writing algebraic expressions. In each exercise, you will be given a word problem, and you will need to translate it into an algebraic expression. This will require you to identify the key information, such as the variables, operations, and any given numbers.
Remember to carefully read each word problem and break it down step by step. Look for key words and phrases that indicate the mathematical operations or relationships involved. Then, use these clues to write the corresponding algebraic expressions.
By the end of this homework practice, you should feel more confident in your ability to write algebraic expressions. This skill is essential for solving equations and working with more advanced concepts in algebra. So, let’s get started and practice writing algebraic expressions!
Lesson 4 Homework Practice Algebra Write Expressions Answer Key
In this lesson, we will go over the answer key for the homework practice on algebraic expressions. These expressions involve variables and operations, and it is important to correctly write and simplify them.
1. Write the following phrase as an algebraic expression:
The sum of twice a number and six.
To write this as an expression, we can let the variable n represent the unknown number. The phrase “twice a number” can be written as 2n, and “the sum of” can be represented by the plus sign (+). So the algebraic expression is: 2n + 6.
2. Write the following phrase as an algebraic expression:
The product of a number and four, decreased by ten.
Let’s use the variable x to represent the unknown number. The phrase “the product of a number and four” can be written as 4x, and “decreased by ten” can be represented by the minus sign (-). So the algebraic expression is: 4x – 10.
3. Write the following phrase as an algebraic expression:
The quotient of a number and three, increased by five.
Using the variable y to represent the unknown number, the phrase “the quotient of a number and three” can be written as y/3, and “increased by five” can be represented by the plus sign (+). So the algebraic expression is: y/3 + 5.
- Overall, it is important to carefully analyze the given phrase and accurately translate it into an algebraic expression.
- Variables are used to represent unknown numbers or quantities, and operations such as addition, subtraction, multiplication, and division are indicated by the appropriate signs.
- Simplifying the expressions will help in further problem-solving and mathematical calculations.
What is Algebra?
Algebra is a branch of mathematics that uses letters, symbols, and equations to represent and solve mathematical problems. It is a powerful tool for understanding and analyzing mathematical relationships and patterns. With algebra, we can solve equations, manipulate variables, and express complex relationships in a concise and systematic way.
In algebra, we use variables to represent unknown quantities. These variables can be represented by letters or symbols, and they allow us to generalize mathematical statements and formulas. By using variables, we can express relationships between quantities and solve equations to find the values of the variables.
Algebraic expressions are combinations of variables, constants, and operations such as addition, subtraction, multiplication, and division. These expressions can be simplified, manipulated, and evaluated to find their values. Algebraic equations, on the other hand, are statements that equate two algebraic expressions. Solving algebraic equations involves finding the values of the variables that make the equation true.
Algebra is not just about solving equations and simplifying expressions. It also helps us analyze real-world problems and make predictions. By using algebraic language and techniques, we can model and solve problems from various fields such as physics, economics, engineering, and computer science. Algebra provides us with a powerful set of tools to understand and describe the world around us.
Understanding Expressions in Algebra
Expressions in algebra are a fundamental concept that forms the basis of solving equations and inequalities. They are mathematical statements that consist of variables, constants, and operations. By understanding and correctly interpreting expressions, we can analyze and solve various mathematical problems.
Expressions can be as simple as a single variable, such as “x” or “y,” or they can be more complex with multiple variables and operations, such as “(3x + 2y) / 5.” Variables represent unknown quantities, while constants are known values. Operations like addition, subtraction, multiplication, and division are used to manipulate these variables and constants in order to solve mathematical problems.
For example: In the expression “2x + 5,” “2x” represents a variable multiplied by 2, and “5” is a constant. We can substitute different values for “x” and simplify the expression. If we substitute “x = 3,” the expression becomes “2(3) + 5,” which simplifies to “6 + 5” or “11.”
Expressions can also include parentheses to indicate the order of operations. For instance, in the expression “(4 + 2) * 3,” the addition inside the parentheses is done first, giving us “6 * 3” or “18.”
Understanding expressions in algebra is crucial for solving equations and inequalities. By manipulating and simplifying expressions, we can find solutions to various mathematical problems. Additionally, expressions help us analyze real-life situations and make predictions based on mathematical models. Whether it’s solving equations or modeling real-world scenarios, a strong understanding of expressions is essential in algebra.
The Importance of Homework Practice
Homework practice plays a crucial role in the academic development of students. It is an opportunity for them to reinforce what they have learned in class and apply it to real-world scenarios. By completing homework regularly, students can internalize and deepen their understanding of the subject matter.
Enhancing Knowledge Retention and Understanding
Homework practice allows students to actively engage with the material outside of the classroom setting. It gives them the chance to review and consolidate the concepts and skills they have learned. By working on assignments and exercises on their own, students can explore different approaches and problem-solving strategies, which enhances their critical thinking and analytical skills. Moreover, completing homework helps students retain information for an extended period, as it requires them to revisit and apply what they have previously learned.
Developing Responsibility and Time Management
Regular homework practice teaches students important life skills such as responsibility and time management. It requires them to manage their time effectively, prioritize tasks, and meet deadlines. By adhering to a homework schedule, students learn to be organized and develop a sense of discipline. These skills are transferable and can be beneficial in various aspects of their lives, including future academic endeavors and professional careers.
Preparing for Assessments and Evaluations
Homework practice serves as a valuable preparation tool for assessments and evaluations. Through homework, students can practice different types of questions and reinforce their understanding of the subject. It allows them to identify areas of weakness and seek additional help or clarification if needed. Additionally, completing homework regularly helps students build confidence in their abilities, which is essential when facing assessments or exams.
In conclusion, homework practice is essential for academic success. It helps students consolidate their knowledge, develop important skills, and prepare for assessments. By dedicating time and effort to complete their homework, students can improve their academic performance and overall understanding of the subject matter.
Common Mistakes to Avoid in Algebraic Expressions
In algebra, it is common for students to make mistakes when working with algebraic expressions. These mistakes can often lead to incorrect answers or confusion in solving equations. It is important to be aware of these common mistakes and take steps to avoid them.
1. Forgetting to Use Parentheses
One common mistake is forgetting to use parentheses when necessary. Parentheses are used to indicate the order of operations in algebraic expressions. This helps to clarify which operations should be done first. It is important to always use parentheses when needed to ensure accurate and clear expressions.
2. Combining Like Terms Incorrectly
An error often made in algebra is combining like terms incorrectly. Like terms are terms that have the same variables raised to the same power. When combining like terms, it is important to add or subtract the coefficients of these terms, while keeping the variables and exponents the same. This mistake can result in an incorrect simplified expression.
3. Misusing Negative Signs
Another mistake commonly made is misusing negative signs. Negative signs should be carefully placed in front of terms to indicate subtraction, while positive signs are often omitted to indicate addition. Misusing negative signs can lead to errors in the overall expression and incorrect solutions to equations.
4. Inconsistent Notation
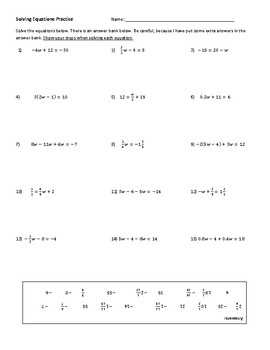
Using inconsistent notation in algebraic expressions is another common mistake. It is important to use the same notation throughout the expression, such as using either the exponent notation or the multiplication notation for exponents. Inconsistent notation can make it difficult to understand and solve the expression accurately.
By being aware of these common mistakes and actively avoiding them, students can improve their algebraic skills and accuracy in solving equations. Practice and understanding of these concepts will help ensure success in algebra.
Tips for Writing Algebraic Expressions
Writing algebraic expressions can be challenging, but with some practice and understanding of the key principles, you can become proficient in expressing mathematical relationships. Here are some tips to help you write algebraic expressions effectively:
- Identify the variables: Before you start writing an algebraic expression, it’s essential to identify the variables involved. Variables represent unknown quantities and are usually represented by letters such as x, y, or z. Make sure you understand what each variable represents in the context of the problem.
- Understand the operations: Algebraic expressions involve various operations such as addition, subtraction, multiplication, and division. Familiarize yourself with the mathematical symbols used for these operations (+, -, *, /) and understand their meanings. For example, the symbol “x” in an algebraic expression represents multiplication, not a variable.
- Translate word problems: Word problems can be tricky to translate into algebraic expressions. Take your time to read the problem carefully, identify the relevant information, and establish the relationship between the variables. Look for keywords that indicate specific operations, such as “sum,” “difference,” “product,” or “quotient.”
- Simplify whenever possible: Algebraic expressions can sometimes become complex, but it’s always a good practice to simplify them whenever possible. Combine like terms, distribute operations, and simplify fractions to make the expression more concise and easier to work with.
- Check your work: After writing an algebraic expression, it’s crucial to double-check your work to ensure accuracy. Plug in values for the variables and evaluate the expression to see if it matches the desired outcome. Checking your work can help you identify any mistakes or inconsistencies.
Remember, writing algebraic expressions requires practice and familiarity with mathematical concepts. Keep these tips in mind, and don’t hesitate to seek help or clarification if you’re unsure about any aspect of the problem. With time and effort, you’ll become more confident in expressing mathematical relationships using algebraic expressions.
Solving Algebraic Equations

Algebraic equations are mathematical statements that contain variables and operations. Solving these equations involves finding the values of the variables that make the equation true. This process is an essential component of algebra and is often used in various fields of science, engineering, and mathematics.
When solving algebraic equations, it is important to follow a systematic approach. The first step is to simplify the equation by combining like terms and applying the order of operations. This helps to eliminate any unnecessary complexity and makes the equation easier to solve. Next, use inverse operations to isolate the variable. Addition and subtraction can be undone by using the opposite operation, while multiplication and division can be undone by using their respective inverses.
An equation can have one or more solutions. In some cases, there may be no solution or infinitely many solutions. To determine the solution(s) of an equation, substitute the values of the variable back into the original equation and verify that it satisfies the equation. If the equation is true, the values are considered solutions.
In conclusion, solving algebraic equations is a fundamental skill in mathematics. It involves simplifying the equation, isolating the variable, and verifying the solution. Developing a strong understanding of algebraic equations is valuable in various academic and professional fields where problem-solving and critical thinking are necessary.
Step-by-Step Guide to Simplifying Algebraic Expressions
Algebraic expressions can often appear complex and intimidating, but with the right approach, they can be simplified and made more manageable. Here is a step-by-step guide to help you simplify algebraic expressions:
Step 1: Identify the Terms
The first step in simplifying an algebraic expression is to identify the terms. Terms are the individual parts of an expression separated by addition or subtraction. For example, in the expression 3x + 2y – 5z, the terms are 3x, 2y, and -5z.
Step 2: Combine Like Terms
Next, combine like terms by adding or subtracting them. Like terms have the same variable(s) raised to the same powers. For example, in the expression 3x + 2y – 5z, you can combine the like terms 3x and -5z to get 3x – 5z. The term 2y cannot be combined with any other terms because there are no other terms with the variable y.
Step 3: Simplify Using the Distributive Property
If the expression contains parentheses, apply the distributive property to simplify further. The distributive property states that multiplying a number by a sum or difference is the same as multiplying the number by each term within the parentheses and then combining the results. For example, in the expression 3(x + 2), you can use the distributive property to get 3x + 6.
Step 4: Combine Like Terms Again (if necessary)

If there are still multiple terms with the same variable(s) raised to the same powers, combine them again. Repeat step 2 until no more like terms can be combined.
Step 5: Simplify Further (if necessary)

If there are any additional operations, such as multiplication or division, apply them to simplify the expression even further. Follow the order of operations (PEMDAS/BODMAS) to ensure the correct simplification.
By following these steps, you can simplify even the most complex algebraic expressions and make them easier to work with. Remember to take your time and carefully apply each step to ensure an accurate simplification.