
In this article, we will dive into the answers for Practice B in Lesson 5.1 of Geometry. This lesson focuses on various geometric concepts such as angles, lines, and polygons. By understanding and applying these concepts, students will be able to solve problems and analyze different geometric figures.
The Practice B questions in Lesson 5.1 provide an opportunity for students to test their understanding of the material. The answers to these questions will help students gauge their progress and identify any areas where they may need additional practice or clarification. It is crucial to review and understand these answers to ensure a solid foundation in geometry.
Throughout Lesson 5.1, students will encounter questions that require them to identify and categorize different types of angles and lines. They will also explore the properties and characteristics of polygons, such as triangles and quadrilaterals. By applying the principles and formulas taught in this lesson, students will be able to determine the measures of angles and sides in various geometric figures.
By reviewing the answers to Practice B in Lesson 5.1 of Geometry, students can strengthen their understanding of the subject and improve their problem-solving skills. It is essential to practice regularly and seek assistance or clarification when needed to ensure success in geometry.
Overview of Lesson 5.1 Practice B Geometry
In Lesson 5.1 Practice B Geometry, we will be exploring different concepts and solving problems related to angles in geometry.
The lesson begins with a review of basic angle relationships, such as complementary and supplementary angles. We will also learn about vertical angles and how to identify them in geometric figures.
We will then move on to solving problems involving angle measures. This will involve applying our understanding of angle relationships and using algebraic expressions to find missing angle measures.
Throughout the lesson, we will be working on a variety of practice problems to reinforce our understanding of the concepts. These problems will require us to apply the properties of angles and use deductive reasoning to find the solutions.
By the end of Lesson 5.1 Practice B Geometry, we should have a solid understanding of angle relationships and be able to confidently solve problems involving angles in geometric figures. This knowledge will be essential as we continue to explore more advanced geometrical concepts in future lessons.
Understanding the Practice B Geometry Answers
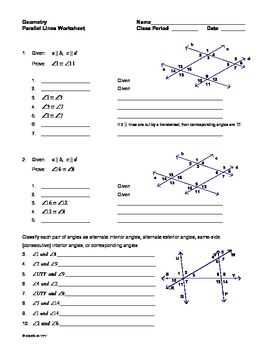
When studying geometry, it is crucial to practice solving different problems to strengthen our understanding of the concepts. Practice B in geometry provides students with a set of exercises that test their knowledge and skills in various areas such as angles, lines, triangles, polygons, and circles.
The Practice B Geometry Answers are a valuable resource for students to check their work and assess their understanding of the material. These answers provide step-by-step solutions and explanations, helping students identify any mistakes they made and learn from them. By using these answers, students can gain confidence in their abilities and improve their problem-solving skills.
The Practice B Geometry Answers can also serve as a study guide for students preparing for exams or quizzes. By reviewing the correct solutions, students can reinforce their understanding of the concepts and identify any areas where they may need additional practice or clarification. Additionally, these answers can be used as a reference for students who are struggling with a particular concept, helping them overcome challenges and gain a deeper understanding of the topic.
It is important to note that while the Practice B Geometry Answers can be a helpful tool, it is essential for students to fully understand the concepts and principles behind the solutions. Simply relying on memorizing the answers without comprehending the underlying concepts will not lead to long-term success in geometry. Therefore, it is crucial for students to actively engage with the material, ask questions, and seek additional resources or assistance when needed.
In conclusion, the Practice B Geometry Answers provide students with a valuable resource to enhance their understanding of geometry. By utilizing these answers as a study tool and learning from the step-by-step solutions, students can strengthen their problem-solving skills and gain confidence in their abilities. However, it is important to remember that true understanding comes from actively engaging with the material and seeking deeper comprehension of the underlying concepts.
Practical Application of Lesson 5.1 Concepts
In lesson 5.1, we learned about various geometric concepts such as line segments, rays, and angles. These concepts have practical applications in our everyday lives, from measuring distances to understanding the angles of objects.
One practical application of lesson 5.1 concepts is in construction and architecture. Architects and engineers use line segments and angles to design and build structures. They use measurements and angles to ensure that buildings are stable and structurally sound.
Measurement of angles is also necessary in various fields such as navigation. Pilots and sailors use angles to determine their position and navigate through the air or water. Understanding angles helps them plot a course and avoid obstacles.
Another practical application of lesson 5.1 concepts is in the field of computer graphics and animation. Animators use concepts such as line segments and vectors to create realistic animations. They manipulate angles and dimensions to give the illusion of movement and depth in their creations.
In conclusion, the concepts learned in lesson 5.1 have practical applications in various fields such as construction, navigation, and computer graphics. Understanding line segments, rays, and angles allows us to measure distances, design structures, navigate, and create realistic animations.
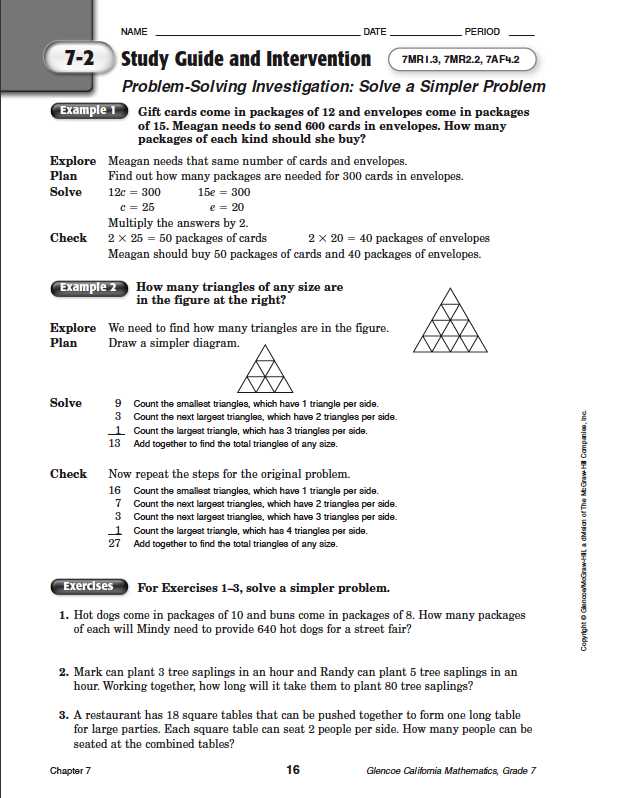
Step-by-Step Solution to Practice B Geometry Problems
In this article, we will provide a step-by-step solution to the Practice B Geometry problems. These problems are designed to test your understanding of various geometric concepts and your ability to apply them in problem-solving situations. By following the steps outlined below, you will be able to approach and solve these problems with confidence.
Step 1: Read the problem carefully
Before attempting to solve the problem, take the time to carefully read and understand what is being asked. Pay attention to any given information, constraints, or diagrams that may be provided. This will help you identify what concepts or formulas are relevant to the problem and how to proceed.
Step 2: Identify known and unknown quantities
Once you have a clear understanding of the problem, identify the known quantities and the unknown quantity you are trying to find. List them out and make sure you have all the necessary information to solve the problem. This step will help you organize your thoughts and determine the approach you need to take.
Step 3: Choose an appropriate strategy
Based on the known and unknown quantities, choose an appropriate strategy to solve the problem. This could involve applying a specific formula or theorem, utilizing geometric properties, or using algebraic techniques. Make sure to choose a strategy that aligns with the given information and will lead you toward finding a solution.
Step 4: Perform the necessary calculations
Once you have chosen your strategy, perform the necessary calculations to find the solution. This may involve simplifying expressions, solving equations, or applying geometric properties. Be sure to show all your work and clearly explain each step to demonstrate your understanding of the problem-solving process.
Step 5: Check your answer
After obtaining a solution, it is crucial to check your answer for accuracy. Review the problem to ensure that your solution aligns with the given information and satisfies any constraints or conditions. Additionally, consider if your answer makes logical sense in the context of the problem. If any errors are found, retrace your steps and correct them accordingly.
By following these steps, you will be able to approach and solve Practice B Geometry problems effectively and accurately. Remember to practice regularly to strengthen your understanding of geometric concepts and problem-solving skills.
Common Mistakes to Avoid in Lesson 5.1 Practice B Geometry
In Lesson 5.1 Practice B of Geometry, there are several common mistakes that students often make. These mistakes can prevent them from fully understanding and applying the concepts being taught. It is important to be aware of these mistakes and avoid them to ensure success in this lesson.
One common mistake is confusing the different types of angles. In this lesson, students are introduced to various angle measures such as complementary, supplementary, and vertical angles. It is important to understand the definitions and properties of each type of angle to correctly identify and solve problems involving them. Confusing these angles can lead to incorrect answers and misunderstandings.
Another mistake to avoid is not properly labeling and identifying geometric figures. In Lesson 5.1 Practice B, students work with different types of polygons and quadrilaterals. It is essential to label the sides and angles of these figures correctly to accurately solve problems related to their properties and attributes. Incorrectly labeling figures can result in incorrect calculations and solutions.
Additionally, it is important to pay attention to the wording and language used in the problems. Misinterpreting the problem statement can lead to incorrect assumptions and errors in the solution process. Students should carefully read and understand the problem before attempting to solve it, ensuring they accurately represent the given information and know what is being asked.
- Confusing angle measures: Make sure to understand the definitions and properties of complementary, supplementary, and vertical angles.
- Labeling figures incorrectly: Take the time to correctly label the sides and angles of polygons and quadrilaterals.
- Misinterpreting the problem: Read and understand the problem statement carefully, ensuring you know what is being asked.
By avoiding these common mistakes, students can enhance their understanding of geometry concepts and improve their problem-solving skills. It is important to practice diligently and seek clarification whenever necessary to ensure success in Lesson 5.1 Practice B and beyond.
Tips and Tricks for Mastering Lesson 5.1 Practice B Geometry
In Lesson 5.1 Practice B in Geometry, you will explore the concept of angle relationships and parallel lines. This lesson is crucial as it lays the foundation for understanding the properties of parallel lines and transversals. Here are some tips and tricks to help you master this lesson:
- Understand the types of angles: Before diving into the practice problems, make sure you have a clear understanding of the different types of angles that exist. Refresh yourself on terms such as complementary, supplementary, vertical, alternate interior, alternate exterior, and corresponding angles.
- Identify parallel lines: Look for lines that never intersect in the given figures or diagrams. These lines will be parallel to each other. Parallel lines have several important properties, such as the fact that corresponding angles are congruent.
- Use angle relationships: Utilize your knowledge of angle relationships to solve the practice problems. For example, if two angles are vertical angles, you know they are congruent. If two angles are corresponding angles, you can use that information to find other missing angles.
- Look for transversals: Keep an eye out for lines that intersect the parallel lines. These lines are called transversals and create additional angles that you can use to find missing measures. Remember that corresponding angles, alternate interior angles, and alternate exterior angles are congruent when a transversal intersects parallel lines.
- Label angles and lines: It can be helpful to label the angles and lines in the given figures before attempting to solve the problems. This way, you can clearly see which angles are congruent or supplementary, and which lines are parallel or intersected by the transversal.
By understanding the different types of angles, identifying parallel lines, using angle relationships, looking for transversals, and labeling the figures, you will be well-equipped to tackle the practice problems in Lesson 5.1 Practice B Geometry. Remember to take your time, draw clear diagrams, and apply the concepts you have learned.
Additional Resources for Further Practice and Study
In order to solidify your understanding of the concepts covered in Lesson 5 1 practice b geometry answers, it is important to engage in additional practice and study. Below are some resources that can help you further enhance your knowledge:
- Online Practice Exercises: Many websites offer free practice exercises and quizzes specifically tailored to geometry. These exercises can help you reinforce the concepts learned in the lesson and identify any areas where you may need further clarification.
- Geometry Textbooks: Consider consulting geometry textbooks for additional practice problems and explanations. These textbooks often include step-by-step solutions to help you understand the concepts better.
- Tutoring Services: If you are struggling with the material, consider seeking the help of a tutor who specializes in geometry. A tutor can provide personalized instruction and guidance to ensure your understanding of the subject.
- Geometry Apps: There are several mobile apps available that can make learning geometry more interactive and engaging. These apps often include interactive quizzes, puzzles, and visualizations to help you grasp the concepts more effectively.
Remember, practice is key when it comes to mastering geometry. By utilizing these additional resources, you can further solidify your understanding and improve your problem-solving skills in geometry. Good luck!