
In geometry, Lesson 7.4 is focused on finding the answers to various problems related to angles and their measures. This lesson involves working with triangles, quadrilaterals, and other polygons to determine the measures of missing angles. By understanding the properties of different shapes and applying the relevant theorems, students can calculate the values of unknown angles in a given diagram.
One concept explored in Lesson 7.4 is the angle sum theorem. This theorem states that the sum of the measures of the interior angles of a triangle is always 180 degrees. By using this theorem, students can find missing angle measures in a triangle by subtracting the measures of the known angles from 180 degrees.
Another topic covered in Lesson 7.4 is the properties of parallel lines and transversals. When a transversal crosses two parallel lines, various angles are formed. Students learn to identify corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles. By understanding these relationships, they can solve for missing angle measures in complex diagrams.
Overall, Lesson 7.4 in geometry requires a solid understanding of angle relationships, theorems, and properties of different shapes. By practicing and applying these concepts, students can confidently find the answers to the problems presented in this lesson.
Lesson 7 4 Practice B Geometry Answers: Exploring the Topic
Lesson 7 4 in geometry is an important lesson that focuses on practice problems related to various concepts in geometry. This particular practice, Practice B, offers students the opportunity to further explore these concepts and solidify their understanding. By working through the practice problems and finding the answers, students can reinforce their knowledge and improve their problem-solving skills.
The practice problems in Lesson 7 4 Practice B cover a range of topics, including angles, polygons, and circles. Students are given different scenarios and asked to identify missing angles, classify polygons, or find the circumference of a circle. This variety of questions allows students to apply their knowledge of geometry to different situations, helping them develop a deeper understanding of the subject matter.
Key Concepts in Lesson 7 4 Practice B Geometry Answers:
- Angles: Students will encounter problems that involve finding missing angles in different geometric figures. They will need to apply properties of angles, such as vertical angles, complementary angles, and supplementary angles, to find the correct measurements.
- Polygons: Students will classify polygons based on the number of sides they have and their angles. They will need to determine whether a figure is a triangle, quadrilateral, pentagon, or another type of polygon.
- Circles: Students will need to calculate the circumference of a circle using the formula 2πr or find the radius or diameter of a circle given its circumference.
By practicing with Lesson 7 4 Practice B Geometry Answers, students can sharpen their geometry skills and become more proficient in solving problems related to angles, polygons, and circles. This practice not only helps them prepare for exams and quizzes but also lays a solid foundation for more advanced concepts in geometry.
Importance of Lesson 7-4 Practice B Geometry

Lesson 7-4 Practice B in Geometry is of great importance as it provides students with valuable exercises and problems to reinforce their understanding of the concepts covered in the lesson. Practice B serves as a tool for students to apply the knowledge they have gained and solidify their skills in geometric reasoning and problem-solving. By engaging in these practice exercises, students are able to refine their abilities and build a strong foundation in Geometry.
One key aspect of Lesson 7-4 Practice B is its focus on angles and their relationships within geometric figures. Through various exercises, students have the opportunity to explore the properties of angles such as vertical angles, alternate interior angles, and corresponding angles. By practicing these exercises, students develop an intuitive understanding of these angle relationships and are better equipped to reason and solve problems involving angles in the future.
The practice problems in Lesson 7-4 Practice B also promote critical thinking and analytical skills. Students are presented with different scenarios and must apply their knowledge to identify and solve the problems. This not only strengthens their problem-solving abilities but also enhances their logical reasoning skills. The ability to think critically and analyze geometric situations is crucial for success in Geometry and in real-life applications of geometry, such as engineering and architecture.
In conclusion, Lesson 7-4 Practice B in Geometry holds significant importance as it provides students with the opportunity to reinforce their understanding of angles and their relationships. By engaging in these practice exercises, students develop their problem-solving skills, enhance their logical reasoning abilities, and build a solid foundation in Geometry. Practice B serves as a valuable tool for students to apply their knowledge and excel in their study of Geometry.
Overview of Lesson 7 4 Practice B Geometry
In Lesson 7 4 Practice B Geometry, students will explore various concepts related to geometry, including angles, triangles, and circles. The practice exercises are designed to help students solidify their understanding of these topics and reinforce their problem-solving skills.
This lesson begins with a review of angle relationships, including complementary and supplementary angles, as well as vertical angles. Students will be asked to identify these types of angles in different diagrams and solve equations involving angle measures.
The lesson then progresses to a discussion of triangles, focusing on different types of triangles such as equilateral, isosceles, and scalene triangles. Students will learn how to calculate the missing side lengths and angles of these triangles using properties and theorems such as the Pythagorean theorem and the angle sum theorem.
In the latter part of the lesson, students will delve into the world of circles. They will learn about the different parts of a circle, including the radius, diameter, and circumference. Students will also explore central angles and inscribed angles, as well as the relationships between these angles and the arcs they intercept.
Throughout Lesson 7 4 Practice B Geometry, students will be asked to apply their knowledge and problem-solving skills to a variety of real-world and mathematical scenarios. By the end of the practice exercises, students will have gained a deeper understanding of angles, triangles, and circles, and will be better equipped to tackle more challenging geometry problems in the future.
Purpose and Objectives of Lesson 7 4 Practice B Geometry Answers
Lesson 7 4 Practice B in geometry aims to provide students with an opportunity to apply their knowledge and skills in various geometric concepts and problem-solving. The purpose of this practice is to reinforce the concepts learned in previous lessons and to assess the students’ understanding of those concepts.
The main objectives of the Lesson 7 4 Practice B in geometry are:
- To review and practice the properties and characteristics of triangles, including angle measures and side lengths.
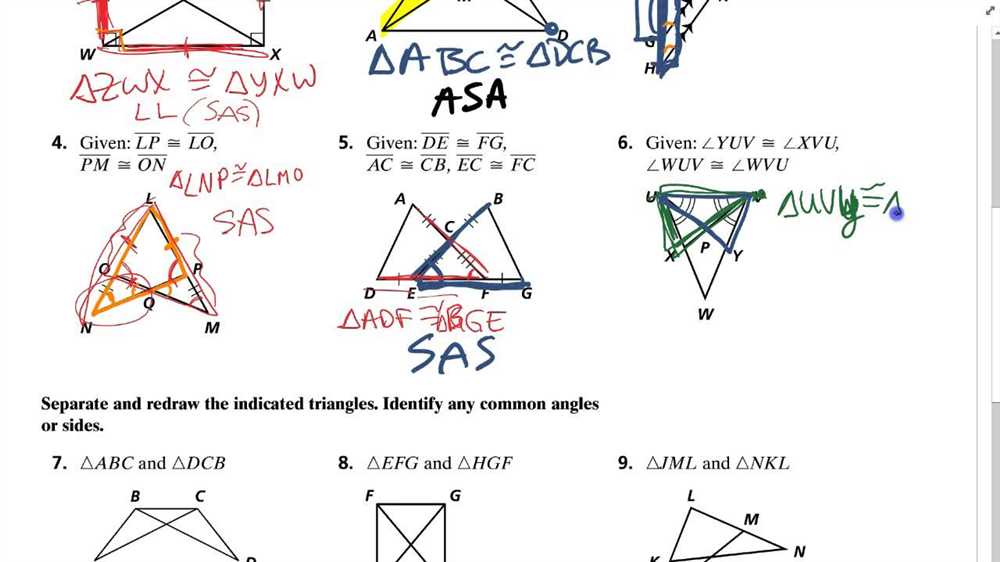
- To apply the triangle congruence criteria, such as Side-Angle-Side (SAS), Angle-Side-Angle (ASA), and Side-Side-Side (SSS) to determine if two triangles are congruent.
- To solve problems involving the measures of angles formed by intersecting lines, parallel lines, and transversals.
- To apply the properties and theorems related to parallel lines, including the Alternate Interior Angles, Alternate Exterior Angles, Corresponding Angles, and Vertical Angles.
- To practice using the Pythagorean Theorem and the converse of the Pythagorean Theorem to solve for missing side lengths in right triangles.
- To solve problems involving the measures of interior and exterior angles of polygons.
By completing Lesson 7 4 Practice B in geometry and answering the corresponding questions, students can enhance their understanding of geometric concepts, improve their problem-solving skills, and reinforce their knowledge for future lessons or assessments.
Understanding Geometric Concepts

Geometry is the branch of mathematics that deals with the properties and relationships of shapes, sizes, angles, and dimensions. It is an essential field of study for understanding the world around us, as geometric concepts are applied in various fields, such as architecture, engineering, art, and design.
Shapes: One of the fundamental concepts in geometry is understanding different shapes. Shapes can be classified into two-dimensional (2D) and three-dimensional (3D). Examples of 2D shapes include squares, circles, triangles, and rectangles, while examples of 3D shapes include cubes, spheres, cylinders, and pyramids.
Angles: Angles are another important concept in geometry. An angle is formed by two rays, called the sides of the angle, that share a common endpoint, called the vertex. Angles can be classified based on their measurements, such as acute angles (less than 90 degrees), right angles (exactly 90 degrees), obtuse angles (between 90 and 180 degrees), and straight angles (exactly 180 degrees).
Dimensions: Geometry also deals with dimensions, which refer to the measurements and extent of an object in different directions. For example, a line segment has one dimension (length), while a rectangle has two dimensions (length and width), and a cube has three dimensions (length, width, and height).
Properties and Relationships: Geometric concepts also involve understanding the properties and relationships between different shapes and objects. For example, parallel lines have the property of never intersecting, while perpendicular lines intersect at a right angle. Additionally, congruent shapes have the same size and shape, while similar shapes have the same shape but different sizes.
By studying and understanding geometric concepts, we can develop spatial reasoning skills, solve real-world problems, and appreciate the beauty and symmetry found in the world around us.
Solving Practice Problems
When studying geometry, it is important to practice solving problems to reinforce your understanding of the concepts and techniques. By actively engaging with practice problems, you can improve your problem-solving skills and gain confidence in your abilities. Here are some tips to help you effectively solve practice problems:
1. Understand the Problem

Before you start solving a practice problem, make sure you fully understand what is being asked. Read the problem carefully and identify the given information and what you are trying to find. Pay attention to any diagrams or figures provided, as they can help you visualize the problem.
2. Use the Relevant Concepts and Formulas
Once you understand the problem, use your knowledge of geometry concepts and formulas to solve it. Review the relevant concepts and formulas beforehand to ensure you apply them correctly. Identify which formula or technique is most appropriate for the given problem.
3. Break Down the Problem into Smaller Steps
If a practice problem seems complex, break it down into smaller, more manageable steps. This can help you organize your thoughts and prevent confusion. Take your time to solve each step carefully and double-check your work before moving on to the next step.
4. Practice Regularly
Consistent practice is key to improving your problem-solving skills in geometry. Set aside dedicated time each day or week to solve practice problems. Start with easier problems and gradually progress to more challenging ones. Seek out additional resources, such as textbooks, online tutorials, or practice worksheets, to expose yourself to a variety of problem types.
5. Review Your Mistakes
When you encounter difficulties or make mistakes while solving practice problems, take the time to analyze and understand your mistakes. Identify where you went wrong and learn from your errors. Review the correct solution and try to understand the underlying concept or technique. This will help you avoid similar mistakes in the future.
Remember, the more you practice solving problems, the more proficient you will become. Make solving practice problems a regular part of your geometry study routine and see your skills improve over time.
Lesson 7 4 Practice B Geometry Answers: Step-by-Step Solutions
In Lesson 7 4 Practice B in geometry, students are provided with a set of problems that require them to apply their knowledge of the concepts covered in the lesson. These problems are designed to help students reinforce their understanding and improve their problem-solving skills. The answers to these problems are available in the lesson materials, allowing students to check their work and identify any misconceptions.
Step-by-step solutions are provided for each problem in Lesson 7 4 Practice B. These solutions break down each problem into smaller, manageable steps, making it easier for students to follow along and understand the process. The solutions also include explanations and reasoning behind each step, helping students develop a deeper understanding of the concepts being applied.
The step-by-step solutions in Lesson 7 4 Practice B guide students through the problem-solving process, helping them develop critical thinking skills and problem-solving strategies. By working through these solutions, students can learn from their mistakes, identify areas where they need more practice, and gain confidence in their ability to solve similar problems in the future.
Overall, Lesson 7 4 Practice B in geometry provides students with an opportunity to practice and apply their knowledge of the concepts covered in the lesson. The step-by-step solutions available for each problem help students check their work, understand the problem-solving process, and improve their problem-solving skills. By actively engaging with the practice problems and the provided solutions, students can strengthen their understanding of geometry and build a solid foundation for future learning.
Explaining the Steps
The process of solving geometry problems often involves several steps that need to be executed in a specific order. Understanding these steps is crucial for successfully solving geometry problems. In this article, we will explain the common steps involved in solving geometry problems.
Step 1: Understand the problem
Before starting to solve a geometry problem, it is important to carefully read and understand the problem statement. Identify what is given and what needs to be found. Pay attention to any special conditions or restrictions mentioned in the problem.
Step 2: Draw a diagram
Once you have understood the problem, the next step is to draw a clear and accurate diagram. This visual representation of the problem will help you visualize the relationships between different elements and make it easier to apply geometric principles.
Step 3: Identify the relevant geometric principles
After drawing the diagram, analyze the problem and identify the relevant geometric principles or theorems that can be applied to solve it. This may involve recognizing congruent or similar triangles, angles formed by intersecting lines, or properties of specific geometric shapes.
Step 4: Set up and solve equations
Once you have identified the relevant principles, set up the necessary equations to solve the problem. This may involve setting up proportions, solving for unknown angles or side lengths, or applying specific formulas related to the geometric principles identified in the previous step.
Step 5: Check and interpret the solution
After solving the equations, it is important to check the solution to ensure it is reasonable and satisfies any given conditions or restrictions. Additionally, interpret the solution in the context of the problem to make sure it makes sense.
By following these steps, you can systematically approach and solve geometry problems with confidence. Practice and familiarity with various geometric principles will help improve your problem-solving skills in this subject.