
In Lesson 7 7 practice a geometry, students are challenged to apply their knowledge of geometric concepts and skills. This lesson focuses on solving problems involving angles and lines, as well as identifying and measuring different types of angles. By practicing these concepts, students will develop a deeper understanding of geometry and its applications in real-world scenarios.
One of the key skills developed in this lesson is the ability to solve problems involving supplementary and complementary angles. Students will learn how to identify these types of angles and use the properties of these angles to find missing angle measures. This skill is essential in many areas of math and science, as well as in everyday situations where angles are involved.
In addition to working with angles, students will also practice identifying and measuring parallel and perpendicular lines. This skill is crucial in geometry, as it allows us to understand and describe the relationships between different lines and shapes. By learning to identify parallel and perpendicular lines, students will be able to make connections between different geometric figures and deepen their understanding of the subject.
Overall, Lesson 7 7 practice a geometry offers students the opportunity to strengthen their geometric reasoning and problem-solving skills. Through hands-on practice and problem-solving, students will develop a strong foundation in geometry and be better equipped to tackle more complex geometric concepts in the future.
Lesson 7 7 Practice A Geometry Answers
In geometry, practice is crucial for understanding and mastering the concepts. Lesson 7 7 focuses on applying the knowledge of geometry to solve problems and find answers. The practice exercises provide an opportunity for students to reinforce their understanding and build confidence in their abilities.
One of the key topics covered in Lesson 7 7 is triangles. Triangles are fundamental shapes in geometry, and understanding their properties and relationships is essential. The practice exercises in this lesson involve identifying and classifying triangles based on their angles and sides. By solving these exercises, students can improve their ability to recognize different types of triangles and understand the criteria for their classification.
Another important topic in Lesson 7 7 is the use of the Pythagorean theorem. The Pythagorean theorem is a fundamental theorem in geometry that relates the lengths of the sides of a right triangle. The practice exercises in this lesson involve applying the Pythagorean theorem to find the lengths of missing sides in right triangles. By solving these exercises, students can enhance their ability to use the Pythagorean theorem and solve problems involving right triangles.
Overall, Lesson 7 7 practice exercises in geometry provide an opportunity for students to reinforce their understanding of triangles and the Pythagorean theorem. By solving these exercises, students can improve their problem-solving skills and build a solid foundation in geometry.
Understanding Geometry Concepts
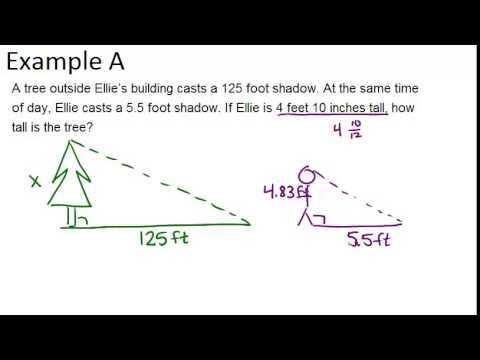
Geometry is a branch of mathematics that deals with the properties and relationships of points, lines, shapes, and spaces. It provides a framework for analyzing and understanding the world around us, from the shape of a building to the path of a satellite orbiting the Earth.
One of the key concepts in geometry is the idea of points, which are infinitely small and have no size. Points can be connected to form lines, which are straight or curved paths that extend infinitely in both directions. Lines can also intersect, creating angles. Angles are formed by the intersection of two lines or two line segments, and they help us measure and compare the amount of rotation or curvature between them.
In addition to points, lines, and angles, geometry also deals with polygons, which are closed shapes with straight sides. Examples of polygons include triangles, squares, and hexagons. Each polygon has its own unique set of properties, such as the number of sides and angles it has. These properties can be used to classify and categorize polygons, making it easier to study and understand their characteristics.
Geometry is not only about memorizing formulas and solving equations. It is about developing spatial reasoning skills and learning to think critically and logically. By understanding the basic concepts of geometry, we can make sense of the world around us and apply mathematical principles to solve real-world problems. Whether it’s calculating the area of a room or designing a bridge, geometry plays a crucial role in our everyday lives.
Solving Geometry Problems
Geometry problems often require logical thinking and the application of various geometric principles and theorems. To solve these problems effectively, it is essential to understand the given information and analyze the relationships between different elements.
Identifying knowns and unknowns: The first step in solving a geometry problem is identifying what is given (knowns) and what needs to be determined (unknowns). This involves carefully reading the problem and extracting all relevant information. It is important to assign variables or labels to unknown quantities to make the problem easier to work with.
Applying geometric principles: Once the knowns and unknowns have been identified, the next step is to apply geometric principles and theorems to find the solution. This may involve using properties of angles, triangles, quadrilaterals, circles, or other geometric shapes. It is crucial to use the correct formulas and theorems and adapt them to the specific problem being solved.
Using logical reasoning: Geometry problems often require logical reasoning to determine the next steps in the solution. This may involve making deductions based on given information, applying the transitive property, using the properties of similar or congruent figures, or using the properties of parallel lines and angles.
Checking and verifying the solution: After obtaining a solution, it is important to check and verify its accuracy. This can be done by substituting the obtained values or measurements back into the problem and ensuring that they satisfy all the given conditions. It is also essential to double-check the calculations and ensure that the solution makes sense in the context of the problem.
By following these steps and practicing regularly, one can become more proficient in solving geometry problems. It is important to approach each problem with a clear understanding of the geometric principles involved and to think logically throughout the solution process.
Applying Geometric Formulas
Geometry is a branch of mathematics that deals with the shapes and properties of figures in space. In geometry, there are various formulas that can be used to calculate the measurements of different shapes and objects. These formulas help us solve problems and find unknown quantities.
One important formula in geometry is the formula for the area of a rectangle. The area of a rectangle can be found by multiplying its length and width. For example, if a rectangle has a length of 10 units and a width of 5 units, the area would be 10 * 5 = 50 square units.
Another important formula is the Pythagorean theorem, which is used to find the length of one side of a right triangle when the lengths of the other two sides are known. According to the Pythagorean theorem, the square of the hypotenuse is equal to the sum of the squares of the other two sides. This formula is often written as a^2 + b^2 = c^2, where a and b represent the lengths of the legs and c represents the length of the hypotenuse. For example, if a right triangle has one leg measuring 4 units and the other leg measuring 3 units, the length of the hypotenuse can be found using the formula 4^2 + 3^2 = c^2, which simplifies to 16 + 9 = c^2. Solving for c, we get c = √25, or c = 5 units.
These are just a few examples of the many geometric formulas that can be used to solve problems in geometry. By understanding and applying these formulas, we can analyze and calculate the measurements of various shapes and objects, helping us to better understand the world around us.
Utilizing Geometry Vocabulary
Geometry is not just about shapes and figures; it also involves a rich vocabulary that helps us describe and understand the properties and characteristics of these shapes. By utilizing geometry vocabulary effectively, we can communicate more precisely and have a deeper understanding of geometric concepts and relationships.
One key vocabulary term in geometry is “angle.” An angle is formed when two rays share a common endpoint, known as the vertex. By using specific vocabulary associated with angles, such as “acute,” “obtuse,” or “right,” we can describe the measure and shape of angles accurately. These terms help us distinguish between different types of angles and identify their properties.
Another important aspect of geometry vocabulary is the terminology related to triangles. Words like “equilateral,” “isosceles,” and “scalene” help describe the lengths of the sides and the angles of a triangle, providing insights into its properties. Additionally, terms like “orthocenter,” “centroid,” and “circumcenter” help us identify special points within a triangle and understand their geometric significance.
Other geometry vocabulary includes terms for different types of quadrilaterals, polygons, and circles. By using words like “rectangle,” “rhombus,” “trapezoid,” “pentagon,” “hexagon,” and “circle,” we can precisely describe the shape and properties of these figures. These terms aid in classifying and categorizing geometric shapes, allowing us to explore their unique traits and relationships.
In conclusion, learning and utilizing geometry vocabulary is crucial for effectively communicating and understanding geometric concepts. By using precise terminology, we can describe shapes, angles, and figures accurately while also gaining insights into their properties and relationships.
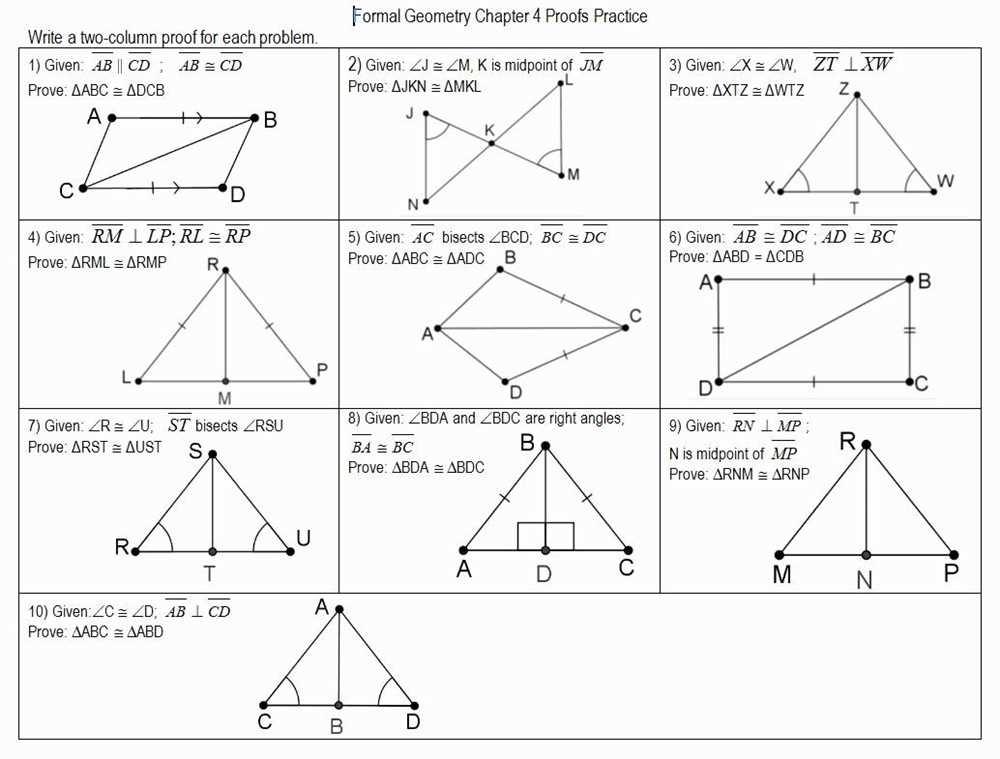
Practicing Geometry Proofs
Geometry proofs are an important part of studying geometry as they help students develop logical reasoning skills and deepen their understanding of geometric concepts. These proofs require students to use previously learned theorems, postulates, and definitions to prove that a given statement is true.
When practicing geometry proofs, it is crucial to understand the structure and format of a proof. A typical proof consists of a series of statements and logical reasoning, with each statement justified using a theorem or definition. It is essential to identify the given information, such as given statements or diagrams, and use them to find a logical sequence of steps to prove the desired conclusion.
One common method for proving geometric theorems is using a two-column proof. In a two-column proof, the statements are listed in one column, and the corresponding justifications or reasons are listed in the other column. This format helps organize the steps of the proof and makes it easier to follow the logical progression.
When practicing geometry proofs, it is beneficial to start with basic theorems and gradually move on to more complex problems. This approach allows students to build their proof-writing skills step by step while gaining confidence in their abilities. It is also essential to review and understand the properties and definitions relevant to the specific problem at hand.
Overall, practicing geometry proofs is a valuable exercise that helps students develop critical thinking and problem-solving skills. By mastering the art of proof-writing, students not only deepen their understanding of geometry but also enhance their logical reasoning abilities, which can be applied to various other fields of study.
Exploring Geometric Transformations
Geometric transformations are a fundamental concept in geometry that involve changing the position, size, or shape of a figure. These transformations can be applied to various 2D and 3D shapes to create new figures or investigate their properties. This article will explore some common geometric transformations, including translations, rotations, reflections, and dilations.
Translations: A translation is a transformation that slides a figure from one location to another without changing its shape or orientation. This is done by shifting each point of the figure a certain distance in a specific direction. Translations can be performed in any direction and magnitude, allowing us to move figures horizontally, vertically, or diagonally.
Rotations: A rotation is a transformation that rotates a figure around a fixed point called the center of rotation. This causes each point of the figure to move along a circular path. Rotations can be clockwise or counterclockwise and can be performed at any angle. They are often described using the degree of rotation and the direction of rotation.
Reflections: A reflection is a transformation that creates a mirror image of a figure across a line called the line of reflection. This line acts as a boundary, with each point of the figure being reflected across it to create the image. Reflections can be performed across any vertical, horizontal, or diagonal line, and they can preserve or reverse the orientation of the figure.
Dilations: A dilation is a transformation that changes the size of a figure by stretching or shrinking it. This is done by multiplying each coordinate of the figure by a scale factor. A scale factor greater than 1 stretches the figure, making it larger, while a scale factor between 0 and 1 shrinks the figure, making it smaller. Dilations can be performed with respect to a point, known as the center of dilation, or with respect to a line or other geometric object.
Summary:
- Geometric transformations involve changing the position, size, or shape of a figure.
- Translations slide a figure from one location to another without changing its shape or orientation.
- Rotations rotate a figure around a fixed point.
- Reflections create a mirror image of a figure across a line.
- Dilations change the size of a figure by stretching or shrinking it.
By understanding and exploring these geometric transformations, we can better understand the properties and relationships between different geometric figures. These transformations are not only important in geometry but also have practical applications in various fields, such as computer graphics, architecture, and engineering.