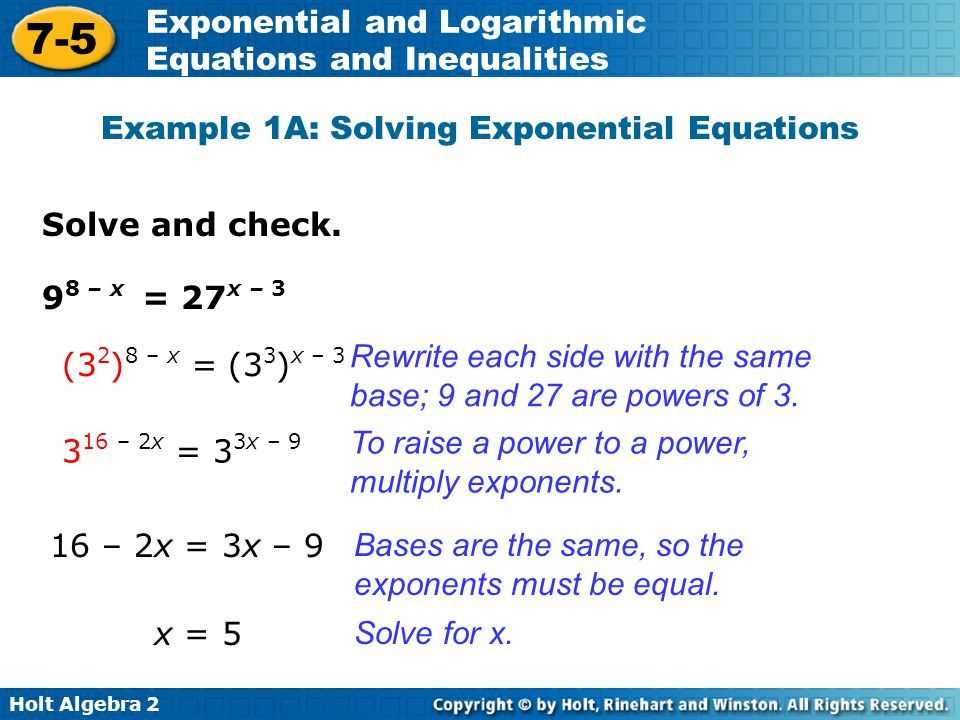
Logarithmic equations can be challenging to solve, but with the right approach and understanding of logarithmic properties, finding the answer becomes much easier. In this article, we will provide you with an answer key to logarithmic equations.
Before we dive into the answer key, let’s briefly review logarithms. Logarithms are the inverse operation of exponentiation. They help us solve equations where the variable is in the exponent. Logarithmic equations often involve logarithms with different bases, such as base 10 (common logarithms) or base e (natural logarithms).
When solving logarithmic equations, there are several key steps to keep in mind. First, isolate the logarithm on one side of the equation. Second, rewrite the logarithm in exponential form to eliminate the logarithm. Next, solve the resulting exponential equation for the variable. Finally, check your solution by substituting it back into the original equation.
Now, let’s take a look at some examples of logarithmic equations and their corresponding answers. By following these steps and using the answer key as a guide, you will be able to confidently solve logarithmic equations and check your work for accuracy.
Logarithmic Equations: What You Need to Know
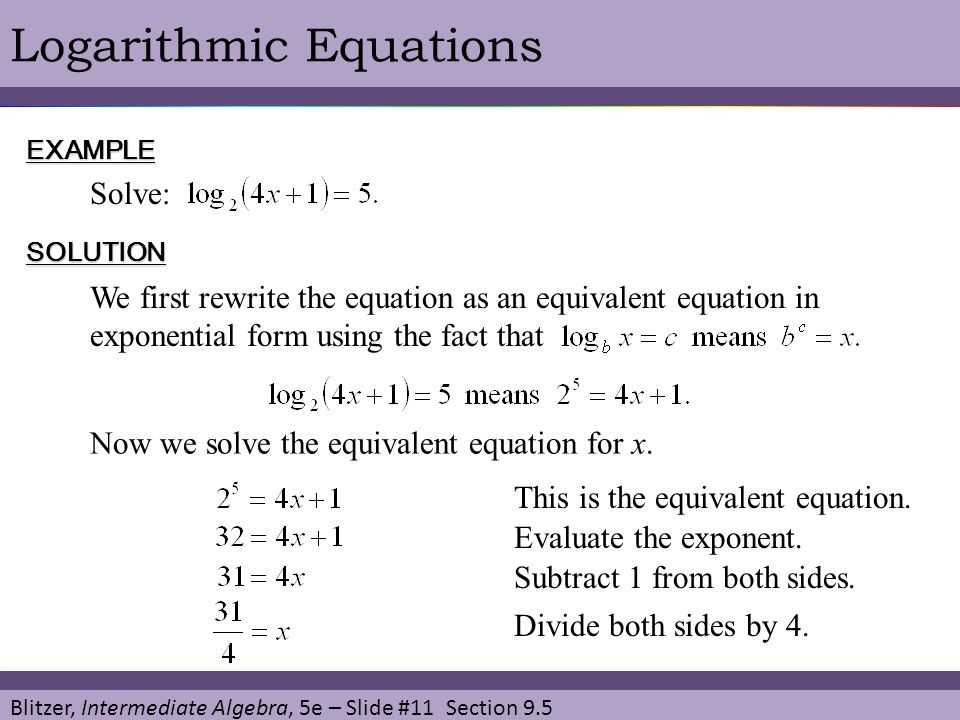
If you have ever encountered logarithmic equations in algebra or calculus, you know that they can be somewhat challenging to solve. Logarithmic equations involve logarithms, which are mathematical functions that measure the power to which a base number must be raised to obtain a given value. Understanding how to manipulate and solve logarithmic equations is an essential skill for anyone studying advanced math or pursuing a career in a related field.
One important property of logarithms that you need to know is the inverse relationship between exponentials and logarithms. Logarithmic equations can be solved by converting them into exponential form and vice versa. This conversion allows you to apply algebraic techniques to isolate the variable and solve for its value.
When solving logarithmic equations, it is crucial to be familiar with the rules of logarithms. These rules include the product rule, quotient rule, and power rule, among others. Understanding these rules will enable you to simplify logarithmic expressions and solve equations more efficiently. However, it is important to note that when applying these rules, you must be careful to follow the restrictions on the domain of the logarithmic function.
Another key aspect of solving logarithmic equations is identifying extraneous solutions. Since logarithmic functions are only defined for positive values, some solutions obtained during the solving process may not be valid. These solutions, known as extraneous solutions, occur when you introduce values that are outside the acceptable domain of the logarithmic function. Identifying and discarding extraneous solutions is essential to ensure accurate results.
In summary, understanding logarithmic equations and how to solve them is an important skill for advanced math students and professionals in related fields. Familiarizing yourself with the properties and rules of logarithms, as well as being able to identify and discard extraneous solutions, will enable you to tackle logarithmic equations confidently and accurately.
Understanding Logarithmic Functions
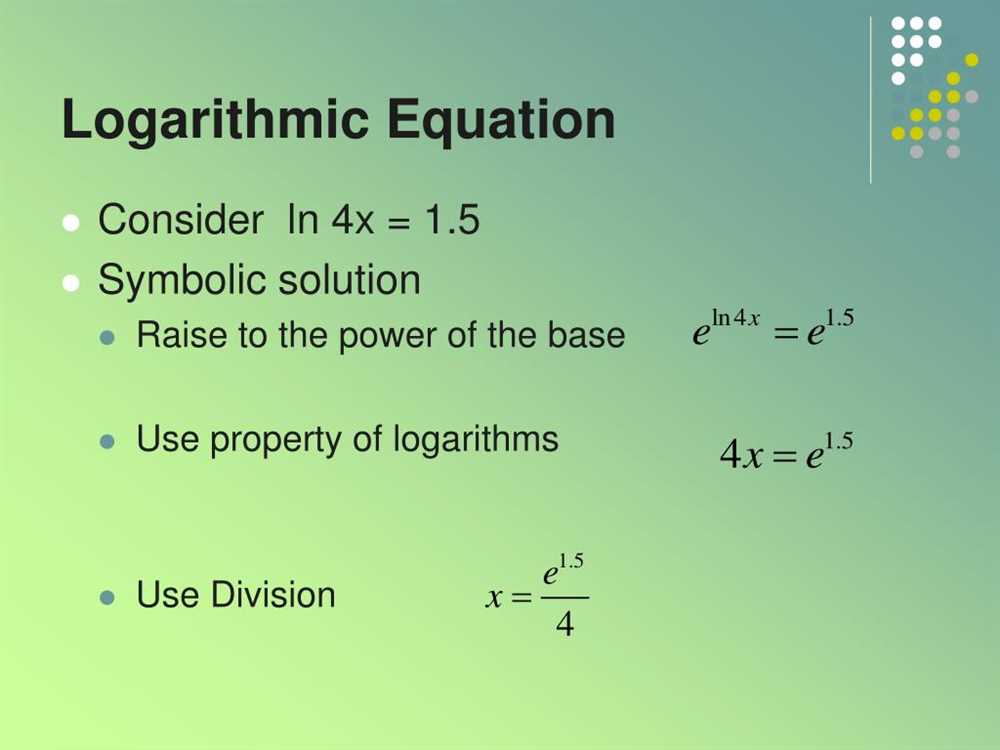
Logarithmic functions are an important concept in mathematics that help us solve equations involving exponents. They are the inverse functions of exponential functions. Understanding logarithmic functions will not only help us solve logarithmic equations, but also understand various real-world applications.
One key concept in logarithmic functions is the logarithm itself. A logarithm is defined as the exponent to which a base must be raised in order to obtain a certain number. The base of a logarithm can be any positive number except 1, and the number inside the logarithm is called the argument. The logarithm function is usually denoted as logb(x), where b is the base and x is the argument.
Logarithmic functions have several properties that are important to understand. One property is that the logarithm of a product is equal to the sum of the logarithms of the individual factors. This property can be written as logb(xy) = logb(x) + logb(y). Another property is that the logarithm of a quotient is equal to the difference of the logarithms of the numerator and the denominator. This property can be written as logb(x/y) = logb(x) – logb(y).
Logarithmic functions also have a property called the change of base formula, which allows us to compute logarithms in different bases. The change of base formula states that logb(x) = loga(x) / loga(b), where a is the base we want to change from and b is the base we want to change to.
- Logarithmic functions are the inverse functions of exponential functions.
- A logarithm is the exponent to which a base must be raised to obtain a certain number.
- Logarithmic functions have properties such as the product property and the quotient property.
- The change of base formula allows us to compute logarithms in different bases.
Overall, understanding logarithmic functions is crucial for solving logarithmic equations and for gaining a deeper understanding of exponential functions and their applications. By studying these functions and their properties, we can better analyze exponential growth and decay, solve equations involving exponents, and make informed decisions in fields such as finance, science, and engineering.
How Logarithmic Equations Work

Logarithmic equations are mathematical expressions that involve logarithms. Logarithms are mathematical functions that relate an exponent to a base number. They are widely used in various fields of study, including mathematics, physics, engineering, and finance.
To understand how logarithmic equations work, it is important to understand the basic properties of logarithms. Logarithms allow us to solve exponential equations, where the variable is in the exponent. They can also be used to condense and expand expressions, simplify calculations, and solve complex problems.
One of the key properties of logarithms is that they can “undo” exponential operations. For example, if we have an exponential equation in the form of “b^x = y,” we can use logarithms to rewrite it as “log base b of y = x.” This allows us to solve for the variable x by taking the logarithm of both sides of the equation.
Logarithmic equations can be solved algebraically or graphically. Algebraic methods involve manipulating the equation to isolate the variable and apply logarithmic properties to simplify the expression. Graphical methods involve plotting the logarithmic equation on a graph and finding the intersection points with other functions or lines to determine the solution.
Overall, logarithmic equations provide a powerful tool for solving complex mathematical problems. By understanding the properties of logarithms and practicing with different types of equations, individuals can develop the skills necessary to solve logarithmic equations effectively.
Solving Logarithmic Equations Step by Step
When solving logarithmic equations, it is important to follow a step-by-step approach to ensure accurate solutions. By applying specific rules and properties of logarithms, we can simplify the equation and solve for the variable.
Step 1: Identify the equation as a logarithmic equation. Logarithmic equations have the logarithmic function (log) or natural logarithmic function (ln) as part of the equation.
Step 2: Isolate the logarithmic function. Move any terms without logarithms to the other side of the equation. This step helps in simplifying the equation and isolating the logarithmic function for further manipulation.
Step 3: Apply logarithmic properties. Use the properties of logarithms to rewrite the equation in a simplified form. These properties include the product, quotient, and power rules of logarithms.
Step 4: Solve for the variable. Transform the equation into an exponential equation by converting the logarithmic form to exponential form. This allows us to solve for the variable using basic algebraic operations.
Step 5: Check for extraneous solutions. After obtaining a solution for the variable, it is important to check if it satisfies the original equation. Sometimes, logarithmic equations may result in extraneous solutions, which do not actually satisfy the equation.
By carefully following these steps, one can accurately solve logarithmic equations and obtain valid solutions. Practice and familiarity with logarithmic properties will contribute to solving such equations efficiently.
Isolating the Logarithmic Expression
When solving logarithmic equations, one of the main steps is isolating the logarithmic expression. This means getting rid of any other terms or variables that may be present in the equation and leaving only the logarithm on one side of the equation. This process allows us to apply properties and rules of logarithms to simplify the equation further and find the solution.
To isolate the logarithmic expression, we need to perform inverse operations on the equation. If there are any terms or variables added or subtracted to the logarithmic expression, we should get rid of them first. Similarly, if there are any terms or variables multiplied or divided with the logarithmic expression, we should undo those operations as well.
Example:
Let’s consider the equation: 2log(x + 3) – 4 = 2.
- First, we isolate the logarithmic expression by getting rid of the constant term. We can do this by adding 4 to both sides of the equation:
- 2log(x + 3) = 6.
- Next, we can remove the coefficient of 2 in front of the logarithm by dividing both sides of the equation by 2:
- log(x + 3) = 3.
- Finally, to isolate the logarithmic expression completely, we can exponentiate both sides of the equation with base 10:
- 10^(log(x + 3)) = 10^3.
- x + 3 = 1000.
By isolating the logarithmic expression, we have simplified the equation and made it easier to find the solution, which in this case is x = 997.
Using Properties of Logarithms
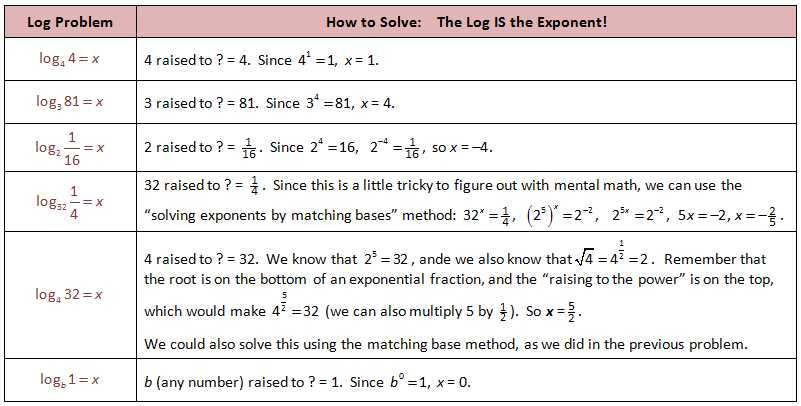
When solving logarithmic equations, it is important to understand the properties of logarithms. These properties can help simplify equations and find the values of variables.
Property 1: Product Rule of Logarithms
- The logarithm of a product is equal to the sum of the logarithms of the individual factors.
- The equation logb(xy) = logb(x) + logb(y) follows this property.
- By applying this rule, we can break down complex logarithmic equations into simpler steps.
Property 2: Quotient Rule of Logarithms
- The logarithm of a quotient is equal to the difference of the logarithms of the numerator and the denominator.
- This rule can be expressed as logb(x/y) = logb(x) – logb(y).
- Using this rule, we can separate the numerator and the denominator in a logarithmic equation.
Property 3: Power Rule of Logarithms
- The logarithm of a number raised to a power is equal to the product of the power and the logarithm of the number.
- This rule can be represented as logb(xn) = n * logb(x).
- By applying this rule, we can simplify logarithmic equations and solve for variables.
Property 4: Change of Base Formula
- If we want to evaluate a logarithm with a base that is not available on our calculator, we can use the change of base formula.
- The change of base formula states that logb(x) = loga(x) / loga(b), where a is the base we have available on our calculator.
- Using this formula, we can calculate logarithmic equations with different bases.
By understanding and applying these properties, we can manipulate logarithmic equations and find their solutions effectively.
Simplifying the Equation
When solving logarithmic equations, one step in the process is simplifying the equation. This involves rearranging the equation to make it easier to solve or to reveal any patterns or properties that can help solve it. There are several techniques that can be used to simplify logarithmic equations.
1. Use the product rule: If the equation contains the product of two logarithms with the same base, the product rule can be applied to simplify it. The product rule states that logb(x * y) = logb(x) + logb(y).
2. Use the quotient rule: If the equation contains the quotient of two logarithms with the same base, the quotient rule can be applied to simplify it. The quotient rule states that logb(x / y) = logb(x) – logb(y).
3. Use the power rule: If the equation contains a logarithm raised to a power, the power rule can be applied to simplify it. The power rule states that logb(xa) = a * logb(x).
4. Combine like terms: If the equation contains logarithms with the same base and the same argument, you can combine them into a single logarithm. For example, if the equation has logb(x) + logb(x) – logb(y), you can combine the first two terms to get 2*logb(x) – logb(y).
By simplifying the equation using these techniques, you can often reduce the complexity of the problem and make it easier to solve. It is important to be familiar with these rules and to practice applying them to various logarithmic equations.
Solving for the Unknown Variable
Solving for the unknown variable is a fundamental concept in mathematics. Whether it’s in algebra, trigonometry, or calculus, finding the value of an unknown variable is a crucial step in problem-solving. In logarithmic equations, the unknown variable is typically found by applying logarithmic functions and properties.
Logarithmic equations involve logarithms, which are the inverse operations of exponential functions. They are commonly used to solve equations involving exponential growth or decay. To find the value of the unknown variable in a logarithmic equation, one must isolate the logarithm and apply appropriate properties, such as the product rule, quotient rule, or power rule.
When solving logarithmic equations, it is important to remember to check for extraneous solutions. Since logarithmic equations involve the operation of taking logarithms of positive numbers, it is possible to obtain solutions that do not satisfy the original equation. This can occur when taking the logarithm of a negative number or when applying logarithmic properties incorrectly.
There are various methods to solve logarithmic equations, depending on the complexity of the equation. Some equations can be solved by rewriting them in exponential form, while others may require a combination of algebraic manipulation and logarithmic properties. Regardless of the method used, solving for the unknown variable in logarithmic equations requires a solid understanding of logarithmic functions and their properties.
In conclusion, solving for the unknown variable in logarithmic equations is an important skill in mathematics. It involves applying logarithmic functions and properties to isolate the logarithm and find the value of the unknown variable. It is crucial to check for extraneous solutions and have a strong understanding of logarithmic functions to solve these equations effectively.