
If you are currently learning about measures of central tendency and need some extra practice, a worksheet with answers in PDF format can be a valuable resource. This comprehensive guide aims to provide you with a step-by-step approach to understanding and solving problems related to measures of central tendency.
Measures of central tendency, such as mean, median, and mode, are statistical tools used to describe the center or average of a set of data. These measures help to summarize a large amount of data in a concise and meaningful way. By studying and practicing with a worksheet, you can gain a deeper understanding of how to calculate and interpret these measures, and apply them to real-life situations.
The worksheet with answers provided in PDF format offers a convenient way to practice your skills anytime and anywhere. You can work through multiple examples and check your solutions using the provided answers. This will help you identify any areas where you may need additional practice or review, allowing you to focus your efforts and improve your understanding.
With the help of this worksheet, you will learn how to calculate the mean, median, and mode for a given set of data. You will also gain insights into how each of these measures can provide different information about the data set. Additionally, the worksheet covers topics such as outliers and how they can affect the measures of central tendency, as well as how to interpret the results in context.
Overall, by utilizing the measures of central tendency worksheet with answers in PDF format, you can enhance your understanding of these statistical tools and improve your proficiency in solving problems related to them. With practice, you will gain the confidence needed to apply these measures to analyze and interpret data effectively.
Measures of Central Tendency Worksheet with Answers PDF
Measures of central tendency are statistical measures used to describe the center or average of a data set. These measures provide valuable information about the data and help in understanding its distribution. One way to practice and reinforce understanding of these measures is through worksheets. A measures of central tendency worksheet with answers in PDF format is a convenient and accessible tool for students and teachers alike.
The worksheet typically consists of a series of problems or questions related to finding measures of central tendency, such as mean, median, and mode. The problems may involve finding the central tendency for small or large data sets, as well as calculating the central tendency for grouped data. The answers to the problems are provided on the worksheet, allowing students to check their work and self-assess their understanding.
The PDF format of the worksheet makes it easy to distribute and access the material. Whether in a traditional classroom or in a remote learning environment, students can download and print the worksheet or view it directly on their devices. The PDF format ensures that the worksheet retains its formatting and can be easily read and completed.
Using a measures of central tendency worksheet with answers PDF can benefit students in several ways. It provides them with an opportunity to practice and apply the concepts they have learned in class. By completing the problems, students can reinforce their understanding of how to calculate the mean, median, and mode and how to interpret their results.
In addition, having the answers readily available allows students to check their work independently. They can compare their solutions to the provided answers and identify any mistakes or areas that need further review. This self-assessment helps students develop their problem-solving skills and gain confidence in their abilities.
Overall, a measures of central tendency worksheet with answers PDF is a valuable resource for both students and teachers. It offers a structured way to practice and reinforce understanding of measures of central tendency, while also allowing for self-assessment and independent learning.
What are Measures of Central Tendency?
Measures of central tendency are statistical values that provide information about the middle, typical, or central value in a dataset. These measures help us understand the central position or average of a set of values. The three commonly used measures of central tendency are mean, median, and mode.
Mean: The mean, also known as the average, is calculated by adding up all the values in a dataset and dividing it by the number of values. It gives us a sense of the typical value in a dataset.
Median: The median is the middle value of a dataset when it is arranged in ascending or descending order. If there is an odd number of values, the median is the value exactly in the middle. If there is an even number of values, the median is the average of the two middle values.
Mode: The mode is the value that appears most frequently in a dataset. It represents the value that occurs with the highest frequency. A dataset can have multiple modes or even no mode if all values occur with equal frequency.
These measures of central tendency provide different insights into the data. The mean is influenced by extreme values, while the median is more robust to outliers. The mode is useful for identifying the most common value or category in a dataset. By using these measures, statisticians and researchers can summarize and describe the central characteristics of a dataset. They help in making comparisons, understanding patterns, and drawing conclusions based on the data.
Why are Measures of Central Tendency Important?
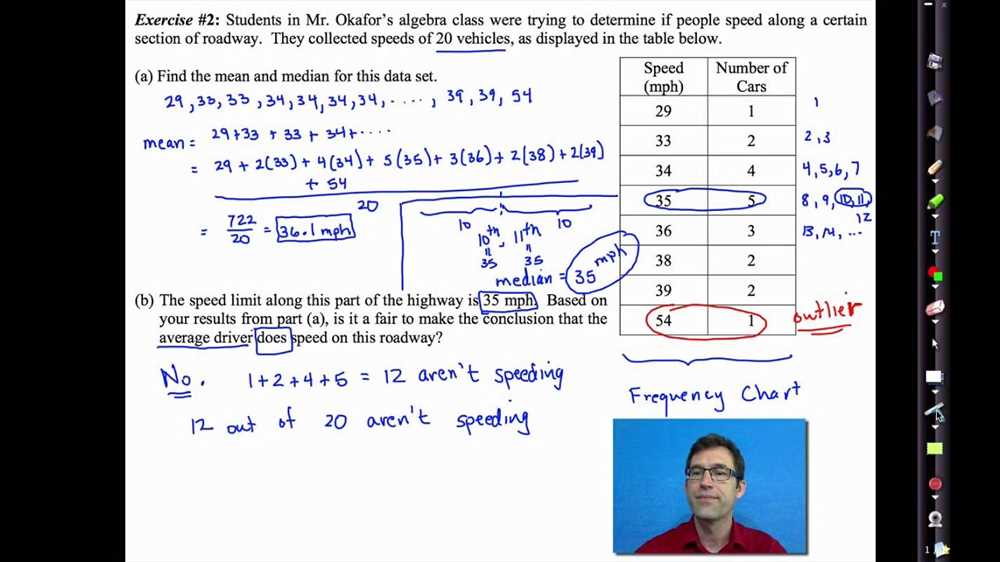
Measures of central tendency are important statistical tools that help us understand and summarize data. They provide information about the typical or average value of a set of data points. One of the most commonly used measures of central tendency is the mean or average. The mean represents the sum of all the data points divided by the total number of data points. It gives us a good idea of the overall value or magnitude of the data.
Measures of central tendency are important in various fields and applications. In business and finance, for example, they are used to analyze sales figures, profits, and market trends. By calculating the mean or average, companies can assess their performance and make informed decisions based on the data. In education, measures of central tendency are used to evaluate student performance and determine class averages. They can also be used to identify outliers or extreme values that may skew the overall picture.
Another useful measure of central tendency is the median. The median represents the middle value of a set of data when it is arranged in ascending or descending order. This measure is especially useful when dealing with skewed data or outliers. It provides a more accurate representation of the typical value of the data, as it is not affected by extreme values.
- Measures of central tendency are also important in research and scientific studies. They help researchers summarize and analyze data, making it easier to draw meaningful conclusions and make accurate predictions. For example, in medical research, measures of central tendency are used to analyze patient data and assess the effectiveness of treatments.
- In addition, measures of central tendency are often used in quality control and manufacturing processes. By calculating the mean or median of measurements or test results, companies can monitor and improve their production processes, ensuring that products meet the required standards and specifications.
In summary, measures of central tendency are important statistical tools that provide valuable insights and summaries of data. They help us understand the average or typical value of a set of data points, making it easier to analyze and interpret the data. From business and finance to education and research, measures of central tendency play a crucial role in various fields and applications.
Types of Measures of Central Tendency
Measures of central tendency are statistical measures that represent the center or average of a set of data. They provide valuable information about the distribution and characteristics of the data. There are several types of measures of central tendency that are commonly used to describe data.
Mean: The mean is the most common measure of central tendency. It is calculated by summing up all the values in a data set and dividing it by the total number of values. The mean represents the average value of the data set and is often used to measure the central value.
Median: The median is another measure of central tendency, and it represents the middle value in a data set when arranged in ascending or descending order. If there is an even number of values, the median is calculated as the average of the two middle values. The median provides an indication of the overall central position of the data set.
Mode: The mode is the most frequently occurring value in a data set. It represents the value that appears with the highest frequency. Unlike the mean and median, the mode does not necessarily represent the central value of the data set. However, it can be useful in identifying dominant values or patterns within the data.
Range: While not technically a measure of central tendency, the range is often used to provide information about the spread or variability in a data set. It is calculated as the difference between the maximum and minimum values in the data set. The range provides an indication of the extent of variation within the data.
Overall, measures of central tendency play an important role in summarizing and understanding data. By providing a single value that represents the center of a distribution, these measures can help researchers and analysts gain insight into the characteristics and patterns of the data.
Mean: Definition and Calculation

The mean is a measure of central tendency that represents the average value of a set of numbers. It is calculated by summing all the numbers in the set and then dividing the sum by the total number of values. The mean is also known as the arithmetic mean or the average.
In order to calculate the mean, you need a set of numerical data. Let’s say you have a set of 5 numbers: 10, 15, 20, 25, and 30. To find the mean, you add up all these numbers: 10 + 15 + 20 + 25 + 30 = 100. Then, you divide the sum by the total number of values, which is 5 in this case. So, the mean of this set is 100 divided by 5, which is 20.
The mean is a useful measure for summarizing a dataset because it takes into account every value in the set. However, it can be influenced by extreme values, also known as outliers. For example, if you have a set of numbers with one very large value, the mean will be skewed towards that value.
When interpreting the mean, it is important to consider the context of the dataset and any potential outliers. In some cases, the median or mode may provide a more appropriate measure of central tendency. Nevertheless, the mean is widely used and understood, making it a valuable tool for data analysis and comparison.
Median: Definition and Calculation
The median is a measure of central tendency that represents the middle value of a dataset. It is calculated by arranging the dataset in order from smallest to largest and finding the value that divides the dataset into two equal halves.
To calculate the median, first, arrange the dataset in ascending order. If the dataset has an odd number of values, the middle value is the median. For example, in the dataset {2, 4, 6, 8, 10}, the median is 6 because it is the middle value.
If the dataset has an even number of values, the median is calculated by taking the average of the two middle values. For example, in the dataset {1, 3, 5, 7}, the two middle values are 3 and 5. Thus, the median is (3 + 5) / 2 = 4.
The median is a useful measure of central tendency, especially when dealing with datasets that have outliers. Unlike the mean, which can be heavily influenced by extreme values, the median is not affected as much by outliers and provides a more representative value for the center of the dataset.
In summary, the median represents the middle value of a dataset and is calculated by arranging the values in order and finding the value that divides the dataset into two equal halves. It is a robust measure of central tendency that is less influenced by outliers compared to the mean.
Mode: Definition and Calculation
The mode is a measure of central tendency that represents the most frequent value or values in a dataset. It is the value that occurs most often. For example, in the dataset {1, 2, 2, 3, 4, 4, 4, 5}, the mode is 4 because it appears more frequently than any other value.
To calculate the mode, you need to determine which value or values appear most frequently in the dataset. This can be done by creating a frequency distribution table or by simply counting the number of times each value appears. The value(s) with the highest frequency is the mode.
It is possible for a dataset to have more than one mode. This occurs when two or more values appear with the same highest frequency. For example, in the dataset {1, 2, 2, 3, 3, 4, 5, 5}, both 2 and 3 are modes because they appear twice, while the other values appear only once.
In some cases, a dataset may not have a mode if no value appears more than once. This is called a “no mode” dataset. For example, in the dataset {1, 2, 3, 4, 5}, there is no mode because each value appears only once.
In summary, the mode is a measure of central tendency that represents the most frequent value(s) in a dataset. It can be calculated by determining which value(s) appear with the highest frequency. A dataset may have one or more modes, or it may have no mode.
Measures of Central Tendency Worksheet
In statistics, measures of central tendency are used to describe the central or typical value of a set of data. These measures provide information about the average or most representative value in a dataset. A measure of central tendency is a single value that represents the entire set of data.
A measures of central tendency worksheet is a useful tool for practicing and understanding these statistical measures. It usually contains a set of data and a series of questions or exercises that require calculating and interpreting measures of central tendency, such as mean, median, and mode.
For example, a worksheet may include a list of numbers and ask students to calculate the mean of the data. The mean is calculated by summing all the numbers in the set and dividing by the total number of values. Students would need to perform these calculations and write down the correct answer on the worksheet.
The worksheet may also include questions that require finding the median, which is the middle value in a dataset when the numbers are arranged in ascending or descending order. Students would need to sort the numbers and identify the middle value as the median.
The mode, which is the value that appears most frequently in a dataset, may also be included in the worksheet. Students would need to find the value that occurs most often and write it down as the mode.
Overall, a measures of central tendency worksheet is a valuable tool for practicing and understanding these statistical measures. It helps students develop their data analysis skills and provides a comprehensive overview of the central or typical values in a dataset. By working through the exercises on the worksheet, students can improve their ability to interpret and analyze data.