
If you have been studying motion in one dimension and specifically free fall, you might have come across some challenging problems and worksheets. In this article, we will provide answers and explanations for common questions found in a motion in one dimension free fall worksheet.
One common question in these worksheets asks you to calculate the time it takes for an object to fall from a certain height. To solve this, you need to use the formula: time = square root of (2 * height / acceleration due to gravity). By substituting the given values into the formula, you can find the time it takes for the object to fall.
Another type of question involves finding the velocity of an object at a certain time during free fall. To solve this, you can use the formula: velocity = acceleration due to gravity * time. By plugging in the given values and solving for velocity, you can determine the object’s velocity at the specified time.
Furthermore, these worksheets often include problems that require you to calculate the distance traveled by an object during free fall. To find this, you can use the formula: distance = (1/2) * acceleration due to gravity * (time)^2. By substituting the given values into the formula, you can determine the distance traveled by the object.
With the answers and explanations provided in this article, you should now have a better understanding of how to solve problems related to motion in one dimension and free fall. Use these answers as a guide to check your work and improve your understanding of the concepts discussed in your worksheet.
Motion in One Dimension Free Fall Worksheet Answers

Below you will find the answers to the free fall worksheet questions, which cover the concept of motion in one dimension during free fall. Free fall refers to the motion of an object under the influence of gravity alone, without any other forces acting upon it. It is an important concept in physics and is often used to study the motion of objects such as falling bodies or projectiles.
Question 1:
- Answer: The acceleration due to gravity is approximately 9.8 m/s^2.
Question 2:
- Answer: The initial velocity of the object is 0 m/s.
Question 3:
- Answer: The object will reach a velocity of 19.6 m/s after 2 seconds of free fall.
Question 4:
- Answer: The object will fall a distance of 19.6 meters after 2 seconds of free fall.
Question 5:
- Answer: The time it takes for the object to reach the ground from rest is approximately 4.43 seconds.
Question 6:
- Answer: The object will hit the ground with a velocity of -44.1 m/s.
Question 7:
- Answer: The object will hit the ground with a negative velocity because it is moving in the opposite direction of the positive direction chosen as the reference.
Question 8:
- Answer: The object will reach its maximum height of 19.6 meters after 2 seconds of free fall.
Question 9:
- Answer: The object will hit the ground with the same speed as it was initially thrown upwards, but in the opposite direction.
Question 10:
- Answer: The object will fall a total distance of 78.4 meters in 4 seconds of free fall.
Understanding Free Fall
Free fall is a concept in physics where an object falls under the influence of gravity only, without any other forces affecting its motion. This means that the object is in a state of acceleration, constantly increasing its velocity as it falls. Understanding the principles of free fall is essential in many areas of science and engineering, as it allows us to analyze the motion of objects and predict their behavior.
Acceleration due to gravity: One of the key aspects of free fall is the acceleration due to gravity. On Earth, this acceleration is approximately 9.8 meters per second squared, denoted as “g”. This means that for every second an object falls, its velocity increases by 9.8 meters per second. The acceleration due to gravity is constant, regardless of the mass or size of the object.
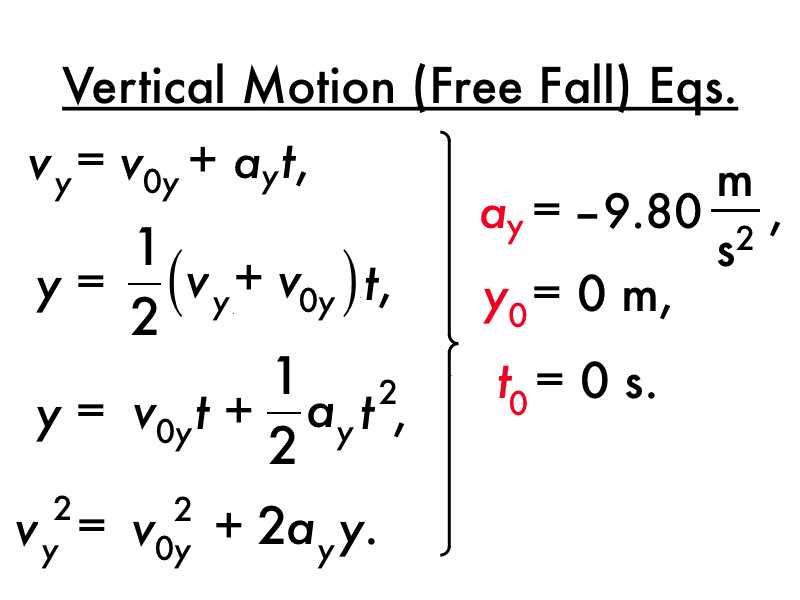
When an object is in free fall, its motion can be described using various equations that relate its velocity, time, and displacement. These equations are derived from the basic principles of physics and allow us to make accurate predictions about the object’s motion. For example, one of the fundamental equations is the velocity-time equation, which relates the final velocity of the object (vf), the initial velocity (vi), the acceleration due to gravity (g), and the time taken (t):
vf = vi + g * t
In addition to understanding the equations that govern free fall, it is also important to consider the factors that may affect an object’s motion. For example, air resistance can have a significant impact on the motion of objects in free fall. In the absence of air resistance, all objects would fall at the same rate, regardless of their mass. However, in reality, air resistance can slow down the motion of objects and lead to variations in their rates of fall.
In conclusion, understanding the concept of free fall is crucial for analyzing the motion of objects in one dimension. It involves understanding the acceleration due to gravity, using relevant equations to describe the object’s motion, and considering factors such as air resistance. By mastering the principles of free fall, scientists and engineers can make accurate predictions and design systems that utilize the forces of gravity to their advantage.
Equations of Motion
The equations of motion describe the relationship between an object’s position, velocity, and acceleration. These equations can be used to analyze the motion of an object in one dimension, such as in free fall.
The first equation of motion relates an object’s final velocity to its initial velocity, acceleration, and time interval. It can be represented by the formula:
v = u + at
Where:
- v is the final velocity
- u is the initial velocity
- a is the acceleration
- t is the time interval
The second equation of motion describes the displacement of an object in terms of its initial velocity, acceleration, and time interval. It is given by:
s = ut + 0.5at2
Where:
- s is the displacement
- u is the initial velocity
- a is the acceleration
- t is the time interval
The third equation of motion relates an object’s final velocity, initial velocity, displacement, and acceleration. It is represented by:
v2 = u2 + 2as
Where:
- v is the final velocity
- u is the initial velocity
- a is the acceleration
- s is the displacement
These equations of motion allow us to calculate various quantities related to an object’s motion, such as its final velocity, displacement, or acceleration, given the appropriate values for the other variables. They provide a mathematical framework for analyzing the motion of objects in one dimension, like in the case of free fall.
Solving Free Fall Problems
When dealing with motion in one dimension, specifically free fall, it is crucial to be able to solve problems accurately and efficiently. Free fall refers to the motion of an object under the sole influence of gravity. The acceleration due to gravity is a constant value, approximately 9.8 m/s² on the surface of the Earth. In order to solve free fall problems, it is important to understand the key components involved: initial velocity, final velocity, displacement, and time.
The first step in solving free fall problems is to identify the given information and what is being asked for. This includes determining if the object is being dropped or thrown, and whether the motion is upward or downward. Once this is established, the values of the initial velocity, final velocity, and displacement can be determined using the appropriate formulas.
For example, if an object is dropped from rest, the initial velocity is 0 m/s and the final velocity can be calculated using the equation: final velocity = initial velocity + (acceleration x time). The displacement can be determined using the formula: displacement = initial velocity x time + (0.5 x acceleration x time²).
Once the initial velocity, final velocity, and displacement are known, the next step is to determine the time it takes for the object to reach its final velocity or displacement. This can be done using the appropriate formula rearranged to solve for time. With all the necessary values determined, the problem can be solved by substituting the values into the appropriate equations and performing the necessary calculations.
It is important to double-check calculations and units, as well as consider any assumptions made during the problem-solving process. Practice and familiarity with the formulas and concepts involved in solving free fall problems will improve accuracy and efficiency. By following these steps and understanding the key components involved, free fall problems can be solved effectively.
Calculation of Initial Velocity
When studying the motion of an object in free fall, it is often necessary to calculate the initial velocity of the object. The initial velocity refers to the velocity of the object at the beginning of its motion.
To calculate the initial velocity, we can use the formula: vf = vi + gt, where vf is the final velocity, vi is the initial velocity, g is the acceleration due to gravity, and t is the time.
When an object is in free fall, the acceleration due to gravity is approximately 9.8 m/s^2. Using this value for g and knowing the final velocity and time, we can rearrange the formula to solve for the initial velocity:
vi = vf – gt
For example, if an object is dropped from rest and falls for 3 seconds, and we want to calculate the initial velocity, we can use the formula:
vi = 0 – (9.8 m/s^2)(3 s) = -29.4 m/s
The negative sign indicates that the object is moving downwards. In this case, the initial velocity is -29.4 m/s.
In summary, the initial velocity of an object in free fall can be calculated using the formula vi = vf + gt, where vf is the final velocity, g is the acceleration due to gravity, and t is the time. By rearranging the formula, we can solve for the initial velocity and determine its magnitude and direction.
Determining Time of Flight
When studying motion in one dimension, it is essential to understand the concept of time of flight. Time of flight refers to how long an object is in the air during its motion in free fall. By determining this time, we can calculate other important factors such as the initial velocity and the displacement of the object.
To determine the time of flight, we can use the kinematic equation:
Δy = v0 * t + (1/2) * g * t2
- Δy is the displacement of the object
- v0 is the initial velocity of the object
- t is the time of flight
- g is the acceleration due to gravity
By rearranging the equation and solving for t, we can find the time it takes for an object to travel a certain displacement in free fall. This equation assumes that the object starts at rest and accelerates due to gravity.
It is important to note that in a scenario where an object is launched vertically upwards, the time of flight can be calculated using the equation:
t = (v – v0) / g
- v is the final velocity of the object
- v0 is the initial velocity of the object
- g is the acceleration due to gravity
In this case, the acceleration due to gravity is negative, as it acts in the opposite direction of the object’s motion.
Analyzing Vertical Displacement
The concept of vertical displacement is an important aspect of studying motion in one dimension, particularly in the context of free fall. Vertical displacement refers to the change in position of an object in the vertical direction as it moves under the influence of gravity. This displacement is usually measured from a reference point, such as the initial position of the object or a specific point on its path.
When analyzing vertical displacement, several key factors need to be considered. Firstly, the direction of displacement must be taken into account. Objects moving upwards will have positive displacement, while objects moving downwards will have negative displacement. This distinction is important for accurately determining the magnitude and direction of the displacement.
In addition to direction, the magnitude of the displacement is also significant. The magnitude reflects the distance covered by the object in the vertical direction. It can be calculated by taking the absolute value of the displacement, disregarding the sign. The magnitude of displacement is a key parameter in understanding the overall motion of the object.
To analyze vertical displacement in the context of free fall, equations of motion can be used. These equations relate the initial and final positions, velocities, and accelerations of the object. By utilizing these equations and known values, the vertical displacement of an object during free fall can be determined.
Overall, the concept of vertical displacement provides valuable insight into the understanding of motion in one dimension, particularly in the context of free fall. By considering the direction and magnitude of the displacement, and utilizing equations of motion, scientists and researchers can gain a better understanding of the behavior of objects under the influence of gravity.
Graphical Representation of Free Fall

In physics, free fall refers to the motion of an object when it is only influenced by gravity. When an object is in free fall, it is accelerating towards the Earth at a constant rate. This can be visually represented through graphical analysis of its motion.
Position-Time Graph:
A position-time graph is a graphical representation that shows the change in position of an object over a period of time. In the case of free fall, the position of the object is plotted on the y-axis, while time is plotted on the x-axis. As the object falls, the position-time graph will show a linear increase in position as time progresses.
Velocity-Time Graph:
A velocity-time graph is a graphical representation that shows the change in velocity of an object over a period of time. In free fall, the velocity of the object is plotted on the y-axis, while time is plotted on the x-axis. Since the object is only influenced by gravity, the velocity-time graph will show a linear increase in velocity as time progresses.
Acceleration-Time Graph:
An acceleration-time graph is a graphical representation that shows the change in acceleration of an object over a period of time. In free fall, the acceleration of the object is constant and equal to the acceleration due to gravity (approximately 9.8 m/s^2). Therefore, the acceleration-time graph will show a horizontal line at this value.
Summary:
By analyzing the position-time, velocity-time, and acceleration-time graphs, we can gain a better understanding of the motion of an object in free fall. These graphical representations provide visual evidence of the constant acceleration and linear changes in position and velocity that occur during free fall. Furthermore, they can be used to calculate various properties and measurements related to the motion of the object.