
In mathematics, functions play a key role in describing and analyzing relationships between variables. Operations and compositions of functions are fundamental concepts in understanding how functions can be combined and manipulated. This article provides an answer key to a maze activity that focuses on operations and compositions of functions.
The maze activity presents a series of mathematical expressions that involve operations such as addition, subtraction, multiplication, and division, as well as compositions of functions. The goal of the maze is to navigate through the various expressions by correctly applying the specified operations and compositions, ultimately arriving at the final answer.
By solving the maze activity, students can practice their skills in performing operations on functions and determining the result of function compositions. This activity helps develop their ability to manipulate mathematical expressions and apply the rules of operations and compositions of functions.
The answer key provided here serves as a guide for students, helping them verify their solutions and ensure they have correctly completed the maze activity. It provides step-by-step explanations of the operations and compositions applied at each stage of the maze, ultimately leading to the final answer. This answer key acts as a valuable resource for both students and teachers, aiding in the understanding and mastery of operations and compositions of functions.
Operations and Compositions of Functions Maze Answer Key
In the study of functions, operations and compositions play a crucial role in understanding how functions can be manipulated and combined to create new functions. The answer key for the Operations and Compositions of Functions Maze provides a detailed guide to solving the maze and finding the correct path.
The answer key starts by outlining the basic operations that can be performed on functions, such as addition, subtraction, multiplication, and division. Each operation is explained using clear examples and step-by-step instructions. The key also highlights important properties of functions, such as the commutative and associative properties, which can be applied when performing operations.
- Addition: To add two functions together, simply add their corresponding values for each input.
- Subtraction: To subtract one function from another, subtract their corresponding values for each input.
- Multiplication: To multiply two functions, multiply their corresponding values for each input.
- Division: To divide one function by another, divide their corresponding values for each input (excluding any inputs where the divisor is equal to zero).
The answer key also demonstrates how to compose functions, which involves plugging one function into another. The key provides examples of how to compose functions and explains the order in which the functions are applied. It also emphasizes the importance of understanding the domain and range of each function when composing them.
Overall, the Operations and Compositions of Functions Maze Answer Key serves as a valuable resource for students and teachers alike, providing a comprehensive guide to understanding and applying the operations and compositions of functions. By following the steps outlined in the key, students can successfully navigate through the maze and gain a deeper understanding of these fundamental concepts in mathematics.
What are Operations and Compositions of Functions?
Operations and compositions of functions are two fundamental concepts in mathematics that allow us to manipulate and combine functions to create new functions. Understanding these concepts is essential in various branches of mathematics, such as calculus, algebra, and analysis.
In mathematics, an operation refers to a rule or procedure that takes one or more inputs and produces an output. When applied to functions, operations can modify or transform the functions in various ways. Some common operations on functions include addition, subtraction, multiplication, division, composition, and inversion.
Compositions of functions, on the other hand, involve combining two or more functions to create a new function. The composed function takes an input, applies the first function to it, and then applies the second function to the result. This process can be repeated multiple times with different functions to create complex compositions.
Operations and compositions of functions allow mathematicians to analyze and manipulate functions in powerful ways. They enable us to solve equations, find derivatives and integrals, simplify expressions, and explore the behavior of functions. These concepts are also essential in applications such as physics, engineering, economics, and computer science, where functions are used to model and solve real-world problems.
Understanding the Maze Activity
In the “Operations and Compositions of Functions Maze” activity, students are presented with a maze puzzle that requires them to navigate a path by solving various mathematical problems. The activity is designed to engage students in practicing their understanding of operations and compositions of functions while also challenging their problem-solving skills.
The maze consists of a series of interconnected pathways, each labeled with a mathematical expression or equation. Students must carefully analyze each expression and apply the appropriate operations to solve for the unknown values or to evaluate the composed functions. By correctly solving each problem, students can determine which pathway to choose in order to reach the final destination of the maze.
The activity encourages students to think critically and apply their knowledge of operations and compositions of functions in a practical and interactive way. It requires them to use problem-solving strategies to navigate through the maze, making connections between different mathematical concepts and applying them to real-world scenarios. This activity helps students develop their analytical and logical thinking skills, as well as their ability to interpret and apply mathematical operations effectively.
By engaging in the “Operations and Compositions of Functions Maze” activity, students can deepen their understanding of these mathematical concepts and build their confidence in solving complex problems. The activity provides a hands-on approach to learning and allows students to actively participate in their own learning process. It also promotes collaboration and communication among students as they work together to solve the maze and discuss their strategies for reaching the solution.
Step-by-Step Solution
Here is a step-by-step solution to the Operations and Compositions of Functions Maze:
1. Start at the beginning
Begin by starting at the entrance of the maze. Look for the first function listed on the maze and locate the corresponding starting point on the diagram.
2. Follow the path
Using the given function, move along the path in the maze according to the operations and compositions indicated. Each step should correspond to the next function on the list.
3. Perform the indicated operations
As you move along the path, perform the operations indicated for each function. This may involve addition, subtraction, multiplication, division, or composition of functions.
4. Find the destination
Continue following the path until you reach the end of the maze. The destination should be indicated on the diagram as the final point.
5. Check your solution
Once you have reached the destination, check your solution to ensure that you have correctly performed all the operations and compositions of functions. Verify that the final result matches the answer key provided.
By following these step-by-step instructions, you should be able to successfully navigate the Operations and Compositions of Functions Maze and find the correct solution.
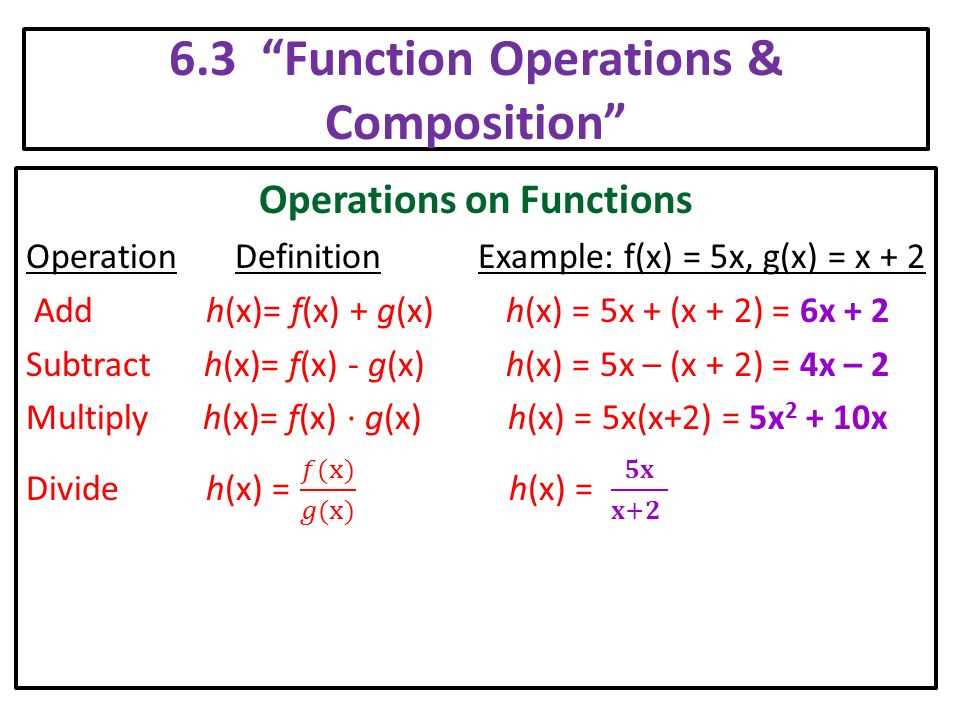
Basic Operations of Functions
The study of functions is a fundamental part of mathematics and plays a crucial role in various fields. Functions are mathematical entities that map an input value to an output value. They can be combined and manipulated using basic operations to create new functions.
One of the basic operations of functions is composition. Composition allows us to take the output of one function and use it as the input of another function. This is done by substituting the output value of the first function into the input of the second function. The composition of two functions, f and g, is denoted as (g o f)(x), which means “g of f of x”.
Another operation of functions is addition and subtraction. Given two functions, f and g, we can add them together or subtract one from the other. This results in a new function, called the sum or difference of the two functions, respectively. The sum of two functions, f + g, is obtained by adding the corresponding output values of the functions for each input value. Similarly, the difference of two functions, f – g, is obtained by subtracting the corresponding output values.
Additionally, functions can be multiplied or divided. When we multiply two functions, f and g, the resulting function, fg, is obtained by multiplying the output values of the functions for each input value. Division of functions is similar, where one function is divided by the other. The resulting function, f/g, is obtained by dividing the output values.
In summary, the basic operations of functions include composition, addition, subtraction, multiplication, and division. These operations allow us to manipulate functions and create new functions. Understanding and applying these operations is essential for solving problems involving functions and analyzing their properties.
Advanced Operations of Functions
When working with functions, we often need to perform more than just basic operations such as addition, subtraction, multiplication, and division. Advanced operations of functions involve compositions, transformations, and combinations to create new functions with unique properties.
One important concept in advanced function operations is composition. Composition of functions is the process of combining two or more functions to create a new function. This is done by substituting the output of one function into another function. For example, if we have two functions f(x) and g(x), the composition of these functions can be represented as (f ∘ g)(x) or f(g(x)). This allows us to apply multiple functions in a specific order to achieve a desired outcome.
Another advanced operation is the transformation of functions. Transformations involve shifting, stretching, shrinking, and reflecting functions to manipulate their graphs. These transformations can be applied to the input or output values of a function, resulting in a change in its shape and position. For instance, adding a constant value to the input of a function shifts the graph horizontally, while multiplying the input by a constant value stretches or shrinks the graph.
In addition to compositions and transformations, we can also combine functions using operations such as addition, subtraction, multiplication, and division. When performing these operations, we apply them to corresponding input and output values of the functions. For example, if we have two functions f(x) = 2x and g(x) = 3x, the sum of these functions would be h(x) = f(x) + g(x) = 2x + 3x = 5x.
In conclusion, advanced operations of functions involve compositions, transformations, and combinations to create new functions with unique properties. These operations allow us to manipulate functions in various ways, enabling us to solve complex mathematical problems and analyze real-world situations from a functional perspective.
Tips and Tricks for Solving the Maze
Solving a maze involving operations and compositions of functions may seem challenging at first, but with the right approach, it can be a fun and rewarding experience. Here are some tips and tricks to help you navigate through the maze:
1. Understand the given functions
Before attempting to solve the maze, take the time to thoroughly understand each function and its properties. Pay attention to the input and output values, as well as any restrictions or special rules that apply to the functions. This will give you a solid foundation to work with.
2. Identify the starting point and goal
Each maze has a starting point and a goal. Take note of these and keep them in mind as you progress through the maze. Knowing where you are starting from and where you need to go will help you make progress in the right direction.
3. Work backwards from the goal
Instead of starting from the beginning and trying to figure out the path forward, it can be helpful to work backwards from the goal. Find the inverse of the goal function and apply it to the desired output. This will give you the input value that you need to reach in order to solve the maze.
4. Look for patterns and shortcuts
As you navigate through the maze, keep an eye out for patterns and shortcuts that can help you simplify the calculations. Look for common functions or compositions that can be simplified or combined to reduce the complexity of the problem.
5. Be systematic and organized
When solving the maze, it’s important to stay organized and keep track of your progress. Use a systematic approach, such as creating a table or a flowchart, to map out the path you are taking and keep track of the intermediate steps.
By following these tips and tricks, you can approach the maze with confidence and effectively solve the operations and compositions of functions puzzle.
Common Mistakes to Avoid
When working with operations and compositions of functions, it is important to be aware of common mistakes that students often make. By being mindful of these mistakes, you can avoid them and improve your understanding of the topic.
1. Neglecting the order of operations: One common mistake is neglecting the order of operations when performing calculations. It is crucial to remember and apply the rules of parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right). Failing to follow the correct order will lead to incorrect results.
2. Misinterpreting function composition: Another mistake is misinterpreting the concept of function composition. Function composition involves applying one function to the output of another function. It is important to clearly understand the order in which the functions are applied and properly substitute the variables. Failing to do so can lead to incorrect compositions.
- 3. Forgetting to simplify expressions: It is important to simplify expressions as much as possible before performing any operations. Skipping this step can lead to complex calculations and potential errors. Always simplify the expressions first to make the calculations easier.
- 4. Confusing domain and range: The domain of a function consists of all possible input values, while the range consists of all possible output values. One common mistake is confusing these two concepts. It is crucial to correctly identify and understand the domain and range to accurately analyze and work with functions.