In the study of geometry, parallel lines and transversals are essential concepts to understand. These concepts play a crucial role in determining various angles and their relationships. To help students grasp these concepts more effectively, a project called “Parallel Lines and Transversals Project City Map” is often introduced in classrooms.
The project involves creating a fictional city map that incorporates parallel lines and transversals. Students are given the task of designing streets, buildings, and landmarks in a way that showcases parallel lines and transversals. The city map can be both creative and informative, giving students an opportunity to demonstrate their understanding of these geometric principles.
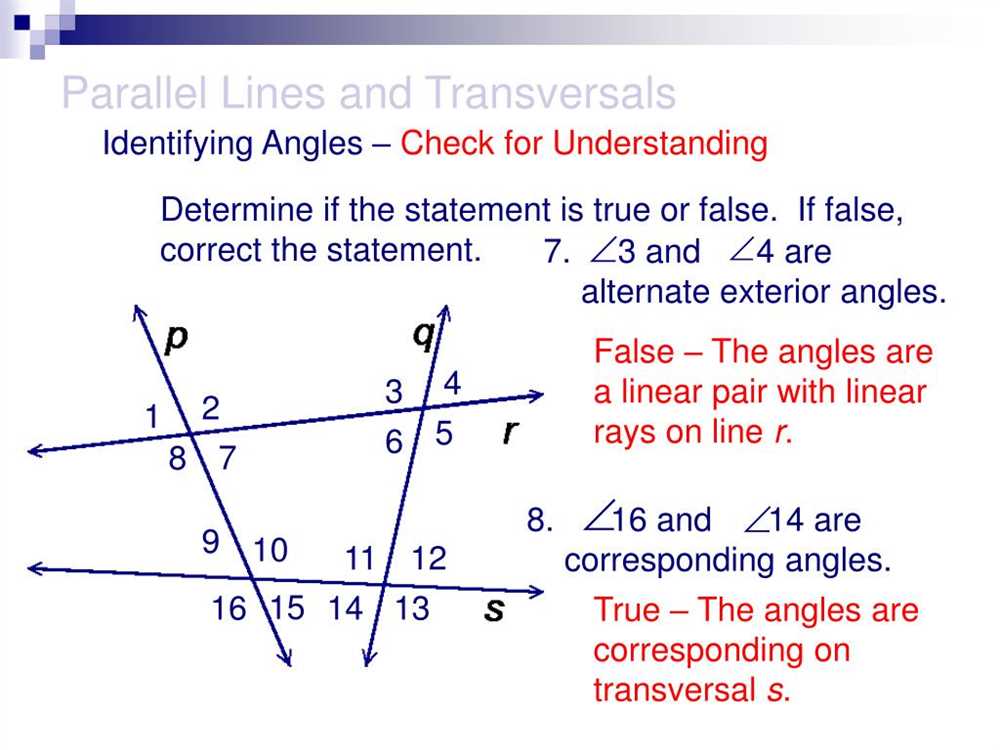
The answer key for the “Parallel Lines and Transversals Project City Map” provides guidance and examples for students to refer to. It showcases correctly drawn parallel lines and transversals in various sections of the city map, as well as the corresponding angles formed by them. This answer key serves as a valuable reference tool for students to check their work and gain a better understanding of the topic.
By completing this project and using the answer key as a guide, students are able to deepen their comprehension of parallel lines and transversals. They become more proficient in identifying angles and understanding their relationships when encountering parallel lines and transversals in real-world scenarios. This hands-on approach to learning geometry helps foster creativity, critical thinking, and problem-solving skills.
Parallel Lines and Transversals Project City Map Answer Key
In this project, we were tasked with creating a city map that demonstrates the concepts of parallel lines and transversals. Our goal was to incorporate various examples of parallel lines and transversals in a real-world context. By doing so, we aimed to enhance our understanding of these geometric principles and their applications.
The city map consists of several streets and intersections, each designed to represent different scenarios involving parallel lines and transversals. The key to understanding the map lies in identifying the various elements and their relationships. For example, parallel streets are represented by lines that never intersect, while transversals are depicted as streets that cut across multiple parallel lines.
One example on the city map is a four-way intersection where two parallel streets intersect with a transversal street. This demonstrates the concept of alternate interior angles, which states that when two parallel lines are cut by a transversal, the alternate interior angles formed are congruent. By identifying the angles at this intersection, we can observe this principle in action.
In another part of the city, there is a pair of parallel streets with a transversal street intersecting them. Here, we can see the concept of corresponding angles, which states that when two parallel lines are cut by a transversal, the corresponding angles formed are congruent. By identifying these angles, we can further solidify our understanding of this geometric theorem.
Overall, the city map project provides a hands-on and visual approach to learning about parallel lines and transversals. It allows us to apply these concepts in a practical setting and reinforces our understanding of the principles involved. By analyzing the various elements and relationships on the map, we can enhance our geometrical knowledge and develop a deeper appreciation for the role of parallel lines and transversals in our everyday lives.
Understanding Parallel Lines and Transversals
Parallel lines and transversals are fundamental concepts in geometry that help us understand the relationships between different lines. Parallel lines are lines that never intersect and remain equidistant from each other at all points. Transversals are lines that intersect two or more other lines. When a transversal intersects two parallel lines, it creates several angles and line segments with specific properties.
One of the key concepts in parallel lines and transversals is the angle relationships that are formed when a transversal intersects parallel lines. These angle relationships include alternate interior angles, corresponding angles, alternate exterior angles, and consecutive interior angles. Understanding these relationships can help us find missing angles and solve geometry problems more efficiently.
Parallel lines and transversals have many real-life applications, such as in city planning and road design. Engineers and architects often use parallel lines and transversals to create intersections, road networks, and city maps. By understanding the angle relationships formed by transversals and parallel lines, they can design efficient and safe transportation systems.
In conclusion, understanding parallel lines and transversals is essential in geometry and has practical applications in various fields. By studying the properties and relationships of parallel lines and transversals, we can solve geometry problems and create efficient designs in real-life situations.
Exploring the City Map Project

In the Parallel Lines and Transversals Project City Map, we are presented with a map that is divided into several streets and intersections. The map serves as a visual representation of parallel lines and transversals in a real-life context. By examining the map, we can identify various examples of parallel lines and transversals, which are fundamental concepts in geometry.
As we explore the city map project, we can see that the streets on the map have been labeled with letters, such as street A, street B, and so on. Each street is parallel to one or more other streets, creating a network of parallel lines throughout the map. Additionally, there are intersections where two or more streets meet, forming transversals. These intersections provide opportunities to observe the angles formed by the intersecting lines.
One interesting feature of the city map project is the presence of different buildings and landmarks along the streets. These buildings and landmarks add depth and realism to the map, allowing us to imagine the map as a real city. By analyzing the angles formed by the streets and the buildings or landmarks, we can further explore the concept of angle relationships in parallel lines and transversals.
To make the exploration of the city map project more engaging, we can create a list of specific examples of parallel lines and transversals found in the map. For instance, we can identify a pair of parallel streets, such as street C and street D, and determine the angle relationships formed by the transversal streets. Moreover, we can highlight the buildings or landmarks that are located at the intersections, and analyze the angles formed by the streets that intersect at those particular points.
In conclusion, the Parallel Lines and Transversals Project City Map offers an interactive and visual way to understand the concepts of parallel lines and transversals. By exploring the map and identifying examples of parallel lines and transversals, we can deepen our understanding of geometry and the relationships between intersecting lines. Through this project, we can also appreciate the real-life applications of these geometric concepts in the layout and design of cities.
Identifying Parallel Lines and Transversals

When studying geometry, it is important to be able to identify parallel lines and transversals. Parallel lines are two lines that never intersect and are always the same distance apart. Transversals, on the other hand, are lines that intersect two or more parallel lines. By understanding these concepts, we can draw accurate diagrams and solve problems related to parallel lines and transversals.
Identifying parallel lines is relatively simple. When given multiple lines, look for pairs of lines that are equidistant from each other and do not intersect. If this condition is met, we can confidently say that the lines are parallel. It is often helpful to use a ruler or a straightedge to measure the distance between the lines and verify their parallel nature. Parallel lines are denoted with arrows placed on top of the lines, indicating their direction.
Transversals can be identified when a line intersects two or more parallel lines. The transversal line can be any line that crosses the parallel lines at different points. When a transversal line intersects two parallel lines, it creates several pairs of congruent angles. These angles are known as corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles. By identifying these angles, we can make connections and solve problems related to the parallel lines and the transversal.
In conclusion, understanding how to identify parallel lines and transversals is crucial in the study of geometry. By being able to recognize these lines, we can accurately analyze and solve problems involving parallel lines and transversals. With practice and familiarity, these concepts become easier to understand and apply in various geometric situations.
Determining Angle Relationships
When working with parallel lines and transversals, it is important to understand the various angle relationships that exist. These angle relationships can help us solve problems and make predictions about the measurements of angles. By examining the intersecting lines and the angles formed, we can determine the types of angle relationships present in a given scenario.
- Corresponding Angles: When a transversal intersects two parallel lines, corresponding angles are formed. These angles are congruent and are located in corresponding positions on the lines.
- Alternate Interior Angles: When a transversal intersects two parallel lines, alternate interior angles are formed. These angles are congruent and are located on opposite sides of the transversal and between the parallel lines.
- Alternate Exterior Angles: When a transversal intersects two parallel lines, alternate exterior angles are formed. These angles are congruent and are located on opposite sides of the transversal and outside the parallel lines.
- Same-Side Interior Angles: When a transversal intersects two parallel lines, same-side interior angles are formed. These angles are supplementary and are located on the same side of the transversal and between the parallel lines.
Understanding these angle relationships can greatly assist in solving problems involving parallel lines and transversals. By identifying the types of angles formed and applying the properties associated with each relationship, it is possible to find missing angle measurements and solve for unknown variables. These angle relationships are fundamental concepts in geometry and are applicable in various real-life scenarios, such as constructing buildings, designing road systems, and analyzing patterns in nature.
Solving Problems with Parallel Lines and Transversals
Parallel lines and transversals are fundamental concepts in geometry. They provide a framework for solving various problems involving angles and lines. By understanding the properties and relationships of parallel lines and transversals, we can solve problems involving angle measurements, congruency, similarity, and more.
When working with parallel lines and transversals, one of the key concepts to understand is the corresponding angles. Corresponding angles are formed when a transversal intersects two parallel lines. They are located in the same position relative to the transversal and have equal measures. By identifying corresponding angles, we can solve equations and find missing angle measures.
Another important concept is the interior and exterior angles formed by parallel lines and transversals. Interior angles are located between the two parallel lines and the transversal, while exterior angles are located on the outside of the parallel lines and transversal. Interior angles have measures that add up to 180 degrees, while exterior angles have measures that add up to 360 degrees. By understanding these properties, we can solve problems involving angle relationships and measurements.
Parallel lines and transversals are also used to solve problems involving congruent and similar triangles. By identifying parallel lines and transversals, we can determine if certain triangles are congruent or similar. This allows us to apply properties and theorems related to congruent and similar triangles to solve complex geometry problems.
In conclusion, parallel lines and transversals provide a powerful tool for solving problems in geometry. By understanding the properties and relationships of these lines, we can find missing angle measures, determine congruency and similarity, and solve a wide range of geometry problems.
Applying Parallel Lines and Transversals in Real Life

Parallel lines and transversals are not just mathematical concepts that we learn in school; they have real-life applications that we encounter every day. Understanding how parallel lines and transversals work can help us navigate and make sense of various situations in the real world.
One practical application of parallel lines and transversals is in architecture and construction. Architects use parallel lines to create symmetrical designs and ensure that structures are stable and balanced. By using transversals, they can determine the angles and lengths of different components of a building, such as beams and columns.
Another area where parallel lines and transversals come into play is in transportation and urban planning. When designing road networks or railway systems, engineers use parallel lines to create multiple lanes or tracks. Transversals, in this case, represent intersections or junctions where different roads or railways intersect. Understanding the angles formed by the transversals can help engineers design safe and efficient transportation systems.
-
The concept of parallel lines and transversals can also be applied in navigation and mapping. When reading a map, we often encounter parallel lines representing latitude and longitude. By understanding the angles formed by the intersections of these lines, we can determine our exact location and navigate to our desired destination.
-
Moreover, parallel lines and transversals are used in computer graphics and image processing. When creating digital graphics or editing images, software algorithms utilize parallel lines and transversals to define perspective and create realistic 3D effects.
In conclusion, parallel lines and transversals have numerous practical applications in various fields, ranging from architecture and construction to transportation and mapping. By understanding and applying these concepts, we can solve real-life problems and enhance our understanding of the world around us.