Polynomials and rational functions are essential topics in algebra and are frequently encountered in various mathematical problems. This article provides the answer key for the mid-unit assessment on polynomials and rational functions, allowing students to check their understanding and accuracy in solving problems in these areas.
The assessment covers a range of concepts, including polynomial operations, factoring polynomials, solving polynomial equations, graphing polynomial functions, and simplifying rational expressions. By practicing and understanding these concepts, students can enhance their problem-solving skills and gain a deeper understanding of polynomial and rational function properties.
The answer key presented here serves as a valuable resource for students, enabling them to compare their answers with the provided solutions. It offers a step-by-step explanation of how to solve each problem, ensuring that students can identify any mistakes made and make necessary corrections. Moreover, it allows them to gain insights into the logical reasoning and techniques used to obtain the correct solutions.
Polynomials and Rational Functions Mid Unit Assessment Answer Key
In the mid-unit assessment for the topic of polynomials and rational functions, students were evaluated on their understanding and application of key concepts in this area of mathematics. The assessment covered topics such as finding zeros of polynomial functions, identifying the behavior of polynomial graphs, and simplifying rational expressions.
The answer key for the assessment provides solutions and explanations for each question, allowing students to review their work and learn from their mistakes. In question 1, for example, students were asked to find the zeros of the polynomial function f(x) = x^3 – 4x^2 – 3x + 18. The answer key would show the correct method of factoring or using the rational root theorem to determine the zeros of the function.
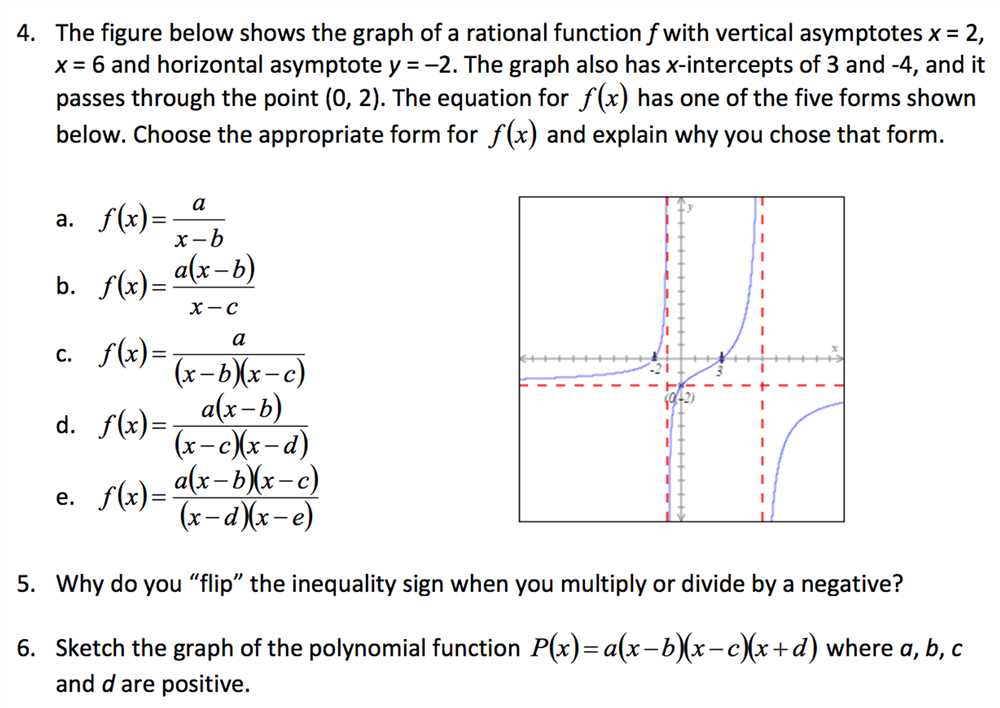
The answer key also provides guidance for interpreting and analyzing graphs of polynomial functions. In question 2, students were given a graph and asked to identify the behavior of the polynomial function represented by the graph. The answer key would provide explanations of how to determine the end behavior, the number of real zeros, and any symmetry or other characteristics of the graph.
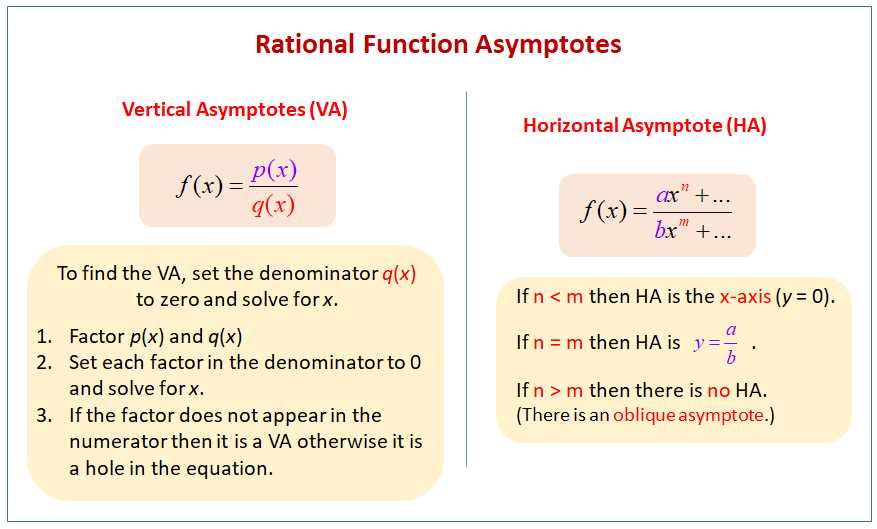
For rational functions, the answer key assists students in simplifying and solving these types of expressions. In question 3, students may have been asked to simplify the rational expression (x^2 + 9)/(x^2 – 16). The answer key would show the steps for factoring and canceling common factors to arrive at the simplified expression.
Overall, the answer key for the polynomials and rational functions mid-unit assessment serves as a valuable tool for students to check their work, understand the correct methods and approaches to solving problems, and reinforce their understanding of key concepts in this area of mathematics.
Understanding Polynomials
In mathematics, a polynomial is an algebraic expression consisting of variables raised to non-negative integer exponents and multiplied by coefficients. It is a fundamental concept in algebra and provides a way to represent and manipulate mathematical functions. Polynomials can be used to model a wide range of real-world phenomena, from the growth of populations to the trajectory of a projectile.
A polynomial function is an equation in which the unknown variable appears as a polynomial. The degree of a polynomial is the highest power of the variable in the equation. For example, the polynomial function f(x) = 3x^2 – 2x + 1 has a degree of 2, since the variable x is raised to the power of 2. The coefficients of the polynomial represent the weights or magnitudes of the different terms.
- Polynomials can be added, subtracted, multiplied, and divided, allowing for various operations and transformations.
- The roots of a polynomial are the values of the variable that make the polynomial equal to zero, and they can be found through methods such as factoring, synthetic division, or using the quadratic formula for quadratic polynomials.
- Polynomials can also be graphed to visualize their behavior and see patterns and trends.
Understanding polynomials is important for solving equations, analyzing functions, and making predictions based on mathematical models. They are extensively used in fields such as physics, engineering, finance, and computer science. By mastering the concepts of polynomials, students can enhance their analytical and problem-solving skills, as well as develop a deeper understanding of various mathematical concepts.
Evaluating Polynomial Expressions
In algebra, a polynomial expression is an expression that consists of variables, coefficients, and exponents, combined using addition, subtraction, and multiplication. Evaluating polynomial expressions involves substituting specific values for the variables and simplifying the expression to find the resulting value. This process allows us to determine the value of a polynomial expression for a given set of values.
To evaluate a polynomial expression, we follow a simple process. First, we substitute the given values for the variables in the expression. Then, we simplify the expression by performing the indicated operations, such as addition, subtraction, and multiplication. Finally, we obtain the resulting value, which gives us the evaluation of the polynomial expression.
For example, let’s consider the polynomial expression: 2x^2 + 3x – 5. If we are asked to evaluate this expression for x = 4, we substitute x = 4 into the expression:
2(4)^2 + 3(4) – 5.
Performing the indicated operations, we simplify the expression to:
2(16) + 12 – 5 = 32 + 12 – 5 = 39.
Therefore, the value of the polynomial expression 2x^2 + 3x – 5 for x = 4 is 39.
Evaluating polynomial expressions is an essential skill in algebra as it allows us to find the value of a given expression for specific values of the variables. This process is used in various applications, including solving equations, graphing polynomial functions, and analyzing data. By mastering the technique of evaluating polynomial expressions, we can gain a deeper understanding of the behavior and properties of polynomials. It enables us to solve real-world problems, make predictions, and make informed decisions based on mathematical analysis.
Applying Polynomial Properties
Polynomials are mathematical expressions consisting of variables and coefficients, combined using addition, subtraction, and multiplication. They play a fundamental role in algebra and are used to model a wide range of real-world phenomena. Understanding the properties of polynomials is essential for effectively working with them and solving polynomial equations.
One important property of polynomials is the degree, which represents the highest power of the variable in the expression. The degree determines the behavior of the polynomial, such as its end behavior and the number of solutions it has. By analyzing the degree of a polynomial, we can gain insight into its characteristics and make predictions about its behavior.
In addition to the degree, polynomials can also have specific properties based on their coefficients. For example, the leading coefficient, which is the coefficient of the term with the highest power of the variable, can determine whether the polynomial opens upward or downward. If the leading coefficient is positive, the polynomial opens upward, and if it is negative, the polynomial opens downward. This property helps us understand the overall shape of the polynomial’s graph.
Furthermore, the zeros or roots of a polynomial are the values of the variable that make the polynomial equal to zero. Finding the zeros of a polynomial is crucial for solving polynomial equations and understanding the behavior of the polynomial. These zeros often correspond to important points on the graph of the polynomial and can help us identify key features such as x-intercepts and turning points.
In conclusion, understanding and applying polynomial properties is essential for analyzing and solving polynomial equations. By considering the degree, leading coefficient, and zeros of a polynomial, we can gain valuable insights into its behavior and characteristics. This knowledge allows us to make predictions, solve equations, and effectively use polynomials to model real-world situations.
Graphing Polynomial Functions

When graphing polynomial functions, it is important to understand the key components and characteristics of these functions. One key aspect is the degree of the polynomial, which is determined by the highest power of the variable in the function. The degree of a polynomial helps to determine the shape and behavior of its graph.
Another important consideration when graphing polynomial functions is the end behavior. The end behavior refers to the behavior of the graph as the input values approach positive or negative infinity. By examining the leading coefficient and degree of the polynomial, we can determine if the graph goes up or down on either side.
It is also important to identify the x-intercepts and y-intercept of the polynomial function. The x-intercepts are the points where the graph intersects the x-axis, while the y-intercept is the point where the graph intersects the y-axis. By finding these intercepts, we can better understand the shape and positioning of the graph.
Furthermore, the graph of a polynomial function can exhibit various features such as symmetry and local extrema. Symmetry can be determined by analyzing the degree and coefficients of the polynomial function. Local extrema refers to the maximum and minimum points on the graph, which can be found by analyzing the critical points and the concavity of the function.
Overall, graphing polynomial functions requires an understanding of their degree, end behavior, intercepts, symmetry, and local extrema. By analyzing these key components, we can accurately sketch the graph and gain valuable insights into the behavior of the function.
Factoring Polynomial Expressions
Factoring polynomial expressions is an essential skill in algebra. It involves breaking down a polynomial into its simplest form by finding its factors. By factoring a polynomial, we can determine its roots, simplify expressions, and solve equations.
The process of factoring involves looking for common factors, such as a binomial or trinomial, that can be divided into the polynomial. The goal is to rewrite the polynomial as the product of these factors. This allows us to simplify the expression and potentially solve for unknowns or identify patterns within the equation.
There are several methods for factoring polynomial expressions, including factoring by grouping, factoring by using the difference of squares, or factoring by using the sum or difference of cubes. Additionally, we can use the rational root theorem to find the possible rational roots of a polynomial equation, which can then be factored further using synthetic division or long division.
Factoring polynomial expressions is an important tool in many areas of mathematics, including algebra, calculus, and number theory. It allows us to manipulate and solve equations more easily, giving us a deeper understanding of the underlying concepts. It is also a crucial skill in applied fields such as physics and engineering, where polynomial equations are frequently encountered.
Overall, factoring polynomial expressions is an essential skill for any student studying mathematics. It provides a foundation for solving equations, simplifying expressions, and understanding the behavior of polynomial functions. By mastering this skill, students can approach more complex mathematical problems with confidence and precision.
Solving Polynomial Equations
In algebra, a polynomial equation is an equation that involves variables raised to non-negative integer powers and consists of terms that are added or subtracted from each other. Solving polynomial equations involves finding the values of the variables that make the equation true.
One method for solving polynomial equations is factoring. To use factoring, one must express the polynomial as a product of linear factors. By setting each factor equal to zero and solving for the variable, the solutions to the equation can be found.
Another method for solving polynomial equations is using the rational root theorem. This theorem states that any rational root of a polynomial equation in the form P(x) = a_nx^n + a_{n-1}x^{n-1} + … + a_1x + a_0, where a_n, a_{n-1}, …, a_1, a_0 are integers, is of the form p/q, where p is a factor of a_0 and q is a factor of a_n. By testing different values of p and q, the rational roots of the equation can be found, and from there, the remaining polynomial factors can be determined.
For higher degree polynomials that cannot be easily factored or have rational roots, one can use numerical methods such as Newton’s method or the bisection method to approximate the solutions. These methods involve iteratively refining an initial guess until the desired level of accuracy is achieved.
Solving polynomial equations is an important skill in algebra and has applications in many areas of mathematics and science. By finding the solutions to polynomial equations, we can understand the behavior of functions, solve real-world problems, and make accurate predictions.
Solving Rational Equations
When solving rational equations, the first step is to find the LCD (Least Common Denominator) of all the fractions in the equation. This will allow us to eliminate the fractions and work with whole numbers instead. To find the LCD, we need to factor the denominators of all the fractions and choose the highest power of each factor.
Once we have the LCD, we multiply every term of the equation by the LCD to eliminate the fractions. This step is called clearing the fractions. After clearing the fractions, we end up with a polynomial equation, which we can solve using algebraic techniques such as factoring, the quadratic formula, or completing the square.
When solving rational equations, it’s important to check our solutions by substituting them back into the original equation. Sometimes, the solutions we get may not be valid due to potential restrictions on the variables. If we get any solutions that make the denominator equal to zero, we need to discard them as they would result in undefined values.
In summary, solving rational equations involves finding the LCD, clearing the fractions, solving the resulting polynomial equation, and checking the solutions for validity. Following these steps will help us find the accurate solutions to rational equations and avoid any potential pitfalls.