
When it comes to solving logarithmic equations and understanding their properties, having access to a reliable worksheet with correct answers is essential. A properties of logarithms worksheet plays a crucial role in helping students grasp the fundamental concepts required for mastering logarithmic equations.
Logarithms are mathematical functions that are the inverse of exponential functions. They are widely used in various scientific fields, finance, and computer science. Working with logarithms requires a solid understanding of their properties, including the product rule, the quotient rule, the power rule, and the change of base rule.
Having a worksheet with answers that carefully explains these properties can make the learning process much more efficient. It provides students with the opportunity to practice applying the rules and consolidating their understanding of logarithmic equations. By seeing the correct answers and the step-by-step solutions, students can identify their mistakes and learn from them.
Logarithm Properties
Logarithms are mathematical functions that can be used to solve equations involving exponential growth and decay. They are especially useful in fields such as finance, engineering, and computer science. Understanding the properties of logarithms is essential for working with these functions effectively.
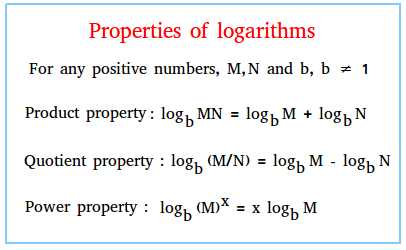
Property 1: Product Rule
The product rule states that the logarithm of a product is equal to the sum of the logarithms of the individual factors. In mathematical terms, if a and b are positive numbers, then logb(a * b) = logb(a) + logb(b). This property allows us to simplify complex equations by breaking them down into simpler components.
Property 2: Quotient Rule
The quotient rule states that the logarithm of a quotient is equal to the difference of the logarithms of the numerator and denominator. In other words, if a and b are positive numbers, then logb(a / b) = logb(a) – logb(b). This property allows us to convert division problems into subtraction problems, making them easier to solve.
Property 3: Power Rule
The power rule states that the logarithm of a number raised to a power is equal to the product of that power and the logarithm of the number. In mathematical terms, if a is a positive number and n is any real number, then logb(an) = n * logb(a). This property allows us to move exponents out of logarithms, simplifying equations and making them more manageable.
Property 4: Change of Base Formula
The change of base formula allows us to convert logarithms with one base to logarithms with another base. If a and b are positive numbers, and c is any positive real number not equal to 1, then logb(a) = logc(a) / logc(b). This property is useful when working with logarithms of different bases and allows us to make comparisons and calculations across different logarithmic scales.
These properties of logarithms form the foundation for solving logarithmic equations and manipulating logarithmic expressions. By understanding and applying these properties, we can efficiently solve problems involving exponential growth, decay, and other logarithmic functions.
Product Property of Logarithms
The product property of logarithms is an essential concept in logarithmic functions. It allows us to simplify expressions involving multiplication by applying logarithmic properties. The product property states that when two numbers are multiplied together, the logarithm of their product is equal to the sum of their individual logarithms.
In mathematical terms, if we have two numbers, a and b, and their logarithms are represented as log base x of a and log base x of b respectively, then the product property states:
log base x of (a * b) = log base x of a + log base x of b
This property is helpful when dealing with complex calculations that involve multiplication or division. By applying the product property, we can break down an expression into separate logarithms and then simplify the calculation.
For example, let’s say we have the expression log base 2 of (5 * 7). We can use the product property to simplify this expression as follows:
log base 2 of (5 * 7) = log base 2 of 5 + log base 2 of 7
By applying the product property, we can now evaluate the individual logarithms and compute the final result. This property is particularly useful in solving logarithmic equations and in various applications of logarithmic functions, such as finance, physics, and computer science.
Quotient Property of Logarithms

The quotient property of logarithms is a useful property that allows us to simplify logarithmic expressions that involve division. It states that the logarithm of the quotient of two numbers is equal to the difference of the logarithms of the individual numbers.
Mathematically, the quotient property of logarithms can be expressed as:
logb(x/y) = logb(x) – logb(y)
Here, logb(x/y) represents the logarithm of the quotient of x and y to the base b, while logb(x) and logb(y) represent the logarithms of x and y respectively to the base b.
Using the quotient property of logarithms, we can simplify complex logarithmic expressions by breaking them down into simpler terms. We can then apply the properties of logarithms, such as the product property or the power property, to further simplify the expression.
For example, let’s consider the expression log2(8/4). Using the quotient property of logarithms, we can rewrite the expression as log2(8) – log2(4). Further simplifying, we get 3 – 2 = 1. Therefore, log2(8/4) = 1.
The quotient property of logarithms provides a valuable tool for manipulating logarithmic expressions and solving various mathematical problems involving logarithms in a more convenient and efficient manner.
Power Property of Logarithms
The power property of logarithms is a useful tool that enables us to simplify calculations involving exponents and logarithms. It states that when raising a number to a power and taking the logarithm of the result, we can instead multiply the exponent by the logarithm of the base.
Mathematically, the power property of logarithms can be expressed as:
logb(xy) = y * logb(x)
This property is helpful in situations where we need to evaluate expressions that involve both exponents and logarithms. By using the power property, we can convert the exponent into a coefficient and simplify the calculation.
For example, if we have the expression log2(83), we can apply the power property to simplify it as follows:
log2(83) = 3 * log2(8) = 3 * log2(23) = 3 * 3 = 9
This shows that log2(83) is equal to 9, which is much simpler to compute compared to evaluating the original expression directly.
The power property of logarithms is an essential tool in logarithmic calculations and plays a crucial role in various fields, including mathematics, physics, and engineering.
Change of Base Formula
The change of base formula is a useful tool in logarithmic calculations that allows us to evaluate logarithms with a base other than a common base, such as 10 or e. The formula states that:
logb(x) = loga(x) / loga(b)
Here, a and b represent the bases of logarithms, and x is the number we want to calculate the logarithm of. This formula allows us to convert logarithms with one base to logarithms with another base, making calculations easier.
The change of base formula is particularly helpful when working with logarithms in practical applications, such as in scientific research, engineering, or financial analysis. It allows us to work with different bases depending on the context of the problem and the available tools or data.
To use the change of base formula, we first calculate the logarithm of the number with a base that we have available (e.g., loga(x)), and then divide this result by the logarithm of the base we want to convert to (e.g., loga(b)). The result gives us the value of the logarithm with the desired base (logb(x)).
It’s important to note that the change of base formula can be used with any base, as long as the logarithms with those bases can be evaluated. Popular choices for the new base include logarithms with a base of 10 (log) and logarithms with a base of the natural logarithm (ln).
Solving Logarithmic Equations
Logarithmic equations involve the use of logarithms to solve for the unknown variable. These equations are typically solved by using the properties of logarithms and basic algebraic techniques.
When solving logarithmic equations, it is important to remember the properties of logarithms, such as the product property, power property, and quotient property. These properties allow us to simplify and manipulate logarithmic expressions, making it easier to solve for the unknown variable.
One common approach to solving logarithmic equations is to isolate the logarithmic term on one side of the equation and then evaluate both sides using the properties of logarithms. This process often involves using exponential functions to convert logarithmic equations into exponential form.
It is also important to check for extraneous solutions when solving logarithmic equations. Since logarithmic equations involve domain restrictions, some solutions may not be valid. To check for extraneous solutions, substitute the obtained solution back into the original logarithmic equation and verify if it satisfies the given domain restrictions.
In summary, solving logarithmic equations requires a good understanding of the properties of logarithms and basic algebraic techniques. By isolating the logarithmic term, applying the properties of logarithms, and checking for extraneous solutions, we can find the solutions to logarithmic equations.
Using Properties of Logarithms to Solve Equations
Properties of logarithms play a crucial role in solving equations that involve logarithmic functions. These properties provide us with various tools and techniques to simplify and manipulate logarithmic expressions, ultimately leading to finding solutions to equations.
One fundamental property is the power property, which states that for any positive base b and any real numbers m and n, the logarithm of b raised to the power of m is equal to m multiplied by the logarithm of b. This property allows us to move exponents out of logarithms, making equations easier to work with.
Another important property is the product property, which states that the logarithm of a product of two numbers is equal to the sum of the logarithms of the individual numbers. This property enables us to break down complex logarithmic expressions into simpler ones and solve equations step by step.
Additionally, the quotient property of logarithms states that the logarithm of a quotient of two numbers is equal to the difference of the logarithms of the individual numbers. This property helps in simplifying equations that involve ratios or fractions.
By utilizing these properties, we can transform equations containing logarithms into equations that are easier to solve algebraically. We can apply the properties to manipulate the equation until we isolate the logarithm term on one side and the constant term on the other side. Once isolated, we can then apply exponentiation to both sides of the equation to solve for the variable.
Properties of logarithms are essential tools in solving equations involving logarithmic functions. They allow us to simplify expressions, break down complex equations, and find solutions through algebraic manipulation. Understanding and utilizing these properties empowers us to confidently solve a wide range of logarithmic equations.
Examples of Logarithmic Equations
Logarithmic equations involve the use of logarithms to solve for unknown variables. They are commonly used in various fields such as mathematics, physics, and finance. In this section, we will explore some examples of logarithmic equations and how to solve them.
Example 1:
Let’s consider the equation:
log(x) = 2
To solve this equation, we need to rewrite it in exponential form. Since the base of the logarithm is not specified, we can assume it to be 10:
x = 10^2
Therefore, x = 100.
Example 2:
Now, let’s take a look at a logarithmic equation involving a different base:
log base 2 (x+3) = 4
To eliminate the logarithm, we can rewrite the equation as:
x+3 = 2^4
x+3 = 16
Now, we can solve for x by subtracting 3 from both sides:
x = 16 – 3
Therefore, x = 13.
Example 3:
Lastly, let’s examine a logarithmic equation with variables on both sides:
log base 5 (x+2) + log base 5 (2x+3) = log base 5 (5)
To simplify this equation, we can combine the logarithms on the left side using the product rule:
log base 5 [(x+2)(2x+3)] = log base 5 (5)
Since the logarithms are equal, the expressions inside the logarithms must also be equal:
(x+2)(2x+3) = 5
Expanding and rearranging the equation, we get:
2x^2 + 7x + 6 = 5
Now, we can solve this quadratic equation to find the value of x.
These examples provide a glimpse into the various types of logarithmic equations and how to approach them. Remember, it’s important to understand the properties of logarithms and how to manipulate them when solving such equations.
Logarithmic Expressions and Simplification
Logarithms are mathematical functions that can be used to simplify and solve complex exponential equations. A logarithmic expression represents the exponent to which a base number must be raised to obtain a given value. Logarithms are denoted by the symbol “log”. Simplifying logarithmic expressions involves applying the properties of logarithms.
The Product Rule: This rule states that the logarithm of a product is equal to the sum of the logarithms of the individual factors. In other words, log(ab) = log(a) + log(b). By using this rule, we can break down a complex product into simpler logarithmic expressions.
The Quotient Rule: The quotient rule states that the logarithm of a quotient is equal to the difference of the logarithms of the numerator and denominator. In other words, log(a/b) = log(a) – log(b). This rule allows us to simplify complex division problems by converting them into subtraction problems.
The Power Rule: The power rule states that the logarithm of a number raised to a power is equal to the product of the power times the logarithm of the base. In other words, log(a^n) = n * log(a). This rule allows us to simplify exponential expressions by distributing the exponent across the logarithmic expression.
In addition to these rules, there are other properties of logarithms, such as the change of base formula and the logarithm of 1, that can be used to simplify and solve logarithmic equations. Understanding and applying these properties can greatly simplify complex logarithmic expressions and make solving equations involving logarithms much easier.