The Pythagorean theorem is one of the fundamental concepts in mathematics, widely used to solve various types of problems involving right triangles. Understanding and applying this theorem is essential for students studying geometry and trigonometry. In this article, we will provide a collection of Pythagorean theorem problems along with their solutions in a convenient PDF format.
These problems will cover a range of scenarios where the Pythagorean theorem can be applied. Whether you are calculating the length of one side of a right triangle given the other two sides, or determining whether a triangle is a right triangle based on its side lengths, these problems will help you practice and develop your problem-solving skills.
The PDF document will contain both the problem statements and the step-by-step solutions, allowing you to learn the concepts and techniques involved in solving each problem. Additionally, it will provide you with an opportunity to test your understanding by attempting the problems on your own before referring to the answers.
By practicing with these Pythagorean theorem problems, you will gain proficiency in applying the theorem to various real-life situations, such as finding the distance between two points on a coordinate plane or calculating the length of a ladder needed to reach a certain height. This will not only enhance your mathematical skills but also improve your problem-solving abilities.
Pythagorean Theorem Problems with Answers PDF
Pythagorean theorem is one of the fundamental concepts in geometry that allows us to find the length of one side of a right triangle when we know the lengths of the other two sides. It states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Practicing Pythagorean theorem problems is essential for students to understand and apply this concept effectively. To make the learning process easier, many resources provide Pythagorean theorem problems with answers in PDF format. These PDFs usually include a variety of problems ranging from basic to advanced levels, allowing students to gradually develop their problem-solving skills.
In a typical Pythagorean theorem problems PDF, you will find questions that require you to find the missing side length in a right triangle, determine if a triangle is a right triangle based on the given side lengths, or solve real-life problems using the Pythagorean theorem. The answers to these problems are also provided in the PDF, allowing students to check their work and gain confidence in their understanding of the concept.
When practicing Pythagorean theorem problems, it is important to remember the formula: a^2 + b^2 = c^2, where “a” and “b” are the lengths of the two legs of the right triangle, and “c” is the length of the hypotenuse. It is also helpful to familiarize yourself with the Pythagorean triplets, which are sets of three positive integers that satisfy the Pythagorean theorem equation.
By practicing Pythagorean theorem problems with answers in PDF format, students can improve their problem-solving skills, become more confident in their abilities, and develop a solid understanding of the Pythagorean theorem. These resources can be used by students, teachers, and parents to supplement classroom learning and provide additional practice opportunities.
Problem 1: Finding the Length of the Hypotenuse

In geometry, the Pythagorean theorem is a fundamental concept that relates the lengths of the sides of a right triangle. One common problem associated with this theorem involves finding the length of the hypotenuse, given the lengths of the other two sides.
To solve these types of problems, we can use the formula a² + b² = c², where “a” and “b” represent the lengths of the two sides of the triangle, and “c” represents the length of the hypotenuse. By substituting the known values into the equation, we can determine the length of the hypotenuse.
For example, consider a right triangle with one side measuring 3 units and another side measuring 4 units. To find the length of the hypotenuse, we can apply the Pythagorean theorem. Substituting the values into the equation, we have 3² + 4² = c². Simplifying, we get 9 + 16 = c². Adding the values, we find that c² = 25. Taking the square root of both sides, we get c = √25 = 5 units.
In this case, the length of the hypotenuse is 5 units. By solving similar problems, we can find the length of the hypotenuse for any right triangle, given the lengths of the other sides.
Problem 2: Finding the Length of a Leg
In this problem, we are given a right triangle and we need to find the length of one of its legs.
Let’s say we have a right triangle with one leg measuring 3 units and the hypotenuse measuring 5 units. We can use the Pythagorean theorem to find the length of the other leg.
Pythagorean theorem: In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
Using the Pythagorean theorem, we can set up the equation:
a2 + b2 = c2
where a and b are the lengths of the legs, and c is the length of the hypotenuse.
In our example, we can plug in the values to get:
32 + b2 = 52
Simplifying, we have:
9 + b2 = 25
Subtracting 9 from both sides, we get:
b2 = 16
Taking the square root of both sides, we find:
b = 4
Therefore, the length of the other leg is 4 units.
Problem 3: Solving for an Unknown Side in a Right Triangle
A right triangle is a triangle that has one angle measuring 90 degrees. One of the most important tools for solving problems involving right triangles is the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
In Problem 3, we are given a right triangle with one side measuring 8 units and another side measuring 6 units. To solve for the unknown side, we can use the Pythagorean theorem. Let’s denote the length of the unknown side as x.
Solution:
- Apply the Pythagorean theorem: x2 = 82 + 62
- Simplify the equation: x2 = 64 + 36 = 100
- Take the square root of both sides to solve for x: x = √100
- Simplify the square root: x = 10
Therefore, the length of the unknown side in this right triangle is 10 units.
Problem 4: Applying the Pythagorean Theorem in Real-life Situations
The Pythagorean theorem, a fundamental concept in geometry, has numerous applications in real-life situations. In problem 4, we will explore how the Pythagorean theorem can be used to solve a real-life problem.
Problem: A ladder is leaning against a wall. The base of the ladder is 5 feet away from the wall and the ladder is 13 feet long. How far up the wall does the ladder reach?
To solve this problem, we can use the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. In this case, the wall, the base of the ladder, and the ladder itself form a right triangle.
Let’s label the length the ladder reaches up the wall as “x”. According to the Pythagorean theorem, we have the equation:
- x^2 = 13^2 – 5^2
- x^2 = 169 – 25
- x^2 = 144
- x = sqrt(144)
- x = 12 feet
Therefore, the ladder reaches up the wall a distance of 12 feet.
This problem demonstrates how the Pythagorean theorem can be applied in real-life situations, such as determining the height a ladder reaches when leaning against a wall. By understanding and utilizing this fundamental geometric concept, we can solve a variety of practical problems.
Problem 5: Using the Pythagorean Theorem to Determine Distance

In this problem, we will be using the Pythagorean Theorem to determine the distance between two points in a two-dimensional coordinate plane.
Let’s say we have two points labeled A and B. Point A has coordinates (x1, y1) and point B has coordinates (x2, y2). We want to find the distance between these two points.
To solve this problem, we can use the formula derived from the Pythagorean Theorem:
Distance = √((x2 – x1)^2 + (y2 – y1)^2)
Let’s walk through an example to illustrate how to use this formula.
Example:
Given point A with coordinates (3, 4) and point B with coordinates (7, 9), we want to find the distance between these two points.
We can plug the values into the distance formula as follows:
Distance = √((7 – 3)^2 + (9 – 4)^2)
Simplifying this expression, we get:
Distance = √(4^2 + 5^2)
Further simplifying, we have:
Distance = √(16 + 25)
Distance = √41
Therefore, the distance between point A and point B is approximately √41 units.
Problem 6: Solving for an Unknown Angle in a Right Triangle
In this problem, we are given a right triangle and we need to find the value of an unknown angle. To solve this problem, we can use the Pythagorean theorem and trigonometric ratios.
Let’s consider a right triangle with two known sides and one unknown angle. We can label the sides as the hypotenuse, adjacent side, and opposite side, using the conventions of trigonometry. The hypotenuse is the side opposite the right angle, the adjacent side is the side adjacent to the unknown angle, and the opposite side is the side opposite the unknown angle.
Step 1: Identify the known sides and the unknown angle in the right triangle.
- The hypotenuse is given as a length of 10 units.
- The adjacent side is given as a length of 6 units.
- We need to find the measure of the unknown angle.
Step 2: Apply the Pythagorean theorem to find the length of the opposite side.
The Pythagorean theorem states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. Using this theorem, we can calculate the length of the opposite side as:
| a^2 = c^2 – b^2 | (where a is the opposite side, c is the hypotenuse, and b is the adjacent side) |
| a^2 = 10^2 – 6^2 | |
| a^2 = 100 – 36 | |
| a^2 = 64 | |
| a = √64 | |
| a = 8 |
Step 3: Use trigonometric ratios to find the measure of the unknown angle.
Trigonometric ratios are relationships between the sides of a right triangle and its angles. We can use these ratios to find the measure of the unknown angle. In this case, we can use the tangent ratio:
| tan(θ) = a/b | (where θ is the unknown angle, a is the opposite side, and b is the adjacent side) |
| tan(θ) = 8/6 | |
| tan(θ) = 4/3 | |
| θ = tan-1(4/3) | |
| θ ≈ 53.13° |
Therefore, the measure of the unknown angle in the right triangle is approximately 53.13 degrees.
Problem 7: Using the Pythagorean Theorem in Three Dimensions
In three-dimensional space, the Pythagorean Theorem can still be used to find the length of the hypotenuse in a right triangle. However, instead of considering just two dimensions, we have to account for the third dimension as well.
For example, let’s say we have a right triangle in three dimensions with one side measuring 3 units in the x-axis, another side measuring 4 units in the y-axis, and the hypotenuse measuring h units in the z-axis. We can apply the Pythagorean Theorem to find the value of h.
The Pythagorean Theorem in three dimensions:
h² = a² + b² + c², where a, b, and c represent the lengths of the three sides of the right triangle.
Applying this formula to our example, we can calculate:
- a² = 3² = 9
- b² = 4² = 16
Let’s substitute these values into the formula:
h² = a² + b² + c²
h² = 9 + 16 + c²
Now, if we know the value of c, we can solve for h. However, if c is unknown, we can still simplify the equation using the Pythagorean Theorem in two dimensions:
c² = a² + b²
c² = 9 + 16
c² = 25
Therefore, we can conclude that c is equal to 5 units. Now we can substitute this value back into the original equation:
h² = 9 + 16 + 5²
h² = 9 + 16 + 25
h² = 50
Finally, taking the square root of both sides, we find that:
h = √50
So, the length of the hypotenuse in this three-dimensional right triangle is √50 units.
Problem 8: Exploring Pythagorean Triples
In this problem, we will explore the concept of Pythagorean triples, which are sets of three positive integers (a, b, and c) that satisfy the Pythagorean theorem: a^2 + b^2 = c^2.
A Pythagorean triple is a combination of three numbers that can form the sides of a right triangle. These triples have been studied for thousands of years and have many interesting properties.
Let’s begin by finding some Pythagorean triples. One commonly known triple is (3, 4, 5). If we substitute these values into the Pythagorean theorem, we can see that 3^2 + 4^2 = 9 + 16 = 25, which is equal to 5^2. Therefore, this triple satisfies the Pythagorean theorem.
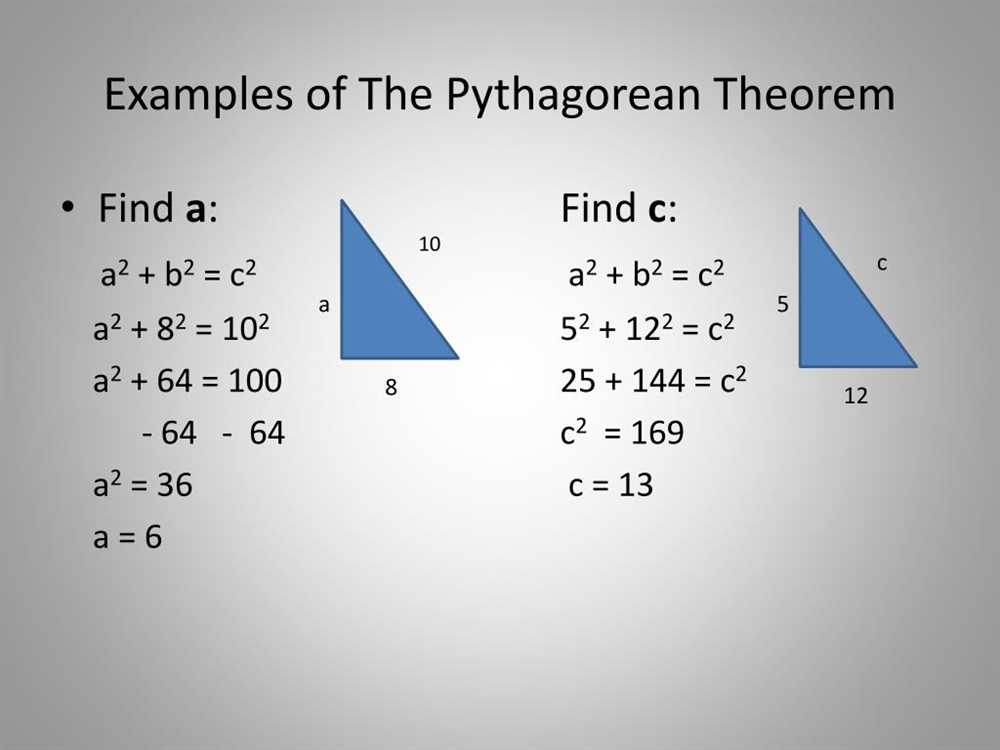
Another well-known triple is (5, 12, 13). We can verify this triple by substituting the values into the Pythagorean theorem: 5^2 + 12^2 = 25 + 144 = 169, which is equal to 13^2.
To find more Pythagorean triples, we can use a common method known as Euclid’s formula. According to this formula, we can generate Pythagorean triples by choosing any two positive integers m and n (where m > n), and then calculating:
- a = m^2 – n^2
- b = 2mn
- c = m^2 + n^2
By using different values for m and n, we can generate an infinite number of Pythagorean triples. For example, if we choose m = 2 and n = 1, we get the triple (3, 4, 5) mentioned earlier. If we choose m = 3 and n = 2, we get the triple (5, 12, 13) mentioned earlier.
In conclusion, Pythagorean triples are sets of three positive integers that satisfy the Pythagorean theorem. They have been studied for centuries and can be generated using Euclid’s formula. Exploring these triples can lead to discovering interesting patterns and relationships within mathematics.