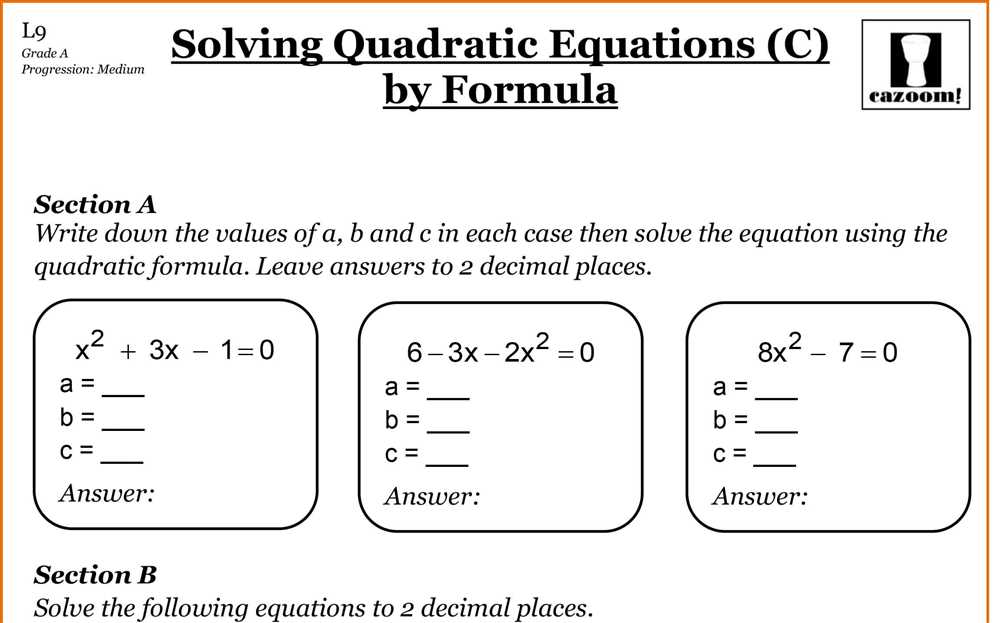
Quadratic equations can sometimes be challenging for students to solve or visualize. However, a coloring activity can make the process more engaging and enjoyable for learners. By providing answers to a quadratic equations coloring activity, students can check their work and gain a better understanding of the concepts.
In a quadratic equations coloring activity, students are typically given a grid with various quadratic equations written in different cells. Each equation corresponds to a specific color. Students solve each equation and color the corresponding cell with the designated color. By the end of the activity, a colorful picture or pattern is revealed.
By providing the answers to this activity, students can assess their accuracy and progress. They can compare their colored grid with the solution provided and make any necessary corrections. Furthermore, having the answers readily available allows students to learn from their mistakes and deepen their understanding of quadratic equations.
The availability of answers also allows teachers or parents to guide students in the learning process. They can help clarify any misconceptions, provide explanations for incorrect answers, and offer additional practice if needed. This collaborative approach fosters a supportive learning environment and helps students build confidence in their problem-solving skills.
What are quadratic equations?
A quadratic equation is a second-degree polynomial equation in a single variable, usually written in the form ax^2 + bx + c = 0, where a, b, and c are constants.
In simpler terms, a quadratic equation is an equation that can be written in the form of a quadratic function. It involves the variable raised to the power of two, hence the term “second-degree.” Quadratic equations are common in algebra and have various applications in mathematics, physics, engineering, and other fields.
Quadratic equations often have two solutions or roots, which can be real or complex numbers. These solutions can be found using various methods, such as factoring, completing the square, or by using the quadratic formula.
Quadratic equations are used to model a wide range of real-life situations, including projectile motion, optimization problems, and finding the dimensions of geometric shapes. They play a significant role in solving problems involving parabolas, which are U-shaped curves that can be described by quadratic functions.
In conclusion, quadratic equations are fundamental mathematical equations that involve a second-degree polynomial function. They have various applications and are essential in solving problems related to parabolas and other real-life situations. Understanding and solving quadratic equations is an important skill in mathematics and many other fields.
Definition of quadratic equations
A quadratic equation is a polynomial equation of the second degree. It involves a variable raised to the power of two, and may also include other terms such as a constant and a linear term. The general form of a quadratic equation is:
ax^2 + bx + c = 0
Here, a, b, and c are coefficients, and x is the unknown variable. The coefficient a must be nonzero, otherwise the equation would become linear.
The solutions to a quadratic equation can be found using various methods, such as factoring, completing the square, or using the quadratic formula:
x = (-b ± √(b^2 – 4ac)) / 2a
Quadratic equations have a variety of applications in mathematics, physics, engineering, and other fields. They are used to model various real-life situations, such as the motion of objects under the influence of gravity, the shape of projectiles, and the behavior of electrical circuits.
- Key phrases: quadratic equation, polynomial equation, variable, coefficient, equation, solutions, factoring, completing the square, quadratic formula, applications
Examples of Quadratic Equations
A quadratic equation is a polynomial equation of the second degree, meaning it can be written in the form: ax^2 + bx + c = 0 where a, b, and c are constants. Quadratic equations have various real-life applications and are commonly used in fields such as physics, engineering, and finance.
Here are a few examples of quadratic equations:
- x^2 + 2x – 3 = 0: This quadratic equation can be factored as (x – 1)(x + 3) = 0, resulting in two solutions: x = 1 and x = -3.
- 3x^2 – 7x + 2 = 0: This quadratic equation cannot be easily factored, so it can be solved using the quadratic formula: x = (-b ± sqrt(b^2 – 4ac)) / 2a. Plugging in the values, we get x = (7 ± sqrt(49 – 24)) / 6, which simplifies to x = (7 ± sqrt(25)) / 6. The solutions are x = 1 and x = 2/3.
- 2x^2 – 4x + 2 = 0: This quadratic equation can be factored as 2(x – 1)^2 = 0, resulting in a double root of x = 1.
Quadratic equations can describe various scenarios in real life, such as projectile motion, optimization problems, and the behavior of financial investments. Understanding how to solve quadratic equations is essential for efficiently solving problems in these fields and more.
The Importance of Solving Quadratic Equations
Quadratic equations play a crucial role in various fields of study, including mathematics, physics, engineering, and economics. These equations are of significant importance as they help us solve real-world problems and model various phenomena.
1. Mathematical Significance: Solving quadratic equations is a fundamental skill in mathematics. It allows us to find the solutions, or roots, of equations that involve quadratic terms. These equations often arise when studying geometric shapes, motion, and optimization problems. Understanding quadratic equations helps build a strong foundation in algebra and serves as a stepping stone to more advanced mathematical concepts.
2. Physical Applications: In physics, quadratic equations are used to describe and predict the behavior of objects in motion. They help analyze projectile motion, gravitational forces, and the behavior of fluids. By solving these equations, we can determine the time, distance, and velocity of an object at different points in its trajectory.
3. Engineering and Technology: Quadratic equations find extensive application in engineering and technology fields. They are used to model and solve problems related to electrical circuits, control systems, structural analysis, and optimization. Engineers rely on quadratic equations to analyze and design various structures, such as bridges, buildings, and aircraft wings.
4. Economic Analysis: Economic theories often involve quadratic equations in the form of supply and demand functions, production functions, and revenue maximization. By solving these equations, economists can determine the optimal level of production, equilibrium price, and profit-maximizing strategies. Quadratic equations help economists analyze market trends, price elasticity, and consumer behavior.
In conclusion, solving quadratic equations is of utmost importance in various fields of study. It helps us understand and model real-world phenomena, solve complex problems, and make informed decisions. Whether in mathematics, physics, engineering, or economics, the ability to solve quadratic equations is a valuable skill that opens doors to further knowledge and understanding.
Real-life applications of quadratic equations
Quadratic equations, which involve variables to the power of two, play a crucial role in various real-life applications. From analyzing the motion of objects to predicting the trajectory of projectiles, quadratic equations provide valuable insights into the physical world.
One notable application of quadratic equations is in the field of physics. For instance, when an object is thrown vertically into the air, its vertical position can be described by a quadratic equation. This equation allows us to determine important variables such as the maximum height the object reaches, the time it takes to reach that height, and the time it takes for the object to hit the ground. Understanding these variables can help engineers design safer structures or assist athletes in optimizing their performance.
Furthermore, quadratic equations are used in financial planning and economics. For instance, in financial forecasting, quadratic equations can be used to model and predict how the value of an investment will fluctuate over time. By analyzing the vertex of the parabolic curve described by the equation, investors can make informed decisions about when to buy or sell stocks, bonds, or other financial instruments.
In conclusion, quadratic equations have a wide range of real-life applications. They allow us to analyze and predict various phenomena, from physical motion to financial trends. By understanding and solving quadratic equations, we can gain valuable insights and make informed decisions in a variety of fields.
Benefits of Understanding Quadratic Equations
Quadratic equations are an important concept in mathematics and have several benefits in various fields. Understanding quadratic equations provides individuals with problem-solving skills and critical thinking abilities that can be applied in real-world situations.
One of the main benefits of understanding quadratic equations is the ability to solve complex mathematical problems. Quadratic equations involve variables raised to the power of two, and solving them requires applying algebraic manipulations and formulas. This process enhances logical reasoning and analytical thinking skills, which are highly valued in fields such as engineering, physics, and computer science.
Furthermore, knowing how to solve quadratic equations allows individuals to model and analyze real-life scenarios. Many natural phenomena and physical laws can be described by quadratic equations, such as projectile motion, the behavior of objects under the influence of gravity, and the growth of populations. By understanding quadratic equations, one can predict and understand the behavior of these systems, which has applications in fields like physics, economics, and biology.
In addition, proficiency in quadratic equations is crucial for success in standardized tests and academic pursuits. Tests like the SAT and ACT often include quadratic equations in their math sections, and a strong understanding of these equations can significantly improve scores. Moreover, quadratic equations are a fundamental topic in high school algebra courses, and a thorough comprehension of this concept sets the foundation for advanced mathematics and higher education in STEM fields.
In conclusion, understanding quadratic equations provides individuals with problem-solving skills, enhances logical reasoning and analytical thinking abilities, allows for modeling and analyzing real-life scenarios, and contributes to success in standardized tests and academic pursuits. By grasping the concepts and applications of quadratic equations, individuals can gain valuable skills and knowledge that are applicable in various fields and academic pursuits.
Interactive Coloring Activity for Quadratic Equations
Quadratic equations can often be challenging for students to understand and solve. However, interactive activities can make the learning process more engaging and enjoyable. One such activity is the quadratic equations coloring activity, which combines math and art to create an interactive learning experience.
In this activity, students are given a worksheet with a grid of squares. Each square contains a quadratic equation to solve. Instead of simply solving the equation and writing down the answer, students also color the square based on the solution. This adds a creative element to the activity and allows students to visualize the solutions in a more tangible way.
To solve the equations, students can use various methods such as factoring, completing the square, or using the quadratic formula. After finding the solutions, they can use a coding system to assign different colors to different types of solutions. For example, they can color squares with real solutions in one color and squares with complex solutions in another color. This coding system helps students visually identify the types of solutions and reinforce their understanding of quadratic equations.
One of the benefits of this interactive coloring activity is that it can be easily adapted for different levels of difficulty. Teachers can provide worksheets with equations that require basic factoring skills for younger students, while more advanced students can be challenged with equations that require using the quadratic formula. Additionally, teachers can customize the coding system and color choices to match the needs of their students or to focus on specific learning objectives.
Overall, the quadratic equations coloring activity is an effective and engaging way to reinforce and apply the concepts of quadratic equations. It combines math with art, allowing students to not only solve equations but also express their creativity. By actively participating in this activity, students can develop a deeper understanding of quadratic equations and improve their problem-solving skills.
Overview of the Activity
Quadratic equations coloring activity is a fun and engaging way for students to practice solving quadratic equations. This activity involves solving a series of quadratic equations and coloring a coloring sheet based on the solutions obtained. The coloring sheet has different sections, each corresponding to a specific type of solution.
This activity is designed to reinforce the concepts of solving quadratic equations and identifying different types of solutions, including real solutions, complex solutions, and imaginary solutions. By actively engaging in the coloring activity, students can enhance their understanding of these concepts and improve their problem-solving skills.
During the activity, students are provided with a set of quadratic equations to solve. They need to apply their knowledge of quadratic formula or factoring to find the solutions. Once they have obtained the solutions, they can refer to the coloring sheet to determine which section to color. Each section on the coloring sheet is labeled with the type of solution it represents.
This activity encourages students to think critically and make connections between mathematical concepts and visual representations. By identifying the different types of solutions and coloring the corresponding sections, students can develop a deeper understanding of quadratic equations and their solutions.
This activity can be used as a formative assessment tool to gauge students’ understanding of quadratic equations and provide them with an opportunity to practice their problem-solving skills in a creative and enjoyable way. It can also be used as a group activity or a homework assignment to further reinforce the concepts taught in the classroom.