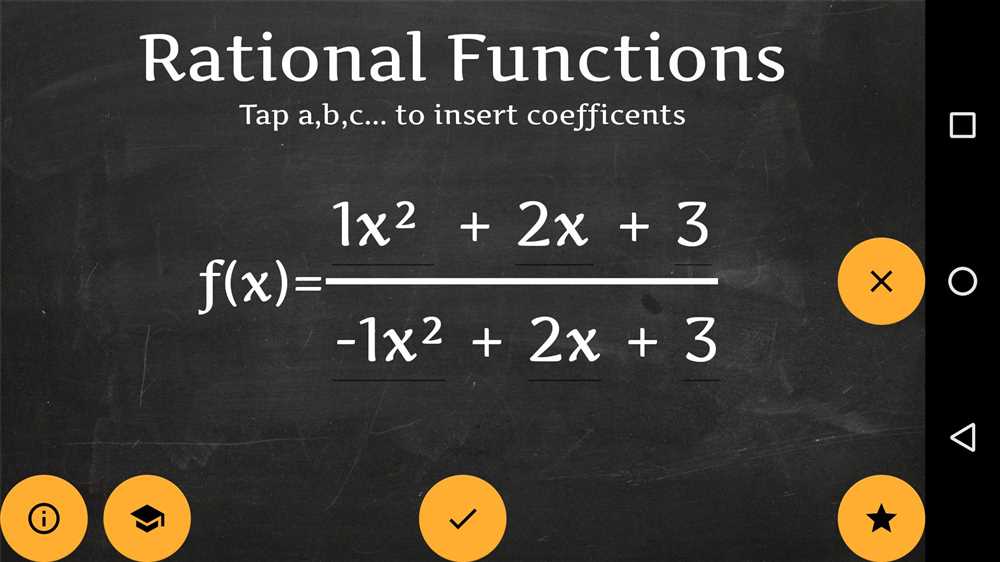
A rational function is a function that can be expressed as the quotient or fraction of two polynomials. These functions are commonly used to model real-world situations, such as the population growth of a species or the depreciation of an asset over time. Testing your understanding of rational functions is essential for a solid grasp of algebraic concepts.
The rational functions test is designed to assess your knowledge and skills in working with rational functions and their properties. It covers topics such as finding asymptotes, domain and range, graphing, simplifying, and solving equations involving rational functions. By successfully completing this test, you can demonstrate your proficiency in manipulating and analyzing rational functions.
Preparing for the rational functions test involves practicing a variety of problem-solving techniques and familiarizing yourself with the key concepts and formulas related to rational functions. It is important to review the properties of rational functions, including the rules for multiplying, dividing, and adding fractions. Additionally, understanding the behavior of rational functions at vertical asymptotes and the impact of holes in the graph is crucial for success on the test.
Rational Functions Test

Rational functions are mathematical functions that can be expressed as the ratio of two polynomials. They are often used to model real-world situations and solve problems involving rates, proportions, and ratios. Understanding rational functions is essential in many fields, including mathematics, physics, and engineering.
A rational functions test is designed to assess a student’s understanding of these types of functions. It may include questions that require the student to identify the key features of a rational function, such as the domain, range, vertical asymptotes, and horizontal asymptotes. The test may also include questions that involve finding the x-intercepts, y-intercepts, and holes of a rational function.
To prepare for a rational functions test, students should review the basic concepts and properties of rational functions. This includes understanding how to simplify and factorize rational expressions, how to find the domain and range of a rational function, and how to graph a rational function. Additionally, students should practice solving various types of problems involving rational functions, such as solving equations involving rational expressions and solving word problems that can be modeled using rational functions.
During the test, it is important for students to carefully read each question and show their work. They should also pay attention to any restrictions on the domain and any properties unique to the given rational function. In addition, students should double-check their answers and make sure they have provided clear and accurate explanations.
Overall, a rational functions test is an opportunity for students to demonstrate their knowledge and problem-solving skills in the context of rational functions. By adequately preparing for the test and practicing various types of problems, students can feel confident when tackling questions related to rational functions.
Definition and Characteristics of Rational Functions
A rational function is a mathematical function that can be expressed as the quotient of two polynomial functions, where the denominator is not equal to zero. It can be written in the form f(x) = p(x) / q(x), where p(x) and q(x) are polynomial functions and q(x) is not equal to zero. Rational functions can have different degrees for their numerator and denominator.
One characteristic of rational functions is that they may have vertical asymptotes. These are vertical lines that the graph of the function approaches but never intersects. Vertical asymptotes occur when the denominator of the rational function is equal to zero but the numerator is not. The location of the vertical asymptotes can be found by finding the values of x that make the denominator zero.
Another characteristic of rational functions is that they may have horizontal asymptotes. These are horizontal lines that the graph of the function gets closer and closer to as x approaches positive or negative infinity. Horizontal asymptotes can be determined by comparing the degrees of the numerator and denominator. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is at y = 0. If the degree of the numerator is equal to the degree of the denominator, the horizontal asymptote is the ratio of the leading coefficients. If the degree of the numerator is greater than the degree of the denominator, there is no horizontal asymptote.
Rational functions can also have oblique asymptotes. These are slanted lines that the graph of the function gets closer and closer to as x approaches positive or negative infinity. Oblique asymptotes occur when the degree of the numerator is exactly one more than the degree of the denominator. The oblique asymptote can be found by performing polynomial long division on the rational function.
In conclusion, rational functions are mathematical functions that are expressed as the quotient of two polynomial functions. They can have vertical, horizontal, or oblique asymptotes, depending on the properties of their numerator and denominator. Understanding these characteristics is essential in analyzing and graphing rational functions.
Graphing Rational Functions
Rational functions are functions that can be expressed as the ratio of two polynomials. They are often represented by the equation f(x) = p(x)/q(x), where p(x) and q(x) are polynomials. Graphing rational functions can provide valuable insights into their behavior and help us understand their properties.
When graphing rational functions, it is important to consider their key features, such as asymptotes, intercepts, and points of discontinuity. These features can be determined by analyzing the numerator and denominator of the rational function. Vertical asymptotes occur at the values of x for which the denominator q(x) equals zero. Horizontal asymptotes, on the other hand, can be found by evaluating the limits as x approaches positive or negative infinity. Intercepts can be calculated by setting either the numerator or denominator equal to zero. Points of discontinuity can occur when the rational function has a common factor in the numerator and denominator that cancels out.
Once we have identified the key features, we can plot the graph of the rational function. This is often done by plotting points using a table of values and then connecting them with smooth curves. The shape of the graph can vary depending on the degree of the numerator and denominator polynomials. It can have vertical or horizontal asymptotes, holes, or slant asymptotes. It is also important to consider the domain and range of the function to ensure that the graph accurately represents the behavior of the rational function.
In summary, graphing rational functions involves identifying key features such as asymptotes, intercepts, and points of discontinuity, and using this information to plot the graph. Understanding the behavior of rational functions can provide valuable insights into their properties and help us analyze and solve problems involving these functions.
Finding Vertical and Horizontal Asymptotes
In the study of rational functions, one important concept to understand is the concept of asymptotes. Asymptotes are lines that a graph approaches but never crosses. In particular, we are interested in finding the vertical and horizontal asymptotes of a rational function.
To find the vertical asymptotes of a rational function, we look for values of the variable that make the denominator of the function equal to zero. These values represent points where the function is undefined. If we find any such values, they will correspond to vertical lines that the graph approaches as the variable approaches these values. For example, if the denominator has a factor of (x – a), where ‘a’ is a constant, then there will be a vertical asymptote at x = a.
On the other hand, to find the horizontal asymptote of a rational function, we need to analyze the degrees of the numerator and denominator. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote will be the x-axis (y = 0). If the degree of the numerator is greater than the degree of the denominator, there will be no horizontal asymptote. However, if the degrees are equal, we need to compare the leading coefficients of both polynomial expressions. If the leading coefficients are equal, the horizontal asymptote will be the line y = the fraction of the leading coefficients. Otherwise, there will be no horizontal asymptote.
By understanding and identifying the vertical and horizontal asymptotes of a rational function, we can gain valuable insights into the behavior of the function and its graph. These asymptotes help us understand the limits of the function and provide valuable information for graphing and analyzing rational functions.
Simplifying Rational Expressions
Rational expressions, also known as rational functions, are expressions that are defined as the ratio of two polynomial functions. These expressions often contain variables and can be simplified by factoring out common factors and canceling out terms.
One common method for simplifying rational expressions is to factor both the numerator and the denominator and then cancel out common factors. For example, if we have the expression (2x+4)/(x+2), we can factor both the numerator and denominator to get 2(x+2)/(x+2). The (x+2) terms can then be canceled out, leaving us with the simplified expression 2.
- Factor the numerator and denominator
- Cancel out common factors
- Simplify the expression
It’s important to note that when simplifying rational expressions, we need to be careful about any restrictions on the variable. If there are any values that would make the denominator equal to zero, then those values should be excluded from the domain of the simplified expression.
Overall, simplifying rational expressions involves factoring and canceling out common factors to simplify the expression and make it easier to work with. This process can be particularly useful in solving equations or finding limits of rational functions.
Operations with Rational Functions
Rational functions are mathematical expressions that represent the division of two polynomial functions. These functions have a numerator and a denominator, both of which can be polynomials. Operations with rational functions involve adding, subtracting, multiplying, and dividing these expressions.
When adding or subtracting rational functions, the first step is to find a common denominator for the two fractions. This involves factoring the denominators, identifying the common factors, and multiplying by the necessary factors to make the denominators the same. Once the denominators are the same, the numerators can be added or subtracted accordingly.
Multiplying rational functions is a relatively straightforward process. Multiply the numerators together to get the new numerator, and multiply the denominators together to get the new denominator. Simplify the resulting fraction if possible by factoring out any common factors.
Dividing rational functions requires a few additional steps. To divide one rational function by another, you must first multiply the numerator and the denominator of the first fraction by the reciprocal of the second fraction. This is done by flipping the second fraction and then performing the multiplication. After multiplying, simplify the resulting fraction if possible.
Overall, operations with rational functions involve manipulating the numerators and denominators of these expressions to perform addition, subtraction, multiplication, and division. It is important to simplify the resulting fractions whenever possible to achieve the most simplified form of the function.
Solving Equations Involving Rational Functions
When solving equations involving rational functions, there are a few key steps to follow. First, you’ll want to identify any restrictions on the variables that might make the equation undefined. These restrictions can be found by finding the values that would make the denominator of the rational function equal to zero. Once you have identified these restrictions, you can set up the equation and simplify it.
Next, you’ll want to multiply through by the least common denominator (LCD) to eliminate any fractions in the equation. This step allows you to work with a polynomial equation, which is typically easier to solve. After multiplying through by the LCD, you can simplify the equation further and collect like terms.
At this point, you should have a polynomial equation that you can solve using various techniques such as factoring, the quadratic formula, or completing the square. Once you have found the values of the variable that satisfy the equation, you’ll need to check if they are also valid solutions by making sure they do not violate any of the restrictions on the variables.
In summary, solving equations involving rational functions involves identifying restrictions, multiplying through by the LCD, simplifying the equation, solving a polynomial equation, and checking the solutions for validity. By following these steps, you’ll be able to solve equations involving rational functions accurately and efficiently.
Common Mistakes to Avoid in Rational Functions
When working with rational functions, it’s important to be aware of common mistakes that students often make. By understanding and avoiding these mistakes, you can improve your understanding and performance in this topic. Here are some common mistakes to be aware of:
1. Dividing instead of factoring:
One common mistake is dividing the numerator and denominator in a rational function instead of factoring. When simplifying a rational function, it’s crucial to first factor both the numerator and denominator and then simplify. Dividing instead of factoring can lead to incorrect results and a misunderstanding of the concept.
2. Forgetting to exclude values:
Another common mistake is forgetting to exclude values that result in an undefined expression. Rational functions can have restricted values where the denominator is zero, leading to an undefined expression. It’s important to identify these excluded values and indicate them in the final answer or solution.
3. Misinterpreting horizontal and vertical asymptotes:
Understanding the concept of asymptotes is crucial in rational functions. One common mistake is misinterpreting the direction and location of horizontal and vertical asymptotes. Horizontal asymptotes represent the behavior of the function as x approaches positive or negative infinity, while vertical asymptotes represent the behavior as x approaches a specific value. It’s vital to correctly identify and interpret these asymptotes to accurately describe the behavior of the function.
By being aware of these common mistakes, you can avoid them and improve your understanding and proficiency in rational functions. Practice identifying and correcting these errors to strengthen your skills in this topic.