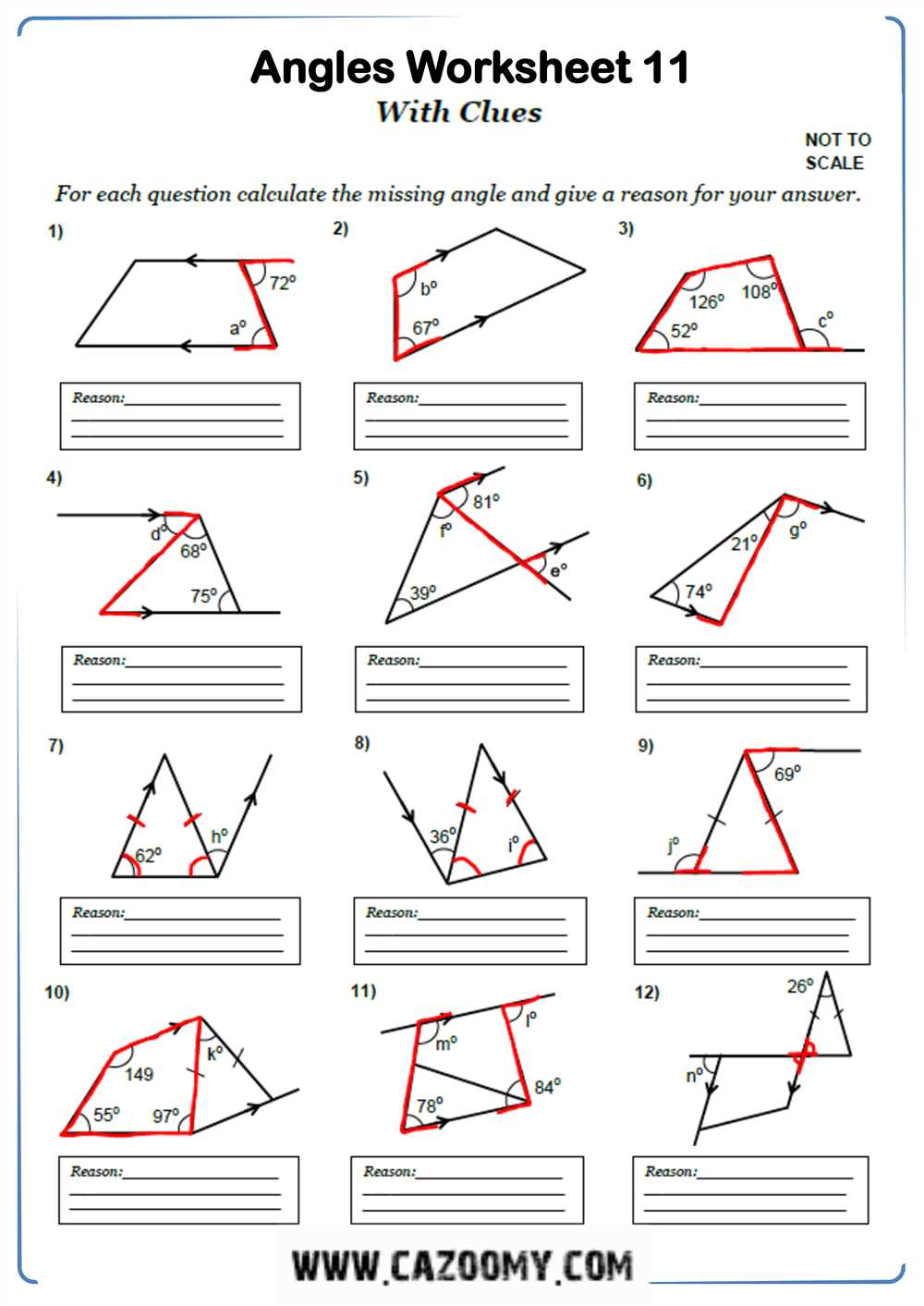
Understanding reference angles is crucial in trigonometry as it allows us to simplify trigonometric functions and solve problems involving angles. A reference angle is the acute angle formed between the terminal side of an angle, when it is in standard position, and the x-axis. By finding the reference angle, we can easily determine the exact value of trigonometric ratios for any given angle.
To help students practice and master this concept, a reference angle worksheet with answers is a valuable resource. This worksheet typically includes a variety of problems, such as finding the reference angle for a given angle, determining the exact value of trigonometric ratios using reference angles, and solving word problems involving reference angles.
The answers provided in the worksheet allow students to check their work and understand where they might have made mistakes. This fosters a self-assessment process, reinforcing their learning and helping them improve their understanding of reference angles. Moreover, the worksheet format provides a structured and organized way for students to practice and reinforce their knowledge of reference angles in a systematic manner.
Reference Angle Worksheet with Answers
In trigonometry, reference angles are used to simplify calculations and find the solutions to various trigonometric equations. A reference angle is the acute angle formed between the terminal side of an angle in standard position and the x-axis. It is always positive and within the range of 0° to 90°.
To practice finding reference angles, you can use a reference angle worksheet with answers. This worksheet provides a set of angles in degrees or radians, and you need to determine their reference angles. The answers are provided to check your work and ensure accuracy.
Here is an example of a reference angle worksheet question along with its answer:
- Question: Find the reference angle for the angle -135°.
- Answer: The reference angle for -135° is 45°. (-135° + 360° = 225°, which is in the second quadrant. The difference between 225° and 180° is 45°, so 45° is the reference angle.)
Completing a reference angle worksheet with answers helps strengthen your understanding of reference angles and improves your ability to solve trigonometric problems. It allows you to practice identifying the appropriate quadrant and determining the reference angle for angles in different positions.
Definition and Calculation of Reference Angles
The concept of reference angles is an important concept in trigonometry. A reference angle is the acute angle formed between the terminal side of an angle in standard position and the x-axis. It is used to simplify calculations and provide a common reference point for angles of different measurements.
To calculate a reference angle, you can follow a few steps. First, determine the quadrant in which the angle lies. This can be done by looking at the sign of the x-coordinate and y-coordinate of the point where the terminal side of the angle intersects with the unit circle. Then, find the angle that is coterminal with the given angle and lies within the same quadrant as the reference angle. Finally, subtract this coterminal angle from 360 degrees or 2π radians to find the reference angle.
For example, let’s say we have an angle of 150 degrees. Since the x-coordinate is negative and the y-coordinate is positive in the second quadrant, we know that the angle lies in the second quadrant. Next, we find the coterminal angle in the second quadrant, which is 180 degrees. Subtracting this from 360 degrees gives us a reference angle of 180 – 150 = 30 degrees.
Reference angles are useful in many trigonometric calculations, such as finding the trigonometric ratios of angles, solving trigonometric equations, and graphing trigonometric functions. They help streamline calculations and provide a consistent starting point for analyzing angles in different contexts.
Practice Problems for Finding Reference Angles
In trigonometry, reference angles are used to simplify calculations and understand the relationship between angles. Finding reference angles involves determining the smallest positive angle formed by the terminal side of an angle and the x-axis. This can be done by subtracting the angle from 360 degrees or 2π radians if the angle is in radians.
To practice finding reference angles, here are some sample problems:
- Problem 1: Find the reference angle for an angle measuring -45 degrees.
- Solution 1: To find the reference angle, we need to determine the positive equivalent of -45 degrees. Adding 360 degrees to a negative angle gives us its positive equivalent. Thus, the reference angle for -45 degrees is 315 degrees.
- Problem 2: Find the reference angle for an angle measuring 225 degrees.
- Solution 2: Since 225 degrees is already within the first quadrant (0 to 90 degrees), its reference angle is simply the original angle itself. Therefore, the reference angle for 225 degrees is 225 degrees.
- Problem 3: Find the reference angle for an angle measuring -π/4 radians.
- Solution 3: To convert -π/4 radians to degrees, we multiply by 180/π. This gives us -45 degrees. However, since -45 degrees is in the fourth quadrant, we need to find its reference angle in the first quadrant. Adding 360 degrees to -45 degrees, we get the positive equivalent of 315 degrees. Therefore, the reference angle for -π/4 radians is 315 degrees.
By practicing these types of problems, you’ll become more familiar with finding reference angles and gain a better understanding of trigonometric concepts.
Steps for Determining Reference Angles
When working with angles in mathematics, it is often helpful to find their corresponding reference angles. A reference angle is the positive acute angle formed by the terminal side of an angle in standard position and the x-axis. It can be used to simplify calculations and find solutions to trigonometric equations.
Step 1: Identify the Given Angle
The first step is to identify the angle for which you want to find the reference angle. This angle can be measured in degrees or radians and may be positive or negative. Make sure to note the direction of rotation (clockwise or counterclockwise) and any quadrant information if applicable.
Step 2: Determine the Quadrant
Next, determine the quadrant in which the given angle lies. This can be done by looking at the sign of the angle (positive or negative) and using the unit circle or coordinate plane. The quadrant information is important for determining the reference angle, as it helps determine the appropriate angle to use from the reference angle chart.
Step 3: Find the Reference Angle
Based on the quadrant information, use the reference angle chart to find the corresponding reference angle. The reference angle chart provides the reference angles for various angles in each quadrant. For example, if the given angle is in the first quadrant, the reference angle would be the same as the given angle. If the given angle is in the second quadrant, the reference angle would be 180 degrees minus the given angle.
By following these steps, you can easily determine the reference angle for any given angle. This will help simplify calculations and solve trigonometric equations more efficiently.
Reference Angle Worksheet
A reference angle is an angle that is formed by rotating a given angle in standard position (anti-clockwise) until it lies between 0° and 90°. It helps in simplifying trigonometric calculations and determining the values of trigonometric functions. In this reference angle worksheet, students are provided with various angles and they are required to find their reference angles.
This worksheet consists of different problems where students need to apply the concept of reference angles. They will be given angles in degrees as well as in radians, and they have to determine the positive acute angle formed by rotating the given angle in standard position. Students will also be asked to find the reference angle in both degrees and radians format.
For example, if the given angle is 180°, the reference angle will be 0°, as the given angle lies on the x-axis and is formed by rotating 180° anti-clockwise. Similarly, if the given angle is -45°, the reference angle will be 45°, as the given angle is formed by rotating 45° clockwise from the positive x-axis.
This reference angle worksheet will help students practice and reinforce their understanding of finding reference angles. It will provide them with ample opportunities to apply the concept and develop their trigonometric skills. Students can also check their answers using the provided answer key, which will help them in self-assessment and monitoring their progress.
Sample Solutions for Reference Angle Worksheet
In the study of trigonometry, understanding reference angles is essential for solving various problems involving angles. A reference angle is the acute angle formed between the terminal side of an angle and the x-axis in standard position. To help students practice finding reference angles, a worksheet with different angle measurements is provided. Here are some sample solutions to the reference angle worksheet:
Problem 1:
Angle: 150 degrees
Solution: The given angle is in the second quadrant. To find the reference angle, we subtract 180 degrees from the given angle since the reference angle is always positive. Reference angle = 150 degrees – 180 degrees = 30 degrees. Therefore, the reference angle for 150 degrees is 30 degrees.
Problem 2:
Angle: 300 degrees
Solution: The given angle is in the fourth quadrant. Since the reference angle is always positive, we subtract 360 degrees from the given angle to find the reference angle. Reference angle = 300 degrees – 360 degrees = -60 degrees. However, the reference angle cannot be negative, so we add 360 degrees to make it positive. Reference angle = -60 degrees + 360 degrees = 300 degrees. Therefore, the reference angle for 300 degrees is 60 degrees.
Problem 3:
Angle: -45 degrees
Solution: The given angle is in the fourth quadrant. Since the reference angle is always positive, we add 360 degrees to the given angle to find the reference angle. Reference angle = -45 degrees + 360 degrees = 315 degrees. Therefore, the reference angle for -45 degrees is 315 degrees.
These are just a few examples of how to find reference angles. By practicing with different angle measurements, students can develop a better understanding of trigonometry and how angles relate to each other. Solving the reference angle worksheet will help reinforce the concept of reference angles and their importance in solving trigonometric problems.
Tips for Mastering Reference Angle Calculation
Understanding reference angles is an essential skill in trigonometry. A reference angle is the positive acute angle formed between the terminal side of an angle and the x-axis in standard position. Here are a few tips to help you master reference angle calculation:
- Familiarize Yourself with the Quadrants: Before calculating the reference angle, it’s important to identify the quadrant in which the angle lies. Each quadrant has specific characteristics that influence the calculation process.
- Use the Unit Circle: The unit circle can be a valuable tool when determining reference angles. By analyzing the coordinates of the points on the unit circle, you can easily find the reference angle for a given angle.
- Remember the Basic Angles: Memorizing the reference angles for the basic angles (0°, 30°, 45°, 60°, and 90°) can save you time during calculations. These angles frequently appear in trigonometric functions and knowing their reference angles can help simplify the process.
- Apply Symmetry: Reference angles exhibit symmetry across the x-axis and y-axis. This means that the reference angle for an angle in a specific quadrant can be found by subtracting the angle from either 90° or 180°, depending on the quadrant.
- Practice with Worksheets: Utilize reference angle worksheets to practice your calculation skills. These worksheets typically provide various angles and require you to identify their reference angles. By practicing consistently, you can improve your speed and accuracy.
By following these tips and consistently practicing, you can become proficient at calculating reference angles. This skill is not only important for trigonometry problems but is also applicable in various real-world scenarios, such as navigation and engineering.