
Exponential and logarithmic equations are fundamental concepts in mathematics and commonly encountered in various fields of science and engineering. These equations involve variables in exponents or logarithms, and finding their solutions can be challenging. To aid students in mastering these equations, a solving exponential and logarithmic equations worksheet with answers in PDF format is a valuable resource.
This worksheet provides a collection of equations and problems that involve exponential and logarithmic functions. It guides students through the process of solving these equations step-by-step, helping them develop a solid understanding of the underlying principles and techniques. The answers provided in the worksheet enable students to check their work and gain confidence in their problem-solving abilities.
The PDF format of the worksheet allows for easy printing and distribution, making it a convenient tool for both teachers and students. Additionally, the worksheet can be used as a self-study resource, allowing students to practice solving exponential and logarithmic equations at their own pace and review the answers to assess their progress.
Overall, a solving exponential and logarithmic equations worksheet with answers in PDF format is an effective tool for enhancing mathematical skills and confidence in tackling complex equations. By practicing with a variety of problems and checking their solutions, students can solidify their understanding and become proficient in solving these types of equations.
What are exponential and logarithmic equations
An exponential equation is an equation in which the variable appears in the exponent. These equations involve exponential functions, which are functions of the form f(x) = a^x, where a is a positive constant. The variable x can represent a variety of quantities, such as time, distance, or population size.
Logarithmic equations are the inverse of exponential equations. They involve logarithmic functions, which are functions of the form f(x) = log_a(x), where a is a positive constant. The logarithmic function is the inverse of the exponential function, so if f(x) = a^x, then the logarithmic equation log_a(f(x)) = x.
Exponential and logarithmic equations are both important in various fields of mathematics and science. They can be used to model various real-world phenomena, such as population growth, radioactive decay, and the spread of diseases. Solving these equations allows us to find the value of the variable that satisfies the equation, which can provide valuable insights and information.
When solving exponential and logarithmic equations, there are different methods and strategies that can be used. These include using properties of logarithms, taking logarithms of both sides of the equation, or using the change of base formula. It is important to pay attention to the domain and range of the functions involved, as well as any restrictions on the variable.
Understanding the basics of exponential equations
Exponential equations are a fundamental concept in mathematics and play a crucial role in various fields, including finance, computer science, physics, and biology. These equations involve exponential functions, which have a specific form where the variable is raised to a constant power.
In its basic form, an exponential equation can be written as y = a * b^x, where a is a constant base, b is the base of the exponential function, x is the exponent, and y is the result. The exponent x represents the number of times the base is multiplied by itself, while the constant base a determines the starting point or initial value.
To solve exponential equations, it is important to understand the properties of exponential functions. One key property is the exponential growth or decay. When the base is greater than 1, the exponential function exhibits exponential growth, meaning that the values increase rapidly as the exponent increases. On the other hand, when the base is between 0 and 1, the function shows exponential decay, and the values decrease rapidly as the exponent increases.
Solving exponential equations often involves finding the value of the exponent or the base, given the other variables. This can be done through various methods, such as using logarithms, simplifying the equation, or using algebraic techniques. In some cases, graphing the exponential function can also help visualize the solution.
In conclusion, understanding the basics of exponential equations is essential for tackling complex mathematical problems and real-life applications. It provides a framework for analyzing and predicting exponential growth or decay, and helps in solving a wide range of problems across different disciplines.
Methods for solving exponential equations
Exponential equations involve variables raised to a power. These equations can be challenging to solve, but there are several methods that can be used to find the solution.
1. Isolate the exponential term: If the exponential term is being added or subtracted to other terms, move all other terms to one side of the equation and isolate the exponential term on the other side. Then, take the logarithm of both sides to solve for the variable.
2. Use the properties of exponents: If the exponential term has the same base on both sides of the equation, you can equate the exponents and solve for the variable. For example, if 2^x = 2^3, then x = 3.
3. Apply logarithmic functions: If the exponential term is not easily simplified, you can use logarithmic functions to solve the equation. Take the logarithm of both sides using a base that matches the exponential term’s base. Then, use logarithmic properties to simplify and solve for the variable.
4. Use graphing technology: If the equation is difficult to solve algebraically, you can use graphing technology to visualize the graph of the equation. The solution will be the x-coordinate of the point where the graph intersects the line y = 0.
These methods provide different strategies for solving exponential equations, and the choice of method depends on the complexity of the equation and the desired level of accuracy. Practice and familiarity with these methods will help improve problem-solving skills in this area.
Guidelines for solving logarithmic equations
When it comes to solving logarithmic equations, there are a few key guidelines to keep in mind. These guidelines can help streamline the process and ensure that you arrive at the correct solution.
1. Identify the base: The first step in solving a logarithmic equation is to identify the base of the logarithm. This is the number that is being raised to a power. It is usually written as a subscript after the logarithm, such as logb(x). Knowing the base is crucial for solving the equation.
2. Use the properties of logarithms: Logarithms have certain properties that can be used to simplify equations. These include the product rule, quotient rule, and power rule. By applying these properties, you can often transform a complex logarithmic equation into a simpler form.
3. Isolate the logarithm: Once you have simplified the equation using logarithmic properties, the next step is to isolate the logarithm on one side of the equation. This may involve moving other terms to the opposite side or combining like terms.
4. Solve for the variable: Once the logarithm is isolated, you can solve for the variable using algebraic techniques. This may involve exponentiating both sides of the equation, applying inverse operations, or using the properties of logarithms to simplify further.
5. Check your solution: After finding a potential solution, always remember to check your answer. Plug the value back into the original equation to ensure that it satisfies the equation and is a valid solution.
By following these guidelines, you can approach logarithmic equations with confidence and effectively solve for the variables. Practice and familiarity with logarithmic properties will also help you become more proficient in solving these types of equations.
Common mistakes to avoid when solving exponential and logarithmic equations
When solving exponential and logarithmic equations, it is important to be aware of common mistakes that can easily be made. These mistakes can lead to incorrect solutions or confusion along the way. By understanding and avoiding these mistakes, you can ensure accurate problem-solving.
1. Mixing up exponential and logarithmic properties
One common mistake is mixing up the properties of exponential and logarithmic functions. It is crucial to remember the properties such as the exponential property that states a^x = b, where a is the base, x is the exponent, and b is the resulting value. Logarithmic functions, on the other hand, are the inverse of exponential functions, and they follow the property log(a, b) = x, where a is the base, b is the value being logged, and x is the resulting exponent. Mixing up these properties can easily lead to confusion and incorrect calculations.
2. Incorrectly applying the laws of exponents and logarithms
The laws of exponents and logarithms are essential tools when solving equations. However, applying them incorrectly can result in mistakes. For example, when simplifying expressions with exponents, it is important to remember the laws such as a^m * a^n = a^(m+n). Similarly, when solving logarithmic equations, it is crucial to correctly apply the laws such as log(a*b) = log(a) + log(b). Failing to apply these laws correctly can lead to incorrect solutions.
3. Not checking for extraneous solutions
Extraneous solutions are solutions that do not satisfy the original equation when plugged back in. This commonly occurs when solving logarithmic equations. It is important to always check the solutions obtained by plugging them back into the original equation. Failing to do so may result in accepting incorrect solutions as valid, leading to inaccurate answers.
4. Misinterpreting the equation

Misinterpreting the equation is another common mistake made when solving exponential and logarithmic equations. It is crucial to correctly understand the given equation and its domain and range. Misinterpreting the equation can lead to incorrect assumptions, incorrect use of properties, and ultimately incorrect solutions.
By being aware of these common mistakes and taking extra caution while solving exponential and logarithmic equations, you can improve your problem-solving skills and achieve accurate results.
Where to find a worksheet for practicing exponential and logarithmic equations
If you are looking for a worksheet to practice solving exponential and logarithmic equations, there are several resources available online. These worksheets are designed to help reinforce your understanding of these mathematical concepts and provide you with the opportunity to practice solving equations in a structured way.
Mathematics websites: Many mathematics websites offer free worksheets for practicing various topics, including exponential and logarithmic equations. Some popular websites include Math-Aids.com, Math Worksheets 4 Kids, and Kuta Software. These websites provide a variety of worksheets with different levels of difficulty, allowing you to choose the ones that suit your needs.
Online tutoring platforms: Online tutoring platforms often provide resources and worksheets for practice. Websites like Khan Academy and Mathway offer free worksheets on exponential and logarithmic equations, as well as step-by-step solutions and explanations. These resources can be helpful if you are looking for additional guidance and support.
Math textbooks or workbooks: If you have access to a math textbook or workbook, there is a high chance that it will include practice problems on exponential and logarithmic equations. Check the index or table of contents to locate the relevant section, and you will likely find a set of practice problems or a worksheet that you can work through.
Teachers and educators: Reach out to your math teacher or educator for additional resources. They may have worksheets or handouts specifically designed for practicing exponential and logarithmic equations. They can also recommend online resources or provide personalized guidance to help you strengthen your skills in this area of mathematics.
Downloadable PDF with answers for exponential and logarithmic equations
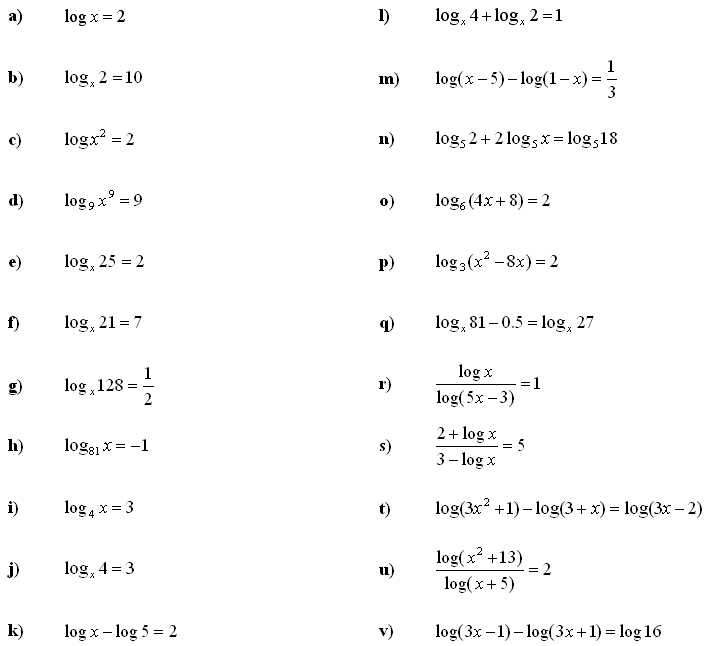
If you are studying exponential and logarithmic equations and need practice solving them, a downloadable PDF with answers can be a helpful resource. This PDF provides a collection of equations and their solutions, allowing you to practice and check your work.
The PDF contains a variety of problems involving exponential and logarithmic functions. These equations may include different bases, constants, and variables. By working through these problems and comparing your answers with the provided solutions, you can gain a better understanding of the concepts and improve your problem-solving skills.
The answers in the PDF can serve as a guide to help you identify any mistakes or misconceptions you may have. They can also provide explanations for the steps involved in solving each equation, helping you to grasp the underlying principles and techniques.
The convenience of having the PDF file allows you to access it anytime and anywhere, whether you are studying at home or on the go. You can print out the worksheets to work on them offline or use a digital device to solve them online. The ability to review the answers and solutions as many times as needed can enhance your learning experience and reinforce your understanding of exponential and logarithmic equations.
Overall, a downloadable PDF with answers for exponential and logarithmic equations can be a valuable tool in your study of these topics. It provides a structured way to practice and gauge your progress, offering opportunities for self-assessment and improvement. So, why not download one today and start mastering exponential and logarithmic equations?
Tips for effective practice and mastering exponential and logarithmic equations
Exponential and logarithmic equations can be challenging to solve, but with effective practice and understanding of the concepts, you can master them. Here are some tips to help you in your journey:
- Review the basics: Before diving into solving more complex exponential and logarithmic equations, make sure you have a solid understanding of the basic properties and rules associated with them. This includes understanding the laws of exponents and logarithms.
- Work on building your algebra skills: Solving exponential and logarithmic equations often involves manipulating algebraic expressions. Practice solving basic algebraic equations to strengthen your skills in simplifying and rearranging equations.
- Practice different types of problems: Exponential and logarithmic equations come in various forms, such as equations with exponential or logarithmic expressions on both sides or equations involving exponential or logarithmic functions. Work on solving different types of problems to become familiar with the various approaches and techniques.
- Understand the properties and rules: Exponential and logarithmic equations have specific properties and rules that can help you simplify and solve them. Understand concepts like the power rule, logarithmic identities, and properties of logarithms to apply them effectively when solving equations.
- Keep track of your steps: When solving exponential and logarithmic equations, it’s essential to keep track of each step and justify every transformation you make. This will help you identify any mistakes and understand the logic behind each step.
- Use online resources and practice worksheets: There are many online resources and practice worksheets available that can provide you with additional practice opportunities. Work through these exercises to reinforce your understanding and improve your problem-solving skills.
- Seek help when needed: If you find yourself struggling with certain concepts or equations, don’t hesitate to seek help from your teacher, classmates, or online communities. Sometimes a different perspective or explanation can make a big difference in your understanding and ability to solve the equations.
By following these tips and dedicating regular practice time, you can effectively work towards mastering exponential and logarithmic equations. Remember, practice and persistence are key in improving your problem-solving skills in this topic.