
Math mazes are a fun and interactive way to practice solving multi-step equations. Level 2 of the math maze is designed to challenge students with more complex equations and varied operations. In order to successfully navigate through the maze and find the correct path, students must correctly solve each equation and use the answer to guide them to the next equation.
The answer key for solving multi-step equations math maze level 2 provides students with the solutions to each equation. This allows them to check their work and ensure they are on the right path. The answer key serves as a helpful tool for both students and teachers, providing immediate feedback and reinforcement of the concepts being learned.
Solving multi-step equations is an essential skill in algebra and lays the foundation for more advanced mathematical concepts. By using math mazes, students can engage in a hands-on and interactive approach to learning, making the process more enjoyable and effective. The answer key for level 2 of the math maze provides a valuable resource for students to practice and master these important skills.
Solving Multi Step Equations Math Maze Level 2 Answer Key

Completing multi-step equations can be challenging, but with the help of the Solving Multi Step Equations Math Maze Level 2 Answer Key, students can easily check their work and determine if they have solved the equations correctly. This answer key serves as a valuable tool for both students and teachers, providing a clear and concise explanation of the correct answers to each problem in the math maze.
The Solving Multi Step Equations Math Maze Level 2 Answer Key contains step-by-step solutions to each equation, ensuring that students can understand the process and reasoning behind each solution. This allows students to not only find the correct answer but also learn the necessary problem-solving techniques for similar equations in the future.
The answer key is organized in a logical manner, with each equation and its corresponding solution clearly labeled and numbered. This makes it easy for students to navigate through the answer key and find the solution to a specific problem they are struggling with. Additionally, the answer key may include helpful hints or tips to assist students in understanding the concepts and strategies used to solve the equations.
With the Solving Multi Step Equations Math Maze Level 2 Answer Key, students can gain confidence in their ability to solve multi-step equations and improve their overall math skills. By comparing their answers to the provided solutions, students can identify any mistakes or misunderstandings they may have and work towards resolving them. This answer key serves as a valuable resource for both independent practice and classroom learning, ensuring that students can effectively master the concept of solving multi-step equations.
Steps for Solving Multi Step Equations
Solving multi step equations can be challenging, but by following a step-by-step process, you can simplify the equation and find the solution. Here are the steps to solve multi step equations:
-
Step 1: Simplify both sides of the equation. Start by simplifying both sides of the equation by combining like terms and using the distributive property if necessary. This will help you eliminate any unnecessary elements and make the equation easier to solve.
-
Step 2: Move the variable terms to one side. Identify any terms with variables and move them to one side of the equation by isolating them. Use inverse operations such as addition, subtraction, multiplication, and division to balance the equation while keeping it equal.
-
Step 3: Move the constant terms to the other side. After isolating the variable terms, move the constant terms (numbers without variables) to the other side of the equation. Again, use inverse operations to maintain equality and simplify the equation further.
-
Step 4: Combine like terms. Once you have separated the variable and constant terms, combine like terms on each side of the equation. This will help you simplify the equation even further and bring you closer to finding the solution.
-
Step 5: Solve for the variable. Use inverse operations to solve for the variable. If the variable is still being multiplied or divided by a coefficient, simplify the expression by dividing or multiplying both sides of the equation accordingly. Eventually, you should be left with the variable isolated on one side of the equation.
-
Step 6: Check your solution. After finding the value of the variable, substitute it back into the original equation to ensure that both sides are equal. If they are, then your solution is correct. If not, recheck your work and look for any mistakes that may have been made during the solving process.
By following these steps, you will be able to solve multi step equations efficiently and accurately. Practice is key in mastering this skill, so be sure to engage in various exercises and problems to strengthen your understanding.
Understanding Level 2 Math Maze
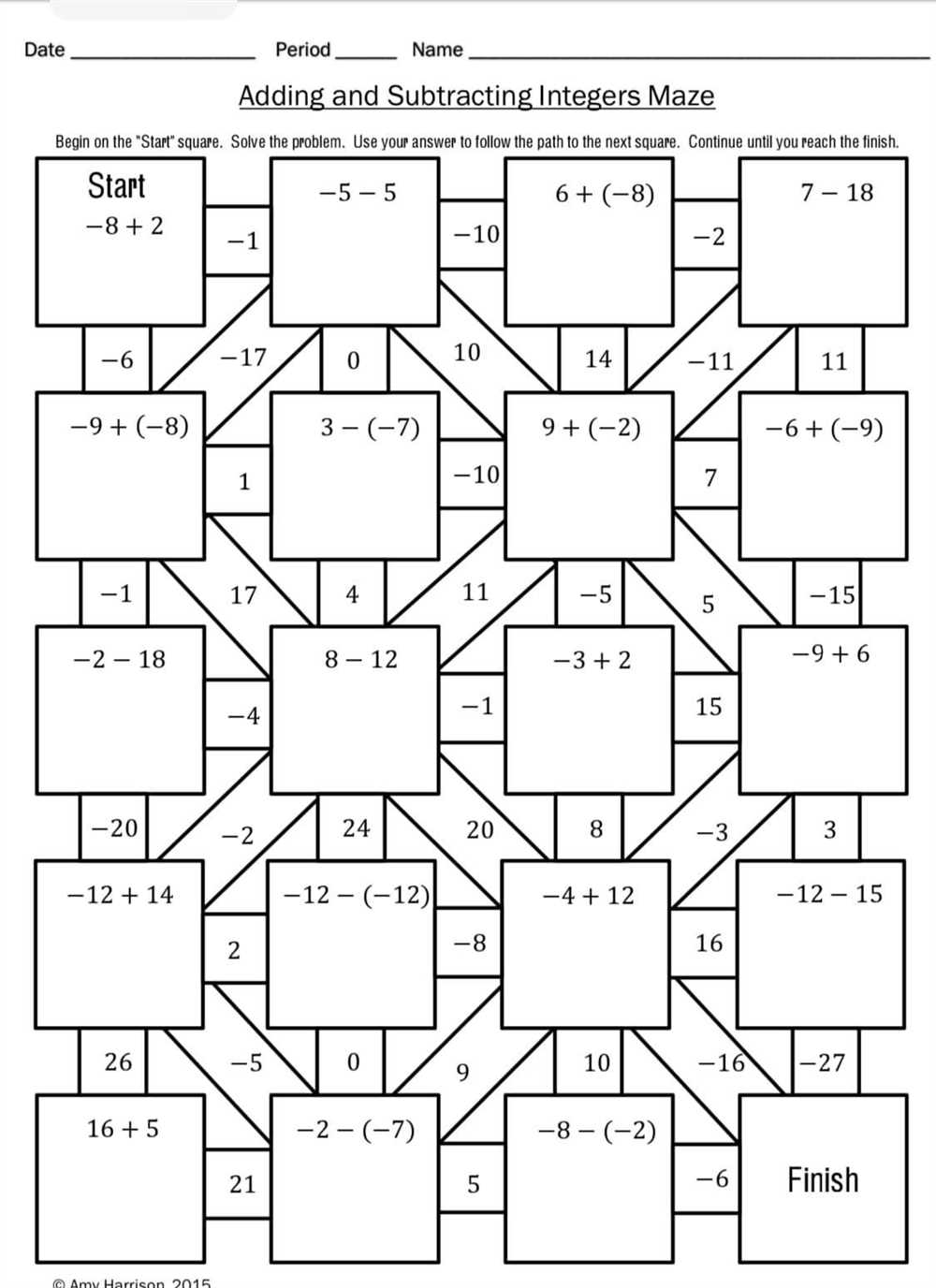
Level 2 of the Math Maze is designed to challenge students’ problem-solving skills by introducing multi-step equations. In this level, students are required to solve equations with multiple operations and variables. It is important for students to have a solid understanding of basic algebraic concepts before attempting this level.
The Math Maze is an interactive activity that helps students develop their critical thinking and logical reasoning skills. The maze consists of a series of equations, and the goal is to solve each equation to find the correct path through the maze. Each equation has multiple steps, which require students to apply various mathematical operations in the correct order.
To successfully complete the Math Maze, students must carefully analyze each equation and break it down into smaller, manageable steps. They need to identify the variables and constants in the equation, and then apply the rules of algebra to isolate the variable. This may involve adding, subtracting, multiplying, or dividing both sides of the equation.
The Math Maze is an excellent tool for reinforcing the concepts learned in the classroom and helping students develop their problem-solving skills. It challenges students to think critically and apply their knowledge of algebraic concepts in a fun and engaging way. Level 2 of the Math Maze provides a more complex and challenging set of equations, allowing students to deepen their understanding of algebra and build their confidence in solving multi-step equations.
Working with Variables and Constants
In mathematics, variables and constants play an important role in solving equations and understanding algebraic concepts. A variable is a symbol that represents a quantity that can change, while a constant is a fixed value that remains the same throughout the equation.
When working with variables and constants, it is crucial to understand the rules and operations involved in manipulating them. In equations, variables are typically represented by letters, such as “x” or “y,” and constants are represented by numbers.
To solve multi-step equations, it is necessary to isolate the variable on one side of the equation. This involves performing various operations, such as addition, subtraction, multiplication, and division, on both sides of the equation. By using inverse operations, the variable can be isolated and its value can be determined.
Math mazes, like the “Solving multi-step equations math maze level 2,” provide a fun and interactive way to practice working with variables and constants. These mazes require students to solve a series of equations by applying the appropriate operations to move through the maze and reach the solution. By completing these mazes, students can develop their problem-solving skills and reinforce their understanding of equations and variables.
Applying Operations to Both Sides
In mathematics, when solving equations, it is often necessary to apply operations to both sides of the equation in order to isolate the variable and find its value. This process involves performing the same operation on both sides of the equation, ensuring that the equality is maintained.
For example, if we have the equation 2x + 5 = 13, we can solve for x by applying operations to both sides. To isolate the variable x, we can subtract 5 from both sides of the equation:
2x + 5 – 5 = 13 – 5
By subtracting 5 from both sides, the equation becomes 2x = 8. Next, we can divide both sides of the equation by 2 to solve for x:
(2x) / 2 = 8 / 2
This simplifies to x = 4, so the solution to the equation is x = 4.
Applying operations to both sides of an equation allows us to maintain the equality while manipulating the equation to solve for the unknown variable. This technique is essential in solving multi-step equations and finding the values of variables in various mathematical problems.
Simplifying Equations by Combining Like Terms
In mathematics, simplifying equations by combining like terms is a crucial skill that helps reduce complex expressions into simpler forms. By combining like terms, we streamline equations and make them easier to solve and understand.
Like terms have the same variables raised to the same powers. They can be added or subtracted because they represent the same quantity. When combining like terms, we add or subtract the coefficients of the like terms while keeping the variables unchanged.
Example:
Consider the equation: 3x + 2y – 4x + 5y. To simplify this equation, we combine the like terms with the same variable.
- Combining like terms for x: 3x – 4x = -x
- Combining like terms for y: 2y + 5y = 7y
The simplified equation becomes: -x + 7y.
Simplifying equations by combining like terms is a fundamental step in algebra. It allows us to express complex expressions in a more concise and intuitive manner, making it easier to solve equations and analyze mathematical relationships. This skill is especially useful when solving multi-step equations, as it helps us eliminate unnecessary complexity and focus on the essential elements of the problem.
Applying the Distributive Property
The Distributive Property is a fundamental concept in mathematics that allows us to simplify expressions involving multiplication. It states that when we multiply a number by a sum or difference of two or more terms, we can distribute the multiplication to each term individually and then combine the results. This property is often used in algebra to simplify equations and solve problems.
For example, let’s consider the expression 3(x + 2). To apply the Distributive Property, we distribute the multiplication of 3 to each term inside the parentheses. This gives us 3(x) + 3(2), which simplifies to 3x + 6. By distributing the multiplication, we have broken down the original expression into simpler terms that are easier to work with.
The Distributive Property can also be used to solve equations. For instance, if we have an equation such as 2(3x – 5) = 16, we can use the Distributive Property to eliminate the parentheses. This gives us 6x – 10 = 16. From here, we can proceed to solve for x using inverse operations.
Understanding and applying the Distributive Property is crucial for solving multi-step equations like the ones found in math mazes. By correctly distributing the multiplication and simplifying each term, we can efficiently navigate through the maze and find the solution. It is a powerful tool that simplifies complex expressions and helps us solve equations in a systematic way.
Overall, the Distributive Property is an important concept in mathematics that allows us to simplify expressions and solve equations. By understanding and applying this property, we can confidently tackle multi-step equations and other mathematical problems.
Checking and Verifying Solutions
When solving multi-step equations, it is important to check and verify our solutions to ensure that they are correct. While the steps we followed may seem logical, it is always possible to make a mistake along the way. By checking our solutions, we can confirm that we have found the correct answer.
One way to check our solution is to substitute the value we found back into the original equation. If the equation holds true when we substitute the solution, then we know that our answer is correct. For example, if we solve the equation 2x + 5 = 13 and find x = 4, we can substitute x = 4 back into the equation: 2(4) + 5 = 13. If the left side of the equation equals the right side, then we have verified that our solution is correct.
Another method to check our solution is to solve the equation using the inverse operation. For example, if we solved the equation 3y – 7 = 8 and found y = 5, we can check our answer by adding 7 to both sides of the equation: 3(5) = 8 + 7. If the left side of the equation equals the right side, then our solution is correct.
In conclusion, checking and verifying solutions is an important step in solving multi-step equations. It helps to ensure that we have found the correct answer and allows us to catch any mistakes we may have made along the way. By using substitution or the inverse operation, we can confidently confirm the accuracy of our solutions.