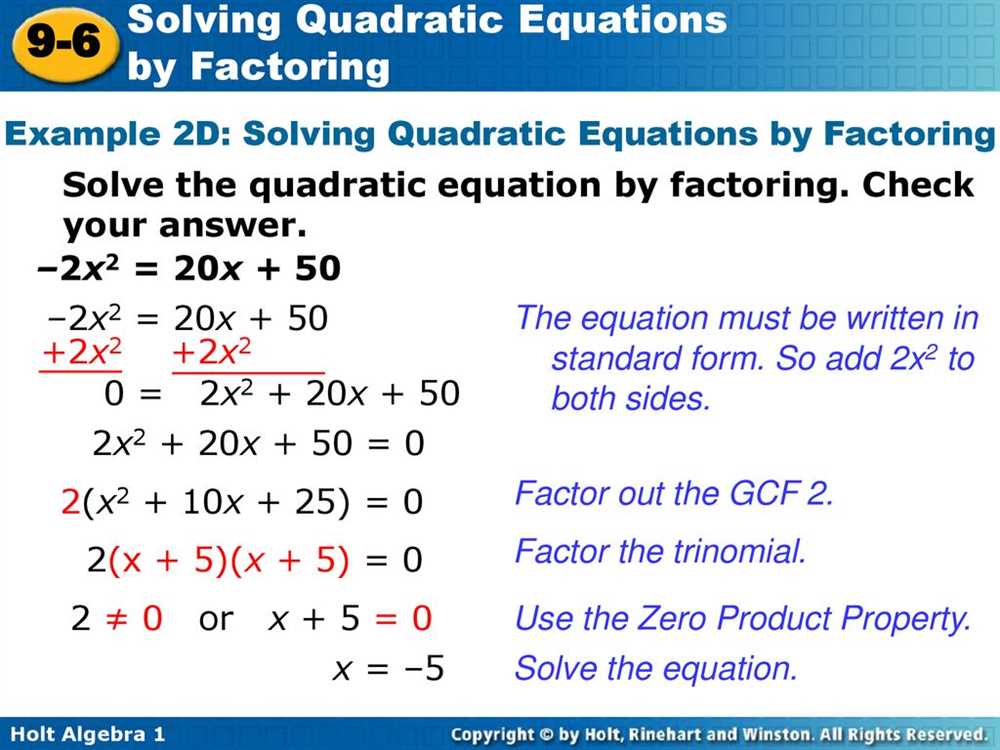
If you’re studying algebra and working on solving quadratic equations by factoring, you may have encountered various types of problems and need some guidance in finding the correct answers. In this article, we will provide you with the answer key to a maze activity that involves solving quadratic equations by factoring.
Solving quadratic equations by factoring is an important skill in algebra as it allows you to find the solutions to equations of the form ax² + bx + c = 0. By factoring the equation, you can determine the values of x that satisfy it, which are known as the solutions or roots.
The maze activity that we will provide the answer key for consists of a series of quadratic equations that need to be factored. Each equation is represented by a lettered path in the maze, and you need to factor the equation correctly to find the correct path through the maze. By following the correct path, you will be able to solve all the equations and find the final destination.
In the answer key provided, we will guide you step by step through the process of factoring each equation correctly. By following the provided explanations, you will gain a deeper understanding of how to solve quadratic equations by factoring. This knowledge will not only help you complete the maze activity successfully but also enhance your overall problem-solving skills in algebra.
Solving Quadratic Equations by Factoring Maze Answer Key
In the topic of solving quadratic equations by factoring, it is important to have answer keys for practice exercises like mazes. An answer key provides students with a way to check their work and ensure they are on the right track. This is particularly useful when solving quadratic equations by factoring, which can involve multiple steps and potential pitfalls.
The solving quadratic equations by factoring maze answer key provides the correct solutions to the maze. It presents the step-by-step process of factoring quadratic equations and arrives at the final answer. This allows students to compare their own solutions with the answer key and identify any mistakes they may have made.
By using the solving quadratic equations by factoring maze answer key, students can gain a better understanding of the concept and improve their problem-solving skills. They can also learn from any errors they make and work towards improving their accuracy and efficiency in solving quadratic equations by factoring.
Example Answer Key
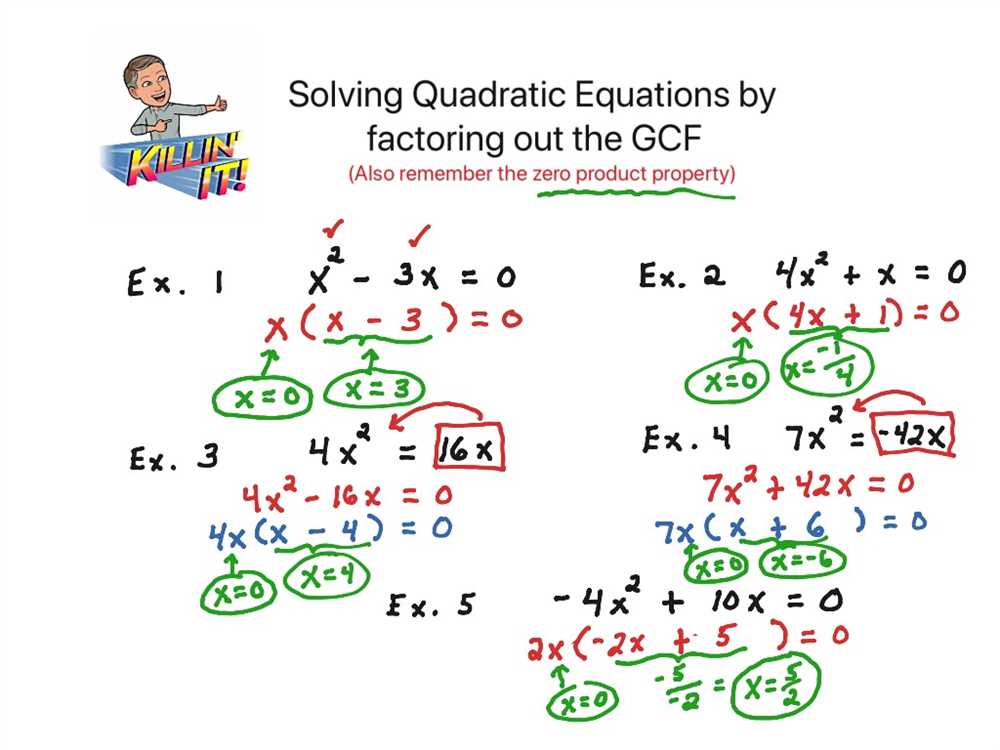
- Step 1: Write the quadratic equation in the form of (ax^2 + bx + c = 0).
- Step 2: Factor the quadratic equation into two binomials.
- Step 3: Set each binomial equal to zero and solve for (x).
- Step 4: Check the solutions by substituting them back into the original equation.
| Example Problem | Answer |
|---|---|
| (x^2 – 5x + 6 = 0) | (x = 2) or (x = 3) |
| (2x^2 + 7x – 3 = 0) | (x = -3) or (x = frac{1}{2}) |
The solving quadratic equations by factoring maze answer key is a valuable resource for both students and teachers. It allows students to check their work and learn from their mistakes, while also providing teachers with a tool to assess student understanding and progress. By practicing with the answer key, students can strengthen their problem-solving skills in solving quadratic equations by factoring.
What is a quadratic equation?
A quadratic equation is a second-degree polynomial equation in one variable, typically written in the form: ax^2 + bx + c = 0, where “a”, “b”, and “c” are coefficients and “x” is the variable. In this equation, the highest power of the variable is 2, making it a quadratic equation.
Quadratic equations can have various forms, but they can always be rearranged to the standard form. The quadratic equation can represent a parabola, a U-shaped curve, on a graph. The solutions of a quadratic equation, also known as the roots, correspond to the x-coordinates where the parabola intersects the x-axis.
Quadratic equations often arise in real-world problems, particularly in physics and engineering. They are used to model and solve various situations, such as finding the maximum height of a projectile, determining the roots of a quadratic function, or calculating the time it takes for an object to reach a certain distance.
Solving quadratic equations can be done through several methods, such as factoring, using the quadratic formula, completing the square, or graphing. Each method has its advantages and may be more appropriate depending on the specific equation and context. Factoring is a common method to solve quadratic equations as it involves finding the factors that can be multiplied to produce the original equation. This method is often used when the quadratic equation can be easily factored.
Overall, quadratic equations play a crucial role in mathematics and have practical applications in various fields. Understanding how to solve quadratic equations is essential for problem-solving and mathematical analysis.
Why do we solve quadratic equations by factoring?
Factoring is a valuable method for solving quadratic equations because it allows us to break down the equation into smaller, manageable parts. By factoring a quadratic equation, we can find the values of x that make the equation equal to zero, which are known as the roots or solutions of the equation.
One of the main advantages of factoring quadratic equations is that it can be a relatively straightforward and intuitive process. By factoring out common factors or using other factoring techniques, we can often express the quadratic equation as a product of two binomial expressions. This allows us to set each binomial equal to zero and solve for the values of x. Factoring often provides a clear and concise solution to quadratic equations.
In addition to its simplicity, factoring can also help us gain a deeper understanding of the quadratic equation and its behavior. By factoring the equation, we can identify the factors that contribute to the equation being equal to zero. This can help us determine critical points, intercepts, and other key features of the quadratic equation’s graph.
Overall, solving quadratic equations by factoring is a powerful method that allows us to find the roots of the equation and gain insight into its behavior. Factoring provides a clear and intuitive approach to solving quadratic equations, making it an essential tool in algebra and mathematics.
Step-by-step guide to factoring quadratic equations
Factoring quadratic equations is an essential skill in algebra, allowing us to easily solve equations of the form ax^2 + bx + c = 0. By factoring the quadratic expression into two binomial factors, we can set each factor equal to zero and solve for x. This step-by-step guide will walk you through the process of factoring quadratic equations.
Step 1: Check for special cases
Before factoring a quadratic equation, it’s important to check if it fits any special cases, such as when the leading coefficient is 1 or when the quadratic expression is a perfect square trinomial. If it does, you can skip to Step 4. Otherwise, proceed to Step 2.
Step 2: Multiply the leading coefficient and the constant term
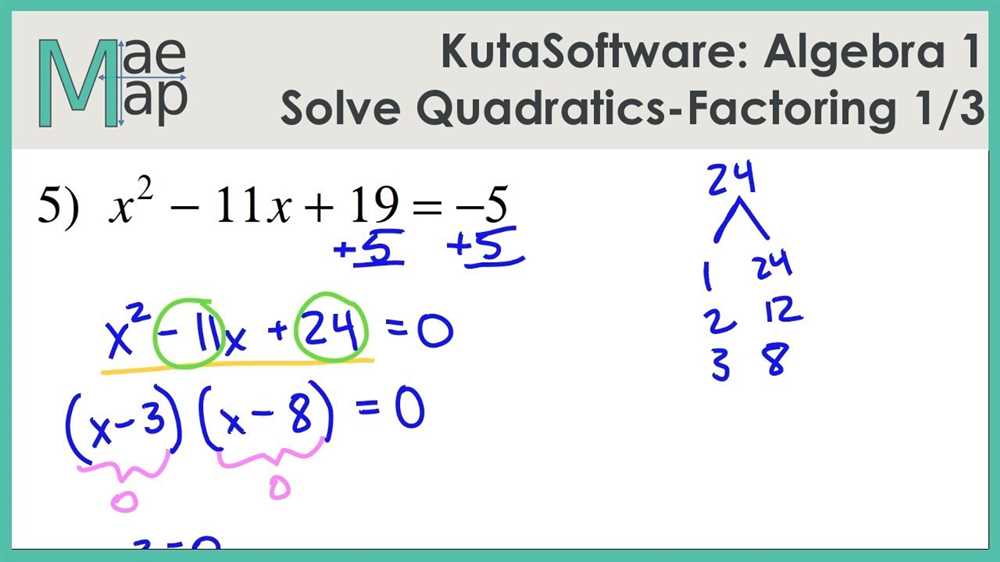
Multiply the coefficient ‘a’ and the constant term ‘c’ of the quadratic expression. This product will be used to find pairs of factors in Step 3.
Step 3: Find pairs of factors
Find pairs of factors of the product from Step 2 that add up to the coefficient ‘b’ of the linear term. These factors will be used to create two binomial factors in Step 4.
Step 4: Write the quadratic expression as a product of binomials
Using the pairs of factors from Step 3, write the quadratic expression as a product of binomials. Set each binomial factor equal to zero and solve for x to find the solutions to the quadratic equation.
Step 5: Check for extraneous solutions
After finding the solutions to the quadratic equation, it’s important to check if any of them result in the division by zero or the use of undefined values. These solutions are called extraneous solutions and should be discarded.
By following these steps, you can successfully factor quadratic equations and solve for x. Practice and repetition will help you become more proficient in this fundamental algebraic skill.
Understanding the maze method for solving quadratic equations
The maze method is a visual and systematic approach for solving quadratic equations by factoring. It helps students understand the process of factoring and provides a clear path to finding the solutions. By following the steps of the maze, students can navigate through the equation and determine the factors needed to solve it.
The maze method starts by writing the quadratic equation in standard form, where the equation is set equal to zero. This allows for easier factoring and solving. Then, the equation is factored by finding two binomials whose product is equal to the original equation. The coefficients of these binomials are determined by the factors of the quadratic equation’s terms.
The maze method provides a visual representation of the factoring process. The equation is written in a grid format with lines and boxes. Each line represents a factor, and each box represents a term in the equation. By following the lines and boxes, students can determine the factors and solve the equation.
The maze method also helps students understand the concept of finding the roots of a quadratic equation. The roots are the values of x that make the equation equal to zero. By factoring the equation and setting each factor equal to zero, students can find the values of x that satisfy the equation.
Overall, the maze method is an effective strategy for solving quadratic equations by factoring. It provides a visual and systematic approach that helps students understand the process of factoring and finding the solutions. By following the steps of the maze, students can confidently solve quadratic equations and deepen their understanding of algebraic concepts.
Tips and Tricks for Solving Quadratic Equations by Factoring
Solving quadratic equations by factoring is an important skill in algebra. By finding the factors of a quadratic equation, we can determine its roots and solve for the unknown variable. Here are some tips and tricks to help you solve quadratic equations by factoring:
- 1. Look for common factors: Start by factoring out any common factors from the quadratic equation. This simplifies the equation and makes it easier to find the remaining factors.
- 2. Use the quadratic formula: If the quadratic equation cannot be easily factored, you can always resort to using the quadratic formula, which is x = (-b ± √(b^2 – 4ac)) / 2a. This formula will give you the roots of the quadratic equation.
- 3. Try factoring by grouping: If the quadratic equation has four terms, you can try factoring by grouping. Group the terms in pairs and look for common factors within each pair. Then, factor out the common factors and see if you can further simplify the equation.
- 4. Look for perfect square trinomials: Sometimes, a quadratic equation can be factored into a perfect square trinomial. This means that the quadratic has two equal factors. Look for patterns in the equation that indicate a perfect square trinomial.
- 5. Practice factoring techniques: The more you practice factoring quadratic equations, the better you will become at recognizing patterns and finding the factors. Use worksheets, online resources, and math software to practice factoring techniques.
Solving quadratic equations by factoring may seem challenging at first, but with practice and a good understanding of factoring techniques, you can become proficient in solving these equations. Remember to always check your solutions by substituting them back into the original equation to ensure they are correct.
Common Mistakes to Avoid When Factoring Quadratic Equations
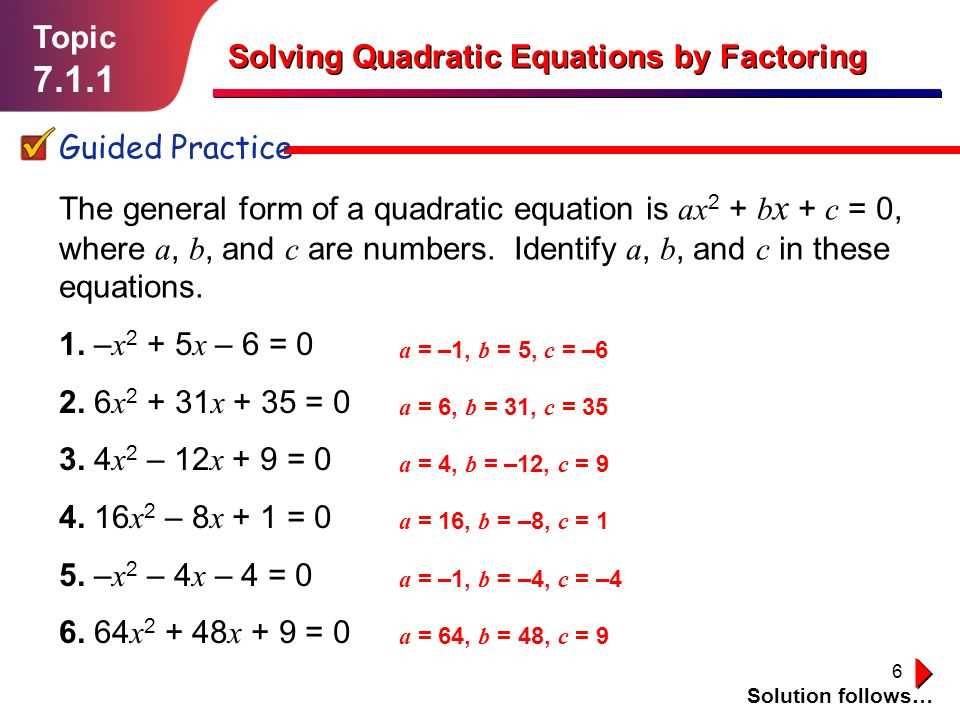
Factoring quadratic equations can be a challenging task, especially if you are not familiar with the proper steps and techniques. Here are some common mistakes to avoid when factoring quadratic equations:
- Incorrectly identifying the quadratic equation: One of the most common mistakes is not correctly identifying the quadratic equation. It is important to remember that a quadratic equation is a polynomial equation of degree 2, meaning it contains a term with x squared.
- Skipping the step of setting the equation equal to zero: Another mistake is skipping the crucial step of setting the equation equal to zero. When factoring a quadratic equation, you need to move all terms to one side of the equation and set it equal to zero to begin the factoring process.
- Missing the common factor: Sometimes, students overlook the common factor when factoring a quadratic equation. It is essential to always check if there is a common factor that can be factored out before continuing with the factoring process.
- Forgetting to reverse the signs: Another common mistake is forgetting to reverse the signs when factoring a quadratic equation. When using the factoring method, it is important to reverse the signs of the constant terms in the factored equation.
- Not checking the factored equation: Finally, it is crucial to check the factored equation to ensure that it is correct. This can be done by multiplying the factored terms and simplifying the equation to see if it is equivalent to the original quadratic equation.
Avoiding these common mistakes will help you successfully factor quadratic equations and reach the correct solutions. Practice and familiarity with the factoring process will also contribute to improved accuracy and efficiency in solving quadratic equations.
How to check your answers for quadratic equations by factoring
After solving a quadratic equation by factoring, it is important to check your answers to ensure accuracy and correctness. Here are a few steps to help you go through the process of checking your solutions:
- Substitute the values back into the original equation: Start by substituting the solutions you obtained back into the original equation in order to verify if they satisfy the equation. Simply replace the x variable with the solution and check if both sides of the equation are equal.
- Simplify and evaluate: Once you have substituted the values, simplify the equation by performing all the necessary calculations and determine if the left side of the equation is equal to the right side. Double check your arithmetic throughout this step to avoid any errors.
- Verify consistency: Check whether the solutions obtained are consistent with the nature of quadratic equations. For example, a quadratic equation can have two real solutions, one real solution, or no real solutions. Cross-check the number of solutions obtained with what is expected.
- Graphically analyze the solutions: If possible, graph the quadratic equation and identify the x-values of the solutions obtained. Verify if the points where the graph intersects the x-axis match with the solutions you found.
It is crucial to take the time to check your answers for quadratic equations solved by factoring as it helps in identifying any potential mistakes or errors made during the process. This practice not only helps in improving your problem-solving skills but also reinforces your understanding of factoring and quadratic equations as a whole.