
Understanding the concepts of speed and velocity is crucial in physics and everyday life. Speed refers to how quickly an object moves, while velocity takes into account both speed and direction. In simple terms, speed is a scalar quantity, while velocity is a vector quantity.
When solving problems involving speed and velocity, it is important to have a clear understanding of the key terms and equations involved. The answer key provides detailed explanations and solutions to common questions related to speed and velocity.
One of the key equations used to calculate speed is the formula speed = distance / time. This equation allows us to determine how fast an object is moving based on the distance it has traveled and the time it took to travel that distance. The answer key breaks down this equation step by step to help you understand how to use it in various scenarios.
Velocity adds another layer of complexity to the concept of speed. While speed only considers how fast an object is moving, velocity takes into account both speed and direction. This means that two objects can have the same speed but different velocities if they are moving in different directions.
The answer key also covers topics such as average speed, instantaneous speed, and acceleration. These concepts are essential for understanding how objects move and change their motion over time. By mastering the answer key, you will gain a solid foundation in speed and velocity, allowing you to solve more advanced physics problems with ease.
Speed and Velocity Answer Key
When studying the concepts of speed and velocity, it is essential to have a clear understanding of the key terms and formulas associated with these principles. This will enable you to solve problems and calculate values with accuracy. Here is an answer key that provides the necessary information to help you navigate through speed and velocity questions.
Key Terms:
- Speed: The distance traveled by an object per unit of time.
- Velocity: The speed of an object in a given direction.
- Distance: The total path traveled by an object.
- Displacement: The change in position of an object, taking into account direction.
- Scalar: A quantity that only has magnitude (e.g., speed).
- Vector: A quantity that has both magnitude and direction (e.g., velocity).
Formulas:
- Speed: Speed = Distance / Time
- Velocity: Velocity = Displacement / Time
- Speed with direction: Speed with direction = Distance / Time + Direction
- Acceleration: Acceleration = Change in Velocity / Time
Using these key terms and formulas, you can answer various speed and velocity questions. Remember to pay attention to the units used in the problem and ensure they are consistent throughout your calculations. Practice solving different types of problems to strengthen your understanding of these concepts and improve your problem-solving skills.
Understanding the Difference between Speed and Velocity
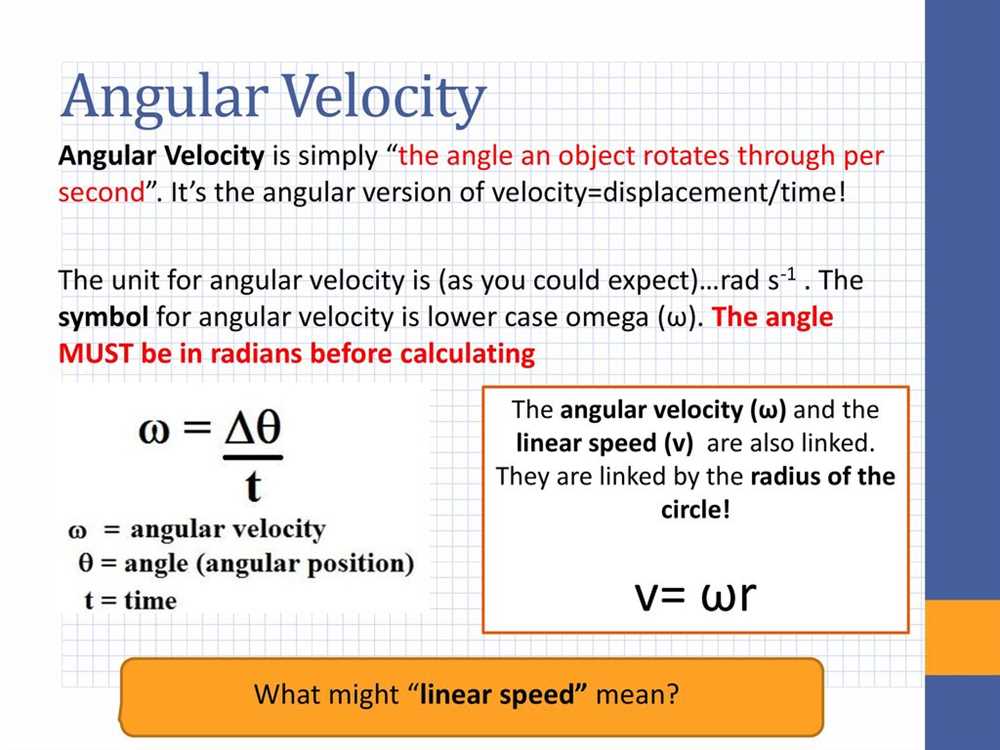
In the context of physics, speed and velocity are often used interchangeably, but they actually have distinct meanings. Speed refers to how fast an object is moving, while velocity takes into account both the object’s speed and its direction of motion. This subtle difference between the two concepts is crucial in understanding the behavior of objects in motion.
Speed is a scalar quantity, meaning it only has magnitude and no direction. It is calculated by dividing the distance traveled by the time taken. For example, if a car covers 100 kilometers in 2 hours, its speed would be 50 kilometers per hour. Speed is often represented by the symbol “v”. It is a fundamental concept in kinematics, the branch of physics that deals with the motion of objects.
Velocity, on the other hand, is a vector quantity, meaning it has both magnitude and direction. It takes into account the displacement of an object, which is the straight-line distance between its starting and ending points. Velocity is calculated by dividing the displacement of an object by the time taken. For example, if a car moves 80 kilometers north in 1 hour, its velocity would be 80 kilometers per hour north. Velocity is often represented by the symbol “v”. It is a key concept in both kinematics and dynamics.
Understanding the difference between speed and velocity is essential because it allows scientists and engineers to accurately describe and predict the motion of objects. For example, a car may have a high speed, but if it is constantly changing direction, its velocity may be low. Similarly, two objects may have the same speed, but if they are moving in opposite directions, their velocities will be different. By considering both speed and direction, velocity provides a more complete description of an object’s motion.
Calculating Speed and Velocity
Speed and velocity are important concepts in physics that help us understand how objects move. Both speed and velocity involve the measurement of an object’s change in position over time, but they differ in their directionality. Speed is a scalar quantity that only considers the magnitude of an object’s motion, while velocity is a vector quantity that includes both the magnitude and direction of an object’s motion.
Calculating speed is a relatively straightforward process. It is defined as the distance traveled by an object divided by the time taken to travel that distance. To calculate speed, one can use the formula:
Speed = Distance/Time
- Speed: The rate at which an object moves.
- Distance: The length of the path traveled by an object.
- Time: The duration of the motion.
For example, if an object travels a distance of 100 meters in 20 seconds, the speed can be calculated as:
Speed = 100 meters / 20 seconds = 5 meters per second
Calculating velocity requires an additional consideration of direction. Velocity is defined as the displacement of an object divided by the time taken to cover that displacement. Displacement is the change in position of an object, taking into account its starting and ending points. To calculate velocity, one can use the formula:
Velocity = Displacement/Time
- Velocity: The rate at which an object changes its position.
- Displacement: The change in position of an object.
- Time: The duration of the motion.
For example, if an object starts at position 5 meters and ends at position 15 meters in 10 seconds, the displacement is 10 meters (15 meters – 5 meters). The velocity can be calculated as:
Velocity = 10 meters / 10 seconds = 1 meter per second (in the positive direction)
Calculating speed and velocity allows us to quantitatively analyze and describe the motion of objects. These calculations are fundamental in various fields, including physics, engineering, and sports science, and provide valuable insights into the dynamics of motion.
Speed and Velocity Formulas

In physics, speed and velocity are important concepts to understand the motion of objects. Speed is a scalar quantity that measures how fast an object is moving, while velocity is a vector quantity that not only measures the speed but also the direction of the motion. To calculate speed, we use the formula speed = distance / time. This formula compares the distance traveled by an object to the time it takes to travel that distance. It is important to note that speed does not indicate the direction of motion, only how fast an object is moving.
On the other hand, velocity takes into account both the speed and the direction of motion. It is calculated using the formula velocity = displacement / time. Displacement refers to the change in position of an object, while time represents the time taken for the displacement to occur. Velocity is a vector quantity because it has both magnitude (the speed) and direction.
Example: Let’s say an object travels a total distance of 100 meters in 10 seconds. To calculate the speed, we divide the distance by the time: speed = 100 meters / 10 seconds = 10 meters per second. This means the object is moving at a speed of 10 meters per second.
Example: Now let’s consider an object that travels a displacement of 50 meters north in 5 seconds. To calculate the velocity, we divide the displacement by the time: velocity = 50 meters / 5 seconds = 10 meters per second north. This means the object is moving at a velocity of 10 meters per second in the north direction.
It is important to remember that speed and velocity are different concepts, where velocity includes both speed and direction. The formulas provided can be used to calculate speed and velocity accurately, allowing us to understand and analyze the motion of objects in various situations.
Units Used to Measure Speed and Velocity
When measuring the speed and velocity of an object, it is important to use specific units of measurement. These units help to provide a standardized way to compare different objects and their rates of motion. The most commonly used units for measuring speed and velocity include meters per second (m/s), kilometers per hour (km/h), and miles per hour (mph).
Meters per second (m/s) is the standard unit used to measure both speed and velocity in the International System of Units (SI). This unit represents the distance traveled in meters divided by the time taken in seconds. It is often used in scientific and mathematical calculations to provide precise measurements of motion.
Kilometers per hour (km/h) is another commonly used unit to measure speed. As its name suggests, this unit represents the distance traveled in kilometers divided by the time taken in hours. Kilometers per hour is often used in everyday situations, such as measuring the speed of a car or a runner, as it provides a more relatable and easily understood measurement.
Miles per hour (mph) is the unit used to measure speed in most countries that do not use the metric system. Similar to kilometers per hour, this unit represents the distance traveled in miles divided by the time taken in hours. It is commonly used in the United States and other non-metric countries, particularly in relation to speed limits and vehicle speeds.
In conclusion, the units used to measure speed and velocity, such as meters per second, kilometers per hour, and miles per hour, play a crucial role in accurately quantifying an object’s rate of motion. These units provide a standardized and easily relatable way to compare different objects and their speeds, whether in scientific calculations or everyday situations.
Graphing Speed and Velocity
Graphing speed and velocity is an important skill in understanding the motion of an object. By plotting data on a graph, we can visually analyze the relationship between time and distance, and determine the speed and velocity of the object.
Speed: Speed is a measure of how fast an object is moving. It is calculated by dividing the distance traveled by the time taken. When graphing speed, we plot distance on the y-axis and time on the x-axis. The resulting graph will show a linear relationship, with the slope representing the speed of the object. A steeper slope indicates a higher speed, while a shallower slope indicates a slower speed.
Velocity: Velocity is similar to speed, but it also takes into account the direction of motion. It is defined as the rate of change of displacement. When graphing velocity, we plot displacement on the y-axis and time on the x-axis. The resulting graph may not always be linear, as it depends on the object’s acceleration and changes in direction. The slope of the graph represents the average velocity over a specific time interval.
In addition to analyzing the slope of the graph, we can also use the shape of the graph to determine other properties of an object’s motion. For example, a horizontal line on a speed graph indicates that the object is not moving, while a negative slope indicates that the object is moving in the opposite direction. Similarly, on a velocity graph, a positive slope indicates motion in the forward direction, while a negative slope indicates motion in the opposite direction.
Overall, graphing speed and velocity provides valuable insights into an object’s motion. It allows us to quantitatively analyze the relationship between time and distance or displacement, and helps us understand how speed and velocity change over time.
Speed and Velocity in Newtonian Physics
In Newtonian physics, the concepts of speed and velocity are fundamental in understanding the motion of objects. Both speed and velocity describe how fast an object is moving, but they differ in their definition and the information they provide.
Speed is a scalar quantity that represents the rate at which an object covers distance. It is determined by dividing the distance traveled by the time taken. Speed is always positive and does not have a specific direction. It tells us how quickly an object is moving, but it doesn’t provide any information about the direction of the motion.
Velocity, on the other hand, is a vector quantity that includes both the speed of an object and its direction. It is determined by dividing the displacement of an object by the time taken. Displacement is a vector that represents the change in position of an object and has both magnitude and direction. Velocity tells us not only how fast an object is moving but also in which direction it is moving.
The relationship between speed and velocity can sometimes be confusing. For example, if an object travels in a straight line at a constant speed, its speed is equal to its magnitude of velocity. However, if the object changes its direction, even if the magnitude of its velocity remains constant, its speed will differ from its velocity. In general, speed is a scalar quantity and velocity is a vector quantity.
To summarize, in Newtonian physics, speed represents the rate at which an object covers distance, while velocity includes both speed and direction. Understanding these concepts is essential for predicting and analyzing the motion of objects in the physical world.