
When it comes to understanding the surface area of a sphere and hemisphere, having a comprehensive worksheet with answers can be invaluable. It allows students to practice their skills and check their work, ensuring they have a solid understanding of the concepts.
In this article, we will provide a complete worksheet with answers for calculating the surface area of a sphere and hemisphere. These exercises will cover a range of difficulty levels, allowing students to progress at their own pace while gaining confidence in their abilities.
The worksheet will include problems that require students to find the surface area using both the diameter and radius. It will also cover questions that involve finding the surface area of a hemisphere, a half of a sphere.
By offering detailed solutions and explanations for each problem, this worksheet will serve as an excellent resource for teachers and students alike. It will help students grasp the fundamental concepts and strengthen their skills in calculating the surface area of spheres and hemispheres.
What is Surface Area?
Surface area refers to the total area of the outer surface of an object. It is a measure of how much space is occupied by the object’s surface. Surface area is an important concept in geometry and is used to calculate the amount of material needed to cover an object or the amount of paint required to paint it.
The surface area of an object can be found by adding up the areas of all its individual faces or surfaces. For example, a cube has six equal-size square faces, so its surface area is the sum of the areas of those squares.
When dealing with curved objects, such as spheres or hemispheres, calculating the surface area becomes more complex. In these cases, formulas specific to the shape are used. For example, the surface area of a sphere is given by the formula 4πr^2, where r is the radius of the sphere.
Understanding surface area is useful in many practical applications. For instance, architects need to calculate the surface area of walls to determine the amount of materials required for construction. Manufacturers need to know the surface area of objects to calculate packaging requirements. Additionally, surface area plays a role in various scientific fields, such as chemistry and physics.
In summary, surface area is the total area of the outer surface of an object and is calculated by adding up the areas of its individual faces or by using specific formulas for curved objects. It is a crucial concept in geometry with practical applications in fields like construction, manufacturing, and science.
Definition of Sphere and Hemisphere
A sphere is a perfectly round three-dimensional object in space. It is defined as the set of all points equidistant from a common center point. This center point is known as the center of the sphere. The distance from the center to any point on the surface of the sphere is called the radius.
A hemisphere, on the other hand, is half of a sphere. It is a three-dimensional shape that is formed by cutting a sphere into two equal halves along its equator. The equator of a sphere is a circle that divides the sphere into two hemispheres – the upper hemisphere and the lower hemisphere. Each hemisphere has its own center and radius.
Properties of Sphere:
- A sphere has no edges or vertices.
- All points on the surface of a sphere are equidistant from the center.
- The surface area of a sphere can be calculated using the formula 4πr2, where r is the radius.
- The volume of a sphere can be calculated using the formula 4/3πr3.
Properties of Hemisphere:
- A hemisphere has a curved, convex surface.
- The base of a hemisphere is a circle.
- The surface area of a hemisphere can be calculated using the formula 2πr2 + πr2, where r is the radius.
- The volume of a hemisphere can be calculated using the formula 2/3πr3.
Understanding the definitions and properties of sphere and hemisphere is important when solving problems related to surface area and volume. By knowing these concepts, we can accurately calculate and analyze the measurements of these three-dimensional objects.
Formulas for Surface Area
Surface area is the total area of all the surfaces of a three-dimensional object. It is an important concept in geometry and is often used to calculate the amount of material needed to cover an object or the amount of paint needed to coat it.
For different types of geometric objects, there are specific formulas to calculate their surface area. Here are some common formulas:
- Cube: The surface area of a cube can be found by multiplying the length of one side by itself and then multiplying that result by 6. The formula is 6s², where s is the length of a side.
- Rectangular prism: The surface area of a rectangular prism can be found by adding the areas of all six faces. The formula is 2lw + 2lh + 2wh, where l is the length, w is the width, and h is the height.
- Sphere: The surface area of a sphere can be found by multiplying the square of its radius by 4π. The formula is 4πr², where r is the radius.
- Cylinder: The surface area of a cylinder can be found by adding the areas of its two circular bases and the area of its curved surface. The formula is 2πr² + 2πrh, where r is the radius and h is the height.
- Cone: The surface area of a cone can be found by adding the area of its circular base and the area of its curved surface. The formula is πr² + πrl, where r is the radius and l is the slant height.
These formulas can be used to find the surface area of various objects, allowing for precise calculations in real-world applications such as construction, manufacturing, and architecture.
Surface Area of Sphere Formula
When it comes to calculating the surface area of a sphere, there is a specific formula to follow. The surface area of a sphere is given by the formula:
Surface Area = 4πr2
In this formula, π represents the mathematical constant Pi, which is approximately equal to 3.14159, and r represents the radius of the sphere. The radius is the distance from the center of the sphere to any point on its surface.
The formula for the surface area of a sphere can be derived by using calculus, but it is often taught as a basic mathematical formula in geometry. It can be understood intuitively by visualizing the sphere as a collection of infinitely many small circular plates, each with an area of 4πr2. When all these circular plates are added together, the result is the total surface area of the sphere.
Calculating the surface area of a sphere is useful in various real-world applications, such as determining the amount of material needed to cover a spherical object or calculating the heat transfer rate from a hot sphere to its surroundings. It is also an important concept in mathematics and geometry, providing insights into the properties and characteristics of three-dimensional objects.
Overall, the surface area of a sphere formula is a fundamental equation that allows us to quantify and analyze the surface area of spheres in various contexts. Understanding and applying this formula can help in solving problems and making accurate calculations related to spheres and their surface areas.
Surface Area of Hemisphere Formula
The surface area of a hemisphere is a key concept in geometry. It refers to the total area of the curved surface of a hemisphere, which is half of a sphere. The formula to calculate the surface area of a hemisphere is derived from the formula for the surface area of a sphere.
The surface area of a hemisphere can be found using the formula:
Surface Area = 2πr²
- The symbol π represents the mathematical constant pi, which is approximately equal to 3.14159.
- The variable r represents the radius of the hemisphere, which is the distance from the center of the hemisphere to any point on its curved surface.
To calculate the surface area of a hemisphere, you need to know the radius of the hemisphere. Once you have the radius, you can substitute it into the formula and perform the necessary calculations to find the surface area.
It’s important to note that the surface area of a hemisphere only includes the curved surface. It does not take into account the flat, circular base of the hemisphere. If you want to find the total surface area including the base, you would need to add the area of the circular base to the surface area of the curved surface.
Understanding the surface area of a hemisphere is crucial for various applications, such as architecture, engineering, and manufacturing. For example, when designing a dome-shaped structure, knowledge of the surface area of a hemisphere can help determine the amount of materials needed and estimate the cost of construction.
In summary, the surface area of a hemisphere is calculated using the formula Surface Area = 2πr², where π represents pi and r represents the radius of the hemisphere. This formula is essential in geometry and has practical applications in various fields.
Example Problems
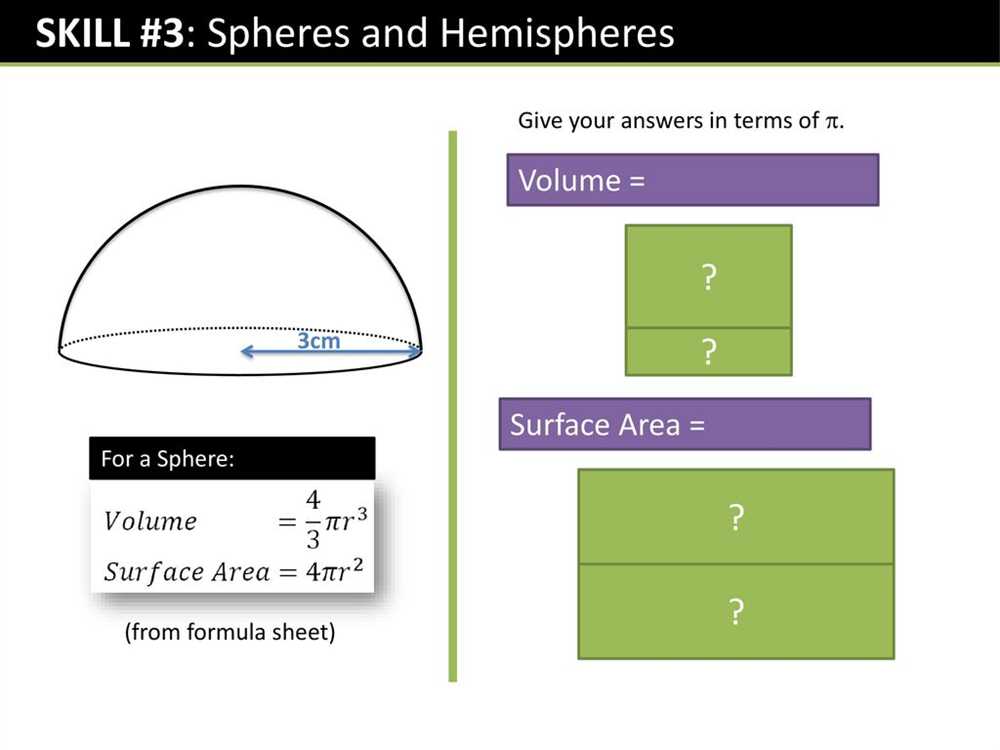
In order to understand and apply the concepts of surface area and volume of spheres and hemispheres, it is important to work through example problems. This will help to solidify the understanding of the formulas and techniques used to solve these types of problems.
Finding the Surface Area of a Sphere:
Example 1: Find the surface area of a sphere with a radius of 5 centimeters.
To find the surface area of a sphere, we use the formula SA = 4πr^2, where r is the radius of the sphere.
Given that the radius is 5 centimeters, we can substitute this value into the formula:
- SA = 4π(5)^2
- SA = 4π(25)
- SA = 100π
So, the surface area of the sphere is 100π square centimeters.
Finding the Surface Area of a Hemisphere:
Example 2: Find the surface area of a hemisphere with a radius of 8 inches.
The formula to find the surface area of a hemisphere is SA = 2πr^2, where r is the radius of the hemisphere.
Given that the radius is 8 inches, we can substitute this value into the formula:
- SA = 2π(8)^2
- SA = 2π(64)
- SA = 128π
Therefore, the surface area of the hemisphere is 128π square inches.
Additional Tips:
1. When finding the surface area of a hemisphere, remember to multiply the result by 2, as a hemisphere is half of a sphere.
2. Always use the appropriate units in your final answer.
By working through example problems like these, you can gain a better understanding of how to calculate the surface area of spheres and hemispheres. Practice is key when it comes to mastering these concepts, so don’t hesitate to try more problems on your own!
Finding the Surface Area of a Sphere
The surface area of a sphere is the measure of the total area that encloses it. It is different from the volume, which measures the space inside the sphere. To find the surface area of a sphere, we can use a formula that involves the radius of the sphere.
Formula: The surface area of a sphere is given by the formula: 4πr^2, where r is the radius of the sphere.
To use this formula, we need to know the radius of the sphere. The radius is the distance from the center of the sphere to any point on its surface. Once we have the radius, we can substitute it into the formula and calculate the surface area.
For example, if we have a sphere with a radius of 5 units, we can calculate its surface area using the formula: 4π(5^2) = 4π(25) = 100π square units.
It is important to note that the surface area of a sphere is always measured in square units, as it represents the area of a two-dimensional shape. This measure allows us to compare the surface areas of different spheres and understand their relative sizes.
In summary, finding the surface area of a sphere involves using the formula 4πr^2 and substituting the radius of the sphere into it. This calculation gives us the total area that encloses the sphere and allows us to compare the sizes of different spheres.
Finding the Surface Area of a Hemisphere
When it comes to finding the surface area of a hemisphere, there is a specific formula that needs to be followed. A hemisphere is half of a sphere, and its surface area can be thought of as the curved part of the sphere plus the circular base. The formula for finding the surface area of a hemisphere is:
Surface Area = 2πr2 + πr2
The first term in the formula, 2πr2, represents the curved surface area of the hemisphere. This is calculated by finding the area of a circle with radius r and multiplying it by 2π. The second term, πr2, represents the surface area of the circular base.
To find the surface area of a hemisphere, you need to know the radius of the hemisphere. The radius is the distance from the center of the sphere to any point on its surface. Once you have the radius, you can substitute it into the formula and calculate the surface area.
For example, let’s say you have a hemisphere with a radius of 5 units. Plugging this into the formula, we get:
- Surface Area = 2π(5)2 + π(5)2
- Surface Area = 50π + 25π
- Surface Area = 75π
So, the surface area of the hemisphere with a radius of 5 units is 75π square units.
In conclusion, finding the surface area of a hemisphere requires using the formula that combines the curved surface area and the surface area of the circular base. By knowing the radius of the hemisphere, you can easily calculate its surface area using this formula.
Worksheet Answers

Math worksheets are invaluable tools for students to practice and reinforce their understanding of various mathematical concepts. They provide an opportunity for students to apply what they have learned in class to solve problems independently. However, it is essential for students to have access to accurate and reliable worksheet answers to check their work and ensure they are on the right track. Worksheet answers serve as a valuable resource for self-assessment, allowing students to identify and correct any mistakes they may have made.
The answers to worksheets on surface area of spheres and hemispheres are essential for students to verify their calculations and ensure accuracy. These topics involve complex formulas and calculations, making it crucial to have reliable answers for comparison. By checking their answers, students can identify any areas of confusion or misunderstandings and seek clarification from their teacher if needed. Moreover, having access to correct answers helps students build confidence in their problem-solving abilities and reinforces their understanding of the concepts.
For example:
- Calculating the surface area of a sphere involves using the formula A = 4πr², where r is the radius. Students can verify their calculated surface area by comparing it to the provided answer. If there is a discrepancy, they can review their calculations and identify any errors.
- Similarly, finding the surface area of a hemisphere requires calculations using the formula A = 2πr², where r is the radius. Students can use the provided answers to ensure accuracy in their calculations and identify any mistakes.
Having accessible worksheet answers encourages students to take ownership of their learning and develop problem-solving skills. It enables them to identify their strengths and weaknesses, allowing them to devote additional practice time to areas where they need improvement. Additionally, comparing their answers to the correct solutions fosters critical thinking and enhances their ability to identify patterns and relationships in mathematical problems.
In conclusion, worksheet answers play a vital role in helping students learn and understand concepts related to surface area of spheres and hemispheres. They provide a means for self-assessment, error correction, and reinforcement of learning. By having access to accurate answers, students can build confidence and achieve a deeper understanding of mathematical concepts.