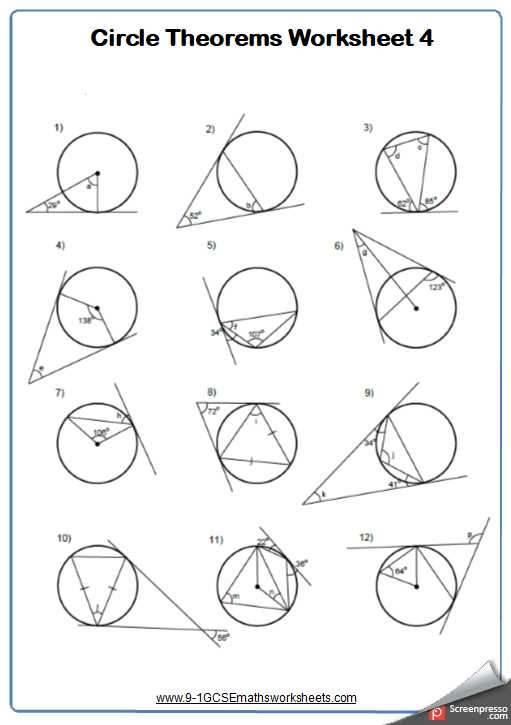
In geometry, tangents to circles are lines that intersect the circles at only one point, called the point of tangency. These tangents play a crucial role in understanding the properties of circles and their relationship with other geometric figures. To test your understanding of tangents to circles, you can use a worksheet that provides questions and problems related to this topic.
A tangents to circles worksheet in PDF format is a convenient way to practice and reinforce your knowledge of tangents. It contains a set of exercises with different levels of difficulty, allowing you to gradually challenge yourself and improve your skills. By solving these problems, you can develop a deeper understanding of the concepts and principles underlying tangents to circles.
The answers to a tangents to circles worksheet in PDF format are provided so that you can check your work and assess your progress. These answers are usually included at the end of the worksheet or in a separate answer key. By comparing your answers with the correct ones, you can identify any mistakes or misunderstandings you may have and learn from them.
Working on a tangents to circles worksheet in PDF format can be a helpful study tool for geometry students. It allows you to practice applying theorems and formulas related to tangents, solidify your understanding of the topic, and improve your problem-solving skills. Additionally, it provides immediate feedback through the answer key, helping you track your progress and make necessary revisions.
Tangents to Circles Worksheet PDF Answers
In the context of geometry, tangents to circles are lines that just touch a circle at one point without actually intersecting it. These tangents are perpendicular to the radius of the circle at the point of tangency. Understanding and working with tangents to circles is an essential skill for solving various geometry problems.
A worksheet is a highly effective tool for practicing and mastering concepts related to tangents to circles. A Tangents to Circles Worksheet PDF provides a collection of questions and problems that require students to apply their knowledge of tangents to circles in a variety of scenarios. The PDF format makes it easy to distribute and print the worksheet for individual or classroom use.
The Tangents to Circles Worksheet PDF often includes questions that involve finding the length of tangents, determining the angle formed between a tangent and a radius, and solving for unknown variables using the properties of tangents to circles. The answers provided in the Tangents to Circles Worksheet PDF Answers serve as a guide for students to check their work and understand the correct solutions.
By solving the problems in the Tangents to Circles Worksheet PDF, students can enhance their understanding of the properties and relationships of tangents to circles. They can develop problem-solving skills and practice applying the relevant formulas and theorems. The answers in the PDF also provide a way for students to self-assess their progress and identify areas that require further study or clarification.
Overall, the Tangents to Circles Worksheet PDF Answers serve as a valuable resource for both teachers and students. Teachers can use the worksheet to assess their students’ understanding and progress in the topic of tangents to circles. Students can utilize the answers to verify their solutions, learn from their mistakes, and reinforce their comprehension of tangents to circles.
Tangent to a Circle Definition

In geometry, a tangent to a circle is a line that touches the circle at exactly one point, without intersecting it. The point where the tangent line touches the circle is called the point of tangency. This line is perpendicular to the radius of the circle at that point of tangency.
A tangent line can be visualized as a straight line that just grazes the circle’s edge, barely touching it. It does not go through the circle or extend beyond it. The tangent line is always perpendicular to the radius of the circle drawn to the point of tangency. This means that the angle between the tangent line and the radius is 90 degrees.
To find the equation of a tangent to a circle, we need the coordinates of the center of the circle and the radius. Using these, we can determine the equation of the line that passes through the center of the circle and the point of tangency. The equation of this tangent line can then be calculated using point-slope form or slope-intercept form.
Intersecting lines and tangent lines have different properties when it comes to circles. While tangent lines touch the circle at exactly one point, intersecting lines can meet the circle at multiple points. This distinction is important in geometry and has significant implications for solving problems and proving theorems related to circles.
In summary, a tangent to a circle is a line that touches the circle at exactly one point, without intersecting it. It is perpendicular to the radius of the circle at that point of tangency. Tangent lines have unique properties that differentiate them from intersecting lines, making them an important concept in geometry.
Properties of Tangents to Circles
A tangent line is a straight line that intersects a circle at exactly one point. It is important to understand the properties of tangents to circles in order to solve problems and find the relationship between different elements of a circle.
1. A tangent line is perpendicular to the radius that intersects the point of tangency. This means that the tangent line forms a right angle with the radius at the point of tangency.
2. The length of the tangent segment from the point of tangency to the intersection point is equal to the radius of the circle. This property can be used to find the length of the radius or vice versa.
3. Two tangent lines drawn from an external point to a circle are congruent. This means that the lengths of the tangent segments from the external point to the point of tangency are equal.
4. The angle formed between the tangent line and the radius at the point of tangency is equal to the angle formed between the radius and the chord that intersects the circle at the same point. This property can be used to find missing angles in a circle.
5. In a circle, a chord is considered a secant, but when extended, it becomes a tangent. This means that a tangent is a special case of a secant that intersects the circle at one point only.
- To find the equation of a tangent line to a circle at a given point, the slope of the tangent line is equal to the negative reciprocal of the slope of the radius at that point.
- When two circles are tangent to each other externally, the distance between their centers is equal to the sum of their radii.
Identifying Tangents in Circles

When studying circles, one important concept to understand is the concept of tangents. A tangent is a line that intersects a circle at exactly one point, and is perpendicular to the radius at that point. Being able to identify tangents in a circle is crucial in solving problems involving circles and their properties.
To identify tangents in a circle, it is important to remember that a tangent line will always be perpendicular to the radius at the point of intersection. This means that the angle formed between the tangent line and the radius will always be 90 degrees. By visually inspecting the circle, one can look for lines that meet this criterion to identify potential tangents.
One way to determine if a line is a tangent to a circle is to draw the radius that intersects the circle at the point of intersection with the line. If the line is indeed a tangent, the radius drawn will be perpendicular to the line. Another method is to examine the angles formed by the line and the radius at the point of intersection. If the angle measures 90 degrees, then the line is a tangent.
Identifying tangents in circles is an important skill in solving problems involving circles, such as finding the length of a tangent or determining the position of a point outside the circle relative to the tangent. By understanding the properties of tangents and the relationships they have with circles, one can confidently solve problems involving tangents in circles.
Finding Tangents to Circles Given Center and Point
Tangents to circles are lines that touch the circle at only one point. Finding the equation of a tangent to a circle requires knowing the center of the circle and a point on the circle. By using the distance formula and the slope formula, the equation of the tangent line can be determined.
To find the equation of a tangent to a circle, start by finding the slope of the line connecting the center of the circle to the given point on the circle. This can be done using the formula (y2 – y1) / (x2 – x1) where (x1, y1) is the center of the circle and (x2, y2) is the given point. The slope of the tangent line will be the negative reciprocal of this slope.
Next, use the point-slope form of a line equation, which is y – y1 = m(x – x1), where (x1, y1) is the given point on the circle and m is the slope of the tangent line. Substitute the values for the given point and the slope into this equation to obtain the equation of the tangent line.
Finally, simplify the equation to put it into a desired form. This may involve distributing, combining like terms, or rearranging the equation. The equation should be in the form y = mx + b, where m is the slope of the tangent line and b is the y-intercept of the line.
In conclusion, finding tangents to circles given the center and a point on the circle involves finding the slope of the line connecting the center and the point, determining the negative reciprocal of this slope, using the point-slope form of a line equation, and simplifying the equation to put it into the desired form.
Finding Tangents to Circles Given Two Points on the Circle
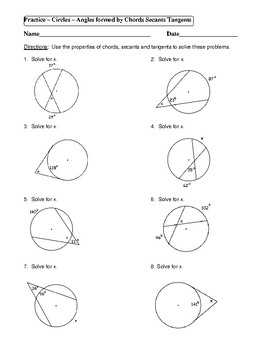
When given two points on a circle, it is possible to find the tangent lines to the circle at those points. Tangent lines are lines that touch the circle at only one point, and are perpendicular to the radius of the circle at that point.
To find the equation of the tangent line to a circle, we can use the following steps:
- First, find the slope of the line that connects the two given points on the circle. This slope is also the slope of the radius of the circle connecting those two points.
- Next, find the negative reciprocal of the slope found in step 1. This will be the slope of the tangent line.
- Using the point-slope form of a line, plug in the coordinates of one of the given points and the slope found in step 2 to find the equation of the tangent line.
It is important to note that the equation of the tangent line will vary depending on which two points on the circle are given.
By following these steps, we can find the equation of the tangent line to a circle given two points on the circle. This can be helpful in various applications, such as finding the tangent line to a curve at a specific point or determining the direction of motion for an object moving along a circular path.
Finding the Length of a Tangent Segment
A tangent segment is a line segment that touches a circle at exactly one point, called the point of tangency. Finding the length of a tangent segment can be useful in various geometric problems and real-life applications.
To find the length of a tangent segment, we can use the theorem that states that the radius drawn to the point of tangency is perpendicular to the tangent line. This means that we can form a right triangle with the radius as the hypotenuse and the tangent segment as one of the legs.
Step 1: Identify the radius of the circle and the point of tangency. The radius is a line segment that connects the center of the circle to the point of tangency.
Step 2: Draw a line segment from the center of the circle to the point of tangency, making sure it is perpendicular to the tangent line. This line segment is the radius.
Step 3: Use the Pythagorean theorem to find the length of the tangent segment. The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. In this case, the hypotenuse is the radius and the other leg is the tangent segment. Substitute the known values and solve for the unknown length.
By following these steps, you can find the length of a tangent segment using basic geometric principles. This information can be used in various mathematical calculations and real-life scenarios.
Proofs Involving Tangents to Circles

When working with circles, tangents play an important role in various proofs and geometric constructions. A tangent to a circle is a line that intersects the circle at exactly one point, known as the point of tangency. In this article, we will explore some common proofs involving tangents to circles.
One commonly used proof is the theorem that states that a line drawn from the center of a circle to a point of tangency is perpendicular to the tangent line. This theorem can be proved using basic geometric principles, such as the definition of a tangent line and the properties of right angles. By showing that the line from the center to the point of tangency and the tangent line form a right angle, we can conclude that they are perpendicular.
Another proof involving tangents to circles is the tangent-chord theorem. This theorem states that when a tangent and a chord intersect at a point on a circle, the measure of the angle formed is equal to half the measure of the intercepted arc. This theorem can be proven using the properties of inscribed angles and intercepted arcs. By showing that the angles formed by the tangent and the chord are inscribed angles and that they intercept the same arc, we can conclude that they have equal measures.
These are just a few examples of proofs involving tangents to circles. By understanding and applying the properties of tangents, chords, and inscribed angles, we can solve various geometric problems and prove a wide range of theorems. Tangents to circles are an essential concept in geometry and have numerous applications in real-world situations.
Practice Exercises and Tangents to Circles Worksheet PDF Answers
Tangents to circles are a fundamental concept in geometry that involve understanding the relationship between a line and a circle. To help students practice this concept, educators often provide worksheets with practice exercises.
These practice exercises usually include various problems that require students to find the equation of a tangent line to a circle or determine the length of a tangent segment. The purpose of these exercises is to help students develop their problem-solving skills and gain a deeper understanding of the properties of tangents to circles.
One way to assess their progress is to provide students with the Tangents to Circles Worksheet PDF. This PDF includes a series of practice problems and their corresponding answers. By reviewing the answers, students can check their work and identify any mistakes they may have made.
The Tangents to Circles Worksheet PDF provides a comprehensive set of exercises covering different aspects of tangent lines, such as finding the point of tangency, determining the slope of the tangent line, and calculating the length of the tangent segment. It is a valuable resource for both teachers and students in their journey to master this fundamental geometric concept.
Overall, the Tangents to Circles Worksheet PDF and its answers are an essential tool in helping students practice and reinforce their understanding of tangents to circles. By working through these problems and verifying their answers, students can build their confidence and proficiency in this area of geometry.