
In the study of physics, waves play a crucial role in explaining various phenomena in the universe. Transverse waves, in particular, are fascinating as they exhibit unique characteristics that enable them to transmit energy perpendicular to the direction of wave propagation. To enhance understanding of these waves, worksheets are often used to assess knowledge and provide practice problems. In this article, we will delve into transverse waves worksheet answers to gain a better grasp of the concepts.
One common question on transverse waves worksheets is about identifying the different parts of a transverse wave. A transverse wave consists of several key elements, such as crest and trough, amplitude, wavelength, and frequency. The crest represents the highest point of the wave, while the trough is the lowest point. The amplitude refers to the maximum displacement from equilibrium, while the wavelength is the distance between two consecutive crests or troughs. Finally, the frequency is the number of waves passing a point in a given time.
Another important aspect covered in transverse waves worksheets is wave propagation. Students may be asked to explain how transverse waves travel through a medium or even in a vacuum. It is essential to understand that transverse waves do not require a material medium to propagate; they can travel through empty space, unlike longitudinal waves. This characteristic makes transverse waves invaluable in explaining phenomena such as electromagnetic waves, which can transmit energy through a vacuum.
Furthermore, transverse waves worksheets often touch upon the relationship between wave properties. For example, students may be asked to calculate the frequency of a wave given its wavelength and the speed at which it propagates. Understanding this relationship helps in comprehending the fundamental principles of waves and how their properties are interconnected. Overall, by reviewing transverse waves worksheet answers, one can deepen their understanding of transverse waves and their applications in various fields of science and technology.
What are Transverse Waves?

A transverse wave is a type of wave in which the particles of the medium vibrate perpendicular (or at right angles) to the direction in which the wave travels. In other words, the motion of the particles is side to side or up and down, while the wave itself moves forward.
One common example of a transverse wave is a wave traveling along a rope that is being shaken at one end. As the rope is shaken up and down, the wave moves along the length of the rope, while the particles of the rope move back and forth perpendicular to the direction of the wave.
Transverse waves can also occur in other types of mediums, such as water. When a stone is thrown into a pond, it creates ripples that move outwards. These ripples are transverse waves, as the particles of the water move up and down as the wave travels horizontally.
Transverse waves have several key properties. They have crests and troughs, which are the highest and lowest points of the wave, respectively. They also have amplitude, which is the maximum displacement of a particle from its equilibrium position, and wavelength, which is the distance between two consecutive points on the wave that are in phase. The speed of a transverse wave is determined by the frequency of the wave and the wavelength.
Overall, transverse waves are an important concept in physics and have many practical applications, such as in communication systems, optics, and music. Understanding their behavior and properties is essential for understanding the world around us.
Properties of Transverse Waves
Transverse waves are a type of wave that move perpendicular (or at right angles) to the direction of the wave’s propagation. They are characterized by a series of crests and troughs, where the crests are the highest points of the wave and the troughs are the lowest points. Here are some key properties of transverse waves:
- Amplitude: The amplitude of a transverse wave refers to the maximum displacement of any point on the wave from its equilibrium position. It is measured in units of distance, such as meters.
- Wavelength: The wavelength of a transverse wave is the distance between two consecutive crests or troughs. It is measured in units of distance, such as meters.
- Frequency: The frequency of a transverse wave is the number of complete oscillations (or cycles) the wave completes in a given time period. It is measured in units of hertz (Hz).
- Period: The period of a transverse wave is the time it takes for one complete oscillation (or cycle) of the wave to occur. It is the reciprocal of the frequency and is measured in units of time, such as seconds.
- Speed: The speed of a transverse wave is the rate at which the wave propagates through a medium. It is determined by the wavelength and the frequency of the wave and is measured in units of distance per time, such as meters per second.
These properties of transverse waves are important in understanding how they behave and interact with different mediums. They can be used to calculate various quantities related to the wave, such as its energy and power.
Overall, transverse waves have distinct characteristics that distinguish them from other types of waves. Understanding these properties is fundamental in fields such as physics, engineering, and telecommunications.
Understanding Wave Motion
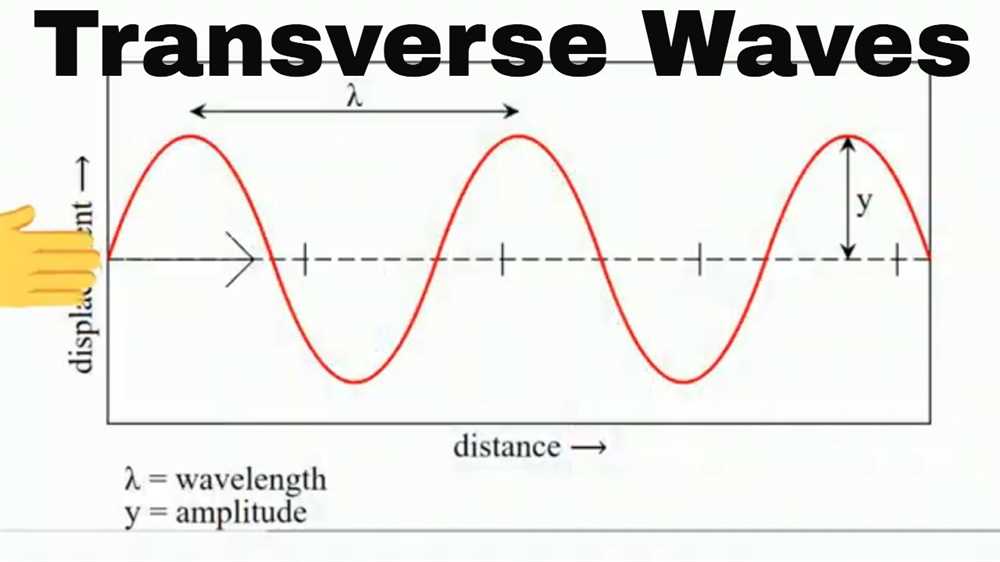
Wave motion is a fascinating phenomenon that can be observed in various aspects of our daily lives. From sound waves to water waves, understanding how waves move and interact is essential in multiple fields of science and engineering. Waves can be categorized into two main types: transverse waves and longitudinal waves. In this article, we will focus on understanding transverse waves and their characteristics.
A transverse wave is a type of wave motion where the particles oscillate perpendicular to the direction of energy transfer. Imagine a rope being shaken from one end; the disturbance travels along the rope, creating peaks and troughs. These peaks and troughs represent the crests and troughs of the transverse wave. The distance between two consecutive crests or troughs is known as the wavelength. The time taken for a complete wave cycle to pass through a point is called the period, and the number of complete wave cycles passing through a point per second is known as the frequency.
Characteristics of Transverse Waves:
- Particle motion: The particles in a transverse wave move perpendicular to the direction of wave propagation.
- Amplitude: The amplitude of a wave is the maximum displacement of a particle from its equilibrium position.
- Wavelength: The wavelength is the distance between two consecutive crests or troughs of a wave.
- Period: The period is the time taken for a complete wave cycle to pass through a point.
- Frequency: The frequency is the number of complete wave cycles passing through a point per second.
Transverse waves play a crucial role in many fields, including physics, telecommunications, and even music. Understanding the concepts and properties of these waves allows scientists and engineers to manipulate and utilize them for various applications. Whether it’s transmitting information through radio waves or enjoying the melody of a guitar string, the principles of transverse waves are at the core of these phenomena.
By studying and comprehending wave motion, we can gain a deeper understanding of the natural world and harness its power for technological advancements. From the behavior of light to the movement of seismic waves, waves are an essential aspect of our physical reality. So, next time you marvel at the beauty of ocean waves or enjoy the sounds of your favorite song, remember the intricate science behind wave motion and the wonders it brings.
Concept of Wave Motion
Wave motion is a fundamental concept in physics that involves the transfer of energy through oscillatory disturbances in a medium. Waves can be characterized by their wavelength, frequency, amplitude, and speed. They are classified into two main types: transverse waves and longitudinal waves.
In transverse waves, the oscillations occur perpendicular to the direction of wave propagation. This means that the particles of the medium vibrate up and down or side to side as the wave passes through them. Examples of transverse waves include light waves, water waves, and electromagnetic waves.
Longitudinal waves, on the other hand, have oscillations that occur parallel to the direction of wave propagation. In this type of wave, the particles of the medium vibrate back and forth in the same direction that the wave is moving. Sound waves are a common example of longitudinal waves.
The key properties of waves include wavelength, which is the distance between two consecutive points in phase; frequency, which is the number of oscillations per unit of time; and amplitude, which is the maximum displacement of particles from their equilibrium position. Waves also have a speed, which is the rate at which the wave travels through the medium.
Understanding the concept of wave motion is essential in various areas of science and engineering. It is used in fields such as acoustics, optics, telecommunications, and seismology. Waves play a crucial role in transmitting information, generating sound, producing images, and detecting earthquakes.
Wave Parameters and Equations
A transverse wave is a type of wave that moves perpendicular to the direction of the wave’s propagation. It is characterized by several parameters and equations that describe its properties and behavior. Understanding these parameters and equations is essential in studying and analyzing transverse waves.
Amplitude: The amplitude of a transverse wave is the maximum displacement of the wave from its equilibrium position. It represents the maximum height or depth of the wave. The greater the amplitude, the more energy the wave carries.
Wavelength: The wavelength of a transverse wave is the distance between two consecutive crests or troughs of the wave. It is represented by the symbol λ (lambda) and is usually measured in meters. The wavelength determines the spatial extent of the wave.
Frequency: The frequency of a transverse wave is the number of complete waves passing through a point in one second. It is represented by the symbol f and is measured in hertz (Hz). The frequency is inversely proportional to the wavelength, meaning that as the wavelength increases, the frequency decreases, and vice versa.
Period: The period of a transverse wave is the time it takes for one complete wave to pass a point. It is represented by the symbol T and is measured in seconds. The period is inversely proportional to the frequency, meaning that as the frequency increases, the period decreases, and vice versa.
Wave Speed: The wave speed of a transverse wave is the speed at which the wave travels through a medium. It is represented by the symbol v and is calculated by multiplying the wavelength by the frequency: v = λf. The wave speed determines how quickly the wave propagates through a medium.
Understanding and using these parameters and equations allows scientists and engineers to predict and control the behavior of transverse waves. By manipulating the amplitude, wavelength, frequency, period, and wave speed, various applications in fields such as telecommunications, engineering, and medicine can be developed and improved.
Transverse Waves in Different Mediums
A transverse wave is a type of wave in which the particles of the medium vibrate perpendicular to the direction of wave propagation. This means that the particles move up and down or side to side as the wave passes through the medium. Transverse waves can be found in various mediums, each with its own unique properties and behavior.
In a solid medium, such as a metal rod or a rope, transverse waves travel by causing the particles of the medium to oscillate up and down or left and right. The tightly packed particles in a solid allow the wave to propagate quickly and efficiently. This is why sound waves can travel faster through solids than through liquids or gases.
In a liquid medium, such as water or oil, transverse waves also cause the particles of the medium to move in an up and down or side to side motion. However, the particles in a liquid are not as tightly packed as those in a solid. As a result, transverse waves in liquids may propagate slower compared to solids.
Transverse waves can also travel through a gaseous medium, such as air. In a gas, the particles are much more spread out compared to solids and liquids. This makes it more difficult for the wave to propagate, resulting in slower wave speed. However, transverse waves can still pass through a gas, causing the particles to move perpendicular to the wave direction.
Understanding how transverse waves behave in different mediums is crucial in various scientific and technological fields. For example, in the field of seismology, the study of earthquakes, scientists analyze how transverse waves travel through different layers of the Earth to gather information about its internal structure. Additionally, engineers and designers consider the behavior of transverse waves in different mediums when developing devices such as ultrasonic cleaners or acoustic instruments.
Transverse Waves in Solids
In physics, waves are disturbances that propagate through a medium, transferring energy from one point to another without the actual transfer of matter. One type of wave is a transverse wave, which is characterized by the particles of the medium oscillating perpendicular to the direction of the wave’s motion.
In solids, transverse waves can propagate through the material in a unique manner. When a transverse wave travels through a solid, the particles of the material oscillate perpendicular to the direction of the wave. This means that while the wave is moving forward, the particles within the solid are moving up and down or side to side.
Properties of transverse waves in solids:
- Amplitude: The maximum displacement of the particles from their equilibrium position.
- Wavelength: The distance between two consecutive peaks or troughs of the wave.
- Frequency: The number of complete oscillations or cycles of the wave per unit of time.
- Propagation speed: The speed at which the wave is traveling through the solid.
Transverse waves in solids can have various applications in different fields. For example, in the field of non-destructive testing, transverse waves are used to detect flaws or defects in solid materials. By analyzing the reflection, transmission, and scattering of transverse waves, engineers and scientists can assess the structural integrity of a solid object.
In conclusion, transverse waves in solids are characterized by the particles of the material oscillating perpendicular to the direction of the wave. These waves have distinct properties such as amplitude, wavelength, frequency, and propagation speed. Understanding the behavior of transverse waves in solids is crucial in various applications, including non-destructive testing.