
Are you studying trigonometry and looking for a way to test your knowledge? Look no further! In this article, we will provide you with a practice test that covers a wide range of trigonometry topics. Whether you’re a student preparing for an exam or simply looking to sharpen your trigonometry skills, this practice test will challenge you and help you identify areas where you may need additional practice.
The practice test consists of a series of multiple-choice questions that cover various trigonometric concepts such as angles, ratios, identities, and equations. Each question is accompanied by four possible answers, and you are to choose the one that you believe is correct. To make it even more convenient for you, we have provided the answers in a PDF format, so you can easily download and print out the test.
By taking this practice test, you will not only gain a better understanding of trigonometry but also get accustomed to the format of actual trigonometry exams. It is important to remember that practice makes perfect, and by regularly testing yourself, you can improve your problem-solving skills and boost your confidence when it comes to tackling trigonometry problems.
So, if you’re ready to put your trigonometry knowledge to the test, download the practice test with answers PDF and get started. Make sure to carefully read each question and take your time to think through the options before selecting your answer. Good luck!
Trigonometry Practice Test with Answers PDF

Trigonometry can be a challenging subject for many students, requiring a strong understanding of angles, triangles, and trigonometric functions. To help students prepare for exams, practice tests are a valuable resource. One such resource is the Trigonometry Practice Test with Answers PDF. This downloadable PDF provides a range of trigonometry problems along with their answers, allowing students to practice their skills and check their work.
The Trigonometry Practice Test with Answers PDF covers various topics within trigonometry, including angles, right triangles, trigonometric functions, and inverse trigonometric functions. The questions in the practice test are designed to test students’ understanding and application of these concepts, providing a comprehensive review of the subject matter.
The Trigonometry Practice Test with Answers PDF includes a mix of multiple-choice questions, problem-solving questions, and word problems. This variety ensures that students are exposed to different question types and can practice applying trigonometry to various real-life scenarios. Additionally, the PDF includes step-by-step answers and explanations for each question, allowing students to understand the reasoning behind each solution.
By using the Trigonometry Practice Test with Answers PDF, students can assess their knowledge and identify areas where they may need additional study or practice. This resource can be used independently for self-study or as a supplement to classroom lessons. Whether preparing for a trigonometry exam or simply looking to strengthen their understanding of the subject, the Trigonometry Practice Test with Answers PDF is a valuable tool for students seeking to improve their skills in trigonometry.
Key phrases:
- Trigonometry Practice Test with Answers PDF
- angles, triangles, and trigonometric functions
- practice tests
- range of trigonometry problems
- check their work
- covers various topics within trigonometry
- multiple-choice questions, problem-solving questions, and word problems
- step-by-step answers and explanations
- assess their knowledge
- identify areas where they may need additional study or practice
Section 2: Basic Trigonometric Functions
In this section, we will review the basic trigonometric functions and their properties. Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles. Understanding the fundamental trigonometric functions is crucial for solving various problems involving angles and distances.
1. Sine: The sine of an angle is defined as the ratio of the length of the side opposite the angle to the length of the hypotenuse. It is represented by the abbreviation “sin” and is often used to find the vertical component of a given angle.
2. Cosine: The cosine of an angle is defined as the ratio of the length of the adjacent side to the length of the hypotenuse. It is represented by the abbreviation “cos” and is often used to find the horizontal component of a given angle.
3. Tangent: The tangent of an angle is defined as the ratio of the length of the side opposite the angle to the length of the side adjacent to the angle. It is represented by the abbreviation “tan” and is often used to find the slope or inclination of a line.
4. Cosecant: The cosecant of an angle is defined as the reciprocal of the sine of the angle. It is represented by the abbreviation “csc” and is often used in trigonometric identities and equations.
5. Secant: The secant of an angle is defined as the reciprocal of the cosine of the angle. It is represented by the abbreviation “sec” and is often used in trigonometric identities and equations.
6. Cotangent: The cotangent of an angle is defined as the reciprocal of the tangent of the angle. It is represented by the abbreviation “cot” and is often used in trigonometric identities and equations.
Remember to always use the appropriate trigonometric function based on the given problem and the known information. Practice applying these basic trigonometric functions to different scenarios to reinforce your understanding and improve your problem-solving skills.
Section 3: Solving Trigonometric Equations
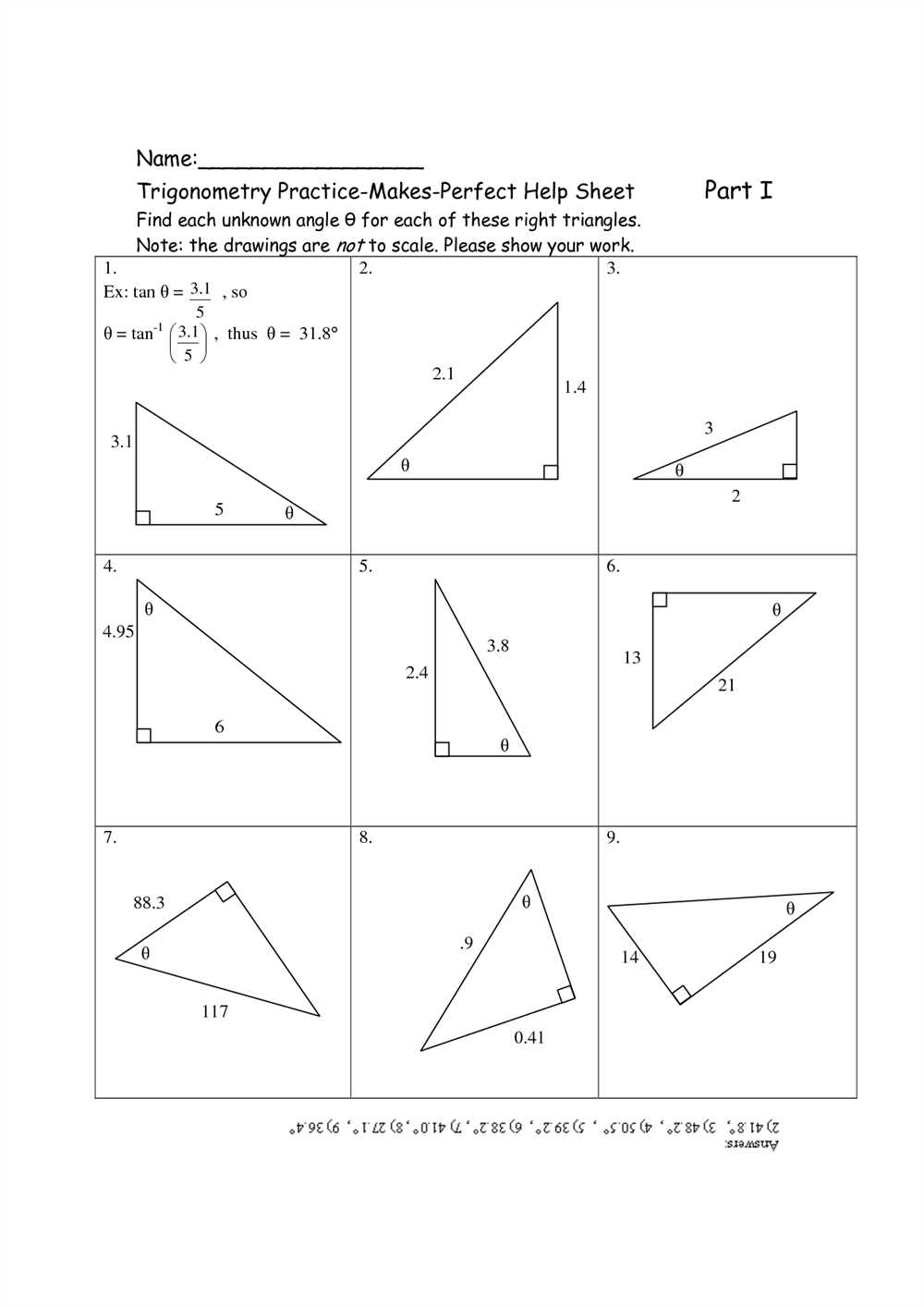
In trigonometry, solving trigonometric equations involves finding the values of the unknown angles or variables that satisfy the given equation. These equations typically involve trigonometric functions such as sine, cosine, and tangent.
To solve trigonometric equations, it is important to understand the properties and identities of trigonometric functions. This knowledge allows us to manipulate the equations and simplify them to find the solutions. The solutions can be expressed in terms of specific angles, or they can be given as general solutions using variables.
When solving trigonometric equations, we often use inverse trigonometric functions to isolate the variable. For example, if we have an equation in the form sin(x) = a, we can use the arcsine function (sin^(-1)(a)) to find the possible values of x.
It is important to note that trigonometric equations can have multiple solutions, as there are often multiple angles that satisfy the equation. These solutions can be periodic, repeating every 360 degrees or 2π radians, depending on the unit of measurement.
Solving trigonometric equations requires a combination of algebraic manipulation, knowledge of trigonometric identities, and an understanding of the properties of trigonometric functions. Practice and familiarity with various types of trigonometric equations can help develop these skills and improve problem-solving abilities in trigonometry.
Section 4: Trigonometric Identities
In trigonometry, identities are mathematical equations that involve trigonometric functions and are true for all values of the variables. They are useful for simplifying expressions, solving equations, and proving other mathematical statements. This section focuses on some of the fundamental trigonometric identities that are frequently used in trigonometry.
1. Pythagorean Identities:
The Pythagorean identities are a set of three formulas that relate the sine, cosine, and tangent functions with the unit circle and right triangles. They are derived from the Pythagorean theorem, which states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
- The first identity is: sin²θ + cos²θ = 1
- The second identity is: 1 + tan²θ = sec²θ
- The third identity is: 1 + cot²θ = csc²θ
2. Even and Odd Function Identities:
Trigonometric functions can be classified as even or odd based on their symmetry properties. Even functions are symmetric about the y-axis, while odd functions are symmetric about the origin. The identities for even and odd functions are:
- cos(-θ) = cos(θ) (cosine is an even function)
- sin(-θ) = -sin(θ) (sine is an odd function)
- tan(-θ) = -tan(θ) (tangent is an odd function)
- cot(-θ) = -cot(θ) (cotangent is an odd function)
- sec(-θ) = sec(θ) (secant is an even function)
- csc(-θ) = -csc(θ) (cosecant is an odd function)
3. Reciprocal Function Identities:
The reciprocal identities involve the reciprocal of trigonometric functions. The identities for reciprocal functions are:
- sinθ = 1/cscθ
- cosθ = 1/secθ
- tanθ = 1/cotθ
- cosecθ = 1/sinθ
- secθ = 1/cosθ
- cotθ = 1/tanθ
These identities can be used to simplify trigonometric expressions and solve trigonometric equations. Mastery of these identities is crucial for further understanding and application of trigonometry.
Section 5: Trigonometric Ratios
In trigonometry, certain ratios are used to relate the angles of a right triangle to the lengths of its sides. These ratios are known as trigonometric ratios and are widely used in various applications.
Sine Ratio: The sine ratio, denoted as sin(theta), is defined as the ratio of the length of the side opposite the angle theta to the hypotenuse of the triangle. It can be calculated as:
- Sine(theta) = (Opposite Side) / (Hypotenuse)
Cosine Ratio: The cosine ratio, denoted as cos(theta), is defined as the ratio of the length of the side adjacent to the angle theta to the hypotenuse of the triangle. It can be calculated as:
- Cosine(theta) = (Adjacent Side) / (Hypotenuse)
Tangent Ratio: The tangent ratio, denoted as tan(theta), is defined as the ratio of the length of the side opposite the angle theta to the side adjacent to the angle theta. It can be calculated as:
- Tangent(theta) = (Opposite Side) / (Adjacent Side)
These trigonometric ratios are fundamental in trigonometry and are used to solve various problems involving right triangles. They are also used in fields such as physics, engineering, and navigation.
Section 6: Inverse Trigonometric Functions
The inverse trigonometric functions are used to find angles in right triangles when the lengths of the sides are known. These functions are the inverse of the trigonometric functions and allow us to find the angle whose trigonometric ratio is given. The inverse trigonometric functions are useful in many real-life applications, such as navigation, engineering, and physics.
The inverse trigonometric functions include arcsin, arccos, and arctan, which are denoted as sin⁻¹, cos⁻¹, and tan⁻¹, respectively. These functions give us the angle whose sine, cosine, or tangent is a given value. For example, sin⁻¹(x) will give us the angle whose sine is x.
The range of the inverse trigonometric functions is typically restricted to certain values in order to make them single-valued. The range of the arcsin function, for example, is -π/2 to π/2, whereas the range of the arccos function is 0 to π. The range of the arctan function is -π/2 to π/2.
- The arcsin function is used to find the angle whose sine is known.
- The arccos function is used to find the angle whose cosine is known.
- The arctan function is used to find the angle whose tangent is known.
It is important to note that the inverse trigonometric functions are only defined for certain values of their arguments. For example, the arcsin function is only defined for values between -1 and 1. If the input to an inverse trigonometric function is outside of its defined range, an error will occur.
To solve problems involving inverse trigonometric functions, it is important to understand the relationships between angles and trigonometric ratios. It is also helpful to be familiar with the unit circle, as it can be used to visualize these relationships.
Section 7: Applications of Trigonometry

In this section, we will explore various applications of trigonometry and how it can be used to solve real-world problems. Trigonometry is not just a theoretical concept; it has practical applications in fields such as architecture, engineering, physics, and navigation.
1. Architecture: Trigonometry is used in architecture to calculate the measurements and angles of various structures. Architects use trigonometric principles to design buildings with precise measurements and to ensure that the structure is stable and well-balanced.
2. Engineering: Trigonometry plays a crucial role in engineering, especially in fields such as civil engineering and mechanical engineering. Engineers use trigonometry to calculate forces, angles, and distances in order to design and construct structures and machines. Trigonometric equations are also used in electrical engineering to analyze and solve complex circuits.
3. Physics: Trigonometry is essential in physics to describe and analyze the motion and forces of objects. It is used to calculate angles, distances, velocities, and accelerations in various physical phenomena. Trigonometry is particularly useful in studying projectile motion, waves, and vibrations.
4. Navigation: Trigonometry is used extensively in navigation, whether it is for land, sea, or air. It helps determine the position, distance, and direction of objects or landmarks. Trigonometric principles like the laws of sines and cosines are applied in navigation to solve problems related to aircraft navigation, GPS systems, and ship navigation.
Overall, trigonometry is a powerful tool that enables us to understand and solve a wide range of real-world problems. Its applications are numerous and diverse, making it an essential branch of mathematics in various fields of study and professions.