Understanding equations and inequalities is a fundamental skill in mathematics. In this unit, we will explore various types of equations and inequalities and provide answers and solutions to help you master these concepts. From simple one-variable equations to complex systems of equations, we will cover it all. By the end of this unit, you will have a solid foundation in solving equations and inequalities and be able to apply these skills to real-life situations.
Equations are mathematical expressions that show the relationship between two quantities. They often involve unknown variables, and the goal is to find the value of the variable that satisfies the equation. Inequalities, on the other hand, compare the values of two quantities and indicate whether one is greater than, less than, or equal to the other. Whether you are solving an equation or an inequality, the process involves applying various operations to both sides of the expression to isolate the variable.
In this unit, we will start with simple linear equations and inequalities and gradually progress to more complex ones, including quadratic equations and absolute value inequalities. We will provide step-by-step answers to guide you through the solving process and explain the reasoning behind each step. Additionally, we will discuss strategies for solving word problems that involve equations and inequalities, as these are often encountered in real-life scenarios.
By practicing the exercises in this unit and referring to the answers provided, you will develop a strong understanding of equations and inequalities and gain confidence in solving them. These skills are not only important for success in mathematics but also have practical applications in fields such as science, engineering, and finance. So, let’s dive in and explore the world of equations and inequalities!
Unit 1 Equations and Inequalities Answers

Unit 1 of the Equations and Inequalities course covers the fundamental concepts and techniques related to solving equations and inequalities. In this unit, students will learn how to solve linear equations, quadratic equations, and systems of equations. They will also explore the concepts of absolute value and inequalities. This unit serves as the foundation for further exploration of algebraic concepts.
One of the key topics covered in this unit is solving linear equations. Students will learn how to isolate variables and use inverse operations to find the solution. They will practice solving equations with one variable as well as equations with multiple variables. The process of solving equations involves simplifying expressions, distributing, and combining like terms.
Another important topic covered in this unit is solving quadratic equations. Students will learn different methods for solving quadratic equations such as factoring, completing the square, and using the quadratic formula. They will also explore the relationship between the solutions of a quadratic equation and the graph of a parabola.
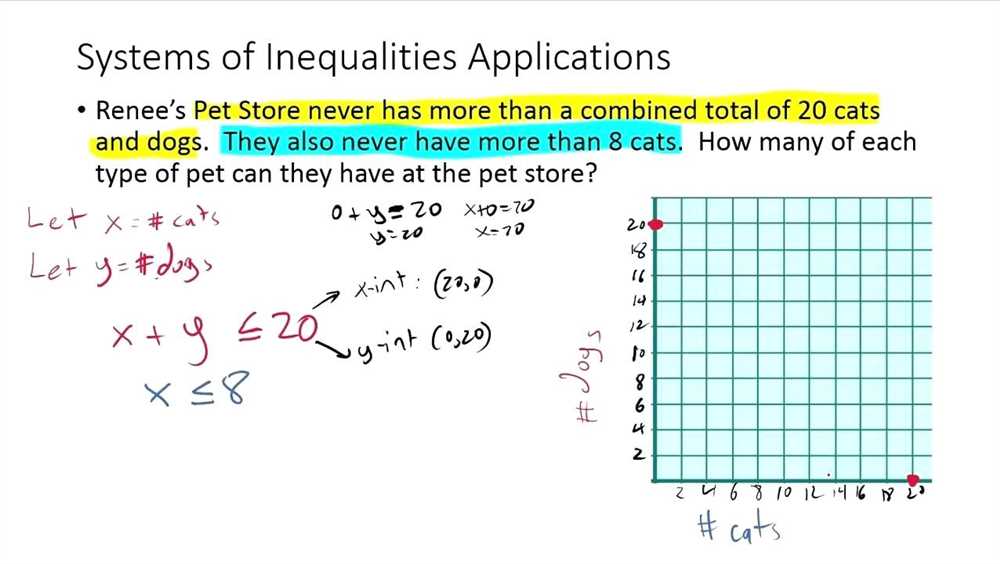
In addition to solving equations, students will also learn how to solve systems of equations. They will learn different methods for solving systems of linear equations, such as graphing, substitution, and elimination. They will practice using these methods to find the solution to systems of equations in both real-world and mathematical contexts.
Overall, this unit provides students with the foundational skills and techniques necessary to solve a variety of equations and inequalities. It prepares them for further study in algebra and lays the groundwork for future problem-solving in math and other disciplines.
The Basics of Equations and Inequalities
An equation is a mathematical statement that shows the equality between two expressions. It consists of two sides, the left-hand side (LHS) and the right-hand side (RHS), connected by an equal sign (=). The purpose of an equation is to find the value(s) of the unknown variable(s) that make the equation true.
When solving an equation, the goal is to isolate the variable on one side of the equation. This can be done by performing the same operation on both sides of the equation to maintain equality. The basic operations used in solving equations are addition, subtraction, multiplication, and division.
- For example, in the equation 2x + 5 = 13, the variable x represents an unknown value. To isolate x, we can subtract 5 from both sides of the equation: 2x = 8. Then, if we divide both sides by 2, we find that x = 4. Therefore, 4 is the solution to the equation.
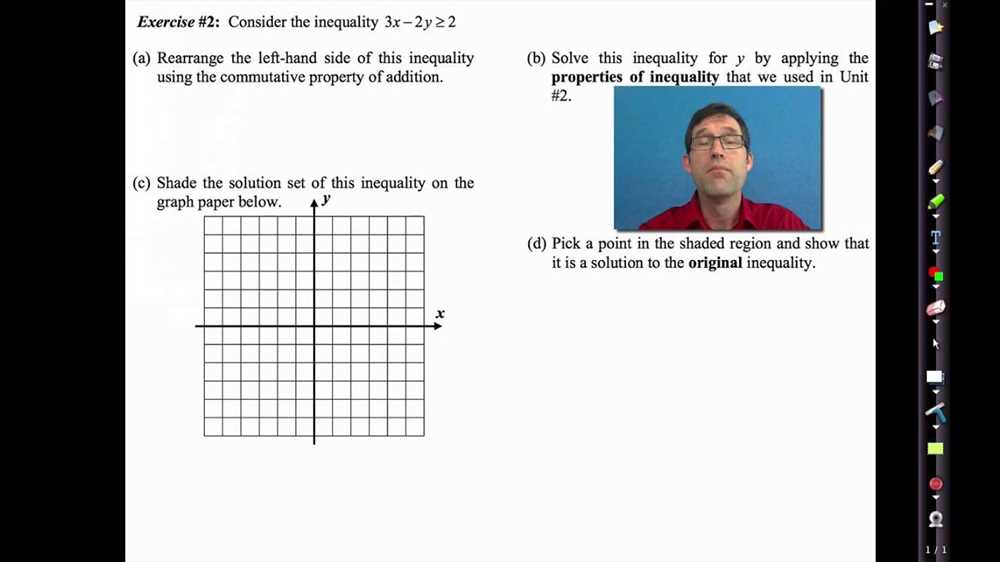
An inequality, on the other hand, shows the relationship between two expressions, where one expression is less than, greater than, or equal to the other. Inequalities are represented by symbols such as < (less than), > (greater than), <= (less than or equal to), and >= (greater than or equal to).
Solving an inequality involves finding all possible values of the variable that satisfy the given inequality. Similar to solving equations, operations such as addition, subtraction, multiplication, and division can be used to manipulate the inequality and isolate the variable.
- For example, in the inequality 3x – 2 < 10, we want to find the values of x that make the expression 3x - 2 less than 10. By adding 2 to both sides of the inequality, we get 3x < 12. Dividing both sides by 3 gives us x < 4. Therefore, all values of x less than 4 satisfy the inequality.
Equations and inequalities are fundamental concepts in mathematics and are used in various applications, such as solving real-life problems, modeling relationships, and proving mathematical theorems.
Solving One-Step Equations
The process of solving one-step equations involves finding the value of the variable that satisfies the given equation. This is done by performing the same operation on both sides of the equation in order to isolate the variable. The key is to keep the equation balanced by applying the inverse operation. Let’s take a look at an example:
Example: Solve the equation 2x + 5 = 15
To isolate the variable x, we need to get rid of the number 5 on the left side of the equation. Since 5 is added to 2x, we can subtract 5 from both sides to maintain balance:
2x + 5 – 5 = 15 – 5
After simplifying, we have:
2x = 10
Next, we want to isolate the variable x on one side of the equation. Since 2 is multiplied by x, we can divide both sides of the equation by 2:
(2x) / 2 = 10 / 2
Therefore, the solution to the equation is:
x = 5
By applying the correct operations, we were able to find the value of x that makes the equation true. It is important to remember to perform the same operation on both sides of the equation to maintain balance and solve for the variable.
Solving Two-Step Equations
When solving two-step equations, it is important to follow a specific order of operations to find the solution. The goal is to isolate the variable and determine its value.
To begin, it is helpful to first identify the operations being performed on the variable. This may include addition, subtraction, multiplication, or division. The order in which these operations are applied is crucial to solving the equation correctly.
Once the operations have been identified, the next step is to perform the inverse operation on each side of the equation to isolate the variable. For example, if the variable is being multiplied by a number, the inverse operation would be division. Applying this inverse operation to both sides of the equation will cancel out the multiplication and leave the variable alone on one side of the equation.
If there are additional operations after the variable has been isolated, such as addition or subtraction, the same process should be followed to eliminate those operations and isolate the variable completely. This may involve further inverse operations and simplification. Once the variable has been fully isolated, its value can be determined and the equation is solved.
It is important to note that these steps should be followed systematically and meticulously to ensure an accurate solution. Careful attention to detail and understanding of the order of operations are key in successfully solving two-step equations.
Solving Multi-Step Equations
Solving multi-step equations involves using different operations to isolate the variable and find its value. These equations often require several steps and can involve various mathematical operations such as addition, subtraction, multiplication, and division.
The first step in solving a multi-step equation is to simplify both sides of the equation by combining like terms and using the distributive property if necessary. This allows us to eliminate any unnecessary variables or constants and focus on isolating the variable we are trying to solve for.
After simplifying the equation, the next step is to get rid of any constants or coefficients that are attached to the variable. This is done by performing inverse operations, such as adding or subtracting, to eliminate these terms.
Once the variable is isolated on one side of the equation, the final step is to solve for its value. This can be done by using the properties of equality and performing any necessary operations to find the value of the variable.
It is important to check the solution by substituting the value of the variable back into the original equation to ensure that it satisfies the equation. If the solution is correct, it will make both sides of the equation equal.
In conclusion, solving multi-step equations requires a systematic approach and the use of various mathematical operations. By simplifying the equation, eliminating constants and coefficients, and solving for the variable, we can find the value that satisfies the equation.
Equations with Variables on Both Sides
In algebra, equations with variables on both sides are a common type of equation that students learn to solve. These equations have the variable (typically represented by the letter “x”) on both sides of the equation, rather than just on one side. This can make solving the equation more challenging, as the goal is to isolate the variable on one side of the equation.
When solving equations with variables on both sides, the main goal is to get all the variable terms on one side and the constant terms on the other side. This involves using the properties of equality to simplify the equation. The steps to solve these equations typically include combining like terms, using the distributive property, and performing inverse operations such as addition, subtraction, multiplication, and division.
For example, let’s consider the equation 3x + 5 = 2x – 3. In order to solve this equation and isolate the variable, we would start by combining like terms. By subtracting 2x from both sides and subtracting 5 from both sides, we can simplify the equation to x = -8. This means that -8 is the value of x that makes the equation true.
It’s important to note that when solving equations with variables on both sides, it’s possible for the equation to have no solution or an infinite number of solutions. This can occur when the variable terms cancel out and the equation simplifies to a false statement (such as 0 = 1) or when the variable terms cancel out and the equation simplifies to an identity (such as 0 = 0).
In summary, solving equations with variables on both sides requires using the properties of equality and performing inverse operations to isolate the variable. These equations can be challenging to solve, but with practice and a clear understanding of the steps involved, students can become proficient in solving these types of equations.
Solving Equations with Fractional Coefficients
When solving equations with fractional coefficients, it is important to handle them carefully to ensure accurate solutions. The process involves getting rid of the fractions by multiplying both sides of the equation by the least common denominator (LCD). By doing so, the equation is transformed into one with whole number coefficients.
To illustrate the process, let’s consider an example equation: 3/4x + 1/2 = 5/8. The LCD is 8, so we multiply both sides of the equation by 8 to eliminate the fractions. This gives us 8 * (3/4x) + 8 * (1/2) = 8 * (5/8), which simplifies to 6x + 4 = 5.
Next, we isolate the variable by subtracting 4 from both sides of the equation: 6x = 5 – 4 = 1. Finally, dividing both sides by 6 gives us the solution: x = 1/6.
It is important to note that when solving equations with fractional coefficients, it is crucial to watch out for potential division by zero. If the LCD includes a zero in it, the equation becomes undefined and there is no solution. Additionally, it is always a good practice to check the solution by substituting it back into the original equation and verifying that it holds true.
Solving Equations with Grouping Symbols

When solving equations that involve grouping symbols, such as parentheses or brackets, it is important to follow the correct order of operations. This means that you must simplify any expressions inside the grouping symbols before proceeding with the rest of the equation.
One common strategy for solving equations with grouping symbols is to use the distributive property. This property allows you to eliminate the grouping symbols by multiplying both the term inside the parentheses or brackets and the term outside of the parentheses or brackets. This can help simplify the equation and make it easier to solve.
For example, let’s consider the equation 3(x + 4) = 15. To solve this equation, we need to eliminate the parentheses by distributing the 3 to both the x and the 4. This gives us 3x + 12 = 15. From here, we can isolate the variable by subtracting 12 from both sides of the equation to get 3x = 3. Finally, we can solve for x by dividing both sides of the equation by 3, giving us x = 1.
It is important to carefully apply the distributive property when solving equations with grouping symbols. Keep in mind that you must multiply every term inside the grouping symbols by the term outside the grouping symbols. Additionally, be sure to combine like terms and simplify the equation as much as possible before proceeding with solving for the variable. By following these steps, you can effectively solve equations with grouping symbols.