
Geometry is a branch of mathematics that deals with the properties and relationships of shapes, sizes, angles, and dimensions. It plays a fundamental role in our understanding of the physical world and is used in a wide range of fields, from architecture to engineering.
In Unit 1 of a geometry course, students are introduced to basic concepts and principles of geometry. This unit often includes topics such as points, lines, angles, triangles, and circles. Students learn how to identify and classify different types of angles and triangles, as well as how to calculate their measures and properties.
The Unit 1 geometry test is designed to assess students’ knowledge and understanding of these concepts. It typically includes a variety of questions, such as multiple-choice, short-answer, and problem-solving questions. The answer key provides students with the correct answers to these questions, allowing them to check their work and understand where they made any mistakes.
Having access to the Unit 1 geometry test answer key is beneficial for both students and teachers. It helps students assess their understanding of the topics covered in the unit and identify areas that require further study. For teachers, the answer key serves as a valuable tool for grading and providing feedback to students, as well as for identifying any common misconceptions or difficulties that students may have encountered during the unit.
Unit 1 Geometry Test Answer Key

In this article, we will provide the answer key for the Unit 1 Geometry test. This test covers various topics related to geometry, such as angles, lines, polygons, and circles. The answer key will help students check their answers and understand the concepts better.
Here are the answers to the questions in the Unit 1 Geometry test:
- Question 1: What is the sum of the interior angles of a triangle?
- Answer: The sum of the interior angles of a triangle is always 180 degrees.
- Question 2: What is the relationship between vertical angles?
- Answer: Vertical angles are always congruent, which means that they have the same measure.
- Question 3: What is the measure of a straight angle?
- Answer: A straight angle measures 180 degrees.
- Question 4: How many degrees are in a circle?
- Answer: There are 360 degrees in a circle.
- Question 5: What is the measure of each interior angle of a regular hexagon?
- Answer: Each interior angle of a regular hexagon measures 120 degrees.
These are just a few examples of the questions and answers you may find in the Unit 1 Geometry test. It is important to review and understand these concepts to succeed in future geometry exams.
Remember to always show your work and provide clear explanations for your answers. Geometry is a visual subject, so diagrams and sketches can also be helpful in illustrating your solutions.
Overview of the Unit 1 Geometry Test

The Unit 1 Geometry Test covers various topics related to geometry, including angles, lines, polygons, and measurements. The test is designed to assess students’ understanding of geometric concepts and their ability to apply them in problem-solving situations.
The test consists of multiple-choice questions, short-answer questions, and problem-solving tasks. It requires students to demonstrate their knowledge of key geometric definitions, properties, and formulas, as well as their ability to analyze and explain geometric relationships and solve geometric problems.
The test includes questions that assess students’ understanding of the basic geometric concepts, such as types of angles (acute, obtuse, right), types of lines (parallel, perpendicular), and properties of polygons (number of sides, angles, symmetry). It also includes questions that require students to apply these concepts to real-world situations and solve problems using geometric reasoning and calculations.
It is important for students to review and study the key concepts and definitions covered in the unit, as well as practice solving a variety of geometric problems, in order to prepare for the test. Effective preparation will involve reviewing class notes, completing assigned homework, and seeking clarification on any areas of confusion.
The Unit 1 Geometry Test provides an opportunity for students to demonstrate their mastery of the foundational concepts in geometry and their ability to think critically and analytically. By thoroughly preparing for the test, students can ensure that they are well-equipped to tackle the challenges and excel in their understanding of geometry.
Understanding Basic Geometric Concepts

In geometry, understanding basic geometric concepts is essential for solving problems and mastering more advanced concepts. These concepts lay the foundation for studying shapes, spatial relationships, and measurements. By having a solid grasp of basic geometric concepts, individuals can analyze and solve problems involving lines, angles, shapes, and spatial arrangements.
Points: One of the fundamental concepts in geometry is the point. A point represents a specific location in space and is typically depicted as a dot. It has no dimension, meaning it has no length, width, or height. Points are often labeled with uppercase letters to distinguish one from another.
Lines: Lines are made up of an infinite number of points and extend infinitely in both directions. They have no width or thickness. Lines can be straight or curved. Straight lines do not have any bends or curves, while curved lines have varying degrees of curvature. Lines are commonly labeled with lowercase letters.
Angles: Angles are formed when two lines or line segments intersect. They are measured in degrees. Angles can be classified as acute (less than 90 degrees), right (exactly 90 degrees), obtuse (greater than 90 degrees but less than 180 degrees), or straight (exactly 180 degrees). Understanding angles is crucial when solving geometric problems involving shapes, lines, and measurements.
Shapes: Shapes are defined as enclosed spaces or boundaries created by lines or curves. They can be two-dimensional (flat) or three-dimensional (solid). Common two-dimensional shapes include triangles, quadrilaterals, and circles. Three-dimensional shapes include cubes, spheres, and cylinders. Understanding different shapes and their properties is necessary for identifying, classifying, and analyzing geometric figures.
Measurements: Geometry involves the measurement of various geometrical quantities, such as length, area, volume, and angles. Measuring these quantities accurately is essential for solving geometry problems and making calculations. Units of measurement, such as inches, centimeters, and degrees, are used to quantify geometrical attributes.
In conclusion, understanding basic geometric concepts is crucial for further studies in geometry. By grasping the concepts of points, lines, angles, shapes, and measurements, individuals can navigate and solve geometric problems effectively and efficiently.
Following the study of lines, angles, and triangles in Unit 1 of geometry, students will gain a solid understanding of these fundamental geometric concepts. Lines are one-dimensional and extend infinitely in both directions. They can be classified into different types based on their properties, such as parallel lines, perpendicular lines, and intersecting lines. Parallel lines never intersect and are always equidistant from each other, while perpendicular lines intersect at a 90-degree angle. Intersecting lines cross each other at any angle other than 90 degrees.
Angles, on the other hand, are formed by two rays that share a common endpoint called the vertex. There are different types of angles, including acute angles (less than 90 degrees), right angles (exactly 90 degrees), obtuse angles (greater than 90 degrees but less than 180 degrees), and straight angles (exactly 180 degrees). Students will learn how to measure angles using a protractor and how to classify them based on their measurements.
Triangles, as the name suggests, are three-sided polygons. They are classified into different types based on the lengths of their sides and the measures of their interior angles. The three main types of triangles are equilateral triangles (where all sides and angles are congruent), isosceles triangles (where two sides and two angles are congruent), and scalene triangles (where no sides or angles are congruent).
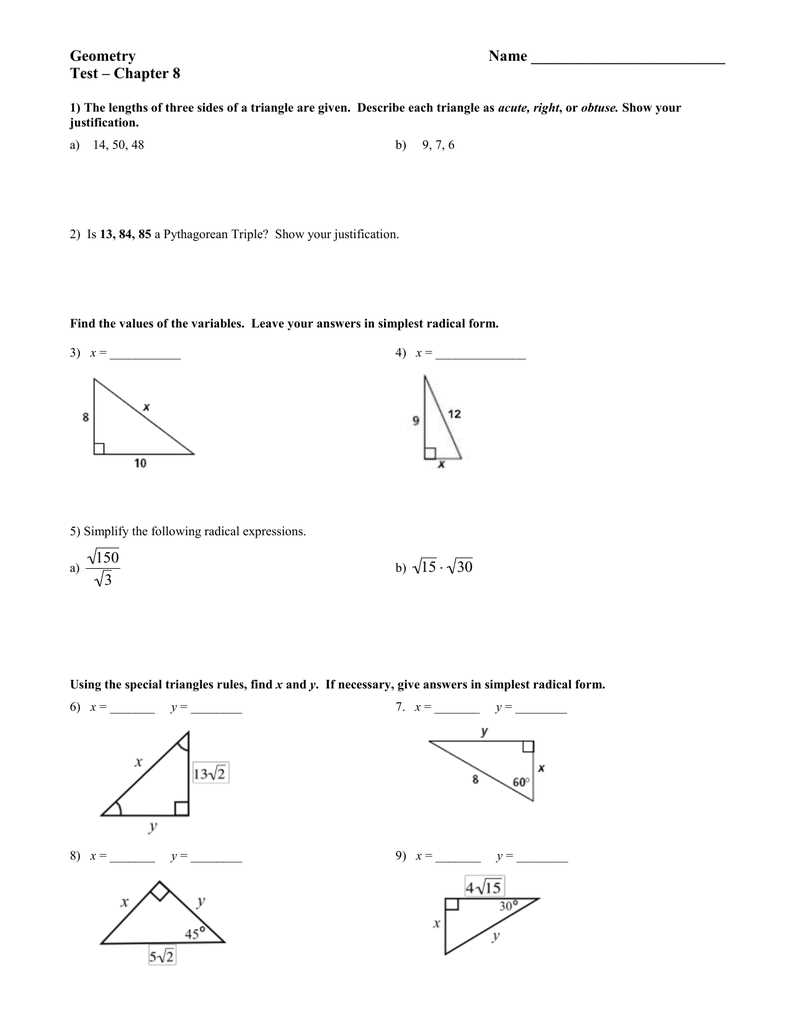
During the unit, students will also learn about the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. They will apply this theorem to solve problems involving right triangles.
Overall, the study of lines, angles, and triangles is crucial in building a strong foundation in geometry. These concepts form the basis for more advanced topics in geometry and are used in various real-life applications, such as architecture, engineering, and computer graphics.
Solving Problems with Quadrilaterals and Polygons
Quadrilaterals and polygons are geometric shapes that play a significant role in solving various mathematical problems. By understanding their properties and relationships, we can successfully solve problems related to their angles, sides, and diagonals.
One key concept when solving problems with quadrilaterals and polygons is identifying their specific properties. For example, a quadrilateral is a polygon with four sides. However, it can have different properties depending on its shape, such as being a rectangle, square, rhombus, or trapezoid. Recognizing these specific properties allows us to apply the appropriate formulas and theorems to solve problems.
When dealing with quadrilaterals and polygons, it’s crucial to understand the angles they contain. For instance, the sum of the interior angles of a quadrilateral is always 360 degrees. This knowledge helps us determine missing angles and solve problems involving angle measures. Additionally, knowing the properties of special quadrilaterals, such as rectangles having four right angles or parallelograms having opposite angles that are congruent, allows for more specific problem-solving.
Furthermore, solving problems with quadrilaterals and polygons often involves their sides and diagonals. By utilizing formulas and theorems related to these geometric figures, we can find missing side lengths or calculate the lengths of diagonals. For example, the Pythagorean theorem can be used to find the length of a diagonal in a rectangle or a square.
In conclusion, solving problems with quadrilaterals and polygons requires a solid understanding of their properties, angles, sides, and diagonals. By utilizing the appropriate formulas, theorems, and knowledge of specific shapes, we can successfully solve various mathematical problems related to these geometric figures.
Applying Geometric Formulas and Equations
In geometry, formulas and equations are used to calculate various measurements and solve problems related to geometric shapes. These tools enable us to find values such as area, perimeter, volume, and angles, which are essential in understanding and analyzing different geometric figures.
Triangles: One of the most common shapes in geometry is a triangle. To find the area of a triangle, we can use the formula A = 1/2 * base * height. The perimeter of a triangle can be calculated by adding the lengths of all its sides. Additionally, the Pythagorean theorem allows us to find the length of an unknown side of a right triangle by using the equation a^2 + b^2 = c^2, where a and b are the two shorter sides, and c is the hypotenuse.
Circles: Circles are another important geometric shape. The formula for the circumference of a circle is C = 2πr, where r is the radius. The area of a circle, on the other hand, is calculated using the formula A = πr^2. These formulas help us calculate the distance around a circle and the amount of space it occupies, respectively.
Rectangles and squares: To find the area of a rectangle or a square, we can use the formula A = length * width. The perimeter of a rectangle or square is calculated by adding the lengths of all its sides. For a square, all four sides are equal in length.
Volume and surface area: Formulas are also used to calculate the volume and surface area of three-dimensional shapes such as cubes, cylinders, and cones. These calculations involve finding the amount of space inside a shape and the total area of its surfaces, respectively. The formulas for these measurements differ based on the specific shape being considered.
By applying geometric formulas and equations, we can accurately determine the measurements and properties of various shapes. These calculations play a crucial role in real-world applications, including architecture, engineering, and design, as well as in solving mathematical problems and puzzles.
Analyzing Coordinate Geometry
The study of coordinate geometry involves analyzing geometric shapes and figures using a coordinate plane. The coordinate plane is a two-dimensional space that uses a pair of numbers, called coordinates, to pinpoint the location of points. It consists of two perpendicular number lines known as the x-axis and y-axis. The x-axis represents the horizontal values, while the y-axis represents the vertical values. By plotting points on the coordinate plane, we can analyze and determine various properties of geometric objects such as lines, circles, and polygons.
One of the fundamental concepts in coordinate geometry is the distance formula. This formula allows us to calculate the distance between two points in a coordinate plane. The distance between two points (x1, y1) and (x2, y2) can be found using the formula:
√[(x2 – x1)² + (y2 – y1)²]
Another important concept is the slope of a line. The slope represents the steepness of a line and can be found using the formula:
(y2 – y1)/(x2 – x1)
Coordinate geometry also involves analyzing the properties of lines, such as determining parallel and perpendicular lines. Two lines are parallel if they have the same slope, while two lines are perpendicular if the product of their slopes is -1. These concepts are essential in solving coordinate geometry problems and understanding the relationships between various geometric elements.
Overall, coordinate geometry provides a powerful tool for analyzing and understanding geometric shapes and figures. By utilizing the coordinate plane and various formulas and concepts, we can explore the properties and relationships of these geometric objects. This knowledge is essential not only in mathematics but also in fields such as physics, engineering, and computer science.