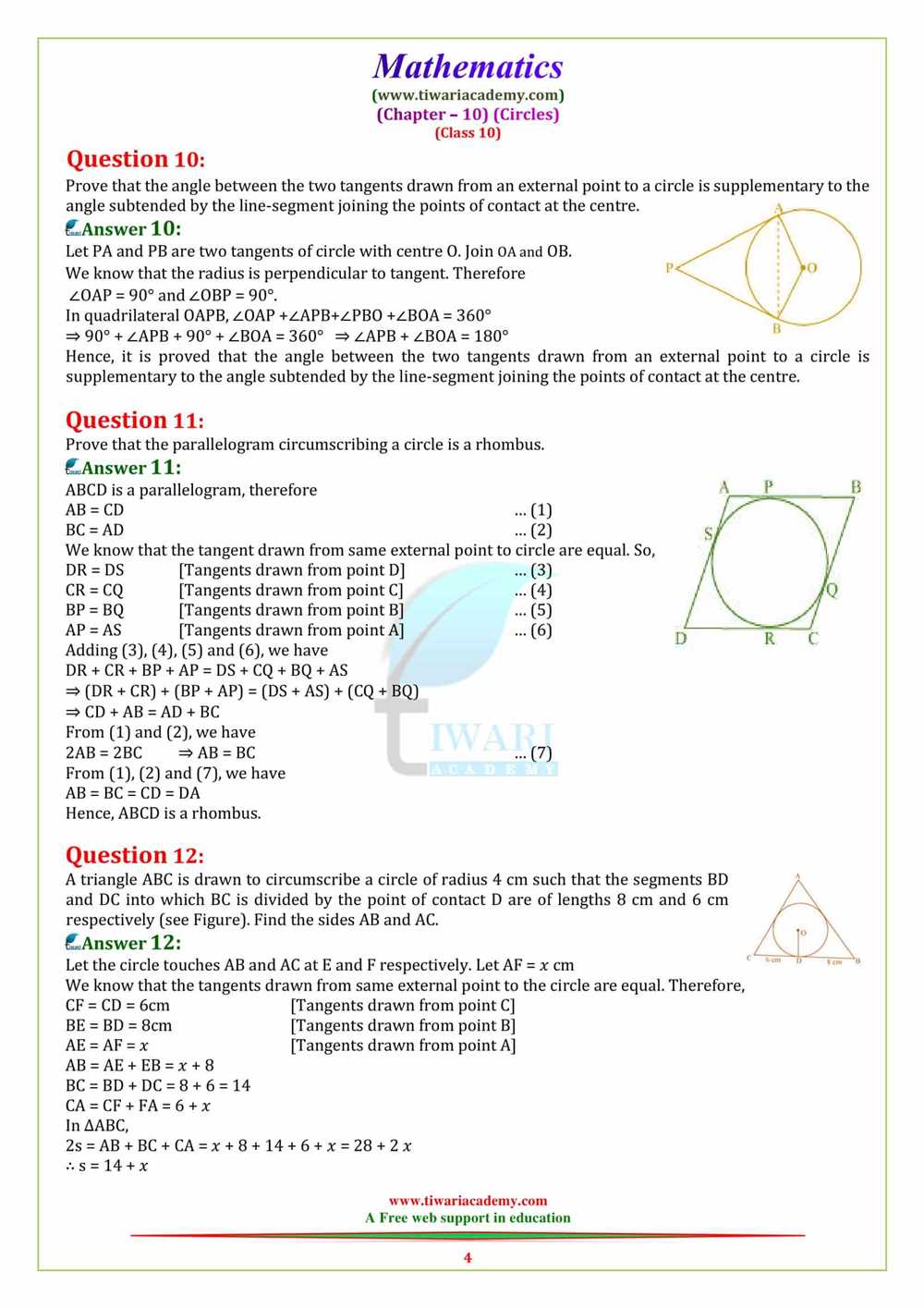
In this study guide, we will be covering the topic of circles. Circles are an important geometric shape that have many unique properties and can be found in various aspects of everyday life. By understanding the concepts and equations associated with circles, you will be able to solve a variety of problems related to their circumference, area, and other related measurements.
One of the fundamental concepts in studying circles is understanding their basic components. Circles are defined by a curved line called a circumference, which is made up of an infinite number of points equidistant from a central point called the center of the circle. The distance from the center to any point on the circumference is called the radius, and the distance across the circle passing through the center is called the diameter.
Circles have many unique properties that make them fascinating to study. For example, the circumference of a circle is directly related to its diameter through a mathematical constant called pi (π). The value of pi is approximately equal to 3.14159 and is used in various equations involving circles. Additionally, circles have a unique symmetry property where any line passing through the center will divide the circle into two congruent halves.
Understanding these basic concepts and properties of circles will be essential for solving problems on the upcoming Unit 10 test. Make sure to review the formulas for finding the circumference and area of a circle, as well as any special relationships between arcs, angles, and chords within circles. With a solid understanding of these concepts, you will be ready to tackle any circle-related question that comes your way!
Prior Knowledge

Prior knowledge plays a crucial role in understanding the concepts of circles. Students should have a solid understanding of basic geometric terms and concepts, such as points, lines, and angles. They should be familiar with the properties of triangles and quadrilaterals, as well as the concepts of perimeter and area.
It is also important for students to have a good grasp of algebraic concepts, such as solving equations and working with variables. This will help them when solving problems involving circle equations, finding the center and radius of a circle, and determining the distance between two points.
- Geometry: Students should be familiar with the properties of lines, angles, triangles, and quadrilaterals.
- Algebra: Students should have a solid understanding of solving equations and working with variables.
- Measurement: Students should be comfortable calculating perimeter and area.
Having a strong foundation in these areas will enable students to better understand and apply the concepts of circles, including calculating the circumference and area of a circle, finding the length of an arc, and solving problems involving tangents and secants.
It is important for students to review and reinforce their prior knowledge before delving into the specific concepts and formulas related to circles. They can do this by reviewing their notes, practicing problems from previous lessons, and using online resources or textbooks for additional practice.
Understanding circle properties
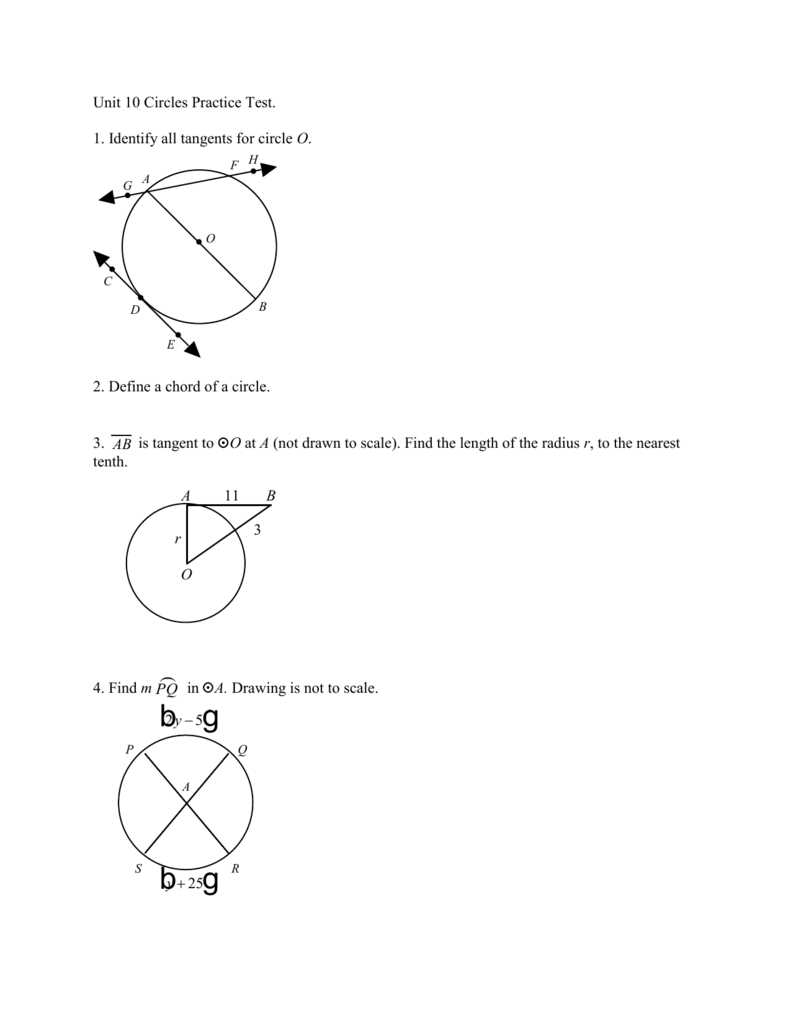
Circles are a fundamental shape in geometry, and understanding their properties is crucial for solving problems involving them. One key property of circles is their radius, which is the distance from the center of the circle to any point on its circumference. Another important property is the diameter, which is the distance across the circle passing through the center. The radius and diameter are related, as the diameter is always twice the length of the radius.
Another key concept when studying circles is the circumference, which is the distance around the circle. The formula for finding the circumference of a circle is C = 2πr, where r is the radius. This formula allows us to calculate the length of a circle given its radius. In addition to the circumference, circles also have an area. The formula for finding the area of a circle is A = πr^2, where r is the radius. This formula can be used to find the area of a circle given its radius.
When working with circles, it is important to understand the relationships between different parts of the circle. For example, the radius, diameter, and circumference are all related, as mentioned earlier. Additionally, if two circles have the same radius, they are congruent, meaning they have the same size and shape. Circles can also be inscribed or circumscribed within other shapes, such as triangles or rectangles. These relationships can be used to solve various geometry problems involving circles.
Understanding the properties of circles is essential when solving geometry problems. Whether calculating the circumference or area, or analyzing the relationships between different parts of the circle, knowing the key concepts and formulas related to circles can help in solving problems efficiently and accurately.
Reviewing Formulas for Circumference and Area
In this study guide, we will review the formulas for finding the circumference and area of a circle. These formulas are essential in solving problems related to circles and can be used to calculate their measurements.
The circumference of a circle is the distance around its outer boundary. It is calculated using the formula C = 2πr, where C represents the circumference and r represents the radius of the circle. To find the circumference, we multiply the radius by 2 and then multiply the result by π (pi), which is approximately 3.14159.
The area of a circle is the measure of the region enclosed by its boundary. It is calculated using the formula A = πr^2, where A represents the area and r represents the radius of the circle. To find the area, we square the radius and then multiply the result by π.
To solve problems involving circles, it is important to remember these formulas and to substitute the given values into the equations correctly. By knowing how to calculate the circumference and area of a circle, we can accurately determine measurements such as the length of a circular track or the amount of space inside a circular garden.
Additionally, it is crucial to understand the concept of π (pi) and its approximate value. Pi is an irrational number that represents the ratio of a circle’s circumference to its diameter. It is a mathematical constant that is often rounded to 3.14 for practical calculations.
By reviewing these formulas and understanding their application, you will be well-prepared to solve problems related to circles and confidently answer questions on the Unit 10 test.
Identifying Circle Parts
A circle is a geometric figure that consists of all the points in a plane that are equidistant from a fixed point called the center. In order to properly understand and analyze circles, it is important to be able to identify and define its different parts.
Center: The center is the fixed point in the plane that is equidistant from all the points on the circumference of the circle. It is often represented by the letter “O”.
Radius: The radius is the segment that connects the center of the circle to any point on its circumference. It is denoted by the letter “r”. The radius determines the size of the circle.
Diameter: The diameter is a line segment that passes through the center of the circle and connects two points on its circumference. It is twice the length of the radius and is denoted by the letter “d”. The diameter is the longest chord of the circle.
Circumference: The circumference is the distance around the circle. It is the perimeter of the circle and is denoted by the letter “C”. The formula to calculate the circumference is C = 2πr or C = πd, where π is a mathematical constant approximately equal to 3.14159.
Arc: An arc is a portion of the circumference of a circle. It is measured in degrees and is denoted by the symbol “∠”. Arcs can be major arcs, minor arcs, or semicircles depending on their size.
Sector: A sector is a portion of the interior of a circle bounded by two radii and an arc. It is similar to a slice of pie. The measure of a sector is given in degrees and can be used to calculate the area and perimeter of the sector.
By familiarizing yourself with these different parts of a circle, you will be better able to solve problems and understand the properties and relationships of circles in geometry.
Defining key terms
In this study guide, we will be discussing key terms related to circles and their properties. It is important to have a clear understanding of these terms in order to solve problems involving circles and to effectively communicate mathematical ideas.
One term that is essential to understand is the radius of a circle. The radius is the distance from the center of the circle to any point on its circumference. It is denoted by the letter “r” and is typically a positive number. The radius is an important measurement when calculating other properties of a circle, such as its circumference and area.
Another key term related to circles is the diameter. The diameter is a line segment that passes through the center of the circle and has endpoints on the circumference. It is twice the length of the radius and is denoted by the letter “d”. The diameter is also an important measurement when calculating other properties of a circle, such as its circumference and area.
- Circumference: The circumference of a circle is the distance around its outer edge. It is calculated using the formula C = 2πr or C = πd, where π is a mathematical constant approximately equal to 3.14159.
- Area: The area of a circle is the measure of the region enclosed by the circle. It is calculated using the formula A = πr^2.
- Tangent: A tangent to a circle is a line that touches the circle at exactly one point. The point of tangency is where the tangent line intersects the circle.
- Chord: A chord is a line segment that connects two points on the circumference of a circle.
- Arc: An arc is a portion of the circumference of a circle.
By understanding these key terms, you will be better equipped to solve problems and communicate ideas related to circles. It is important to practice working with these terms in various example problems to solidify your understanding.
Exploring the center and radius
In the study of circles, it is important to understand the concept of the center and radius. The center of a circle is the point at which all points on the circle are equidistant. This means that if you were to measure the distance from any point on the circle to the center, it would be the same. The center is often denoted as a single point in the form (x, y), where x represents the x-coordinate and y represents the y-coordinate.
The radius of a circle is the distance from the center to any point on the circle. It can be thought of as the length of a line segment that connects the center to a point on the circle’s circumference. The radius is an important measurement when analyzing circles as it provides information about the size and shape of the circle. The radius is always constant for a given circle and can be represented by the letter r.
Properties of the center and radius:
- Any two circles can have different centers, but circles with the same center are identical.
- The radius of a circle is always positive.
- The diameter of a circle is equal to twice the length of the radius.
- A chord of a circle is a line segment connecting two points on the circumference and its length is always less than or equal to the diameter.
- The area of a circle can be calculated using the formula A = πr^2, where A represents the area and r represents the radius.
Understanding the center and radius of a circle is essential for solving problems related to circles in geometry. By knowing these properties, we can analyze and manipulate circles to find their measurements or solve more complex geometric problems.
Calculating Circumference
The circumference of a circle is the distance around its outer edge. It is an important measurement that can help us in various mathematical and real-life applications. To calculate the circumference of a circle, we need to know the radius or diameter of the circle.
Circumference = 2 π r
In the formula, π represents the mathematical constant pi, which is approximately equal to 3.14159. The radius (r) is the distance from the center of the circle to its outer edge. If you have the diameter (d) instead of the radius, you can use the following formula:
Circumference = π d
When calculating the circumference, it is important to use the correct units of measurement. For example, if the radius is given in centimeters, the circumference should be calculated in centimeters as well. Similarly, if the radius is given in inches, the circumference should be in inches.
An easy way to remember the formula for calculating circumference is to think of it as “twice the radius times pi” or “pi times the diameter.” Understanding how to calculate the circumference of a circle is essential in various fields such as geometry, engineering, and physics.
Understanding the concept of circumference
Circumference is a fundamental concept in geometry that refers to the distance around the edge of a circle. It is often represented by the symbol “C” and is calculated using the formula C = 2πr, where “r” represents the radius of the circle and π is a mathematical constant approximately equal to 3.14. This formula shows that the circumference is directly proportional to the radius, meaning that as the radius increases, the circumference also increases.
An important thing to note is that the circumference is different from the area of a circle. While the area measures the space enclosed by the circle, the circumference measures the length of the curve that forms the circle. Imagine taking a piece of string and wrapping it tightly around the edge of a circle – the length of that string is the circumference.
Understanding the concept of circumference is crucial for various practical applications. For example, it is used in construction to measure and layout circular structures such as roundabouts or silos. In engineering, the knowledge of circumference is essential for designing gears or wheels that fit precisely within a given space. In everyday life, knowing the circumference can be useful for estimating distances or determining the amount of material needed to wrap around circular objects.
Calculating the circumference of a circle may seem daunting at first, but with the formula C = 2πr, it becomes a straightforward process. Simply multiply the radius by 2 and then multiply the result by π. Alternatively, if you have the diameter (the distance across the circle passing through the center), you can use the formula C = πd, where “d” represents the diameter. Remember to use an accurate value for π to obtain precise results.
To summarize, understanding the concept of circumference is essential for various real-world applications. It allows us to measure the distance around a circle and is calculated using a simple formula. Whether in construction, engineering, or everyday life, knowing the circumference can help us solve problems and make accurate measurements.