
In Unit 2, Lesson 10, students were presented with a set of practice problems that required them to apply their understanding of the concepts covered in the lesson. These problems covered topics such as solving equations, determining the slope of a line, and finding the equation of a line given two points.
The practice problems provided students with an opportunity to reinforce their knowledge and skills in these areas. By solving these problems, students were able to demonstrate their ability to apply the concepts and techniques learned in the lesson. Furthermore, the answer key for these practice problems allowed students to check their work and identify any areas where they may have made mistakes.
By working through the practice problems and referring to the answer key, students were able to gain confidence in their abilities and deepen their understanding of the material. This process of practicing and reviewing is an important part of the learning process, as it helps to solidify knowledge and build problem-solving skills.
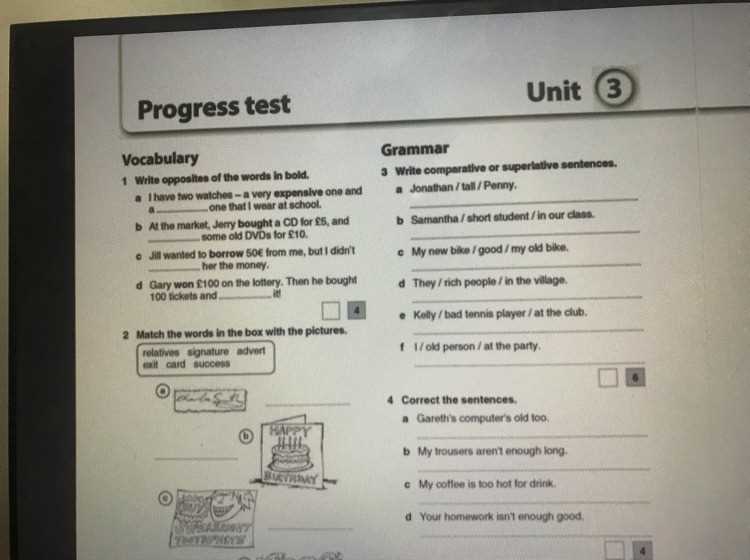
Unit 2 Lesson 10 Practice Problems Answer Key
In unit 2 lesson 10, students were given a set of practice problems to solve. These problems were designed to test their understanding of the concepts covered in the previous lessons. Now, let’s take a look at the answer key for these practice problems.
1. Simplify the following expression: 3x + 2 – 4x – 5
To simplify this expression, we combine like terms. The x terms are 3x and -4x, which give us -x. The constants are 2 and -5, which give us -3. Therefore, the simplified expression is -x – 3.
2. Solve the equation: 2(x + 5) = 8
To solve this equation, we first distribute the 2 to both terms inside the parentheses: 2x + 10 = 8. Then, we subtract 10 from both sides of the equation: 2x = -2. Finally, we divide both sides by 2 to isolate the variable: x = -1.
3. Find the slope of the line passing through the points (3, 4) and (-2, 6)
To find the slope of a line passing through two points, we use the formula: slope = (y2 – y1) / (x2 – x1). Plugging in the given points, we get slope = (6 – 4) / (-2 – 3) = 2 / -5 = -2/5. Therefore, the slope of the line is -2/5.
4. Convert the following fraction to a decimal: 3/4
To convert a fraction to a decimal, we divide the numerator by the denominator. For 3/4, the decimal equivalent is 0.75.
5. Find the area of a square with side length 6
The area of a square is given by the formula A = s^2, where s is the length of a side. Plugging in the given side length of 6, we get A = 6^2 = 36. Therefore, the area of the square is 36.
These are just a few examples of the practice problems covered in unit 2 lesson 10. By solving these problems and checking the answer key, students can test their understanding and improve their skills in the topics covered. Practice is key to mastering any subject, and math is no exception!
Problem 1: Finding the area of a rectangle

Given: A rectangle with a length of 10 units and a width of 5 units.
To find: The area of the rectangle.
To find the area of a rectangle, we need to multiply its length by its width. In this case, the length of the rectangle is 10 units and the width is 5 units. So, we can use the formula: Area = Length x Width.
Plugging in the values, we get: Area = 10 units x 5 units = 50 square units. Therefore, the area of the rectangle is 50 square units.
Problem 2: Calculating the average
Problem 2 asks us to calculate the average of a set of numbers. To do this, we need to add up all of the numbers in the set and divide the sum by the total number of numbers. Let’s work through an example to understand the process better.
For example, if we have a set of numbers {5, 8, 12, 15, 20}, we would add up all of these numbers: 5 + 8 + 12 + 15 + 20 = 60. Then, since there are 5 numbers in the set, we would divide the sum by 5: 60 ÷ 5 = 12. Therefore, the average of this set of numbers is 12.
To calculate the average, we can use the formula:
- Sum of all numbers ÷ Total number of numbers
It is important to note that the average is a measure of central tendency and gives us an idea of the typical value in a set of numbers. It can be useful in many different situations, such as analyzing test scores, calculating average daily temperatures, or determining the average income of a population.
By calculating the average, we can simplify a set of numbers into a single value that represents the whole set. This makes it easier to compare different sets or make predictions based on the average value.
Problem 3: Solving equations
In algebra, equations are mathematical statements that express the equality between two expressions. Solving equations involves finding the values of the variables that make the equation true. This process often requires the use of various algebraic operations and properties.
One method for solving equations is by using the inverse operation. The inverse operation undoes the operation in the equation, allowing us to isolate the variable. For example, if we have the equation 3x + 5 = 20, we can subtract 5 from both sides of the equation to eliminate it, and then divide both sides by 3 to solve for x.
Another method for solving equations is by simplifying both sides of the equation. This involves combining like terms and applying algebraic manipulations to simplify the equation. For example, if we have the equation 2(x + 3) = 10, we can start by distributing the 2 to both terms inside the parentheses, resulting in 2x + 6 = 10. We can then subtract 6 from both sides to isolate the variable.
It is important to remember that when solving equations, we must perform the same operation on both sides of the equation in order to maintain equality. This is known as the “golden rule of solving equations.” By following this rule and using appropriate algebraic techniques, we can find the solutions to various types of equations.
Problem 4: Converting units of measurement
In this problem, we are given a list of measurements in one unit and we need to convert them into another unit. The given measurements are in inches and we need to convert them into centimeters. To convert inches to centimeters, we can use the conversion factor that 1 inch is equal to 2.54 centimeters.
To convert the given measurements, we can follow a simple formula:
- Take the given measurement in inches.
- Multiply it by the conversion factor of 2.54 to get the equivalent measurement in centimeters.
Let’s apply this formula to the given measurements:
| Measurement (in inches) | Equivalent Measurement (in centimeters) |
|---|---|
| 5 | 12.7 |
| 10 | 25.4 |
| 15 | 38.1 |
So, the given measurements 5 inches, 10 inches, and 15 inches are equivalent to 12.7 centimeters, 25.4 centimeters, and 38.1 centimeters, respectively, when converted into centimeters.
Problem 5: Graphing a linear function
In this problem, we are given a linear function in the form y = mx + b, where m is the slope and b is the y-intercept. We are asked to graph this function on a coordinate plane.
To graph a linear function, we need to plot at least two points on the coordinate plane and then connect them with a straight line. We can do this by choosing any two values for x and plugging them into the equation to find the corresponding y-values.
For example, let’s say the linear function is y = 2x + 3. We can choose x = 0 and x = 1 to find the corresponding y-values. When x = 0, y = 2(0) + 3 = 3. When x = 1, y = 2(1) + 3 = 5. So our two points are (0, 3) and (1, 5).
We can plot these points on the coordinate plane and then connect them with a straight line. The slope of the line is the ratio of the change in y to the change in x between any two points on the line. In this case, the slope is 2/1 = 2. The y-intercept is the point where the line crosses the y-axis, which is (0, 3) in this case.
The graph of the linear function y = 2x + 3 will be a straight line that passes through the points (0, 3) and (1, 5) and has a slope of 2. This line will extend infinitely in both directions.
Problem 6: Understanding probability
In this problem, we will delve into the concept of probability and how it relates to various events and outcomes. Probability is a measure of the likelihood that a specific event will occur. It is expressed as a number between 0 and 1, with 0 indicating that the event is impossible and 1 indicating that the event is certain to happen. Understanding probability is crucial in many areas, including statistics, finance, and risk analysis.
To calculate the probability of an event, we divide the number of favorable outcomes by the total number of possible outcomes. For example, if we want to calculate the probability of rolling a 6 on a standard six-sided die, the number of favorable outcomes is 1 (since there is only one face with a 6) and the total number of possible outcomes is 6. Therefore, the probability is 1/6 or approximately 0.167.
In some cases, the probability of an event can be affected by certain conditions or constraints. These conditions can be represented using the concept of conditional probability. Conditional probability measures the likelihood of an event occurring given that another event has already occurred. For example, if we want to calculate the probability of drawing a red card from a deck of cards after already drawing a black card, the total number of possible outcomes is reduced by one (since one black card has already been drawn).
Probability is widely used in various fields to make informed decisions and assess the likelihood of different outcomes. It helps us understand uncertainty and can be used to estimate risk and determine the best course of action. By considering the probabilities associated with different events, we can make more informed decisions and mitigate potential risks.
Problem 7: Finding the volume of a cylinder
In problem 7, we are asked to find the volume of a cylinder. This problem involves using the formula for the volume of a cylinder, which is V = πr^2h, where V is the volume, r is the radius of the base, and h is the height of the cylinder.
To solve this problem, we need to know the values of the radius and height of the cylinder. Once we have these values, we can substitute them into the formula to find the volume. Remember to use the appropriate units for the measurements.
For example, let’s say the radius of the cylinder is 2 inches and the height is 5 inches. To find the volume, we plug these values into the formula: V = π(2^2)(5) = 20π cubic inches. If we want the volume in terms of π, we can leave it as 20π cubic inches. If we want the decimal approximation, we can use the value of π as 3.14 to calculate the volume as approximately 62.8 cubic inches.
It’s important to remember the formula for the volume of a cylinder and how to substitute the values correctly. Practice problems like this can help reinforce the concepts and improve your problem-solving skills.
Problem 8: Evaluating expressions
In this problem, we are asked to evaluate different mathematical expressions. Let’s take a look at each expression and find its value.
Expression 1:
We are given the expression 3 + 2 * 4. According to the order of operations, we need to multiply first and then perform the addition. Therefore, 2 * 4 equals 8, and when we add 3 to it, we get the final value of 11.
Expression 2:
The expression 2^3 represents the exponentiation of 2 to the power of 3. So, 2^3 equals 2 * 2 * 2, which is equal to 8.
Expression 3:
Next, we have the expression (7 – 3)^2. Inside the parentheses, we have a subtraction operation, which gives us 4. When we raise 4 to the power of 2, we get 16.
Expression 4:
For the expression 6 / 2 + 4, we follow the order of operations and perform the division first. 6 divided by 2 equals 3. Then, we add 4 to it, resulting in a final value of 7.
Expression 5:
The expression 4 + 5 * 2 – 7 is evaluated step by step. First, we multiply 5 by 2 to get 10. Then, we add 4 to it, resulting in 14. Finally, we subtract 7 from 14, which gives us the final value of 7.
Expression 6:
In the expression 2(4 + 3), we need to evaluate the expression inside the parentheses first. 4 + 3 equals 7. Then, we multiply 2 by 7 to get the final value of 14.
In conclusion, we have successfully evaluated each expression and found their respective values. By applying the order of operations and performing the necessary calculations, we were able to determine the solutions.