In Unit 6 Lesson 2, students were introduced to various practice problems that tested their understanding of the concepts covered in the lesson. These practice problems were designed to reinforce the key concepts and skills learned, and to provide students with an opportunity to apply their knowledge in real-world scenarios. The answer key for these practice problems is provided to help students check their answers and evaluate their understanding of the lesson.
In this answer key, you will find the correct answers to the practice problems, along with a detailed explanation of the steps involved in solving each problem. The answer key is organized according to the practice problems presented in the lesson, making it easy for students to navigate and find the answers they are looking for.
Using the answer key, students can compare their answers to the correct ones and identify any areas where they may have made errors. This allows them to learn from their mistakes and improve their understanding of the concepts covered in the lesson. Additionally, the detailed explanations provided in the answer key can help students understand the thought process and reasoning behind each step, further enhancing their understanding of the material.
Unit 6 Lesson 2 Practice Problems Answer Key
In Unit 6 Lesson 2, students were tasked with completing a series of practice problems related to the concepts covered in the lesson. The answer key provides the correct answers to these problems, allowing students to check their work and ensure they understand the material.
The practice problems covered a range of topics, including solving equations, identifying linear and nonlinear functions, finding the slope and y-intercept of a line, and graphing equations. The answer key provides step-by-step explanations for each problem, guiding students through the process of solving the equation or graphing the function.
For example, one of the practice problems asked students to solve the equation “3x + 7 = 22”. The answer key would show the steps involved in isolating the variable and solving for x, leading to the correct answer of x = 5.
In addition to providing the correct answers, the answer key also offers explanations and tips to help students understand the underlying concepts. This allows students to not only check their work but also learn from any mistakes they may have made.
The answer key is a valuable resource for students as it allows them to independently practice and assess their understanding of the material. By using the answer key, students can identify any areas of weakness and seek additional support or clarification from their teacher or peers.
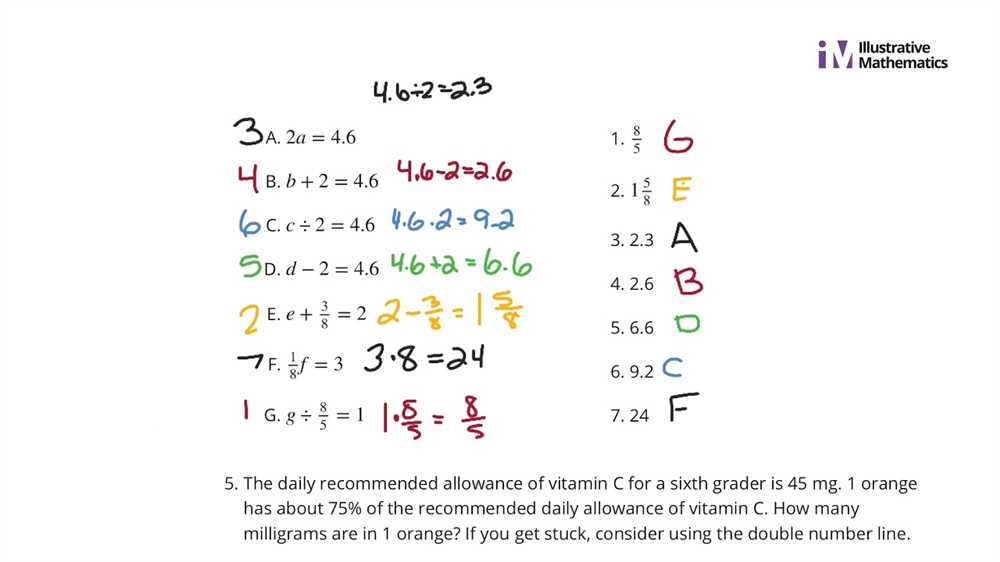
Problem 1: Solving Equations
In this problem, we will be solving equations. Equations are mathematical expressions that contain an equals sign (=) and an unknown variable. The goal is to find the value of the variable that makes the equation true. To solve equations, we use properties of equality and perform operations to isolate the variable on one side of the equation.
Let’s take a look at an example:
Example:
3x + 7 = 22
To solve this equation, we want to isolate the variable x. We can do this by performing inverse operations. First, we subtract 7 from both sides of the equation to get:
3x = 15
Next, we divide both sides of the equation by 3 to get:
x = 5
Therefore, the solution to the equation is x = 5. This means that when we substitute the value 5 for x in the original equation, it will be true:
3(5) + 7 = 22
15 + 7 = 22
22 = 22
In this case, x = 5 is the only value that satisfies the equation and makes it true.
Problem 2: Graphing Linear Equations
Graphing linear equations is an essential skill in mathematics. It allows us to visually represent the relationships between variables and understand the behavior of the equation. In this problem, we will practice graphing linear equations to gain a better understanding of their behavior and patterns.
The first step in graphing a linear equation is identifying its slope-intercept form: y = mx + b, where m represents the slope and b represents the y-intercept. Once we have the equation in this form, we can easily plot points on the coordinate plane and connect them to form a straight line.
Let’s consider an example: y = 2x + 3. Here, the slope is 2, which means that for every one unit increase in x, y increases by 2 units. The y-intercept is 3, which indicates that the line intersects the y-axis at the point (0, 3). To graph this equation, we can start from the y-intercept and use the slope to find additional points on the line.
To find another point, we can use the slope by taking the rise (vertical change) over run (horizontal change). For example, if we increase x by 1 unit, y would increase by 2 units. Starting from the point (0, 3), we can move 1 unit to the right and 2 units up to get the point (1, 5). By connecting these two points, we can draw the line representing the equation y = 2x + 3.
By graphing linear equations, we can visually analyze their behavior and identify the slope, intercepts, and other important characteristics. This helps us understand the relationship between variables and make predictions about their values in different situations.
Problem 3: Systems of Equations
In the topic of systems of equations, we are given multiple equations with multiple variables and we need to find the values of those variables that satisfy all the equations simultaneously. This is often used to solve real-world problems where multiple variables are involved.
In this practice problem, we are given the following system of equations:
- 2x + 3y = 7
- x – y = 1
We need to find the values of x and y that satisfy both equations.
To solve this system of equations, we can use the method of substitution or the method of elimination. Let’s use the method of substitution for this problem.
From equation 2, we can rearrange it to express x in terms of y:
x = y + 1
Now substitute this expression for x in equation 1:
2(y + 1) + 3y = 7
Simplifying the equation:
2y + 2 + 3y = 7
5y + 2 = 7
5y = 5
y = 1
Now substitute the value of y back into the expression for x:
x = 1 + 1
x = 2
Therefore, the solution to this system of equations is x = 2 and y = 1.
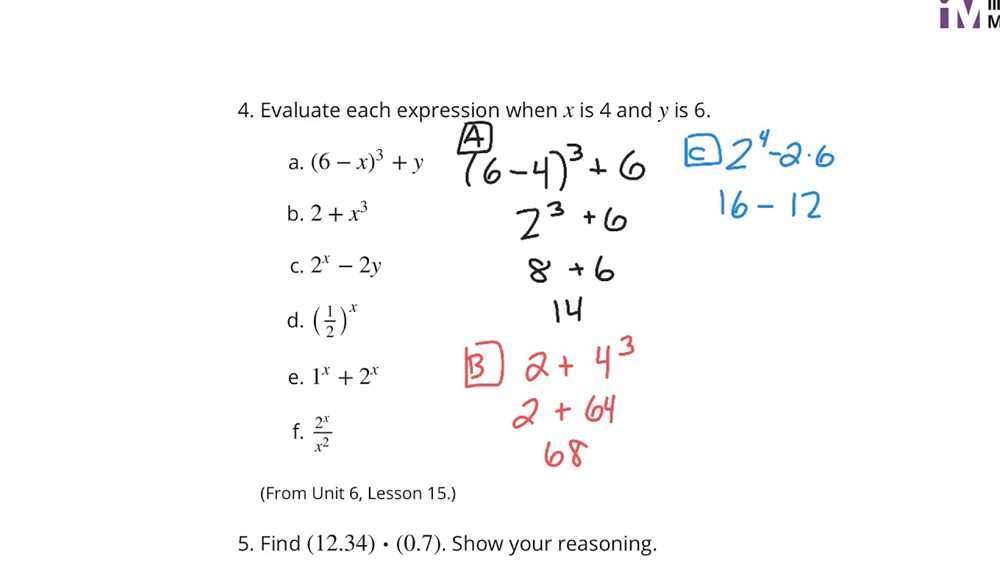
Problem 4: Quadratic Equations
In this problem, we will be solving quadratic equations using the quadratic formula. A quadratic equation is an equation of the form ax^2 + bx + c = 0, where a, b, and c are constants and x is the variable.
The quadratic formula is used to find the roots, or solutions, of a quadratic equation. The formula is: x = (-b ± √(b^2 – 4ac)) / 2a.
When using the quadratic formula, it is important to remember that the discriminant, b^2 – 4ac, can help determine the type of solutions. If the discriminant is positive, the equation has two real and distinct solutions. If the discriminant is zero, the equation has one real solution. If the discriminant is negative, the equation has two complex solutions.
To solve the quadratic equation, substitute the values of a, b, and c into the quadratic formula and simplify. Then, use the discriminant to determine the type of solutions and calculate the values of x, if they exist.
It is also worth noting that quadratic equations often arise in real-world problems, such as calculating the trajectory of a projectile or finding the roots of a polynomial function.
Problem 5: Exponential Equations

In this problem, we will be working with exponential equations. Exponential equations are equations in which the variable appears in the exponent. Solving exponential equations involves isolating the variable and using logarithms.
Let’s consider an example to understand how to solve exponential equations. If we have the equation 2x = 8, we need to determine the value of x that satisfies this equation. We can rewrite 8 as 23, so the equation becomes 2x = 23. Now, we can equate the exponents and solve for x. Our equation becomes x = 3, so the solution to the equation is x = 3.
It is important to note that exponential equations can have multiple solutions. For example, if we have the equation 2x = 16, we can rewrite 16 as 24. Our equation becomes 2x = 24. Now, we can equate the exponents and solve for x. Our equation becomes x = 4, so one solution is x = 4. However, we also know that 22 equals 4, so another solution is x = 2. Therefore, the equation 2x = 16 has two solutions: x = 2 and x = 4.
When solving exponential equations, it is important to check your solutions to ensure they are valid. Sometimes, solutions can result in non-real or extraneous values. By substituting the solutions back into the original equation, you can verify if they are true solutions.
In summary, exponential equations involve variables in the exponents. To solve these equations, you will need to manipulate the equations using logarithms and isolate the variable. Exponential equations can have multiple solutions, so it is important to check your solutions to ensure they are valid.
Problem 6: Rational Equations

In this problem, we are dealing with rational equations. A rational equation is an equation that contains one or more rational expressions. A rational expression is a fraction in which the numerator and/or the denominator are polynomials.
In order to solve a rational equation, we need to find the values of the variable that make the equation true. To do this, we can follow these steps:
- Identify any restrictions on the variable. These are values that would make the denominator of a rational expression equal to zero, resulting in an undefined expression.
- Simplify the equation by clearing the fractions. This can be done by finding a common denominator for all the fractions in the equation.
- Solve the resulting polynomial equation by factoring or using other algebraic methods.
- Check the solutions by substituting them back into the original equation to make sure they satisfy the equation.
Let’s look at an example to see how these steps can be applied. Consider the rational equation:
(3/x) + 2 = (5/(x+1))
First, we need to identify any restrictions on the variable. In this case, the variable x cannot be zero or -1, as these values would make the denominators equal to zero.
Next, we can simplify the equation by clearing the fractions. To do this, we can find a common denominator for all the fractions, which in this case is x(x+1). Multiplying both sides of the equation by x(x+1) will eliminate the denominators:
x(x+1)(3/x) + 2x(x+1) = x(x+1)(5/(x+1))
Simplifying further, we have:
3(x+1) + 2x(x+1) = 5x
Expanding and combining like terms:
3x + 3 + 2x^2 + 2x = 5x
Now we have a polynomial equation that can be solved using algebraic methods. In this example, we can rearrange the terms to get a quadratic equation:
2x^2 -2x – 3 = 0
We can solve this quadratic equation by factoring, completing the square, or using the quadratic formula:
(2x + 1)(x – 3) = 0
So the solutions are x = -1/2 or x = 3.
Finally, we need to check if these solutions satisfy the original equation. Substituting x = -1/2 into the equation, we get:
(3/(-1/2)) + 2 = (5/((-1/2)+1))
-6 + 2 = -10
Since this equation is not true, we reject the solution x = -1/2.
Substituting x = 3 into the equation, we get:
(3/3) + 2 = (5/(3+1))
1 + 2 = 5/4
Since this equation is true, we accept the solution x = 3.
The solution to the rational equation (3/x) + 2 = (5/(x+1)) is x = 3.
Problem 7: Absolute Value Equations
To solve this type of equation, we need to consider two cases:
- When (x – a) is non-negative: In this case, the absolute value signs are unnecessary and we can simply set x – a equal to b. Solving for x, we find x = a + b.
- When (x – a) is negative: In this case, the absolute value signs will make (x – a) positive. To remove the absolute value signs, we can rewrite the equation as -(x – a) = b. Solving for x, we find x = a – b.
Therefore, the solution to the absolute value equation |x – a| = b is x = a + b or x = a – b, depending on whether (x – a) is non-negative or negative. It is important to check the solution(s) obtained by substituting them back into the original equation to ensure they satisfy the given condition.
Problem 8: Radical Equations
In this problem, we are given a radical equation and we need to solve for the variable. A radical equation is an equation that contains a square root, cube root, or higher order radical.
Let’s start by looking at the given equation:
- Equation: √(2x + 3) – 4 = 5
The objective is to isolate the variable on one side of the equation. To do this, we follow these steps:
- Add 4 to both sides of the equation to eliminate the -4 on the left-hand side.
- We now have √(2x + 3) = 9.
- Next, square both sides of the equation to eliminate the square root.
- We get 2x + 3 = 81.
- Now, subtract 3 from both sides of the equation to eliminate the 3 on the left-hand side.
- We obtain 2x = 78.
- Finally, divide both sides of the equation by 2 to solve for x.
- We end up with the solution x = 39.
Therefore, the solution to the given radical equation is x = 39.