
In the study of geometry, polygons and quadrilaterals are important concepts that help us understand the properties and characteristics of various shapes. Unit 7 of our mathematics curriculum focuses on these topics, and this answer key is a valuable resource for both students and teachers.
With this PDF answer key, students can easily check their work and confirm their understanding of the material covered in Unit 7. It provides step-by-step solutions to the exercises and problems presented in the textbook, ensuring that students can identify any errors and learn from them.
Furthermore, this answer key is designed to facilitate a deeper understanding of polygons and quadrilaterals. Each solution is explained in a clear and concise manner, highlighting the key concepts and strategies used to arrive at the correct answer. This not only helps students correct their mistakes but also enhances their problem-solving skills.
Teachers will also find this answer key to be an invaluable tool in their instruction. It allows them to quickly assess their students’ progress and identify any areas where additional instruction may be needed. The detailed explanations provided in the answer key can also serve as a valuable resource for lesson planning and classroom discussions.
In conclusion, the Unit 7 Polygons and Quadrilaterals Answer Key PDF is an essential resource for both students and teachers. It provides a comprehensive and detailed solution guide to the exercises and problems in the textbook, helping students improve their understanding of the material and assisting teachers in their instruction. By using this answer key, students can confidently approach their geometry studies and educators can effectively guide their students towards success in this field of mathematics.
What Are Polygons and Quadrilaterals?
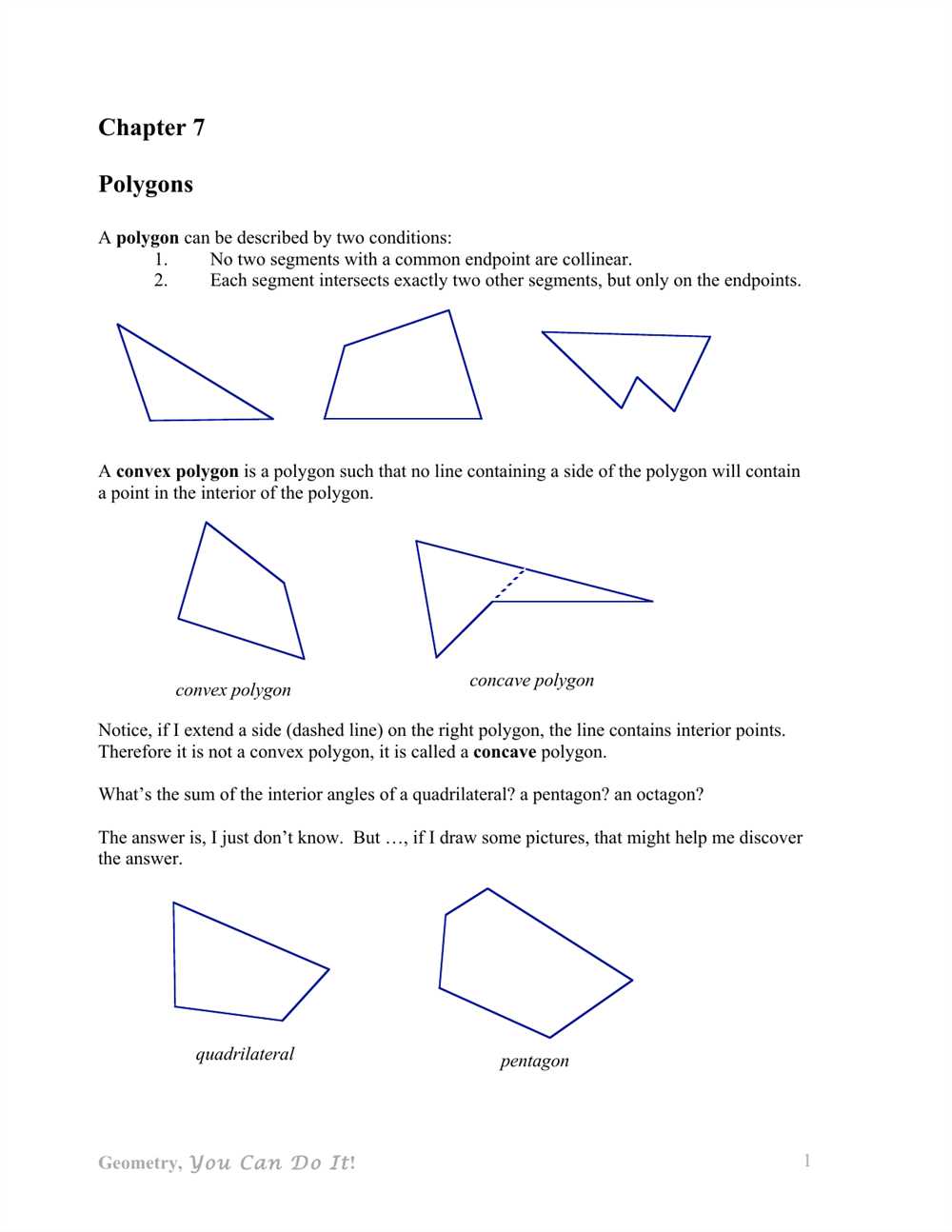
Polygons and quadrilaterals are two fundamental concepts in geometry. A polygon is a closed figure made up of straight line segments, while a quadrilateral is a specific type of polygon that has four sides.
One key characteristic of polygons is that the sum of their interior angles is always equal to (n-2) * 180 degrees, where n represents the number of sides. For example, a triangle (a polygon with three sides) has interior angles that add up to 180 degrees, while a pentagon (a polygon with five sides) has interior angles that add up to 540 degrees.
Quadrilaterals are a special type of polygon that have four sides. They can have different combinations of angles and side lengths, which classifies them into various subcategories. Some common types of quadrilaterals include rectangles, squares, parallelograms, trapezoids, and rhombuses.
Rectangles are quadrilaterals with four right angles, while squares have four congruent sides and four right angles. Parallelograms have opposite sides that are parallel and congruent, and trapezoids have one pair of opposite sides that are parallel. Rhombuses, on the other hand, have opposite sides that are parallel and congruent, but their angles do not necessarily have to be right angles.
Understanding the properties and characteristics of polygons and quadrilaterals is not only important in geometry, but also in many real-life applications. Whether it’s calculating the area and perimeter of a rectangular room or designing a blueprint for a building, knowledge of polygons and quadrilaterals is essential in solving geometric problems and analyzing shapes in the real world.
Definition of Polygons
A polygon is a two-dimensional shape that is formed by straight lines called sides. It is made up of line segments that intersect only at their endpoints. Polygons can have any number of sides, as long as they are connected in a closed loop. The word “polygon” comes from the Greek words “poly,” meaning many, and “gonia,” meaning angles.
There are several types of polygons, including triangles, quadrilaterals, pentagons, hexagons, and so on. Triangles have three sides, quadrilaterals have four sides, pentagons have five sides, and so on. A polygon is classified based on the number of sides it has. For example, a polygon with six sides is called a hexagon.
In addition to the sides, polygons also have vertices, which are the points where two sides intersect. The number of vertices in a polygon is the same as the number of sides. For example, a triangle has three sides and three vertices. The vertices of a polygon are labeled using capital letters.
Polygons can be regular or irregular. A regular polygon is a polygon in which all sides are of equal length and all angles are equal. An irregular polygon is a polygon that does not have equal sides or angles. Regular polygons are often used in geometry because they have certain properties that make them easier to work with.
In conclusion, a polygon is a closed two-dimensional shape formed by straight lines. It can have any number of sides, and its sides intersect only at their endpoints. Polygons can be classified based on the number of sides they have, and they can be regular or irregular. Understanding the properties and classifications of polygons is fundamental in geometry.
Definition of Quadrilaterals
A quadrilateral is a polygon that has four sides, four angles, and four vertices. It is a closed figure formed by four line segments. The word “quadrilateral” comes from the Latin words “quadri” meaning four, and “latus” meaning side. Quadrilaterals can have various properties and can be classified into different types based on their characteristics.
There are several types of quadrilaterals, including squares, rectangles, parallelograms, rhombi, trapezoids, and kites. Each type of quadrilateral has its own unique set of properties. For example, a square is a quadrilateral with all four sides of equal length and all four angles of 90 degrees. A rectangle is a quadrilateral with opposite sides of equal length and all four angles of 90 degrees.
Parallelograms are quadrilaterals with opposite sides parallel and equal in length. Rhombi have all four sides of equal length, while trapezoids have one pair of parallel sides. Kites have two pairs of adjacent sides that are equal in length. Each type of quadrilateral has its own set of formulas and rules that govern its properties and characteristics.
Understanding the properties and classifications of quadrilaterals is essential in geometry and other areas of mathematics. It allows us to identify and differentiate between different types of quadrilaterals and solve problems involving their properties and relationships. By studying quadrilaterals, we can gain a deeper understanding of geometric shapes and their properties.
Properties of Polygons
A polygon is a two-dimensional shape with straight sides. It is formed by connecting line segments called sides, and the endpoints where the sides intersect are called vertices. Polygons can have different numbers of sides and angles, which give them unique properties and characteristics.
1. Number of Sides: The number of sides in a polygon determines its name. For example, a polygon with three sides is called a triangle, while a polygon with four sides is called a quadrilateral. Polygons can have any number of sides, but they must have at least three.
2. Sum of Interior Angles: The sum of the interior angles of a polygon depends on the number of sides it has. The formula to find the sum of the interior angles is (n-2) * 180 degrees, where n is the number of sides. For example, a triangle has three sides, so the sum of its interior angles is (3-2) * 180 = 180 degrees.
3. Regular and Irregular Polygons: A polygon is considered regular if all of its sides and angles are equal. For example, a square is a regular quadrilateral because all four sides and angles are equal. An irregular polygon, on the other hand, has sides and angles of different lengths and measures.
4. Diagonals: Diagonals are line segments that connect non-adjacent vertices of a polygon. The number of diagonals in a polygon can be found using the formula n * (n-3) / 2, where n is the number of sides. Diagonals can divide a polygon into smaller triangles or quadrilaterals.
5. Perimeter and Area: The perimeter of a polygon is the sum of its side lengths, while the area is the measure of the space inside the polygon. The formulas to calculate the perimeter and area of different polygons vary based on their shapes and properties.
Understanding the properties of polygons is essential in geometry, as it allows us to classify and describe different shapes based on their characteristics. These properties help us solve problems and make connections between different geometric concepts.
Number of Sides
Polygons are two-dimensional shapes that are made up of straight sides. The number of sides a polygon has is determined by its name. Different names are given to polygons based on the number of sides they have.
The simplest polygon is a triangle, which has three sides. Triangles can be classified further based on the lengths of their sides and the measures of their angles. Equilateral triangles have three equal sides and three equal angles. Isosceles triangles have two equal sides and two equal angles. Scalene triangles have no equal sides or angles.
Quadrilaterals are polygons with four sides. Some examples of quadrilaterals include squares, rectangles, parallelograms, and trapezoids. Squares have four equal sides and four equal angles. Rectangles have four right angles, but the lengths of their sides can vary. Parallelograms have opposite sides that are equal and parallel. Trapezoids have only one pair of parallel sides.
Pentagons have five sides, and hexagons have six sides. Other polygons include heptagons (seven sides), octagons (eight sides), nonagons (nine sides), and decagons (ten sides). The names of these polygons are derived from the Greek words for the corresponding numbers.
In summary, the number of sides a polygon has determines its name and its properties. Different polygons have different numbers of sides, leading to a wide range of shapes and configurations in geometry.
Sum of Interior Angles
In geometry, the sum of the interior angles of a polygon is a key concept. The interior angles of a polygon are the angles formed by any two sides within the polygon. To find the sum of the interior angles, we can use the formula:
Sum of Interior Angles = (n – 2) × 180°
Where n represents the number of sides or vertices of the polygon.
This formula holds true for any polygon, whether it be a triangle, quadrilateral, pentagon, or any other regular or irregular polygon. By knowing the number of sides of a polygon, we can calculate the sum of its interior angles.
To apply this formula, simply substitute the value of n into the equation and solve for the sum of the interior angles. For example, if we have a pentagon (a polygon with five sides), we can substitute n = 5 into the formula to find the sum of its interior angles:
Sum of Interior Angles = (5 – 2) × 180° = 3 × 180° = 540°
Therefore, the sum of the interior angles of a pentagon is 540 degrees.
Understanding the concept of the sum of interior angles is fundamental in geometry and can help us analyze and solve problems related to polygons and quadrilaterals. By applying this formula, we can determine the total degrees of the interior angles in any given polygon.
Regular vs. Irregular Polygons
In geometry, a polygon is a plane figure that is bounded by a finite chain of straight line segments. Polygons can be classified into two main categories: regular and irregular.
A regular polygon is a polygon in which all sides are congruent (equal in length) and all angles are congruent (equal in measure). This means that a regular polygon has both equal sides and equal angles. Examples of regular polygons include equilateral triangles, squares, and hexagons.
An irregular polygon, on the other hand, is a polygon that does not have equal sides or equal angles. The sides and angles of an irregular polygon may vary in length and measure. This means that an irregular polygon does not have a regular pattern. Examples of irregular polygons include scalene triangles, rectangles, and pentagons.
To determine whether a polygon is regular or irregular, you can examine its sides and angles. If all sides and angles are congruent, the polygon is regular. If any sides or angles are not congruent, the polygon is irregular.
It is important to understand the distinction between regular and irregular polygons because regular polygons have special properties and formulas that can be used to calculate their perimeter, area, and other geometric characteristics. Irregular polygons, on the other hand, require more complex calculations and often involve breaking the polygon into smaller, regular shapes in order to solve geometric problems.
In conclusion, regular polygons have equal sides and equal angles, while irregular polygons do not. Regular polygons have specific formulas and properties that can be used to solve geometric problems, while irregular polygons require more advanced techniques and calculations.
Classification of Quadrilaterals
A quadrilateral is a polygon with four sides. There are different types of quadrilaterals, each with its own unique characteristics and properties. In order to classify quadrilaterals, we consider the types of angles and sides they have.
One way to classify quadrilaterals is based on their angles. A quadrilateral can be classified as either a rectangle, square, parallelogram, rhombus, trapezoid, or kite. A rectangle has four right angles, while a square has four right angles and four congruent sides. A parallelogram has opposite sides that are parallel and equal in length, while a rhombus has opposite sides that are parallel and equal in length, as well as four congruent sides. A trapezoid has one pair of parallel sides, and a kite has two pairs of consecutive sides that are congruent.
In addition to classifying quadrilaterals based on their angles, we can also classify them based on their sides. A quadrilateral can be classified as either a regular quadrilateral or an irregular quadrilateral. A regular quadrilateral, also known as an equilateral quadrilateral, has all four sides congruent, while an irregular quadrilateral has sides of different lengths.