As you prepare for the upcoming Unit 7 test on polynomials and factoring, it’s important to have a solid understanding of the key concepts and skills covered in this unit. Polynomials and factoring are fundamental topics in algebra, and mastering them will provide a strong foundation for future math courses.
A polynomial is an algebraic expression that consists of one or more terms. These terms can be combined using addition, subtraction, multiplication, and division. Understanding the different types of polynomials, such as monomials, binomials, and trinomials, is essential for solving problems involving polynomial operations.
Factoring is the process of breaking down a polynomial into its simpler components, or factors. This skill is crucial for simplifying expressions, solving equations, and finding the roots of polynomials. By factoring a polynomial, you can determine its zeros, which are the values of the variable that make the polynomial equal to zero.
During the Unit 7 test, you can expect a variety of questions that assess your ability to manipulate polynomials, perform operations on them, and factor them. Be prepared to simplify expressions, solve equations using factoring techniques, and find the zeros of polynomials. Practice these skills through a combination of textbook exercises, online resources, and collaborative study sessions with your classmates.
Unit 7 Test Study Guide: Polynomials and Factoring
In this study guide, we will cover important concepts and techniques related to polynomials and factoring. These topics are essential for understanding algebraic expressions and solving equations. By mastering these concepts, you will be well-prepared for the upcoming test.
Polynomials:
- A polynomial is an expression that consists of variables, coefficients, and exponents, combined using addition, subtraction, and multiplication.
- The degree of a polynomial is determined by the highest exponent of the variable. For example, a quadratic polynomial has a degree of 2.
- Polynomials can be classified based on their number of terms. A monomial has only one term, a binomial has two terms, and a trinomial has three terms.
- Operations on polynomials include addition, subtraction, multiplication, and division. It is important to know how to perform these operations accurately.
Factoring:
- Factoring a polynomial involves rewriting it as a product of simpler polynomials.
- Factoring can help in simplifying expressions, solving equations, and finding the roots of a polynomial.
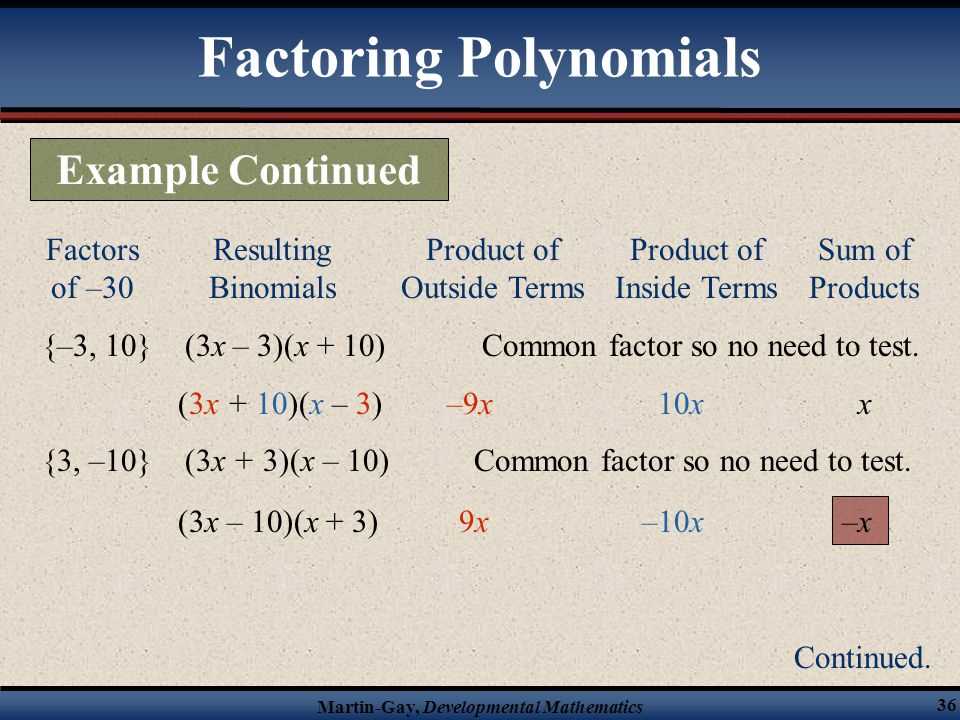
- Common factoring techniques include factoring out the greatest common factor, factoring by grouping, and factoring trinomials using the FOIL method.
- Factoring quadratic trinomials can be done using methods such as the quadratic formula, completing the square, or trial and error.
It is important to practice solving various problems involving polynomials and factoring to gain a solid understanding of these concepts. Reviewing examples, working through practice problems, and seeking clarification on any confusing topics will greatly improve your chances of success on the unit test. Good luck!
Understanding Polynomials
A polynomial is a mathematical expression that consists of variables, coefficients, and mathematical operations such as addition, subtraction, multiplication, and division. Polynomials can have one or more terms, with each term containing a variable raised to a power and multiplied by a coefficient. Understanding polynomials is crucial in various areas of mathematics, including algebra, calculus, and geometry.
One important concept in understanding polynomials is degree. The degree of a polynomial is the highest exponent of the variable in any term. For example, in the polynomial 3x^2 + 2x – 1, the degree is 2 because the highest exponent of the variable x is 2. The degree of a polynomial determines its behavior and properties.
Polynomials can be classified based on their degree. A polynomial with a degree of 0 is called a constant polynomial, as it does not contain any variables. A polynomial with a degree of 1 is called a linear polynomial, while a polynomial with a degree of 2 is called a quadratic polynomial. Higher-degree polynomials, such as cubic, quartic, and quintic polynomials, have degrees of 3, 4, and 5, respectively. Understanding the degree of a polynomial helps in solving equations, graphing functions, and analyzing mathematical problems.
Factoring is another important concept related to polynomials. Factoring involves finding the factors of a polynomial, which are the expressions that, when multiplied together, produce the original polynomial. Factoring allows us to simplify and solve polynomial equations, as well as analyze the behavior of polynomial functions. Understanding different factoring techniques, such as factoring by grouping, factoring trinomials, and factoring by using special patterns, is essential in effectively working with polynomials.
In conclusion, understanding polynomials is crucial for various areas of mathematics. It involves understanding the concept of degree, classifying polynomials based on their degree, and mastering factoring techniques. By having a solid understanding of polynomials, individuals can solve equations, graph functions, and analyze mathematical problems with confidence and accuracy.
Degree and Leading Coefficient
In the context of polynomials, the degree refers to the highest power of the variable in the polynomial. The degree determines the shape and behavior of the polynomial. The leading coefficient is the coefficient of the term with the highest power.
The degree of a polynomial can be determined by examining the exponents of the variable terms. For example, in the polynomial 3x^2 + 2x – 1, the highest power of x is 2, so the degree of the polynomial is 2. The degree of a polynomial can also be determined by considering the terms with nonzero coefficients. In this example, all the terms have nonzero coefficients, so the degree is not affected by any zero coefficients.
The leading coefficient is the coefficient of the term with the highest power. In the polynomial 3x^2 + 2x – 1, the leading coefficient is 3, as it is the coefficient of the term with the highest power, x^2. The leading coefficient plays an important role in determining the end behavior of the polynomial. It helps determine if the graph of the polynomial will be positive or negative for large values of x.
Adding and Subtracting Polynomials
Polynomials are mathematical expressions that consist of one or more terms, where each term is a variable raised to a power and multiplied by a coefficient. Adding and subtracting polynomials involves combining like terms to simplify the expression.
To add or subtract polynomials, you need to match the variables and their corresponding powers. You can think of it as combining similar terms, just like you would when adding or subtracting numbers.
Adding Polynomials:
- First, arrange the polynomials in standard form, with the highest power term first.
- Add the coefficients of the like terms. If a term is missing, assume its coefficient is 0.
- Write the sum of the like terms as the simplified expression.
Subtracting Polynomials:
- To subtract polynomials, change the sign of each term in the polynomial being subtracted (using the distributive property).
- Follow the same steps as adding polynomials.
Remember to always simplify the expression by combining like terms and writing the polynomial in standard form, with the terms in descending order of degree.
Working with polynomials requires careful attention to details and organization. It is important to understand the rules for adding and subtracting polynomials in order to solve polynomial equations and expressions accurately.
Multiplying Polynomials
In algebra, multiplying polynomials is a fundamental operation that involves combining the terms of two or more polynomials to obtain a new polynomial. This process is often used in solving equations, simplifying expressions, and finding the area and volume of geometric shapes.
When multiplying polynomials, it is crucial to remember the distributive property, as it is the key principle behind the process. The distributive property states that the product of a sum is equal to the sum of the individual products of each term. In the context of polynomial multiplication, this means that each term of one polynomial must be multiplied by each term of the other polynomial, and the results are then added together.
To illustrate this, consider the multiplication of two binomials: (a + b) and (c + d). Using the distributive property, we would multiply each term of the first binomial by each term of the second binomial. This leads to four individual multiplications: a * c, a * d, b * c, and b * d. Finally, we combine these terms to obtain the resulting polynomial.
In general, when multiplying polynomials, it is crucial to arrange the terms in descending order of degree to simplify the process. This allows for more efficient multiplication and helps to identify like terms that can be combined later on.
Furthermore, it is essential to practice multiplying polynomials regularly to improve proficiency in algebraic computations and problem-solving. By mastering this skill, students can effectively manipulate expressions and equations, leading to success in other areas of mathematics.
Dividing Polynomials
In algebra, dividing polynomials is an important skill that allows us to simplify complex expressions and solve equations. It involves dividing one polynomial by another polynomial. Before we dive into the process of dividing polynomials, let’s review some key terms and concepts.
A polynomial is an algebraic expression consisting of variables, coefficients, and exponents. It can contain terms of different degrees, such as constants (0th degree), linear terms (1st degree), quadratic terms (2nd degree), and so on. Division of polynomials is similar to division of numbers, but with some additional rules to take into account the variables and exponents.
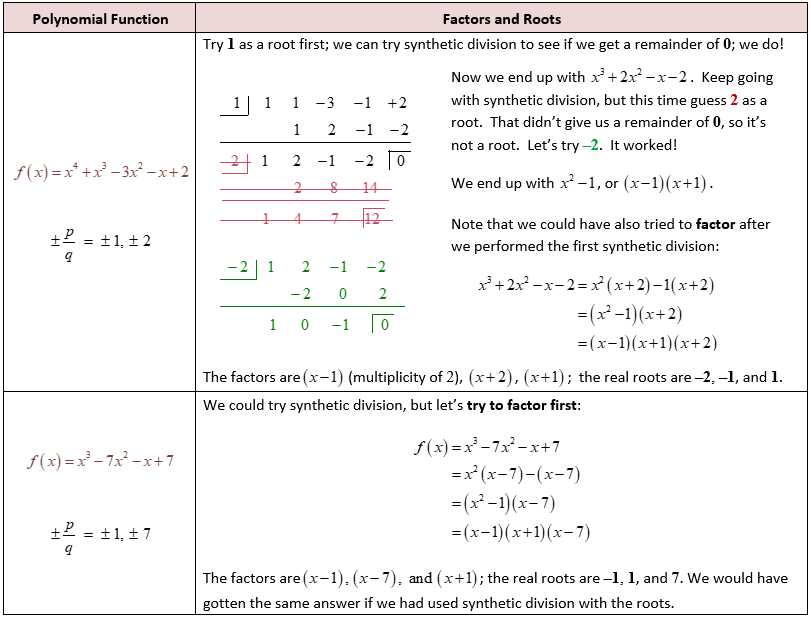
When dividing polynomials, the goal is to simplify the expression by finding the quotient and the remainder. The quotient is the result of the division, while the remainder is any remaining terms that could not be divided evenly. To divide polynomials, we use the long division method or the synthetic division method, depending on the situation.
When using long division, we divide the highest degree term of the dividend by the highest degree term of the divisor, and then multiply the divisor by the result. This product is then subtracted from the dividend, and the process is repeated with the new resulting expression. The process continues until we can no longer divide evenly, at which point we have the quotient and the remainder.
Synthetic division is a quicker method that can be used when dividing by a linear factor of the form (x – a). It involves writing the coefficients of the polynomial in a specific format and performing a sequence of calculations to find the quotient and remainder. Synthetic division is especially useful when dealing with polynomials of higher degrees.
Overall, dividing polynomials is an essential skill in algebra that allows us to simplify expressions and solve equations. It requires an understanding of key concepts and methods like long division and synthetic division. Practice and familiarity with these techniques will help you become proficient in dividing polynomials.
Factoring Polynomials
Factoring polynomials is an important skill in algebra because it allows us to find the roots or zeros of a polynomial equation. By factoring a polynomial, we can break it down into simpler terms and solve for the values of x that make the equation equal to zero.
When factoring a polynomial, we look for common factors, such as binomial factors or trinomial factors, that can be pulled out of the equation. This process of factoring helps us simplify the polynomial and make it easier to work with.
There are different methods for factoring polynomials, depending on the degree and structure of the polynomial. Some common factoring methods include factoring by grouping, factoring quadratics, factoring by trial and error, and factoring by using special product formulas.
Factoring can also be used to solve and graph polynomial equations, as well as to simplify rational expressions. It is an essential skill for solving various real-world problems, particularly in fields such as physics, engineering, and finance.
To be proficient in factoring polynomials, it is important to practice and understand the different factoring techniques and strategies. With enough practice, factoring can become a straightforward process and a valuable tool in algebraic problem-solving.
Solving Polynomial Equations
The process of solving polynomial equations involves finding the values of the variable that satisfy the equation. This is done by manipulating the equation algebraically to isolate the variable on one side of the equation. After isolating the variable, the equation can be solved by finding the roots or zeros of the polynomial function.
To solve polynomial equations, various methods can be used, such as factoring, using the quadratic formula, or using the rational root theorem. Factoring is a common method used when the polynomial can be easily factored into linear factors. By setting each factor equal to zero, the solutions can be found. For more complex polynomials, the quadratic formula can be used to solve for the roots. The rational root theorem is another method that can be used to find the rational roots of a polynomial equation.
In addition to these methods, graphing the polynomial function can also provide insights into the solutions of the equation. The x-intercepts of the graph represent the solutions to the equation. By analyzing the graph, it is possible to determine the number and nature of the solutions, whether they are real, complex, repeated, or irrational.
It is important to note that not all polynomial equations can be solved using these methods. Some polynomial equations may have no real solutions or may require more advanced techniques to solve. In these cases, numerical approximations or computer algorithms may be used to find an approximate solution.
Applications of Polynomials
Polynomials are mathematical expressions that involve variables raised to various powers. They are used in a wide range of applications in fields such as physics, engineering, economics, and computer science. One common application of polynomials is in modeling and analyzing real-world phenomena.
1. Physics: In physics, polynomials are used to describe the motion of objects. For example, the equation of motion for a falling object can be represented by a quadratic polynomial. By solving this equation, we can determine important parameters such as the time of flight and the maximum height reached by the object.
2. Engineering: In engineering, polynomials are used in various applications such as circuit design and signal processing. For instance, electrical engineers use polynomials to model the behavior of complex circuits and analyze their performance. Polynomial interpolation is also commonly used in signal processing to estimate missing data points in a sequence.
3. Economics: Polynomials are often used in economic modeling to analyze supply and demand curves. By fitting polynomial functions to observed data, economists can estimate the relationship between different variables and make predictions about market behavior. Polynomials are also used in optimization problems to find the maximum or minimum of a function, which is useful in economic decision-making.
4. Computer Science: In computer science, polynomials play a crucial role in various algorithms and data structures. They are used in areas such as cryptography, error detection and correction, and computational geometry. For example, polynomial interpolation is used in graphics rendering to reconstruct curves or surfaces from a set of given data points.
In conclusion, the applications of polynomials are vast and diverse. They are fundamental in many scientific and technological fields, providing a powerful tool for modeling, analyzing, and solving complex problems. Understanding the properties and techniques related to polynomials is essential for success in these fields.