
When it comes to solving equations, having the answer key can make a world of difference. Whether you are a student studying for an exam or a teacher preparing a lesson plan, having the answer key provides a valuable resource for checking your work and understanding the solution process.
In the world of mathematics, equations are like puzzles waiting to be solved. They represent relationships between unknown quantities and provide a framework for finding the missing values. Whether it’s a simple linear equation or a complex quadratic equation, being able to solve equations is an essential skill for anyone working with numbers.
The answer key for solving equations serves as a guide, providing step-by-step solutions to help users navigate through the problem-solving process. It not only provides the final answer but also shows the steps involved in reaching that solution, allowing users to understand the logic and reasoning behind each step.
This answer key is especially helpful for students learning how to solve equations. It acts as a tool for self-assessment, enabling students to compare their answers with the correct ones and identify any mistakes or misunderstandings. By using the answer key, students can learn from their mistakes and improve their problem-solving skills.
In conclusion, having the answer key for solving equations is invaluable for both students and teachers. It provides a practical resource for checking answers, understanding solution processes, and improving problem-solving skills. Whether you are just starting out or looking for a refresher, the answer key can be a valuable tool in your mathematical journey.
What is Equation Solving?
Equation solving is the process of finding the values of variables that make an equation true. An equation is a mathematical statement that equates two expressions using an equal sign (=), and it typically involves unknowns or variables. The goal of equation solving is to determine the values of these unknowns that satisfy the equation.
To solve an equation, various methods and techniques can be used, depending on the complexity of the equation. The most basic method is to isolate the variable by performing operations on both sides of the equation, such as addition, subtraction, multiplication, or division. This is done to simplify the equation and ultimately obtain the value or set of values that satisfy the equation.
Equation solving is a fundamental skill in mathematics and is applied in various fields such as physics, engineering, economics, and computer science. It allows us to find solutions to real-world problems and make predictions by representing relationships and dependencies mathematically. Whether it’s determining the time it takes for an object to fall to the ground or analyzing the behavior of electrical circuits, equation solving plays a crucial role in modeling and understanding the world around us.
Key phrases:
- Equation solving
- Variables
- Unknowns
- Mathematical statement
- Isolate the variable
- Operations
- Simplify the equation
- Real-world problems
- Modeling
- Understanding
Understanding the Basics
The topic of solving equations is fundamental in mathematics. It lays the foundation for more advanced concepts as well as serves as a powerful problem-solving tool in various fields such as physics, engineering, and finance. In order to grasp the essence of solving equations, one must first understand the basics.
Solving equations involves finding the value(s) of the variable(s) that make the equation true. This is achieved by performing a series of operations, such as addition, subtraction, multiplication, and division, to isolate the variable on one side of the equation. The goal is to simplify the equation until the variable is isolated on one side and the solution is obtained by evaluating the expression on the other side.
In order to successfully solve equations, it is essential to have a solid understanding of the properties of equality. The transitive property states that if a = b and b = c, then a = c. The symmetric property states that if a = b, then b = a. The reflexive property states that a = a. These properties allow for the manipulation of equations using inverse operations to maintain equality.
There are different types of equations, including linear equations, quadratic equations, and exponential equations. Each type has its own specific method for solving, but they all rely on the fundamental principles of isolating the variable and maintaining equality. By mastering the basics of solving equations, one can confidently approach more complex mathematical problems and gain a deeper understanding of the mathematical concepts involved.
In conclusion, understanding the basics of solving equations is essential for success in mathematics and beyond. By familiarizing oneself with the properties of equality and practicing the techniques for isolating variables, one can develop a solid foundation for tackling more advanced equations and applying mathematical problem-solving in various fields.
Methods for Solving Equations
Equations are mathematical statements that express the equality of two quantities. Solving equations involves finding the values of the variables that make the equation true. There are various methods that can be used to solve equations, depending on the complexity and type of equation.
One common method for solving equations is called the “Inverse Operation Method”. This method involves using the inverse operation of the given operation to isolate the variable and solve for its value. For example, if the equation is 2x + 5 = 15, we can subtract 5 from both sides of the equation to isolate the variable x. Then, we divide both sides by 2 to find the value of x.
Another method for solving equations is the “Substitution Method”. This method involves substituting the value of one variable from one equation into another equation and solving for the other variable. For example, if we have the equations 2x + y = 5 and x – y = 3, we can solve for y in the second equation and substitute it into the first equation to find the value of x. Then, we can substitute the value of x into one of the original equations to find the value of y.
The “Graphing Method” is another approach to solving equations. This method involves graphing the equations on a coordinate plane and finding the point of intersection, which represents the solution to the equations. For example, if we have the equations y = 2x + 3 and y = -x + 5, we can graph these lines and find the point where they intersect to find the values of x and y.
In summary, there are various methods for solving equations, including the Inverse Operation Method, Substitution Method, and Graphing Method. These methods allow us to find the values of the variables that satisfy the given equations and provide solutions to mathematical problems.
Common Types of Equations
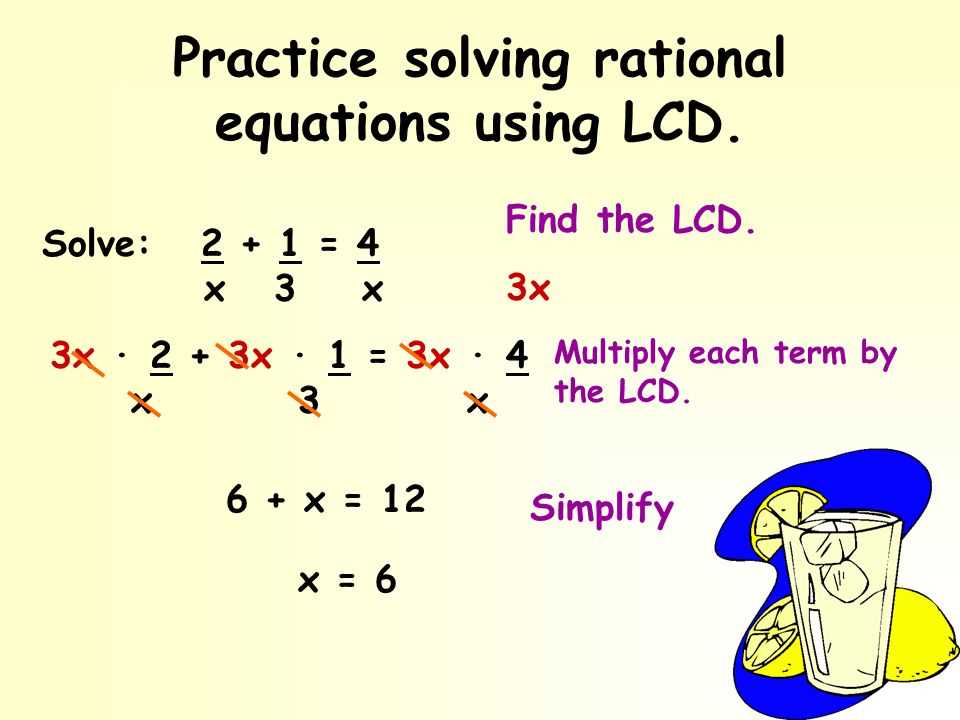
Equations are a fundamental concept in mathematics and are used to represent relationships between variables. There are several common types of equations that are frequently encountered in various fields of study. Understanding these different types of equations is essential for solving problems and finding solutions.
Linear equations are one of the most basic types of equations. These equations can be represented by a straight line when graphed and have the form of y = mx + b, where m is the slope and b is the y-intercept. Solving linear equations involves isolating the variable and finding its value.
Quadratic equations are equations of the form ax^2 + bx + c = 0, where a, b, and c are constants and x is the variable. These equations can have two real solutions, one real solution, or no real solutions at all. Quadratic equations often involve factoring, completing the square, or using the quadratic formula to find the solutions.
Exponential equations involve variables raised to a power. These equations have the form of a^x = b, where a and b are constants. Solving exponential equations typically involves taking logarithms to both sides of the equation.
Trigonometric equations involve trigonometric functions such as sine, cosine, and tangent. These equations can be solved using trigonometric identities and concepts. Trigonometric equations often have multiple solutions within a given range.
Systems of equations involve multiple equations with multiple variables. These equations represent a set of relationships between the variables and can be solved simultaneously. Various methods, such as substitution, elimination, or matrix operations, can be used to solve systems of equations.
Overall, understanding the different types of equations and their characteristics is crucial for effectively solving problems and finding solutions in various mathematical and scientific fields.
Step-by-Step Guide to Solving Equations
When it comes to solving equations, it is important to follow a step-by-step approach in order to arrive at the correct solution. This guide will walk you through the necessary steps to solve any equation, ensuring accuracy and clarity in your mathematical calculations.
Step 1: Simplify
The first step in solving an equation is to simplify both sides of the equation. This involves combining like terms and performing any necessary operations, such as adding, subtracting, multiplying, or dividing. By simplifying the equation, you reduce the complexity and make it easier to isolate the variable.
Step 2: Isolate the Variable
Once the equation has been simplified, the next step is to isolate the variable. This means getting the variable alone on one side of the equation by performing inverse operations. For example, if the variable is being added to or subtracted from another term, you would perform the opposite operation to move the variable to the other side of the equation.
Step 3: Solve for the Variable
After isolating the variable, you can now solve for it. This involves performing the necessary operations to determine the value of the variable. If the equation is linear, which means it only contains the variable to the first power, the solution will be a specific value. However, if the equation is quadratic or involves higher powers of the variable, the solution may be multiple values or even a range of values.
Step 4: Check the Solution
Once you have obtained a solution for the variable, it is important to check if it satisfies the original equation. This involves substituting the value back into the equation and performing the necessary operations to see if both sides are equal. If the substitution yields true statements, then the solution is valid. However, if the substitution does not result in a true statement, it means an error has occurred in the calculation, and you need to revisit the steps to find the correct solution.
By following this step-by-step guide, you can approach any equation with confidence and efficiently solve for the unknown variable.
Challenges in Solving Equations
Solving equations is a fundamental skill in mathematics that is necessary for various applications, such as finding unknown values or understanding the relationships between different variables. However, it is not always an easy task and can present several challenges that need to be overcome.
Complexity of Equations: One of the main challenges in solving equations is the complexity of the equations themselves. Equations can vary in terms of their complexity, ranging from simple linear equations to more complicated quadratic, exponential, or trigonometric equations. Dealing with equations that involve multiple variables or contain logarithms or radicals can further increase the complexity and difficulty of finding solutions.
Lack of a Clear Strategy: Another challenge in solving equations is the lack of a clear strategy or method to approach them. While there are various techniques and methods available, such as substitution, elimination, or factoring, determining the most appropriate approach for a particular equation can be challenging. It requires a deep understanding of the mathematical concepts and the ability to analyze the equation to identify the most efficient strategy for solving it.
Errors and Mistakes: Solving equations involves a series of calculations and manipulations, which increases the likelihood of making errors or mistakes. Whether it’s a simple arithmetic calculation mistake or a misstep in the algebraic manipulations, even a small error can lead to incorrect solutions and misinterpretations of the equation. It is essential to double-check the calculations and follow a systematic approach to minimize errors and ensure accurate solutions.
Real-Life Applications: Applying equations to real-life scenarios can also pose challenges. Real-life problems often involve interpreting word problems and translating them into mathematical equations. Understanding the context and identifying the relevant information can be challenging, as well as determining the appropriate mathematical model to represent the problem accurately. Consequently, solving these equations requires not only mathematical skills but also critical thinking and problem-solving abilities.
Number of Solutions: Another challenge is dealing with equations that have multiple solutions or no solution at all. Some equations may have infinitely many solutions, while others may not have any real solutions. It is important to understand the nature of the equation and interpret the solutions in the appropriate context to avoid any misinterpretation or misunderstanding of the problem.
In conclusion, solving equations presents various challenges, including the complexity of the equations, the lack of a clear strategy, the potential for errors and mistakes, the application to real-life scenarios, and dealing with equations that have multiple or no solutions. Overcoming these challenges requires a strong mathematical foundation, critical thinking skills, and practice in applying different techniques and strategies to solve equations efficiently and accurately.
Solving Equations Answer Key

In this article, we have explored the concept of solving equations and provided a step-by-step guide on how to solve various types of equations. We have covered linear equations, quadratic equations, and systems of equations.
Linear Equations:
A linear equation is an equation that can be written in the form ax + b = c, where a, b, and c are constants. To solve a linear equation, we isolate the variable on one side of the equation using inverse operations. The answer key provides the step-by-step solution of various linear equations.
Quadratic Equations:
A quadratic equation is an equation that can be written in the form ax^2 + bx + c = 0, where a, b, and c are constants. Solving quadratic equations involves factoring, completing the square, or using the quadratic formula. The answer key presents the solved quadratic equations using different methods.
Systems of Equations:
A system of equations is a set of equations with the same variables. Solving a system of equations involves finding the values of the variables that satisfy all the equations simultaneously. There are different methods to solve systems of equations, such as substitution, elimination, and graphing. The answer key demonstrates the solution of systems of equations using these methods.
This answer key serves as a valuable resource for students and individuals who need assistance in solving equations. It provides comprehensive solutions to various equations, enabling readers to understand the process and apply it to their own problems.
Summary:
The solving equations answer key presented in this article offers step-by-step solutions to linear equations, quadratic equations, and systems of equations. By following the provided solutions, individuals can develop a deeper comprehension of these mathematical concepts and enhance their problem-solving skills. Whether solving equations for homework or preparing for an exam, this answer key is a helpful reference.