In mathematics, order of operations is a set of rules that dictate the sequence in which mathematical operations should be performed. Without proper understanding and adherence to these rules, the result of a mathematical expression may be incorrect. Therefore, it is crucial to follow the correct order of operations to ensure accuracy in mathematical calculations.
The order of operations is commonly remembered using the acronym PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). This acronym helps students and professionals alike in correctly solving mathematical expressions.
When dealing with complex mathematical expressions, it is important to prioritize operations enclosed within parentheses or brackets first. Next, any exponents or powers should be evaluated. After that, multiplication and division should be performed from left to right. Finally, addition and subtraction should be carried out from left to right.
The correct order of operations is essential in providing a standardized method for solving mathematical problems. It eliminates confusion and ensures that everyone arrives at the same result when solving the same expression. Understanding and applying the order of operations accurately is a fundamental skill for success in mathematics.
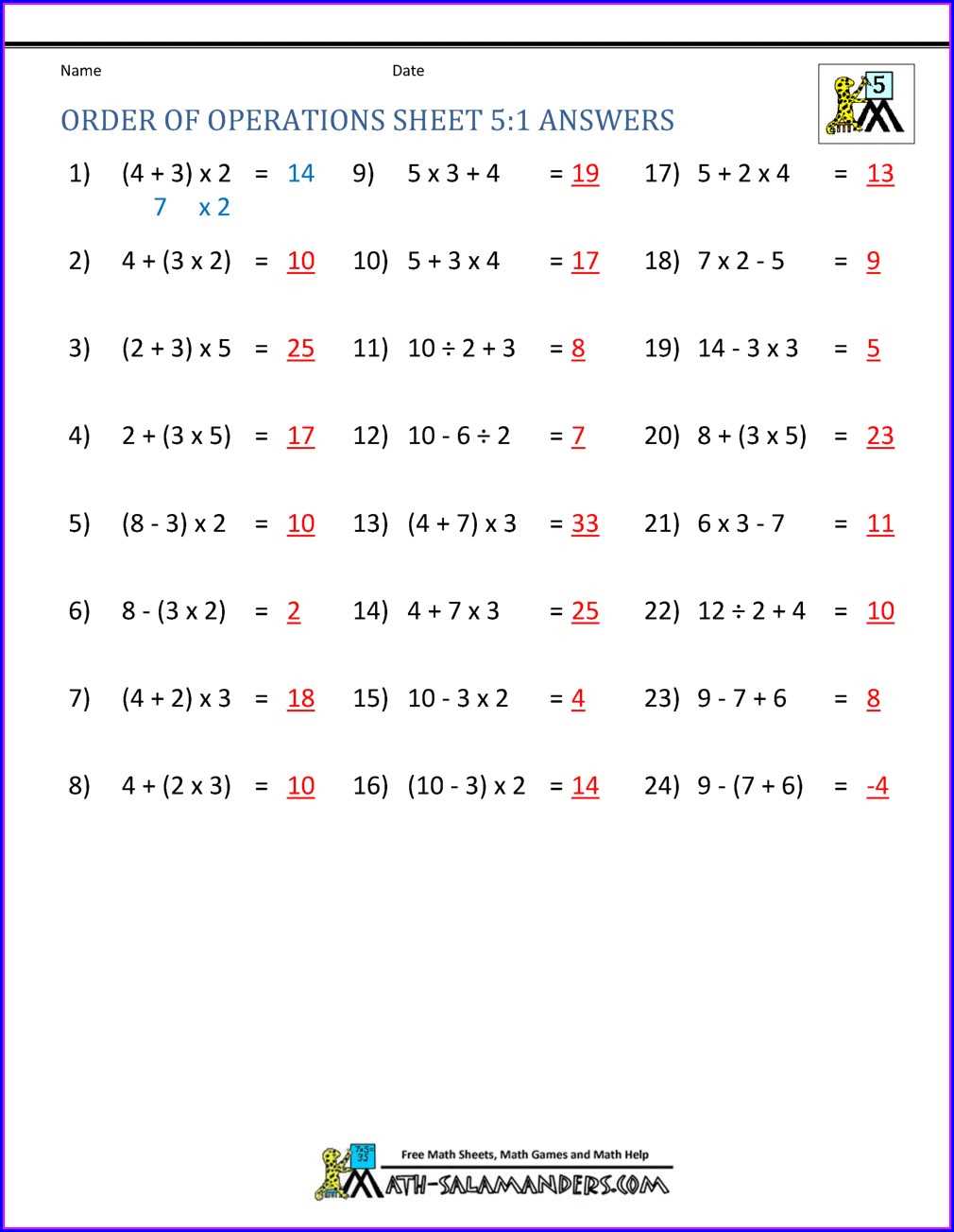
Order of Operations: Answer Key to 1-2 Problems
In mathematics, the order of operations refers to the specific order in which mathematical operations should be performed in a calculation. This is important to ensure that calculations are carried out accurately and consistently. The answer key to 1-2 problems provides the correct answers to the mathematical equations in the 1-2 problem set.
The order of operations, also known as PEMDAS (Parentheses, Exponents, Multiplication and Division from left to right, Addition and Subtraction from left to right), helps to clarify which operations should be performed first in a calculation. By following the order of operations, mathematicians can avoid confusion and ensure that everyone arrives at the same correct answer.
The answer key to 1-2 problems provides the correct answers to a set of mathematical equations that involve addition, subtraction, multiplication, and division. The answer key helps students and teachers check their work and ensure they have correctly followed the order of operations. It can also be a valuable resource for studying and practicing the correct application of the order of operations.
Using the answer key to 1-2 problems, students can compare their own answers to the correct answers and identify any mistakes they may have made. This helps to reinforce the understanding of the order of operations and the importance of following it. Teachers can use the answer key to assess students’ understanding of the concept and provide targeted feedback and guidance.
In conclusion, the answer key to 1-2 problems is a useful tool for both students and teachers to ensure the correct application of the order of operations in mathematical calculations. By checking their work against the correct answers, students can identify and correct any mistakes, while teachers can assess students’ understanding and provide targeted support. It is an essential resource for mastering the order of operations and achieving accuracy in mathematical calculations.
The Basics of Order of Operations
In mathematics, the order of operations refers to the specific rules that dictate the order in which mathematical operations should be performed. These rules ensure consistency in mathematical expressions and prevent ambiguity. Without following the order of operations, the same mathematical expression could yield different results depending on how the operations are grouped and evaluated.
The acronym PEMDAS is commonly used to remember the order of operations:
- P: Parentheses (or brackets)
- E: Exponents (or powers)
- M: Multiplication (or division)
- D: Division (or multiplication)
- A: Addition
- S: Subtraction
To illustrate how the order of operations works, let’s consider the following example:
6 + 2 * 3 – (8 / 4)
Using the order of operations, we start by evaluating the part of the expression inside the parentheses first:
6 + 2 * 3 – 2
Next, we perform any multiplication or division from left to right:
6 + 6 – 2
Finally, we perform any remaining addition or subtraction from left to right:
12 – 2 = 10
Therefore, the answer to the original expression is 10.
By following the order of operations, we can ensure that mathematical expressions are evaluated consistently and accurately, leading to the correct results.
Understanding PEMDAS
The acronym PEMDAS stands for Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). It is a set of rules used to determine the order in which mathematical operations should be performed to solve an expression or equation.
Using PEMDAS correctly is essential in order to obtain the correct answer and avoid confusion. By following the order of operations, you ensure that each operation is performed in the correct sequence. Let’s break down each component of PEMDAS:
- Parentheses: Any calculations inside parentheses must be done first. This allows you to simplify expressions within parentheses and treat them as a single value.
- Exponents: After dealing with parentheses, you need to solve any exponents or powers next. This involves raising numbers to a certain power or taking the square root.
- Multiplication and Division: Once parentheses and exponents have been addressed, you proceed with multiplying and dividing from left to right. These operations have the same priority and can be performed interchangeably.
- Addition and Subtraction: Finally, the last step is to perform addition and subtraction from left to right. Just like multiplication and division, these operations have the same priority and can be done interchangeably.
By understanding and applying PEMDAS correctly, you can solve complex mathematical expressions with confidence and accuracy. It provides a clear and consistent set of rules to guide your calculations and ensure that you arrive at the correct answer. Remember to always start with parentheses, then exponents, followed by multiplication and division, and finish with addition and subtraction. Following these steps will help you avoid common mistakes and maintain mathematical integrity in your calculations.
Applying Order of Operations to Arithmetic Expressions
In mathematics, order of operations determines the sequence in which different mathematical operations should be performed to obtain the correct answer. The order of operations is often abbreviated as “PEMDAS” or “BODMAS”. The letters in these acronyms stand for different mathematical operations: parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right).
When solving arithmetic expressions, it is crucial to follow the order of operations to ensure accuracy. This means that parentheses must be evaluated first, followed by exponents, and then multiplication and division from left to right, and finally addition and subtraction from left to right.
To illustrate the importance of order of operations, let’s consider the expression 2 + 5 * 3. If we simply solve it from left to right, we would get the result 7 * 3 = 21. However, using the order of operations, we should first multiply 5 and 3 to get 15, and then add 2 to obtain the correct answer of 17.
Properly applying the order of operations becomes even more important when dealing with complex expressions that involve multiple operations and parentheses. By following the predefined order of operations, mathematicians can ensure that their calculations are consistent and accurate.
- Parentheses: Evaluate expressions within parentheses first.
- Exponents: Calculate any exponents or square roots.
- Multiplication and Division: Perform multiplication and division from left to right.
- Addition and Subtraction: Perform addition and subtraction from left to right.
Following these rules allows mathematicians to simplify complex expressions and solve mathematical problems correctly. It is crucial to remember the order of operations to avoid mistakes and ensure accurate results in mathematics.
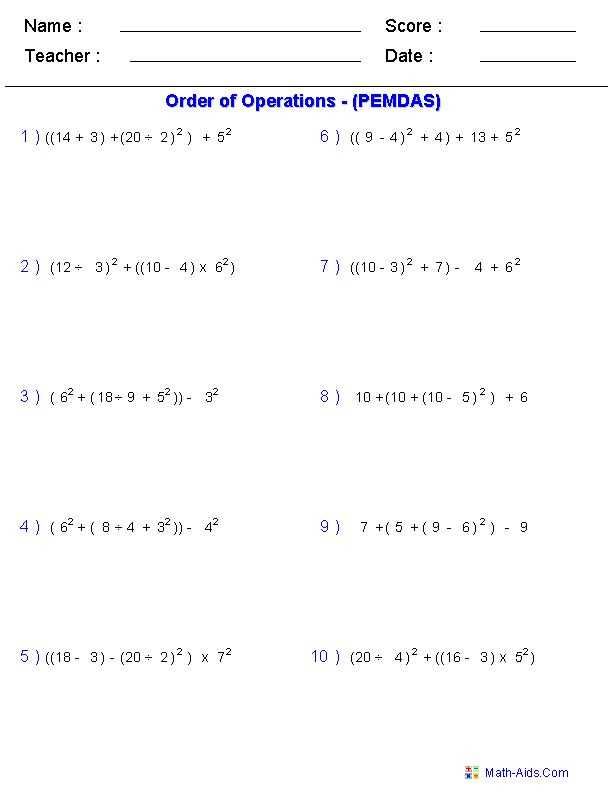
Solving Complex Equations using Order of Operations
When faced with complex equations, it is essential to tackle them in a systematic manner. The order of operations provides a clear framework for solving equations accurately. By following this set of rules, one can simplify even the most intricate equations.
The first step in solving complex equations is to evaluate any expressions within parentheses. This ensures that calculations are performed on the most basic level before proceeding. For example, in the equation 2 + (3 * 4), we would first multiply 3 by 4 to get 12, and then add 2 to obtain the final answer of 14.
After dealing with parentheses, the next step is to address any exponents or square roots. This involves raising numbers to a given power or taking the square root of a number. By resolving these operations, the equation becomes more simplified and easier to work with.
Following the evaluation of exponents, the next step is to perform multiplication and division from left to right. This means that if there are multiple multiplication or division operations in an equation, they should be done in the order they appear. By doing so, we ensure that the equation is solved accurately, giving us the correct answer.
The final step to solving complex equations is to handle addition and subtraction operations. Similar to the previous step, these operations should be performed from left to right, ensuring that the equation is solved in the correct order. By adhering to the order of operations, we can eliminate any ambiguity and confidently arrive at the correct solution.
Common Mistakes in Order of Operations
When it comes to solving math problems using the order of operations, there are several common mistakes that students often make. One of the most frequent errors is forgetting to use parentheses correctly. Parentheses are used to group operations together, and it is important to follow the order of operations within each set of parentheses before moving on to other operations. For example, in the expression 3 + (5 * 2), the multiplication should be done before the addition, resulting in a final answer of 13. However, students may often overlook this rule and perform the addition first, leading to an incorrect answer.
Another common mistake is applying the order of operations incorrectly to expressions with exponentiation or square roots. According to the order of operations, these operations should be performed before multiplication or division. For instance, in the expression 2 + 3^2, the exponentiation should be done first, resulting in a final answer of 11. However, students may mistakenly perform the addition before the exponentiation and arrive at an incorrect answer of 25.
Students also frequently make errors by neglecting to perform multiplication or division from left to right. According to the order of operations, these operations should be performed in the order they appear from left to right. For example, in the expression 6 / 2 * 3, the division should be done first, resulting in a final answer of 9. However, students may incorrectly perform the multiplication first and end up with an incorrect answer of 1.
Overall, understanding and applying the order of operations correctly is essential for solving math problems accurately. By being aware of common mistakes, such as mishandling parentheses, misapplying the order of operations with exponentiation or square roots, or neglecting to perform multiplication or division from left to right, students can improve their problem-solving skills and achieve better results in their math studies.
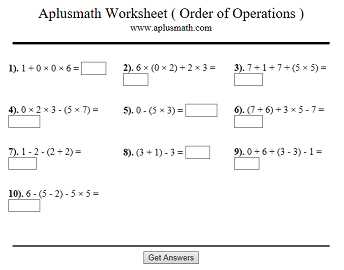
Practice Problems and Answer Key
To solidify your understanding of the order of operations, here are some practice problems for you to solve. Use the knowledge and rules discussed in the previous sections to correctly solve each problem.
Practice Problems:

- Simplify the expression: 5 + 3 * 2
- Simplify the expression: 8 – 2 * 4 + 3
- Simplify the expression: (6 + 2) * (4 – 1)
- Simplify the expression: 10 / 2 + 3 * 5 – 2
- Simplify the expression: 4 + 5 * (3 – 2) / 2
Now that you have attempted the practice problems, let’s check your answers!
Answer Key:
- 5 + 3 * 2 = 11
- 8 – 2 * 4 + 3 = 3
- (6 + 2) * (4 – 1) = 24
- 10 / 2 + 3 * 5 – 2 = 21
- 4 + 5 * (3 – 2) / 2 = 9.5
By correctly following the order of operations, you should have arrived at the given answers. Practicing these problems will help reinforce your understanding and make solving complex expressions easier in the future. Remember to always use parentheses when necessary and work from left to right when evaluating expressions with the same level of precedence.