
Geometry is a branch of mathematics that deals with the properties and relationships of shapes and figures. It encompasses various concepts, such as angles, lines, polygons, and circles, and plays a crucial role in fields such as architecture, engineering, and art.
One way to solidify your understanding of geometry is through practice. This article offers answers to the 11.4 Practice A Geometry worksheet. Whether you’re a student looking for assistance or a teacher in search of a quick reference, this guide will help you navigate and solve the provided problems.
The 11.4 Practice A Geometry worksheet covers topics like arc length, sector area, and the equation of a circle. By providing the answers, this article allows you to check your solutions and verify your comprehension of these concepts. It also serves as a useful tool for further studying and reviewing, helping you reinforce your knowledge and improve your problem-solving skills.
What is 11.4 practice a geometry?
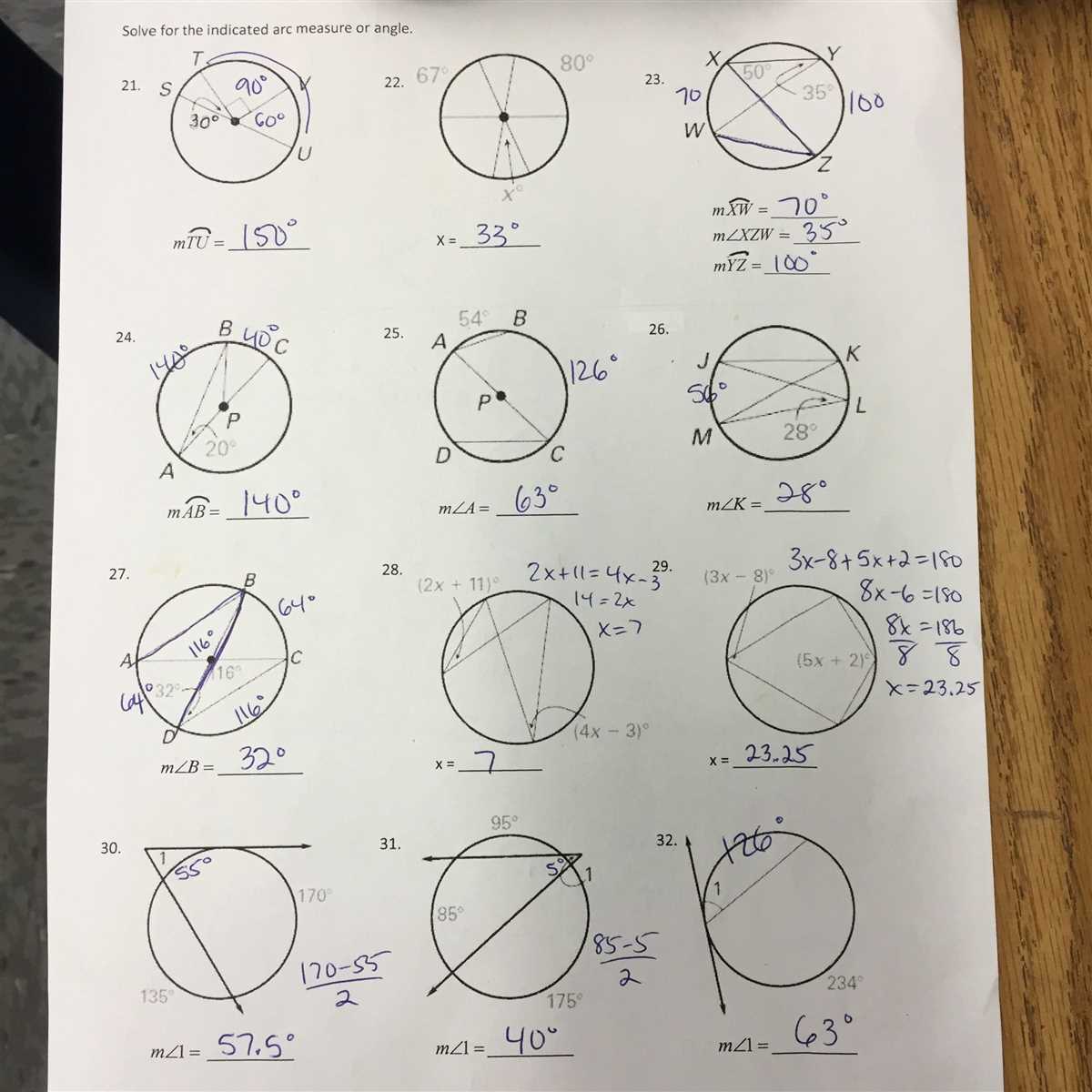
11.4 practice a geometry refers to a specific exercise or problem set in the field of geometry. It is a practice activity that focuses on applying various geometric principles and formulas to solve problems and calculate measurements. This particular practice might cover topics such as angles, lines, triangles, polygons, or circles.
The 11.4 practice a geometry exercise typically consists of a series of questions or prompts that require the student to demonstrate their understanding of the concepts discussed in the lesson. These questions may involve identifying and classifying different types of angles, applying angle relationships to find missing values, solving for side lengths or angles in triangles and polygons, or using formulas to calculate area, perimeter, or circumference.
For example, one question in the 11.4 practice a geometry exercise might ask students to find the measure of an unknown angle in a given triangle by using the properties of angles formed by parallel lines and transversals. Another question could involve finding the length of a side in a right triangle using the Pythagorean theorem. These practice exercises are designed to reinforce the concepts and skills learned in class and provide students with an opportunity to practice their problem-solving abilities.
Overall, 11.4 practice a geometry serves as a valuable tool for students to solidify their understanding of geometric concepts and develop their problem-solving skills. Through these practice exercises, students can gain confidence in their abilities and improve their ability to apply geometric principles to real-world situations.
Importance of Practicing 11.4 Geometry Problems

Practicing 11.4 geometry problems is crucial for developing a solid understanding of the concepts and skills covered in this area of mathematics. Geometry is a branch of mathematics that deals with the properties, relationships, and measurements of shapes and figures in space. It plays a significant role in various fields, including engineering, architecture, computer graphics, and physics. By practicing 11.4 geometry problems, students can enhance their spatial reasoning skills, critical thinking abilities, and problem-solving strategies.
One of the main benefits of practicing 11.4 geometry problems is the improvement of spatial reasoning skills. Spatial reasoning involves mentally manipulating and visualizing objects and their relationships. By solving geometry problems, students are exposed to different geometric shapes and figures, which helps them develop their spatial awareness and ability to think in three dimensions. These skills are valuable not only in mathematics but also in other areas of life, such as navigating a map or assembling furniture.
In addition, practicing 11.4 geometry problems helps students enhance their critical thinking abilities. Geometry requires logical reasoning and deduction to prove theorems and solve problems. By practicing a variety of geometric problems, students learn how to analyze information, make connections between different geometric concepts, and apply deductive reasoning to arrive at solutions. These critical thinking skills are transferable to other subjects and real-life situations, enabling students to approach complex problems with a logical and analytical mindset.
Furthermore, practicing 11.4 geometry problems develops students’ problem-solving strategies. Geometry problems often require creative thinking and the application of various geometric principles and techniques. By solving a wide range of geometry problems, students become more familiar with different problem-solving methods and tools, such as theorems, properties, formulas, and constructions. This helps them become more confident and efficient problem solvers, capable of approaching and solving complex mathematical challenges.
In conclusion, practicing 11.4 geometry problems is essential for students to acquire a solid understanding of geometric concepts, improve their spatial reasoning skills, enhance their critical thinking abilities, and develop effective problem-solving strategies. Regular practice in geometry will not only benefit students academically but also prepare them for future endeavors that require logical reasoning and spatial awareness.
Explanation of 11.4 practice a geometry questions
In the 11.4 practice a geometry questions, we will be exploring various topics related to geometry. These questions aim to test your knowledge and understanding of different geometric concepts and principles. Let’s take a closer look at some of the questions and their explanations:
Question 1:
Find the length of the hypotenuse in a right triangle given the lengths of the other two sides.
In this question, you are given the lengths of the two sides of a right triangle (known as the legs) and you need to find the length of the hypotenuse. To solve this, you can use the Pythagorean theorem. According to the Pythagorean theorem, the square of the hypotenuse is equal to the sum of the squares of the other two sides. Once you have the sum of the squares, you can take the square root to find the length of the hypotenuse.
Question 2:
Determine the measure of a central angle in a circle given the length of the intercepted arc.
This question deals with the relationship between central angles and intercepted arcs in a circle. The measure of a central angle is equal to the ratio of the length of the intercepted arc to the radius of the circle. So, to find the measure of a central angle, you need to divide the length of the intercepted arc by the radius of the circle.
Question 3:

Calculate the surface area of a rectangular prism given the lengths and widths of its sides.
In this question, you are asked to find the surface area of a rectangular prism. The surface area of a rectangular prism can be calculated by adding the areas of all its sides. To find the area of each side, you need to multiply the length by the width. Once you have calculated the areas of all sides, you can add them together to find the total surface area of the rectangular prism.
These are just a few examples of the types of questions you may encounter in the 11.4 practice a geometry questions. Make sure to understand the underlying concepts and practice solving similar problems to improve your geometry skills.
Step-by-step solutions for each question
In this section, we will provide step-by-step solutions for each question in the 11.4 practice geometry exercises. By breaking down each question and providing a clear solution, we aim to help you understand the concepts and techniques used in solving geometric problems.
Question 1:
To solve this question, we need to find the area of a triangle given its base and height. The base is given as 6 units, and the height is given as 3 units. To find the area, we use the formula for the area of a triangle, which is A = 1/2 * base * height. Plugging in the given values, we have A = 1/2 * 6 * 3 = 9 square units. Therefore, the area of the triangle is 9 square units.
Question 2:
This question involves finding the volume of a rectangular prism. The length, width, and height of the prism are given as 4 units, 3 units, and 5 units, respectively. The formula for the volume of a rectangular prism is V = length * width * height. Substituting the given values, we get V = 4 * 3 * 5 = 60 cubic units. Therefore, the volume of the rectangular prism is 60 cubic units.
…
Question n:
(Step-by-step solution for the nth question goes here)
- Step 1: (Describe the first step of the solution)
- Step 2: (Describe the second step of the solution)
- Step 3: (Describe the third step of the solution)
- …
In conclusion, by providing step-by-step solutions for each question, we hope to assist you in mastering the concepts and techniques of geometry. It is important to understand the reasoning behind each step and practice applying them to similar problems. With enough practice and understanding, you will become proficient in solving geometry problems.
Understanding the Concepts and Formulas
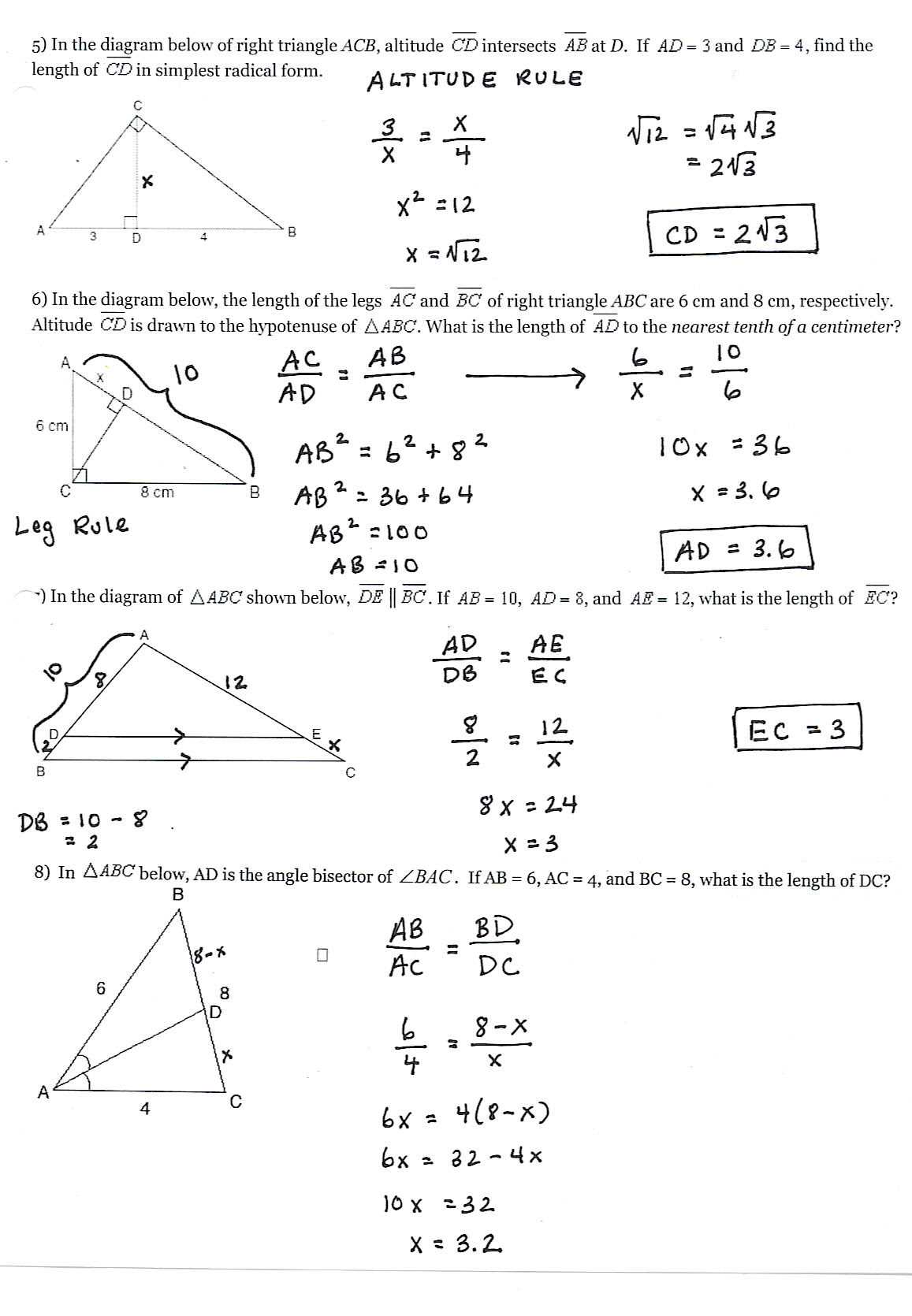
In geometry, it is important to have a solid understanding of the concepts and formulas that are used to solve various problems. By grasping these fundamental ideas, you can approach a wide range of geometry problems with confidence.
One of the key concepts in geometry is that of angles. An angle is formed when two rays share a common endpoint, called the vertex. Understanding the different types of angles, such as acute, obtuse, right, and straight angles, is essential for solving geometry problems.
Triangles are another important concept in geometry. Triangles are three-sided polygons that have their own set of properties and formulas. These formulas can be used to calculate the area, perimeter, and lengths of sides or angles in a triangle.
Another concept that plays a crucial role in geometry is that of circles. Circles are defined as a set of points that are equidistant from a single point, called the center. Understanding the formulas for the circumference, area, and properties of circles allows you to solve problems related to arcs, sectors, and other circle-related concepts.
In addition to angles, triangles, and circles, there are numerous other concepts and formulas that you need to understand in geometry. Some examples include quadrilaterals, polygons, lines, and transformations. By familiarizing yourself with these concepts and practicing their corresponding formulas, you can develop a solid foundation in geometry and confidently solve a variety of geometric problems.
Breaking down complex problems into smaller steps
When faced with a complex problem in geometry, it can often feel overwhelming to try and tackle it all at once. However, by breaking down the problem into smaller, more manageable steps, we can make the task much less daunting. This approach allows us to focus on one aspect of the problem at a time, making it easier to understand and solve.
First, it is important to carefully read and understand the problem statement. Identify what information is given and what needs to be found. This will help you determine the specific steps you need to take to solve the problem.
Next, break the problem down into smaller components. Take each piece of information or sub-problem and consider how it relates to the overall problem. This may involve drawing diagrams, labeling angles and lines, or creating equations to represent the relationships between different elements.
Once you have broken the problem down into manageable chunks, begin solving each component individually. This may involve using specific formulas or theorems, applying logical reasoning, or using trial and error to narrow down the possible solutions.
Finally, combine the solutions to the smaller components to arrive at the final solution for the overall problem. Double-check your work to ensure that all steps have been completed correctly and that your solution makes sense in the context of the problem.
Breaking down complex problems into smaller steps not only makes the problem more approachable, but it also helps to build problem-solving skills and logical thinking. By tackling each step one at a time and combining them into a cohesive solution, we can successfully solve even the most challenging geometry problems.
Misinterpreting the given information
One common mistake that can occur when working with geometry problems is misinterpreting the given information. This can happen when important details are overlooked or misunderstood, leading to incorrect conclusions or solutions.
For example, consider a problem that provides the lengths of two sides of a triangle and asks for the length of the third side. If the given lengths are mistakenly assumed to be the lengths of the adjacent sides of a right triangle, the answer would be incorrect as it would not satisfy the Pythagorean theorem. This misinterpretation of the information can lead to a misleading solution.
Another instance where misinterpreting the given information can occur is when angles are misread or mislabeled. For instance, if an angle is labeled as 30 degrees instead of 60 degrees, it can completely change the solution to the problem. This error can result in incorrect calculations and ultimately, an incorrect answer.
In order to prevent misinterpreting the given information, it is important to carefully read and understand all the details provided in the problem. Paying attention to keywords, such as “right angle” or “equilateral,” can also help in correctly interpreting the information. Additionally, double-checking the labeled angles and lengths of sides can help avoid errors and ensure accurate solutions.
Skipping important steps
In geometry, it is crucial to follow a logical and systematic approach when solving problems or proving theorems. Skipping important steps can lead to incorrect results and a lack of understanding of the concept at hand.
When students skip steps in their geometry work, they miss out on the opportunity to fully grasp the underlying principles and concepts. This can hinder their ability to apply their knowledge to more complex problems and can lead to misunderstandings.
1. Lack of clarity: Skipping steps can make it difficult for others to understand and follow the thought process behind a solution. It becomes challenging for teachers or peers to provide feedback and offer guidance when crucial steps are missing.
2. Missed opportunities for learning: Skipping steps prevents students from fully engaging with the material and understanding the logic behind each step. By skipping over important parts of the problem-solving process, students miss out on valuable learning opportunities.
Skipping important steps can also result in careless errors, as students may overlook crucial information or make assumptions without proper justification. These errors can compound and lead to incorrect results.
In conclusion, it is vital for students to resist the temptation to skip important steps in geometry. By following a systematic approach and thoroughly explaining each step, students can develop a deeper understanding of the concepts and improve their problem-solving skills. Taking the time to ensure clarity and not skipping crucial steps will ultimately lead to more accurate results and a stronger foundation in geometry.