
Geometry is a fascinating branch of mathematics that deals with the properties and relationships of shapes, lines, angles, and more. It plays a crucial role in various fields such as architecture, engineering, art, and even nature. Understanding geometry not only sharpens your analytical skills but also allows you to make sense of the world around you.
In this article, we will delve into 12.2 Practice A Geometry Answers, a set of exercises designed to challenge your geometric knowledge and problem-solving abilities. Whether you’re a student looking for practice materials or someone keen on brushing up on their geometric skills, these questions and answers will serve as a valuable resource.
12.2 Practice A Geometry Answers covers a variety of topics, including congruent triangles, angle bisectors, the Pythagorean theorem, and more. By going through these exercises and their corresponding answers, you will gain a deeper understanding of these concepts and be able to apply them in real-world scenarios.
So, if you’re ready to unlock the secrets of geometry and strengthen your mathematical prowess, let’s dive into the world of 12.2 Practice A Geometry Answers. By the end of this journey, you’ll not only have mastered these particular exercises but also developed a solid foundation in geometry that will serve you well throughout your academic and professional journey.
Understanding the Importance of Practice in Geometry
Geometry is a branch of mathematics that deals with the properties, shape, size, and relationships of figures and spaces. It is a subject that requires logical thinking, visualization, and problem-solving skills. To truly understand and excel in geometry, practice plays a crucial role.
Practice allows students to reinforce their understanding of geometric concepts and develop their problem-solving abilities. By solving a variety of problems, students can apply the knowledge they have learned in class and gain a deeper understanding of the subject. They can also identify any areas where they may be struggling and work on improving their skills in those specific areas.
Moreover, practicing geometry helps students develop their spatial reasoning skills. Through repeated practice, they can learn to visualize and manipulate geometric shapes in their minds, which is an essential skill not only in geometry but also in various real-life situations. For example, architects and engineers often rely on their spatial reasoning abilities when designing buildings or structures.
Regular practice in geometry also helps students build confidence in their abilities. By solving a wide range of problems, students gain confidence in their problem-solving skills and become more comfortable applying their knowledge in different contexts. This confidence can then extend to other areas of their academic and personal lives, as they recognize that they are capable of overcoming challenges and finding solutions.
In conclusion, practice is an integral component of learning and mastering geometry. It allows students to reinforce their understanding, develop problem-solving skills, enhance spatial reasoning abilities, and build confidence. By dedicating time to regular practice, students can unlock their full potential in geometry and apply their knowledge to various real-life situations.
Key Concepts and Skills Covered in Practice A
In Practice A of the geometry section, several key concepts and skills are covered to enhance the understanding of students in the subject. These concepts and skills are essential in solving various problems related to geometry.
1. Identifying congruent figures and their corresponding parts:
The practice questions in this section require students to identify congruent figures and their corresponding parts. This helps in developing spatial awareness and the ability to recognize patterns and symmetries in geometric shapes.
2. Using properties of congruent figures:
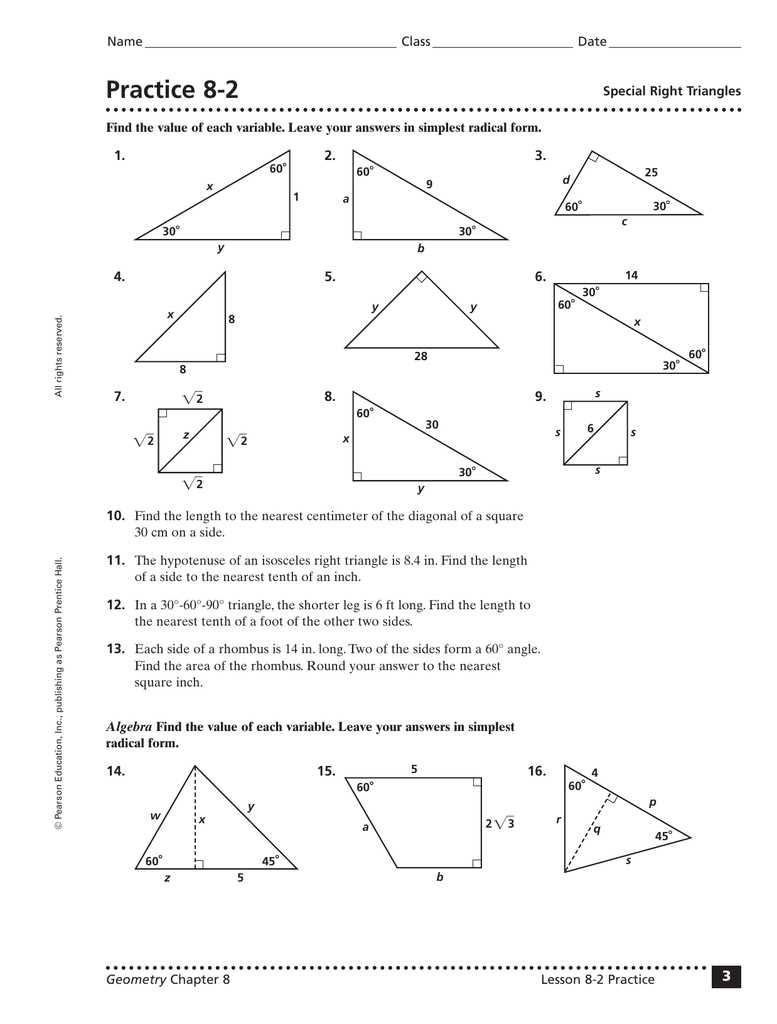
Students will also learn to apply the properties of congruent figures to solve problems. This involves using the information about one figure to determine the properties of another congruent figure, such as angles, side lengths, and diagonals.
3. Solving problems involving angle relationships:
The practice questions cover various types of angle relationships, including supplementary, complementary, vertical, and adjacent angles. Students will develop the skills to identify these relationships and use them to solve problems involving angles.
4. Applying the Pythagorean theorem:
The Pythagorean theorem is a fundamental concept in geometry. Practice A includes questions that require students to apply the theorem to find missing side lengths in right triangles. This skill is important in solving real-world problems involving distances and measurements.
5. Understanding transformations:
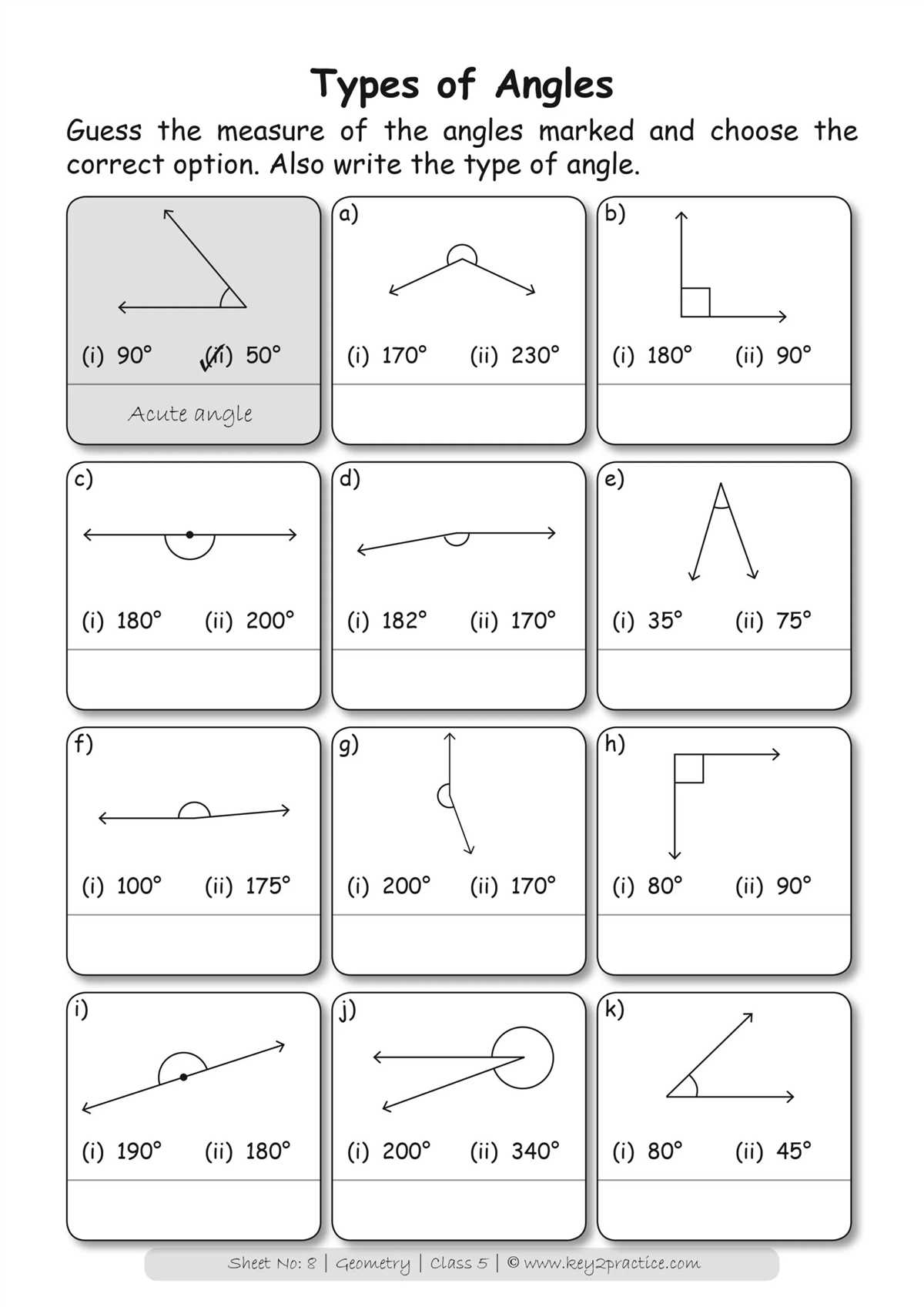
Transformations, such as translations, reflections, and rotations, are covered in this practice set. Students will learn to identify and describe these transformations and apply them to determine the coordinates of the transformed figures.
Overall, Practice A focuses on developing the fundamental skills required to solve geometry problems, including the recognition of congruent figures, angle relationships, the Pythagorean theorem, and transformations. Mastering these concepts and skills will provide a strong foundation for further exploration and understanding in the field of geometry.
Step-by-Step Solutions for Practice A Problems
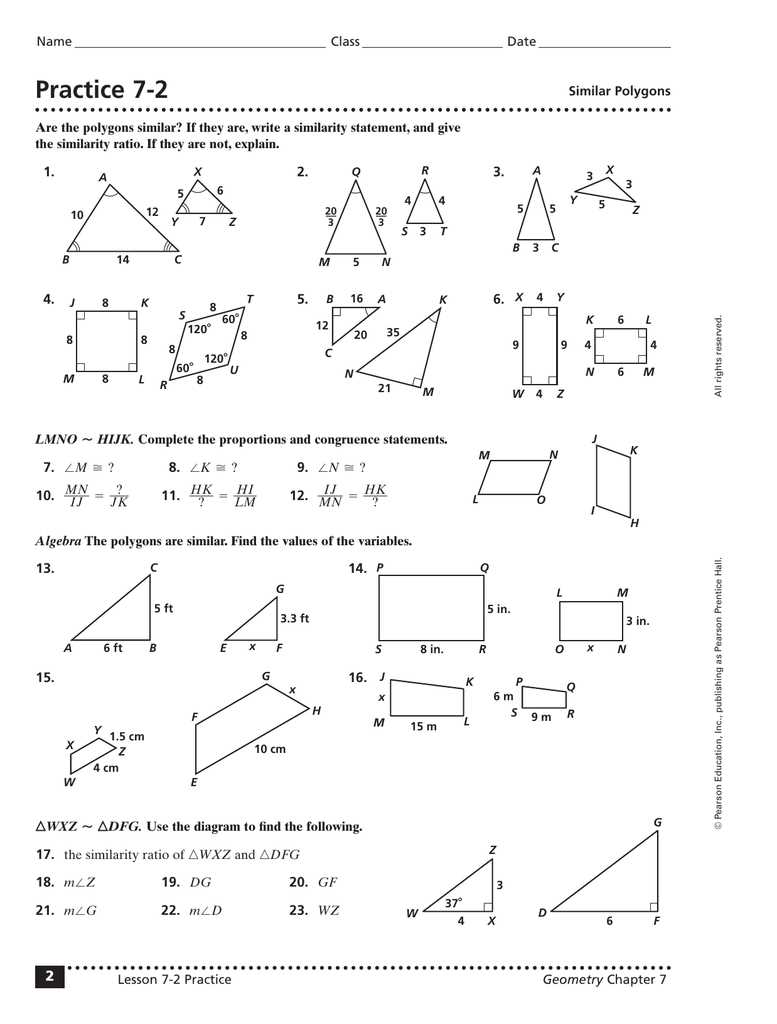
Below are step-by-step solutions for the Practice A problems in Geometry:
Problem 1:
- Given a triangle ABC with sides AB = 4, BC = 5, and AC = 6.
- Using the Law of Cosines, we can find the measure of angle ABC.
- First, we calculate the square of side AC: AC^2 = 6^2 = 36.
- Next, we calculate the square of side BC: BC^2 = 5^2 = 25.
- Finally, we calculate the square of side AB: AB^2 = 4^2 = 16.
- Now, we can use the Law of Cosines to find the measure of angle ABC: cos(ABC) = (25 + 16 – 36) / (2 * 4 * 5) = 5 / 8.
- Taking the inverse cosine of 5 / 8, we find that the measure of angle ABC is approximately 38.66 degrees.
Problem 2:
- Given a rectangle with length 10 and width 5.
- To find the perimeter of the rectangle, we add up all four sides: 10 + 5 + 10 + 5 = 30.
- So, the perimeter of the rectangle is 30 units.
- To find the area of the rectangle, we multiply the length by the width: 10 * 5 = 50.
- So, the area of the rectangle is 50 square units.
These step-by-step solutions show the process for solving each problem and provide the final answer. By following these steps, you can practice your problem-solving skills and improve your understanding of geometry concepts.
Common Mistakes to Avoid in Practice A
When it comes to practicing geometry, there are several common mistakes that students often make in Practice A. These mistakes can hinder their understanding of the concepts and hinder their ability to solve problems accurately. Here are some of the common mistakes to avoid:
- Forgetting the properties and formulas: One common mistake is forgetting the properties and formulas related to angles, triangles, and other geometric shapes. It’s important to review these properties and formulas before attempting Practice A to ensure a solid understanding.
- Not properly labeling diagrams: Another mistake is failing to properly label diagrams. In geometry, accurately labeling points, lines, and angles is crucial for the correct application of theorems and formulas. Students should take their time to label all relevant parts of the diagram.
- Skipping steps in solving problems: Many students have a tendency to skip steps when solving problems, especially if they think they can arrive at the correct answer quickly. However, this can lead to errors and mistaken conclusions. It’s important to show all the steps and explanations clearly, so that the solution can be easily understood.
- Not double-checking calculations: It’s crucial to double-check calculations and mathematical operations throughout the problem-solving process. Small errors in arithmetic can lead to incorrect final answers. Taking a moment to review calculations can help identify and correct any mistakes.
- Not reading the problem carefully: Misinterpreting the problem statement is a common mistake that can lead to incorrect solutions. It’s important to read the problem carefully, identify the given information, and understand what is being asked before attempting to solve it.
Avoiding these common mistakes can significantly improve students’ performance in Practice A. By reviewing the properties and formulas, labeling diagrams accurately, showing all steps, double-checking calculations, and reading the problem carefully, students can enhance their understanding of geometry and improve their problem-solving skills.
How Practice A Can Improve Your Geometry Skills
Geometry is a branch of mathematics that deals with shapes, sizes, and properties of figures and spaces. It is an essential subject that is included in school curricula and is used in various fields such as architecture, engineering, and design. To excel in geometry, it is important to practice regularly to enhance your understanding and skills. Practice A is a valuable resource that can significantly improve your geometry proficiency. Here are some ways in which Practice A can benefit you:
1. Reinforce Concepts
Practice A provides a platform to reinforce and apply the concepts learned in geometry. By solving a wide range of exercises and problems, you can solidify your understanding of geometric principles, formulas, and theorems. This repetitive practice helps to build a strong foundation and enhances your ability to solve complex geometrical problems.
2. Enhance Problem-Solving Skills
Geometry requires logical thinking and problem-solving skills. Practice A offers a variety of challenging problems that require critical thinking, analysis, and application of geometric concepts. By regularly practicing these problems, you can improve your problem-solving skills and develop effective strategies to approach different types of geometry problems.
3. Familiarize with Exam Format
Practice A provides exercises and questions that are similar to those found in geometry exams. By practicing with these materials, you can become familiar with the format and types of questions commonly asked in geometry tests. This familiarity helps you to feel more confident during exams and perform better overall.
4. Identify Weak Areas
Regular practice with Practice A allows you to identify your weak areas and areas that need improvement. By reviewing your mistakes and seeking clarification, you can focus on these specific areas to strengthen your understanding and skills. This targeted practice helps to address your weaknesses and ensure a comprehensive mastery of geometry.
5. Track Progress
Practice A often provides a scoring or tracking system that allows you to monitor your progress. By keeping track of your scores and improvements, you can assess your growth and identify areas where you have made significant progress. This feedback motivates you to continue practicing and strive for further improvement.
In conclusion, regular practice with Practice A is essential for improving your geometry skills. It allows you to reinforce concepts, enhance problem-solving skills, familiarize with exam formats, identify weak areas, and track progress. By dedicating time and effort to practicing geometry regularly, you can significantly enhance your understanding and proficiency in this crucial branch of mathematics.