
In mathematics, radicals and complex numbers are important concepts that are often used in various fields of study, such as algebra and geometry. Understanding and working with radicals and complex numbers are essential for solving equations and problems involving advanced mathematical concepts.
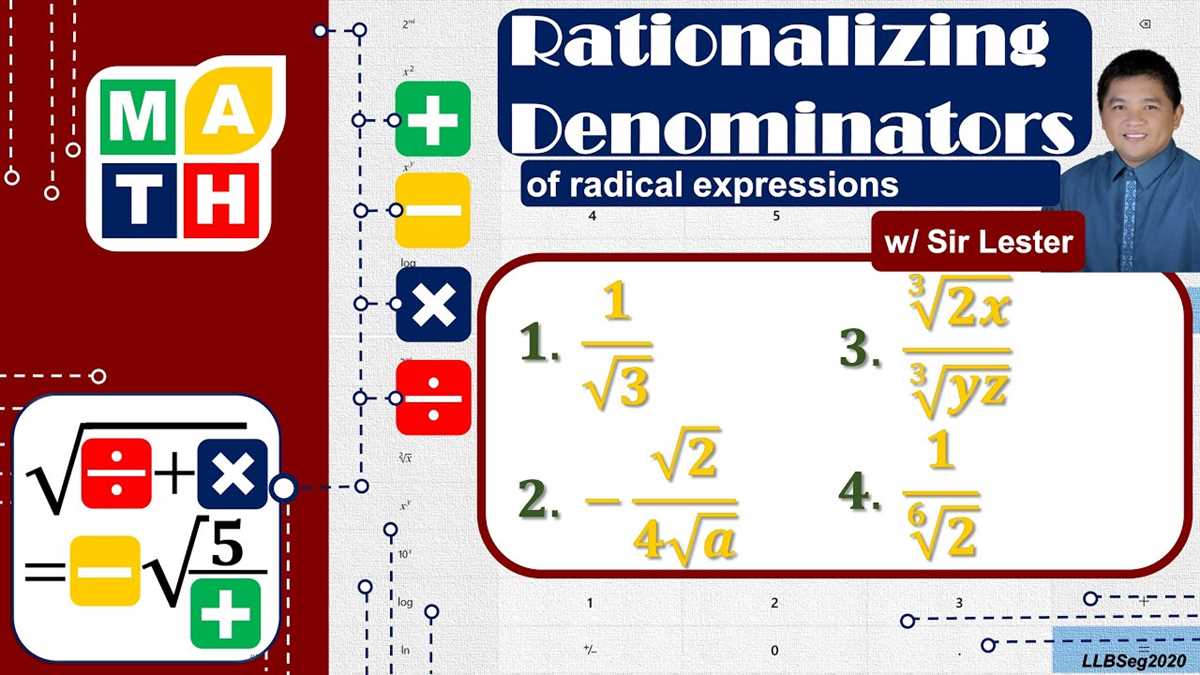
Radicals are mathematical expressions that involve square roots or higher roots of numbers. They are commonly represented by the radical symbol (√) followed by a radicand, which is the number or expression inside the radical. Radicals can be simplified or rationalized to eliminate square roots from the denominator or simplify expressions, making calculations easier.
Complex numbers, on the other hand, are numbers that consist of both a real part and an imaginary part. They are represented in the form a + bi, where “a” is the real part and “bi” is the imaginary part. Complex numbers are used to solve equations and perform operations, such as addition, subtraction, multiplication, and division, with imaginary numbers.
The 2.09 unit test on radicals and complex numbers will assess your knowledge and understanding of these topics. It will require you to simplify radicals, rationalize denominators, solve equations involving radicals, and perform operations with complex numbers. It is important to review the concepts, formulas, and rules associated with radicals and complex numbers to ensure success on the test.
What are radicals?
A radical is a mathematical symbol that represents the root of a number. It is denoted by the symbol √ followed by the number or expression inside the radical. Radicals are a fundamental concept in mathematics and are often used to simplify and solve equations involving roots.
Radicals are typically used to express square roots (√), cube roots (∛), and higher order roots (∜). The number inside the radical is known as the radicand, and the result of evaluating the radical is called the root. For example, the square root of 25 (√25) is 5 because 5 multiplied by itself equals 25.
Radicals can also be used to represent imaginary numbers. An imaginary number is a number that is the square root of a negative number. For example, the imaginary unit i is defined as the square root of -1. Imaginary numbers are often used in complex analysis and have applications in various fields including physics, engineering, and computer science.
When working with radicals, it is important to understand properties such as simplifying radicals, adding and subtracting radicals, and multiplying and dividing radicals. These properties can help simplify mathematical expressions and solve equations involving radicals. Additionally, operations with radicals can often be performed using the rules of exponents.
In conclusion, radicals are mathematical symbols used to represent roots of numbers or expressions. They are used to simplify equations and express solutions involving roots. Radicals can also be used to represent imaginary numbers, which have applications in various fields. Understanding properties and rules associated with radicals is important for mastering mathematical concepts involving roots and imaginary numbers.
How to simplify radicals?
A radical is a mathematical symbol that represents a root of a number. Simplifying radicals involves finding the simplest form of the expression under the radical sign. This is done by determining the factors of the number inside the radical and simplifying it to its smallest terms.
To simplify radicals, it is important to know the properties of exponents. The key concept is that the square root of a number is equivalent to raising that number to the power of 1/2. For example, the square root of 4 can be written as 4^(1/2). This allows us to simplify the expression under the radical sign by looking for perfect square factors.
Here are the steps to simplify radicals:
- Step 1: Identify the perfect square factors of the number under the radical sign.
- Step 2: Write each perfect square factor as its square root.
- Step 3: Multiply the square roots together.
- Step 4: If there are any remaining factors that are not perfect squares, leave them inside the radical sign.
For example, let’s simplify the radical expression sqrt(48). In step 1, we can identify the perfect square factors of 48 as 4 and 12. In step 2, we can write these perfect square factors as sqrt(4) and sqrt(12). In step 3, we multiply these square roots together to get sqrt(4) * sqrt(12) = 2 * sqrt(12). In step 4, 12 is not a perfect square, so we leave it inside the radical sign. Therefore, sqrt(48) can be simplified to 2 * sqrt(12).
In conclusion, simplifying radicals involves identifying perfect square factors and writing them as their square roots. By following the steps mentioned above, you can simplify any radical expression to its simplest form.
Complex Numbers Explained
A complex number is a number that can be expressed in the form a + bi, where a and b are real numbers, and i is the imaginary unit. The real part of the complex number is a, and the imaginary part is b. This concept was introduced to extend the set of real numbers and solve equations that cannot be solved using only real numbers.
The imaginary unit i is defined as the square root of -1. It is important to note that i does not represent a real number, as the square of any real number is always positive. However, by introducing the imaginary unit, equations involving square roots of negative numbers can now be solved.
Complex numbers can be represented geometrically on a complex plane. The real axis represents the real part of the number, while the imaginary axis represents the imaginary part. The origin of the complex plane corresponds to the number 0, and the distance from the origin to a point corresponds to the magnitude of the complex number.
Operations involving complex numbers, such as addition, subtraction, multiplication, and division, can be performed using their algebraic form. Complex numbers also have conjugates, which are obtained by changing the sign of the imaginary part. These conjugates have the same real part and opposite signs for the imaginary part.
Complex numbers have applications in various fields, including physics, engineering, and computer science. They are used to represent quantities that have both a magnitude and a direction, such as alternating current in electrical systems and the amplitude and phase of signals in signal processing.
How to Perform Operations with Complex Numbers?
Complex numbers are numbers that comprise both a real part and an imaginary part. They are typically written in the form a + bi, where a is the real part and bi is the imaginary part. In order to perform operations with complex numbers, we need to understand how to add, subtract, multiply, and divide them.
Addition and Subtraction:
When adding or subtracting complex numbers, we simply add or subtract the real parts and the imaginary parts separately. For example, to add (2 + 3i) and (4 + 5i), we add 2 and 4 to get the real part, and we add 3i and 5i to get the imaginary part. The result is (6 + 8i). Similarly, when subtracting complex numbers, we subtract the real parts and the imaginary parts separately.
Multiplication:
To multiply complex numbers, we use the distributive property and FOIL method. For example, to multiply (2 + 3i) and (4 + 5i), we multiply each term in the first complex number by each term in the second complex number. The result is (8 + 22i + 15i^2). However, since i^2 is equal to -1, we simplify the expression to (8 + 22i – 15). The final result is (-7 + 22i).
Division:

Dividing complex numbers involves multiplying both the numerator and the denominator by the conjugate of the denominator. The conjugate of a complex number a + bi is a – bi. For example, to divide (2 + 3i) by (4 + 5i), we multiply both the numerator and denominator by the conjugate of the denominator, which is (4 – 5i). The result is ((2 + 3i)(4 – 5i))/(4 + 5i)(4 – 5i). Simplifying this expression gives us (-14/41 + 22i/41).
By understanding these basic operations for complex numbers, we can perform calculations and solve equations that involve these numbers. Complex numbers have wide applications in areas such as physics, engineering, and computer science, and being able to manipulate them is an important skill in these fields.
How to solve equations with radicals and complex numbers?
Solving equations that involve radicals and complex numbers requires a solid understanding of these mathematical concepts. Radicals involve finding the square root or cube root of a number, while complex numbers involve both real and imaginary components.
When solving equations with radicals, it is important to isolate the radical on one side of the equation and then square both sides to eliminate the radical. However, it is crucial to check for extraneous solutions, as squaring both sides of an equation can introduce solutions that do not satisfy the original equation.
When dealing with equations that involve complex numbers, it is essential to understand the properties of complex numbers and their operations. Complex numbers are represented as a combination of a real part and an imaginary part, often written as a + bi, where a is the real part and bi is the imaginary part. To solve equations with complex numbers, it may be necessary to perform operations such as addition, subtraction, multiplication, and division on these complex numbers.
To summarize, solving equations with radicals and complex numbers involves understanding the properties of radicals and complex numbers and applying the appropriate mathematical operations to isolate the variable. It is crucial to double-check for extraneous solutions and ensure that the solutions satisfy the original equation.
Tips for preparing for the 2.09 unit test on radicals and complex numbers

Preparing for a unit test on radicals and complex numbers can be challenging, but with the right approach, you can feel confident and ready. Here are some tips to help you study effectively and perform well on the test:
1. Review the concepts
Start by going over the key concepts and principles related to radicals and complex numbers. Make sure you understand the definitions, properties, and operations involved. Take note of any formulas or rules that are important for solving problems in this area.
2. Practice, practice, practice
The more practice problems you solve, the more comfortable and familiar you will become with the material. Look for practice questions in your textbook, online resources, or ask your teacher for additional materials. Try to solve a variety of problems to cover different aspects of the topic.
3. Review your notes
Reviewing your class notes can help reinforce what you have learned in class. Pay attention to any examples or explanations provided by your teacher. If there are any areas that you find confusing, reach out to your teacher or classmates for clarification.
4. Create a study schedule
Plan out your study time in advance to ensure you have enough time to cover all the necessary material. Break down your study sessions into smaller, manageable chunks and allocate specific time periods for each topic. This approach will help you stay organized and prevent last-minute cramming.
5. Seek help if needed
If you are struggling with certain concepts or topics, don’t hesitate to seek help. Reach out to your teacher, classmates, or consider getting a tutor. Sometimes, a different perspective or explanation can make a big difference in your understanding.
By following these tips and putting in dedicated effort, you can feel confident and prepared for the 2.09 unit test on radicals and complex numbers.