
In Algebra, the vertex form is a way to represent quadratic equations. It is written as y = a(x – h)^2 + k, where (h, k) represents the vertex of the parabola. The vertex form is useful because it allows us to easily determine the vertex, direction, and shape of the parabola without having to graph the equation.
In this article, we will explore the answers to a worksheet on vertex form equations. The worksheet will provide various quadratic equations in standard form and ask us to write them in vertex form. By practicing these exercises, we will improve our understanding and skills in converting quadratic equations from standard to vertex form.
Understanding vertex form equations is crucial in solving quadratic equations. It allows us to identify important information such as the vertex and the direction of the parabola. By converting a quadratic equation from standard to vertex form, we can easily identify these key details without having to draw the graph.
Additionally, converting equations to vertex form allows us to manipulate and analyze them more easily. We can use the vertex form to determine the maximum or minimum value of a quadratic equation, find the x-intercepts, and calculate the axis of symmetry. These skills are essential in various areas of mathematics and real-life applications.
By practicing the answers to this 5.2 vertex form worksheet, we can enhance our ability to convert quadratic equations and understand the concepts behind them. This knowledge will not only assist us in solving algebraic problems but also provide us with a solid foundation for further mathematical growth.
What is Vertex Form in Algebra?
The h and k values in the vertex form represent the coordinates of the vertex of the parabola. The vertex is the highest or lowest point on the graph of a quadratic function, and its coordinates are given in the form (h, k). For example, if the vertex form is y = 2(x – 3)^2 + 4, the vertex is at the point (3, 4).
The a value in the vertex form determines the direction and steepness of the parabola. If a is positive, the parabola opens upwards and has a minimum point. If a is negative, the parabola opens downwards and has a maximum point. The absolute value of a also determines the stretch or compression of the parabola.
The vertex form is useful in algebra because it provides a simplified way to write the equation of a quadratic function and easily identify its key characteristics. By looking at the vertex form, we can quickly determine the coordinates of the vertex, the direction of the parabola, and make predictions about its behavior.
Understanding the Basics of Vertex Form

One of the advantages of using vertex form is that it helps us easily identify the vertex of a parabola without having to graph the entire function. The vertex is given by the values of (h, k), which can be directly read from the equation. The value of h represents the x-coordinate of the vertex, while k represents the y-coordinate.
In addition to identifying the vertex, vertex form also provides information about the direction of the parabola. The value of a determines whether the parabola opens upward or downward. If a is positive, the parabola opens upward, while if a is negative, the parabola opens downward.
Another useful feature of vertex form is that it allows us to easily determine the axis of symmetry of a parabola. The axis of symmetry is a vertical line that passes through the vertex and divides the parabola into two symmetrical halves. The equation for the axis of symmetry can be found by setting x – h = 0, which simplifies to x = h.
By understanding the basics of vertex form, we can solve various problems involving quadratic functions more efficiently. Whether it’s finding the vertex, determining the direction of the parabola, or analyzing the axis of symmetry, vertex form provides us with a clear and concise way to express and interpret quadratic functions.
Key Features of the Vertex Form Equation

The vertex form equation is a commonly used form of a quadratic function, and it has several key features that are worth understanding. By expressing a quadratic function in vertex form, we can easily identify the vertex, axis of symmetry, and the direction of the parabola.
Vertex: The vertex form equation of a quadratic function is written as y = a(x – h)^2 + k, where (h, k) represents the coordinates of the vertex. The vertex is the point where the parabola reaches its highest or lowest value, depending on the value of a.
Axis of Symmetry: The axis of symmetry is a vertical line that passes through the vertex and divides the parabola into two equal halves. It is represented by the equation x = h in vertex form. The axis of symmetry is always parallel to the y-axis and is a key characteristic of the shape and symmetry of the quadratic function.
Direction of the Parabola: The value of a in the vertex form equation determines the direction in which the parabola opens. When a is positive, the parabola opens upwards, meaning the vertex represents the minimum point of the function. On the other hand, when a is negative, the parabola opens downwards with the vertex representing the maximum point of the function.
- Vertex: (h, k) represents the coordinates of the vertex in vertex form.
- Axis of Symmetry: The axis of symmetry is a vertical line that passes through the vertex.
- Direction of the Parabola: The value of a determines the direction in which the parabola opens.
Understanding these key features of the vertex form equation can help in graphing quadratic functions and analyzing their behavior. By knowing the vertex, axis of symmetry, and the direction of the parabola, we can quickly sketch the graph and make assertions about its properties without having to rely on extensive calculations or computational tools.
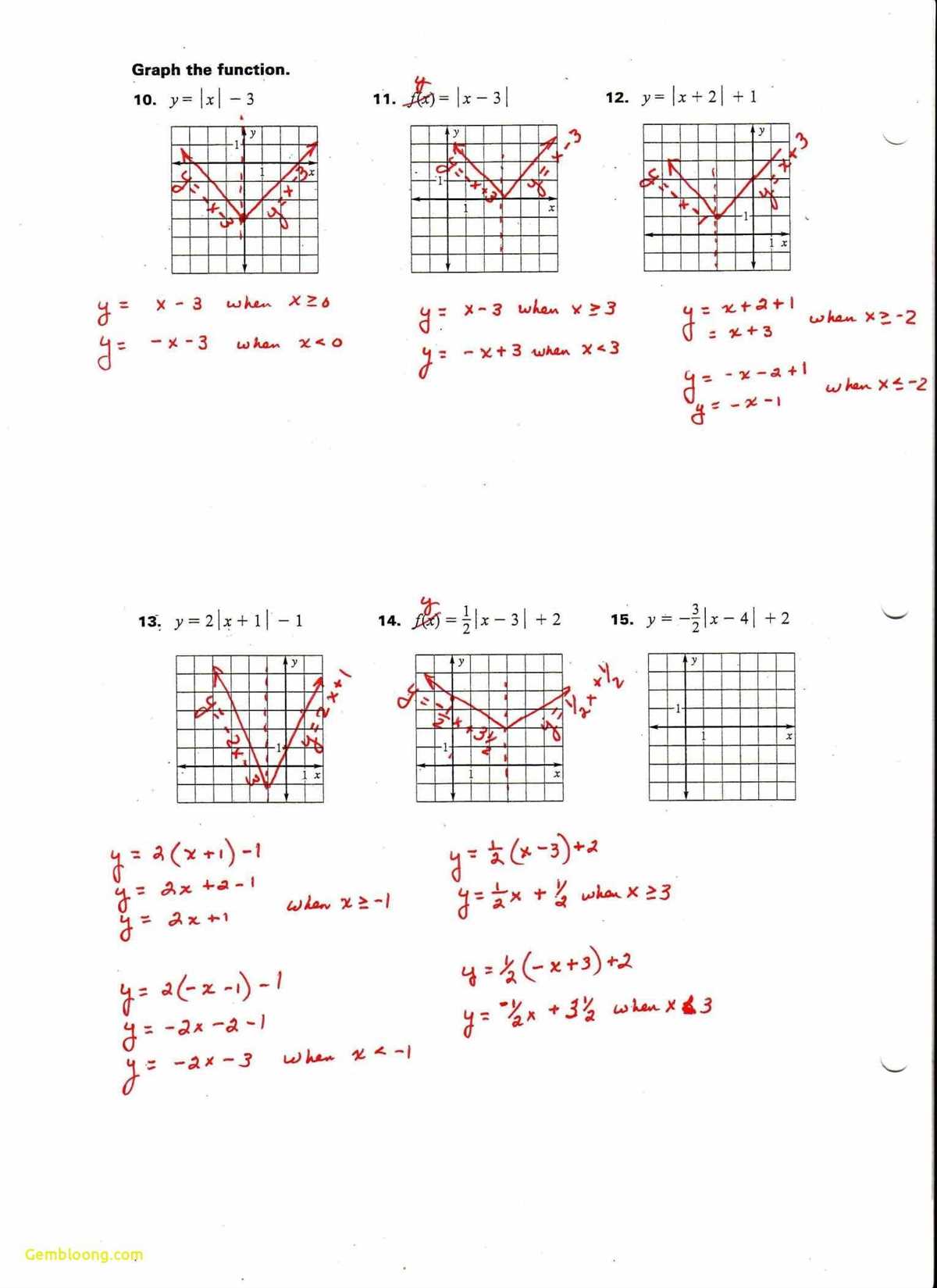
How to Graph Quadratic Functions in Vertex Form
To graph a quadratic function in vertex form, you need to follow a few steps:
- Identify the vertex: The vertex is given as (h, k) in the equation. The values of h and k represent the horizontal and vertical translations of the parabola, respectively.
- Find the axis of symmetry: The axis of symmetry is a vertical line that passes through the vertex. It is given by the equation x = h.
- Determine the direction of the parabola: The coefficient ‘a’ in the equation determines whether the parabola opens upwards (if a > 0) or downwards (if a < 0).
- Plot additional points: Choose a few x-values, substitute them into the equation to find the corresponding y-values, and plot those points on the graph.
- Graph the parabola: Connect the points on the graph to create a smooth curve that represents the parabola. Make sure the graph is symmetrical about the axis of symmetry.
By following these steps, you will be able to accurately graph quadratic functions in vertex form. Remember to pay attention to the vertex, axis of symmetry, and the direction of the parabola, as they are essential in determining the shape and position of the graph.
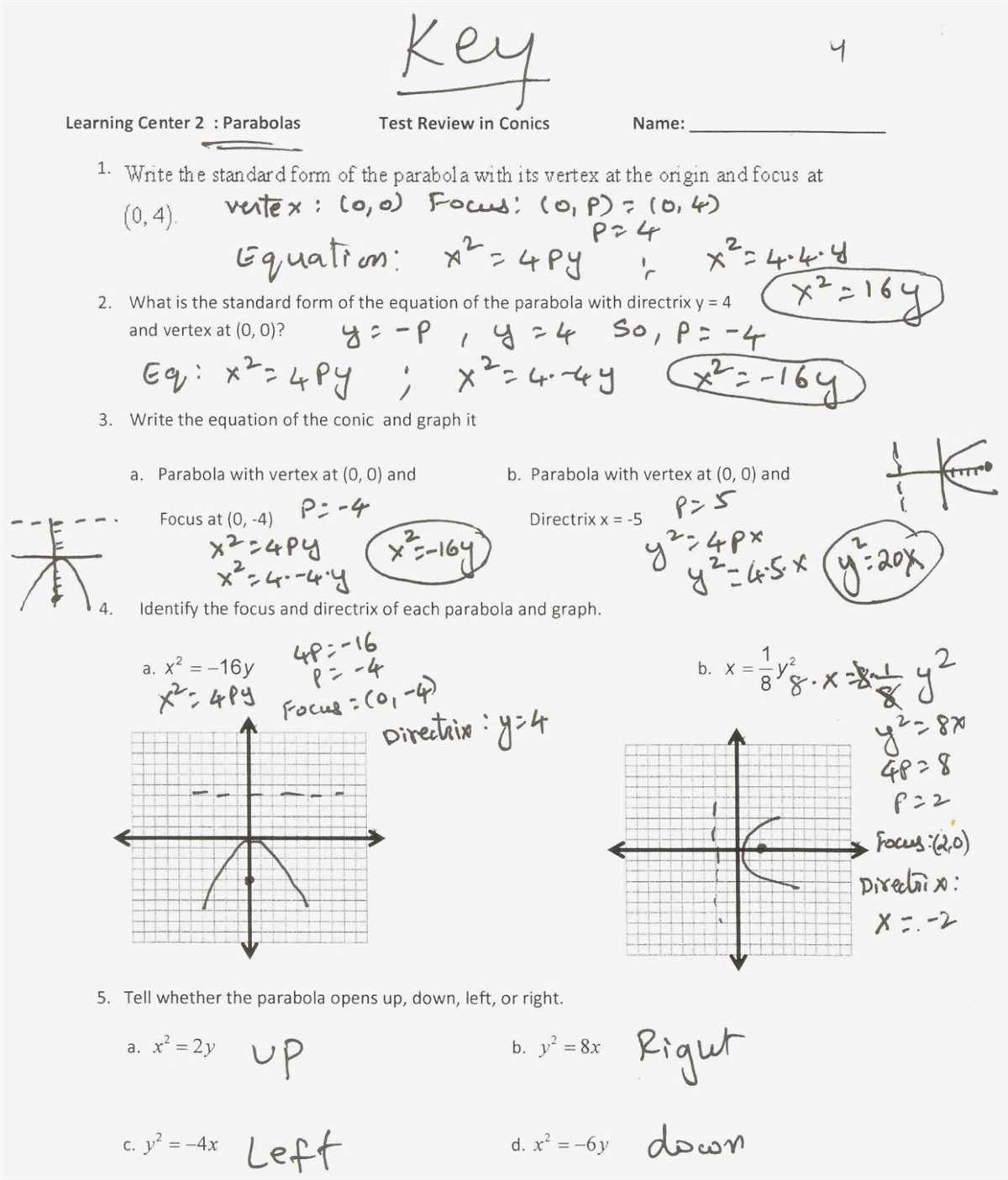
Vertex Form Worksheet with Detailed Solutions
Are you struggling with understanding and solving quadratic equations in vertex form? This worksheet is designed to help you master this topic with detailed solutions and explanations.
The vertex form of a quadratic equation is given by the equation y = a(x-h)^2 + k, where (h, k) represents the coordinates of the vertex. This form allows us to easily identify the vertex and understand the characteristics of the graph.
Using this worksheet, you will practice identifying the vertex of a quadratic equation in vertex form. You will also learn how to determine the values of a, h, and k by analyzing the equation. The detailed solutions provided will guide you through each step of the process, making it easier to grasp the concept and solve problems effectively.
The worksheet includes a variety of quadratic equations in vertex form, ranging in difficulty. It covers topics such as finding the vertex, determining the values of a, h, and k, and analyzing the graph of the equation. Each problem is accompanied by a detailed solution, which breaks down the steps and provides a clear explanation of the solution. This allows you to review and understand each problem thoroughly.
By practicing with this worksheet, you will build confidence in solving quadratic equations in vertex form. You will develop a deeper understanding of the graphical representation of quadratic equations and be able to apply this knowledge to real-world problems. Whether you are a beginner or need to refresh your skills, this worksheet will be a valuable resource for mastering the vertex form of quadratic equations.
Common Mistakes to Avoid in Solving Vertex Form Equations
When solving vertex form equations, there are several common mistakes that students often make. These mistakes can lead to incorrect solutions and a misunderstanding of the concept. It is important to be aware of these mistakes and take steps to avoid them. Here are some common mistakes to watch out for:
1. Incorrect substitution of values

One common mistake is substituting values into the equation incorrectly. It is important to carefully substitute the values of x and y into the equation in order to get the correct solution. Any errors in this step can lead to incorrect solutions.
2. Forgetting to use the correct vertex form equation
Another mistake is forgetting to use the correct vertex form equation. The vertex form equation is in the form y = a(x – h)^2 + k, where (h, k) represents the vertex of the parabola. Using the wrong equation can lead to incorrect solutions.
3. Misinterpreting the meaning of the vertex
Some students may misinterpret the meaning of the vertex. The vertex represents the highest or lowest point of the parabola. It is important to understand that the vertex is not necessarily the maximum or minimum value of the equation. Misinterpreting the vertex can lead to incorrect solutions.
4. Not factoring correctly
Factoring is an important step in solving vertex form equations. However, some students may not factor correctly or may forget to factor completely. Factoring incorrectly can lead to incorrect solutions or make the equation more difficult to solve.
5. Failing to check the solution
Lastly, failing to check the solution is a common mistake. It is important to substitute the obtained solution back into the original equation to ensure that it satisfies the equation. Failing to check the solution can lead to false solutions or missing potential solutions.
Overall, by being aware of these common mistakes and taking steps to avoid them, students can improve their understanding and accuracy in solving vertex form equations. It is important to practice and review these concepts to ensure a thorough understanding of the topic.
Q&A:
What is the vertex form equation?
The vertex form equation is a way to represent a quadratic equation. It is written in the form y = a(x-h)^2 + k, where (h, k) represents the vertex of the parabola.
Why is it important to distribute the ‘a’ coefficient in vertex form equations?
It is important to distribute the ‘a’ coefficient to both terms inside the parentheses because it affects the shape and direction of the parabola. Neglecting to do so can lead to incorrect graphing or solving of the equation.
What does misplacing the sign of the ‘h’ value in the vertex form equation lead to?
Misplacing the sign of the ‘h’ value can lead to a shift in the graph of the parabola. It affects the x-coordinate of the vertex, resulting in an incorrect location of the vertex if not accounted for properly.