
Polynomial functions are an important topic in algebra, and being able to find the answers to practice problems is essential for mastering this concept. In this article, we will be looking at the answers to the 5-3 practice polynomial functions questions in Form G.
Polynomial functions are functions that are defined by a polynomial equation. These equations are made up of terms that consist of variables raised to whole number exponents, multiplied by coefficients. An example of a polynomial function is f(x) = 2x^3 + 5x^2 – 3x + 7. It is important to be able to identify the degree of a polynomial function, which is the highest exponent of the variables in the equation.
The 5-3 practice polynomial functions questions in Form G are designed to test students’ understanding of polynomial functions. They may involve finding the degree of a polynomial, identifying the leading coefficient, or evaluating the function for specific values of x. By practicing these types of problems, students can strengthen their skills and improve their understanding of polynomial functions.
Now, let’s take a look at the answers to the 5-3 practice polynomial functions questions in Form G. It is important to note that these answers may vary depending on the specific polynomial function given in your practice worksheet. Therefore, it is recommended to compare your answers with the given solutions to ensure accuracy.
Understanding Polynomial Functions: 5-3 Practice Answers in Form G
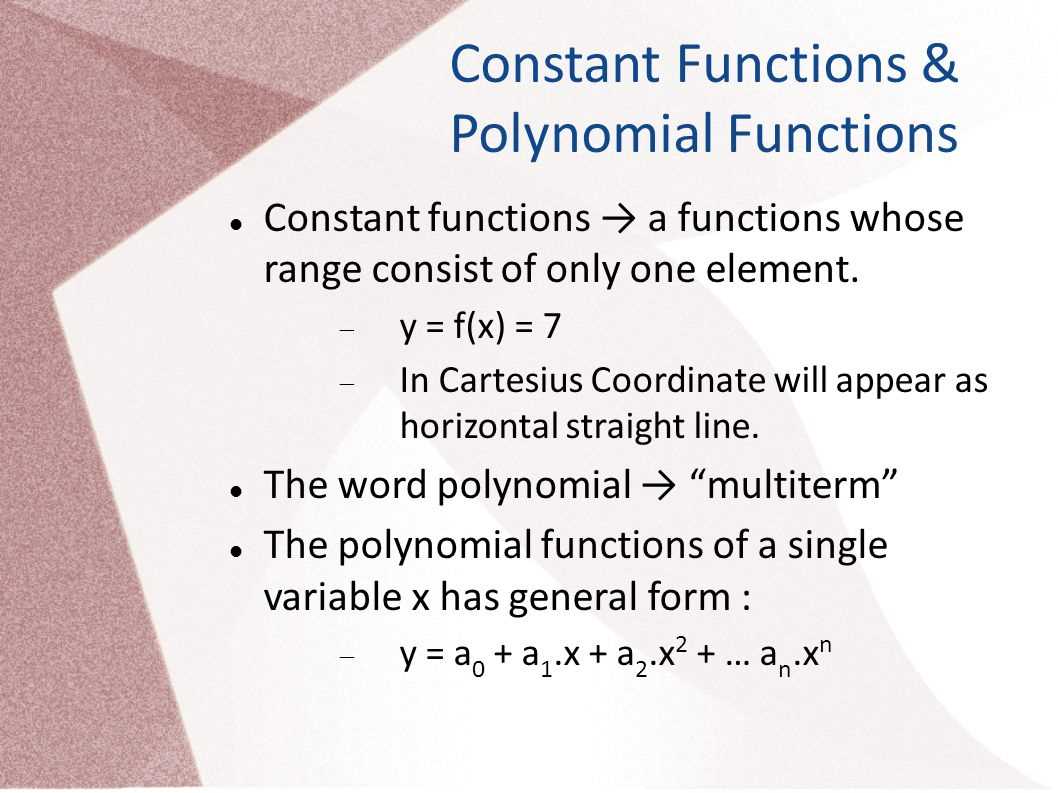
Polynomial functions are an essential topic in algebra, and understanding their properties and behaviors is crucial for solving various mathematical problems. The 5-3 Practice Answers in Form G provide valuable insights into polynomial functions and offer a comprehensive review of their concepts.
The practice answers in Form G cover a range of topics related to polynomial functions, including finding the degree, leading coefficient, and end behavior of a polynomial. By working through these exercises, students can strengthen their understanding of key polynomial concepts and improve their ability to solve polynomial equations.
- Degree of a Polynomial: One of the fundamental aspects of polynomial functions is their degree, which represents the highest power of the variable in the equation. The practice answers in Form G provide examples and explanations on how to determine the degree of a given polynomial.
- Leading Coefficient: Another important aspect of polynomial functions is the coefficient of the term with the highest power. This coefficient, known as the leading coefficient, plays a significant role in determining the behavior of the polynomial function. The Form G practice answers help students identify and understand the significance of the leading coefficient.
- End Behavior: The end behavior of a polynomial function refers to the behavior of the function as the input approaches positive or negative infinity. The 5-3 Practice Answers in Form G provide exercises that allow students to analyze and predict the end behavior of different polynomial functions.
Overall, the 5-3 Practice Answers in Form G are an invaluable resource for students looking to enhance their understanding of polynomial functions. By practicing these exercises and reviewing the solutions, students can develop a solid foundation in polynomial concepts and improve their problem-solving skills in algebra.
Overview of Polynomial Functions
Polynomial functions are an important topic in algebra and calculus. They are defined as functions that can be expressed as a sum of terms, where each term is a constant multiplied by a variable raised to a non-negative integer power. Polynomial functions can take on various forms, but they all follow this basic structure.
Polynomial functions play a crucial role in mathematics, as they can be used to model a wide range of real-life phenomena. They are often used in fields such as physics, engineering, and finance to describe relationships between variables and make predictions or calculations.
Basic Characteristics:
- A polynomial function can have multiple terms, with each term having its own coefficient and exponent.
- The degree of a polynomial function is determined by the highest exponent of the variable.
- The leading coefficient is the coefficient of the term with the highest power of the variable.
- Polynomial functions can have zeros, also known as roots or x-intercepts, which are the values of the variable that make the function equal to zero.
Common Forms:
Polynomial functions can be written in various forms, depending on what information is given or what needs to be determined. Some common forms of polynomial functions include:
- Standard form: f(x) = anxn + an-1xn-1 + … + a1x + a0
- Factored form: f(x) = a(x – r1)(x – r2)…(x – rn)
- Vertex form (for quadratic functions): f(x) = a(x – h)2 + k
Overall, understanding polynomial functions is essential for solving equations, finding roots, graphing functions, and analyzing mathematical models. It is a fundamental concept that lays the groundwork for further studies in algebra and calculus.
Exploring the 5-3 Practice Problems

The 5-3 Practice Problems is a set of exercises designed to help students understand and apply the concepts of polynomial functions. This set of problems focuses on various topics related to polynomials, such as finding zeros, determining end behavior, and factoring.
One of the key aspects of the 5-3 Practice Problems is the emphasis on real-world applications. Many of the problems involve scenarios that students can relate to, such as determining the selling price of a product based on a given polynomial function or finding the area of a garden with polynomial dimensions.
One of the key skills that students develop through these practice problems is the ability to solve polynomial equations. They learn how to apply factoring techniques, long division, synthetic division, and the Zero Product Property to find the roots of a polynomial function. This is an essential skill in algebra and is often used in calculus and other advanced math courses.
The 5-3 Practice Problems also provide opportunities for students to explore the graphical representation of polynomial functions. They learn how to identify key features of a graph, such as intercepts, local maxima and minima, and end behavior. This helps them develop a deeper understanding of the relationship between the algebraic and graphical representations of polynomial functions.
In conclusion, the 5-3 Practice Problems offer a comprehensive set of exercises that allow students to explore the various aspects of polynomial functions. They provide real-world applications, opportunities for problem-solving, and a deeper understanding of the graphical representation of polynomial functions. By engaging with these problems, students can strengthen their algebraic skills and develop a solid foundation for future math courses.
Understanding Form G
Form G is a commonly used format for practicing polynomial functions. It provides students with a series of questions and problems that help them strengthen their understanding of polynomial functions and their properties. By working through Form G exercises, students can improve their problem-solving skills and deepen their understanding of key concepts in polynomial functions.
Form G typically includes a variety of different types of problems, such as finding the degree and leading coefficient of a polynomial function, identifying the end behavior of a function, and solving polynomial equations. These problems often require students to apply their knowledge of polynomial functions and use algebraic techniques to find solutions.
One of the benefits of using Form G is that it allows students to practice their skills in a structured and organized way. The questions and problems in Form G are designed to build on each other, starting with basic concepts and gradually increasing in difficulty. This progression helps students develop a solid foundation in polynomial functions and prepares them for more complex mathematical concepts.
Additionally, Form G provides students with the opportunity to identify and correct any misconceptions they may have about polynomial functions. By reviewing their answers and comparing them to the correct solutions provided in Form G, students can identify areas where they need further clarification or practice.
In conclusion, Form G is a valuable tool for students studying polynomial functions. It provides a structured and organized way to practice key concepts and strengthen problem-solving skills. By working through Form G exercises, students can deepen their understanding of polynomial functions and build a solid foundation for further mathematical learning.
Step-by-Step Solutions to the Practice Problems
In this section, we will provide step-by-step solutions to the practice problems in the “5-3 Practice Polynomial Functions” worksheet. These solutions will help you understand how to solve polynomial function problems and guide you through the process.
Problem 1:
To solve this problem, we will first identify the given equation: f(x) = 2x3 – 5x2 + 3x – 7. We need to find the zeros of the function, which are the values of x that make f(x) equal to zero. This can be done by factoring or using the quadratic formula. Once we find the zeros, we can plot them on a graph and determine the x-intercepts.
Step 1: We can try to factor the given equation. In this case, factoring is not possible, so we move on to the quadratic formula.
Step 2: Apply the quadratic formula: x = (-b ± √(b^2 – 4ac))/(2a). Plugging in the values from the equation, we get x = (-(-5) ± √((-5)^2 – 4(2)(-7)))/(2(2)). Simplifying further, we get x = (5 ± √(25 + 56))/4. This gives us two possible solutions: x = (5 ± √81)/4.
Problem 2:
This problem asks us to find the degree and leading coefficient of the polynomial function g(x) = 4x6 + 2x3 – 7x + 9. The degree of a polynomial is determined by the highest exponent of the variable, while the leading coefficient is the coefficient of the term with the highest degree.
Step 1: Identify the polynomial function: g(x) = 4x6 + 2x3 – 7x + 9.
Step 2: Find the degree: In this case, the term with the highest exponent is 4x6, so the degree of the polynomial is 6.
Step 3: Find the leading coefficient: The leading coefficient is the coefficient of the term with the highest degree. In this case, it is 4.
By following these step-by-step solutions, you can gain a better understanding of how to solve polynomial function problems. Remember to carefully analyze the given equations, apply the appropriate formulas or techniques, and simplify the solutions to get the final answers. Practicing these problems will help you become more proficient in solving polynomial function problems and strengthen your overall math skills.
Common Mistakes to Avoid
When it comes to solving polynomial functions, there are several common mistakes that students often make. These mistakes can lead to incorrect answers and a misunderstanding of the concepts involved. By being aware of these mistakes, you can avoid them and improve your understanding of polynomial functions.
Not factoring correctly: One of the most common mistakes students make is not factoring the polynomial correctly. Factoring is an important step in simplifying and solving polynomial functions. It is crucial to correctly identify and factor out any common factors, then apply the appropriate factoring techniques for higher-degree polynomials.
Forgetting to check for extraneous solutions: Another common mistake is forgetting to check for extraneous solutions. When solving polynomial functions, it’s important to check your solutions to make sure they are valid for the original equation. Sometimes, a solution may satisfy the derived equation but not the original equation, leading to extraneous solutions.
Incorrectly applying the remainder theorem: The remainder theorem is a useful tool for evaluating polynomial functions. However, it’s easy to make mistakes when applying this theorem. Students may forget to divide the polynomial correctly or mix up the variables, resulting in incorrect answers. It’s important to carefully follow the steps of the remainder theorem to ensure accurate results.
Not using the correct degree of the polynomial: It’s essential to use the correct degree of the polynomial when solving polynomial functions. Sometimes, students may mistakenly use the degree of a term instead of the overall degree of the polynomial. This can lead to incorrect calculations and answers.
Skipping steps or rushing through the problem: Finally, one common mistake is skipping steps or rushing through the problem. Polynomial functions often require multiple steps, and it’s important to take the time to carefully work through each step. Rushing through the problem can lead to errors and incorrect answers. Take your time and double-check your work to avoid these mistakes.
Summary and Further Application of Polynomial Functions
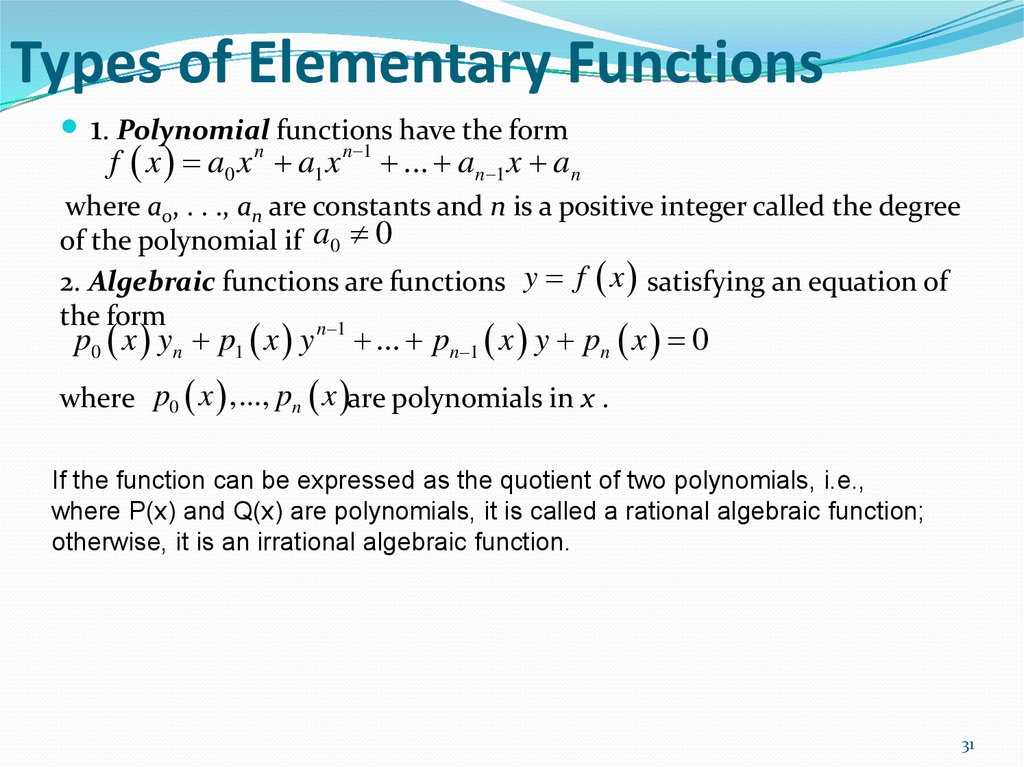
Polynomial functions are a fundamental concept in mathematics that involve equations with multiple terms and coefficients. They can be used to model and solve a wide range of real-world problems, including predicting population growth, analyzing financial trends, and designing optimal structures.
Throughout this article, we have explored the basics of polynomial functions, including their standard form, degree, leading coefficient, and end behavior. We have also examined how to graph polynomial functions, find their zeros, and determine their key features such as the vertex and axis of symmetry for quadratic functions.
To further apply polynomial functions, one can use them to solve applied problems in various fields such as physics, engineering, economics, and biology. By modeling real-world situations with polynomial functions, we can analyze and make predictions about their behavior and make informed decisions.
Moreover, advanced techniques such as factoring, synthetic division, and the rational root theorem can be applied to manipulate and simplify polynomial functions, making them easier to solve and analyze.
In conclusion, polynomial functions are a powerful tool in mathematics that allow us to model, analyze, and solve a wide range of problems. By understanding the concepts and techniques related to polynomial functions, we can gain valuable insights into various real-world phenomena and make informed decisions. They are an essential topic to study for anyone interested in mathematics or fields that use mathematical modeling.