
In mathematics, the study of functions is essential for understanding relationships between variables and solving equations. The 6-1 Study Guide and Intervention: Operations on Functions covers various operations that can be performed on functions, such as addition, subtraction, multiplication, and division. This answer key is a valuable resource for students studying this topic, as it provides step-by-step solutions to the problems presented in the study guide.
By using this answer key, students can check their work and verify if they have correctly solved the problems. It serves as a tool for self-assessment and helps in identifying areas that require further practice and clarification. Additionally, the answer key provides an opportunity for students to learn from their mistakes and improve their problem-solving skills.
The 6-1 Study Guide and Intervention: Operations on Functions answer key also serves as a guide for teachers, offering them a reference for the correct solutions to the problems. It can be used to assess students’ understanding of the topic and provide feedback on their performance. Teachers can also use the answer key to create additional practice problems or customize worksheets to further reinforce the concepts covered in this study guide.
What is a study guide and intervention for operations on functions?
A study guide and intervention for operations on functions is a resource designed to help students learn and practice the concepts and skills related to performing different operations on functions, such as addition, subtraction, multiplication, division, composition, and inverse. It provides step-by-step explanations, examples, and practice problems to guide students through the process of understanding and applying these operations.
One key feature of a study guide and intervention for operations on functions is the inclusion of clear and concise explanations of the underlying concepts. This helps students develop a solid foundation of knowledge and understanding, enabling them to approach different types of function operations with confidence. The guide may also provide visual representations, such as graphs or diagrams, to support the explanations and make the concepts more tangible.
A study guide and intervention for operations on functions typically includes a variety of practice problems to reinforce the learning. These problems may range in difficulty, allowing students to start with basic operations and gradually progress to more complex ones. The guide may also provide answer keys or solutions for the practice problems, allowing students to check their work and identify any areas that may need further review or clarification.
In addition to explanations and practice problems, a study guide and intervention for operations on functions may also include additional resources, such as tables, charts, or summary notes. These resources can serve as quick references for students and help them consolidate their learning. Furthermore, the guide may offer tips and strategies for solving different types of problems, helping students approach the operations more efficiently and effectively.
In summary, a study guide and intervention for operations on functions is a valuable tool for students to enhance their understanding and proficiency in performing different operations on functions. By providing clear explanations, practice problems, and additional resources, the guide supports students in building their knowledge and skills in this important area of mathematics.
Understanding the basics of operations on functions
In mathematics, functions are representations of relationships between two or more variables. Operations on functions involve manipulating these relationships to obtain new functions or information about the original functions. These operations can help us solve problems involving functions, analyze their behavior, or simplify complex expressions.
Basic operations on functions:
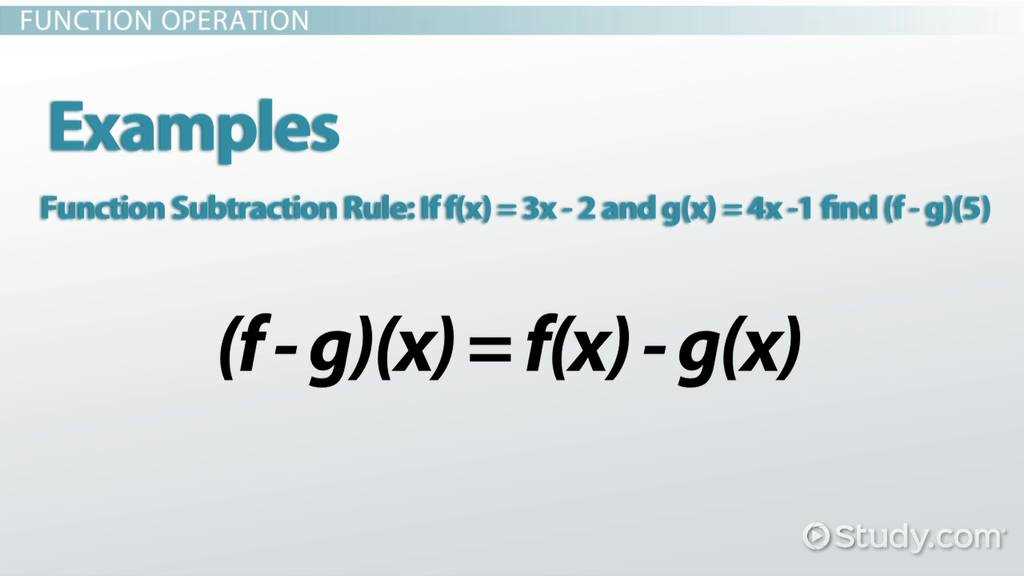
1. Addition and subtraction: When adding or subtracting functions, we simply combine their corresponding terms. For example, if we have two functions f(x) and g(x), the sum of these functions would be (f+g)(x) = f(x) + g(x). Similarly, the difference between these two functions would be (f-g)(x) = f(x) – g(x).
2. Multiplication: When multiplying functions, we can use the distributive property to expand the expression. For example, if we have two functions f(x) and g(x), the product of these functions would be (f*g)(x) = f(x) * g(x). This operation is useful for finding the composition of functions or analyzing their combined effect.
3. Composition: Function composition is a way to combine functions by plugging one function into another. For example, if we have two functions f(x) and g(x), the composition of these functions would be (f ◦ g)(x) = f(g(x)). This allows us to study the effect of one function on the output of another function.
These basic operations on functions provide us with powerful tools for understanding and analyzing mathematical relationships. They enable us to manipulate functions in various ways, allowing us to solve problems, simplify expressions, or gain insights into the behavior of functions. By mastering these operations, we can become proficient in handling functions and applying them to real-world situations.
Exploring various types of operations on functions
Operations on functions are an essential topic in the study of algebra and calculus. Understanding how different operations affect functions is crucial for solving complex mathematical problems and analyzing real-world scenarios. There are several types of operations that can be performed on functions, each with its own set of rules and properties.
One common operation on functions is addition or subtraction. When two functions are added or subtracted, their corresponding terms are combined or subtracted, respectively. This operation is useful for finding the sum or difference of two functions, which can provide insights into the overall behavior of the combined function.
Another important operation is function composition. In function composition, one function is applied to the output of another function. This operation creates a new function that represents the combination of the two original functions. Function composition allows us to analyze the effects of applying one function after another, which can yield interesting and complex results.
One more operation on functions is multiplication or division. When two functions are multiplied or divided, their corresponding terms are multiplied or divided, respectively. This operation is useful for finding the product or quotient of two functions, which can provide information about the growth or decay rates of the combined function.
Overall, exploring the various types of operations on functions helps us analyze and manipulate functions to solve mathematical problems and understand real-world phenomena. By understanding the rules and properties of these operations, we can gain a deeper insight into the behavior and characteristics of functions.
Examining the Importance of Studying Operations on Functions
Operations on functions play a crucial role in mathematics and various fields of science. Understanding these operations is essential for analyzing and manipulating mathematical functions accurately and efficiently. By studying operations on functions, individuals can develop a deeper understanding of the underlying principles and concepts in mathematics.
One key reason why studying operations on functions is important is that it allows us to combine and transform functions to explore new mathematical relationships. Through operations such as addition, subtraction, multiplication, and division, we can manipulate functions to create new functions that represent different mathematical phenomena. This ability to modify functions is fundamental to many areas of mathematics, including calculus, algebra, and statistics.
Operations on functions also enable us to solve complex mathematical problems. By applying operations such as composition, inverse, and combinations of functions, we can simplify equations and solve for unknown variables. This is particularly useful in real-world applications, where functions are often used to model and analyze various phenomena.
Furthermore, studying operations on functions helps develop critical thinking and problem-solving skills. By working with mathematical functions, individuals learn to analyze patterns, identify relationships, and apply logical reasoning to solve problems. These skills are transferable to many other areas of life and are valuable for success in fields such as engineering, computer science, and finance.
In conclusion, studying operations on functions is of paramount importance for anyone interested in mathematics and its applications. By gaining a deep understanding of these operations, individuals can enhance their mathematical abilities and develop valuable problem-solving skills. Whether in academic or real-world settings, operations on functions are essential tools for analyzing, manipulating, and solving complex mathematical problems.
Key components of a study guide and intervention for operations on functions

A study guide and intervention for operations on functions is a valuable tool for students to enhance their understanding of this mathematical concept. It provides a structured approach to learning and practicing operations on functions, ensuring that students have a solid foundation in this area.
1. Clear explanations: A good study guide and intervention should provide clear and concise explanations of the various operations on functions. It should define key terms and concepts, and provide examples to illustrate how these operations are performed.
2. Step-by-step instructions: The guide should break down each operation into step-by-step instructions, making it easy for students to follow along and understand the process. This ensures that students can practice the operations correctly and build confidence in their abilities.
3. Practice problems: A study guide and intervention should include plenty of practice problems for students to work on. These problems should cover a range of difficulty levels, allowing students to gradually build their skills and confidence in performing operations on functions.
4. Answer key: Having an answer key is essential for students to check their work and understand where they went wrong. The answer key should provide detailed explanations for each problem, helping students identify their mistakes and learn from them.
5. Review and reinforcement: A study guide and intervention should include periodic review sections that allow students to reinforce their understanding of operations on functions. These sections can include review questions, summaries, and additional practice problems to ensure that students retain the information.
To maximize the effectiveness of a study guide and intervention for operations on functions, it is important for students to actively engage with the material. They should read the explanations carefully, complete all the practice problems, and seek clarification for any areas of confusion. By doing so, students can develop a strong understanding of operations on functions and be better prepared for future mathematical challenges.
Analyzing the Benefits of Using an Answer Key for Operations on Functions
Using an answer key for operations on functions can offer several benefits for students. Firstly, it provides immediate feedback on whether their solutions are correct or not. This can help students identify any mistakes they may have made and understand the correct steps to solve the problem. It also allows them to check their progress as they work through the exercises, giving them a sense of accomplishment as they see their correct answers.
Moreover, an answer key can be a useful study tool. By comparing their solutions to the correct answers, students can identify any areas of weakness or concepts they still need to work on. This can guide their study efforts and help them focus on specific topics or types of problems they are struggling with. It allows them to practice and refine their problem-solving skills by attempting different approaches until they arrive at the correct answer.
Another benefit of using an answer key is that it can contribute to a more efficient use of class time. By providing students with a tool to check their answers independently, it reduces their reliance on the teacher to confirm their solutions. This frees up valuable classroom time for more interactive and engaging discussions or activities related to the topic, rather than simply reviewing answers. It promotes a sense of self-reliance and ownership of learning among students.
In conclusion, incorporating an answer key for operations on functions into the learning process can have numerous advantages for students. It offers immediate feedback, aids in identifying areas of weakness, encourages independent learning, and maximizes the efficient use of class time. By utilizing an answer key, students are empowered to take control of their learning and improve their understanding of operations on functions.
Strategies for successfully completing a study guide and intervention on operations on functions
Completing a study guide and intervention on operations on functions can be a challenging task, but with the right strategies in place, it can be a manageable and rewarding experience. Here are some tips to help you successfully complete your study guide:
- Start early: Begin working on your study guide well in advance of the due date. This will give you enough time to fully understand the concepts and practice applying them.
- Break it down: Divide your study guide into smaller, more manageable sections. This will make the task seem less daunting and allow you to focus on one concept at a time.
- Create a schedule: Set aside dedicated study time for each section of the study guide. Having a structured plan will help you stay organized and ensure that you cover all the necessary material.
- Seek help when needed: If you come across a concept or problem that you’re struggling with, don’t hesitate to ask for help. Reach out to your teacher, classmates, or online resources for clarification and guidance.
- Practice, practice, practice: The key to mastering operations on functions is practice. Work through as many practice problems as you can, both from the study guide and additional resources. This will help reinforce your understanding and improve your problem-solving skills.
- Review and revise: Before submitting your study guide, take the time to review your answers and make any necessary revisions. Double-check your work for any mistakes and make sure your explanations are clear and concise.
By following these strategies, you’ll be well-prepared to successfully complete your study guide and intervention on operations on functions. Remember to stay focused, stay organized, and seek help when needed. Good luck!