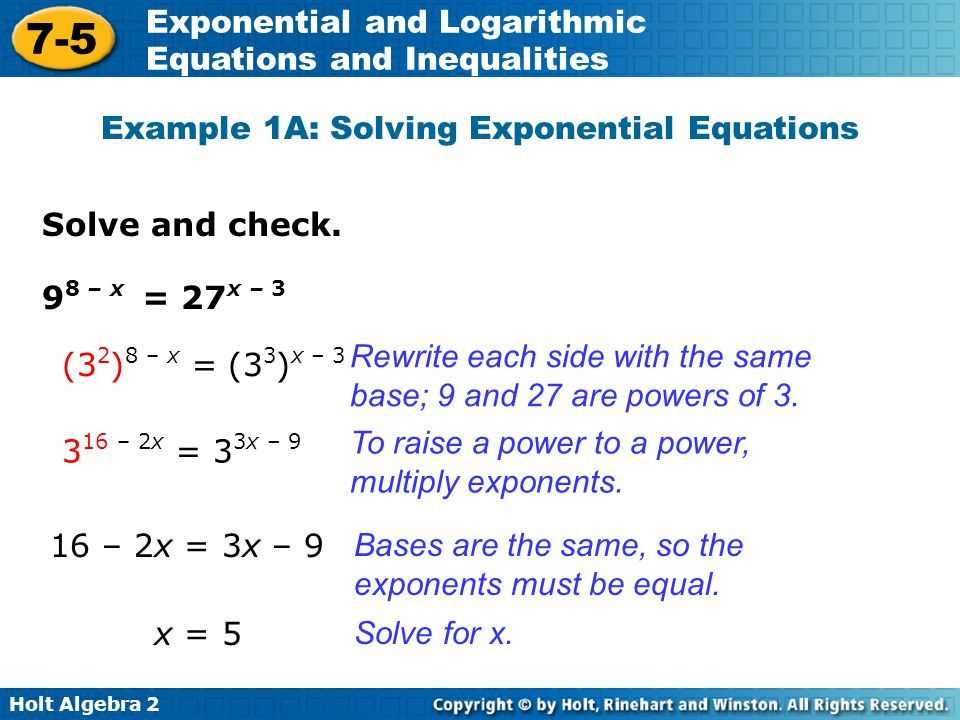
In mathematics, exponential equations and inequalities are a crucial part of solving various problems involving exponential functions. These types of equations and inequalities involve variables in the exponent, which makes them more complex to solve compared to linear equations. However, with the proper knowledge and understanding of the properties of exponential functions, solving these equations and inequalities becomes more manageable.
One method of solving exponential equations and inequalities is by using logarithms. Logarithms allow us to reverse the process of exponentiation, making it easier to isolate the variable. By taking the logarithm of both sides of the equation or inequality, we can transform it into a more familiar and solvable form. This process effectively removes the variable from the exponent and brings it down to a base level.
When solving exponential equations and inequalities, it is essential to consider different scenarios and potential solutions. For example, some equations may have multiple solutions, while others may not have any real solutions at all. Additionally, inequalities involving exponential functions may have different intervals of validity, depending on the properties of the exponential function and its base. Understanding these factors helps us determine the appropriate range of solutions for the given problem.
Overall, solving exponential equations and inequalities is an important skill in mathematics that has applications in various fields, including finance, economics, and physics. By mastering the techniques and strategies for solving these types of equations and inequalities, mathematicians and scientists can solve complex problems and make accurate predictions in a wide range of disciplines.
Solving Exponential Equations and Inequalities: 7 Essential Answers
Exponential equations and inequalities play a crucial role in mathematics and have various applications in real-life scenarios. Understanding how to solve these equations and inequalities is essential for solving problems involving exponential growth or decay, compound interest, population growth, and many other fields.
Here are seven essential answers to commonly asked questions about solving exponential equations and inequalities:
- What is an exponential equation?
- How do you solve an exponential equation with the same base on both sides?
- What if the bases are different?
- How do you solve exponential inequalities?
- What are the possible solutions to an exponential equation or inequality?
- What are extraneous solutions?
- When should you use a different approach to solve exponential equations?
An exponential equation is an equation in which the variable appears in one or more exponents. It can be written in the form (a^x = b), where (a) is the base, (x) is the variable, and (b) is a constant.
To solve an exponential equation with the same base on both sides, you can set the exponents equal to each other and solve for the variable. For example, if you have the equation (3^x = 9), you can rewrite it as (3^x = 3^2) and equate the exponents to get (x = 2).
If the bases are different, you can use logarithms to solve the equation. Take the logarithm of both sides using any base, and then use the properties of logarithms to simplify the equation and solve for the variable.
Solving exponential inequalities involves finding the values of the variable that satisfy the inequality. You can use similar methods as solving exponential equations, such as setting the exponents equal to each other or using logarithms, but you need to consider the signs of the bases and constants to determine the direction of the inequality.
Depending on the values of the base and constant, an exponential equation or inequality can have zero, one, or multiple solutions. It is important to check any solutions obtained to ensure they are valid in the given context.
Extraneous solutions are solutions obtained during the solving process that do not satisfy the original equation or inequality. They can occur when taking the logarithm of a negative number or when raising a negative number to an even exponent. It is crucial to check for extraneous solutions and discard them.
In some cases, it may be necessary to use different approaches to solve exponential equations. These approaches can include factoring, completing the square, or using special properties of exponential functions. It is important to be flexible and determine the most appropriate method based on the given equation and context.
By understanding the answers to these essential questions, you can confidently solve exponential equations and inequalities, and apply this knowledge to various mathematical and real-world problems.
What are Exponential Equations and Inequalities?
Exponential equations and inequalities are mathematical expressions that involve exponential functions. An exponential function is a function in which the variable appears in the exponent. These types of equations and inequalities play a crucial role in various fields of science, such as physics, chemistry, and economics.
In an exponential equation, the goal is to find the value of the variable that satisfies the equation. The equation may involve various operations, such as addition, subtraction, multiplication, or division, including exponential functions. Solving exponential equations requires applying logarithmic properties and using algebraic techniques to isolate the variable.
In an exponential inequality, the goal is to find the range of values for the variable that satisfy the inequality. The inequality may be greater than, less than, greater than or equal to, or less than or equal to an exponential expression. Solving exponential inequalities involves similar techniques used in solving equations but requires careful consideration of the properties of exponential functions and their behavior.
Exponential equations and inequalities often arise in real-world scenarios involving population growth, compound interest, radioactive decay, and more. Understanding how to solve these equations and inequalities allows us to make predictions and analyze exponential phenomena in various disciplines.
Why Solving Exponential Equations and Inequalities is Important

Solving exponential equations and inequalities is an important skill in mathematics that has various applications in the real world. It allows us to model and analyze exponential growth and decay phenomena, which are frequently encountered in fields such as finance, population growth, and scientific research.
One of the key reasons why solving exponential equations and inequalities is important is its relevance to financial planning and investment decisions. Understanding how to solve these equations helps in analyzing compound interest problems, determining the growth or decay rate of an investment, and making informed decisions about borrowing or saving money.
Moreover, solving exponential equations and inequalities is crucial in studying population dynamics. By solving these equations, we can predict the growth or decline of a population, estimate the time it takes for a population to double or triple, and analyze how various factors, such as birth rates and migration, affect population size over time.
In scientific research, solving exponential equations and inequalities plays a vital role in understanding complex phenomena. It allows scientists to model the growth or decay of substances, study the rate at which a drug is metabolized in the body, or analyze the behavior of radioactive materials.
In summary, solving exponential equations and inequalities is a valuable mathematical skill that has wide-ranging applications in finance, population dynamics, and scientific research. It enables us to make informed decisions, predict future outcomes, and analyze complex systems. By mastering this skill, we can better understand and navigate the exponential growth and decay phenomena that are present in various aspects of our lives.
Step-by-Step Guide to Solving Exponential Equations
Exponential equations are mathematical equations that involve exponential functions. These types of equations often arise in various fields such as physics, engineering, and finance. Solving exponential equations requires some knowledge of exponential properties and algebraic manipulation. Here is a step-by-step guide to help you solve exponential equations:
- Step 1: Identify the base and the exponent in the equation. The base is the number that is raised to a power, and the exponent is the power to which the base is raised.
- Step 2: Isolate the exponential term. If the exponential term is on one side of the equation, you can isolate it by moving all other terms to the other side.
- Step 3: Apply logarithms. If the equation is of the form (a^x = b), take the logarithm on both sides of the equation. This allows you to rewrite the equation in terms of logarithms.
- Step 4: Solve for the variable. Use algebraic manipulation and properties of logarithms to simplify the equation and solve for the variable.
- Step 5: Check your solution. Substitute the value you obtained for the variable back into the original equation to ensure that it satisfies the equation.
By following these steps, you can effectively solve exponential equations. It is important to remember that practice is key to becoming proficient in solving these types of equations. As you gain more experience, you will develop a better understanding of the strategies and techniques involved in solving exponential equations.
Common Challenges and Tips for Solving Exponential Equations
Solving exponential equations can be challenging, as they involve variables in both the base and the exponent. However, by following a few key strategies, you can simplify the process and find solutions to these equations.
1. Understanding the properties of exponents: Before attempting to solve exponential equations, it is crucial to have a solid understanding of the properties of exponents. These properties include the multiplication and division rules, the power rule, and the zero exponent rule. Familiarizing yourself with these concepts will make it easier to manipulate equations and simplify them.
2. Isolating the variables: One of the common challenges in solving exponential equations is isolating the variable. To do this, you may need to use logarithms or apply the inverse operations to both sides of the equation. Remember that if the variable is in the exponent, taking the logarithm of both sides can help bring it down, making it easier to solve.
3. Being aware of extraneous solutions: When solving exponential equations, it is essential to check for extraneous solutions. These are solutions that may satisfy the equation algebraically, but when substituted back into the original equation, they do not make sense. Pay close attention to the domain restrictions and any potential values that could result in an undefined expression.
4. Making use of substitution and factoring: In some cases, substitution or factoring can simplify the equation and make it easier to solve. For example, if you have a quadratic equation with an exponential term, you can substitute a new variable to create a quadratic equation that can be factored or solved using the quadratic formula.
5. Practice and review: The key to mastering solving exponential equations is practice and review. The more exposure you have to different types of exponential equations, the more comfortable you will become with the process. Look for opportunities to practice with a variety of problems and seek feedback if possible to improve your skills.
In conclusion, solving exponential equations can be challenging, but with a solid understanding of the properties of exponents, the ability to isolate variables, awareness of extraneous solutions, knowing when to use substitution or factoring, and enough practice and review, you can become proficient in solving these equations.
Strategies for Solving Exponential Inequalities
Exponential inequalities involve equations with variables in the exponent. These equations can be challenging to solve, but with the right strategies, it is possible to find the solution. Here are some strategies to keep in mind when solving exponential inequalities:
- Isolate the exponential term: Start by isolating the exponential term on one side of the inequality. This can be done by moving all other terms to the opposite side.
- Take the logarithm: Once the exponential term is isolated, take the logarithm of both sides of the inequality. This will help in transforming the exponential equation into a logarithmic equation.
- Apply logarithmic properties: Use the properties of logarithms to simplify the equation. These properties include the power rule, product rule, and quotient rule. Applying these properties can help in solving for the variable.
- Solve for the variable: After applying the logarithmic properties, the equation should be transformed into a form where the variable can be easily isolated. Solve for the variable using algebraic methods, such as factoring or the quadratic formula.
- Check for extraneous solutions: Finally, check the solution obtained by substituting it back into the original inequality. Sometimes, the solution may result in an extraneous solution, which does not satisfy the original inequality. Discard any extraneous solutions.
By following these strategies, exponential inequalities can be solved effectively. Practice and familiarity with logarithmic properties are key to mastering the solution techniques for these types of equations. Remember to always check the solution obtained to ensure its validity in the original inequality.
Real-Life Applications of Solving Exponential Equations and Inequalities
In real-life situations, exponential equations and inequalities are commonly encountered in various fields such as finance, population growth, and radioactive decay. By understanding how to solve these equations and inequalities, we can make informed decisions and predictions based on the given data and its exponential pattern.
One practical application of solving exponential equations and inequalities is in finance. For example, compound interest formulas often involve exponential growth or decay, where the interest is compounded over time. By solving exponential equations, we can determine the future value of an investment or the length of time it would take to reach a certain amount.
Another real-life application is in population growth studies. Exponential equations can be used to model and predict population growth or decline. By solving these equations, we can estimate future population sizes, determine factors that affect growth rates, and make projections for urban planning and resource allocation.
Furthermore, exponential equations and inequalities are useful in the field of science, particularly in radioactive decay. The half-life of a radioactive substance can be expressed as an exponential equation, allowing scientists to calculate the amount of time it takes for a substance to decay to a certain level. This information is critical in industries such as nuclear energy, radiology, and archaeology.
In conclusion, the ability to solve exponential equations and inequalities has significant real-life applications in finance, population growth studies, and the field of science. By understanding the underlying exponential patterns and using mathematical tools to solve these equations, we can make informed decisions and predictions that impact our daily lives and various industries.
Q&A:
What are some real-life applications of solving exponential equations and inequalities?
Real-life applications of solving exponential equations and inequalities include calculating population growth, compound interest, radioactive decay, and the spread of diseases.
How can solving exponential equations and inequalities help in calculating population growth?
By solving exponential equations and inequalities, we can determine the rate at which a population is growing or declining over time and predict future population sizes.
What is the significance of solving exponential equations and inequalities in compound interest calculations?
When calculating compound interest, solving exponential equations and inequalities allows us to determine the amount of money earned or owed over time due to the compounding effect.
How can solving exponential equations and inequalities be applied to understanding radioactive decay?
By solving exponential equations and inequalities, we can determine the rate at which a radioactive substance decays over time and predict the amount of remaining radioactive material.
How can solving exponential equations and inequalities be used to model the spread of diseases?
By solving exponential equations and inequalities, we can model the rate at which a disease spreads through a population and predict the number of infected individuals over time.
What are some real-life applications of solving exponential equations and inequalities?
Some real-life applications of solving exponential equations and inequalities include financial planning and investments, population growth and decay in biology and ecology, determining the half-life of radioactive substances in chemistry and physics, and analyzing the spread of infectious diseases.