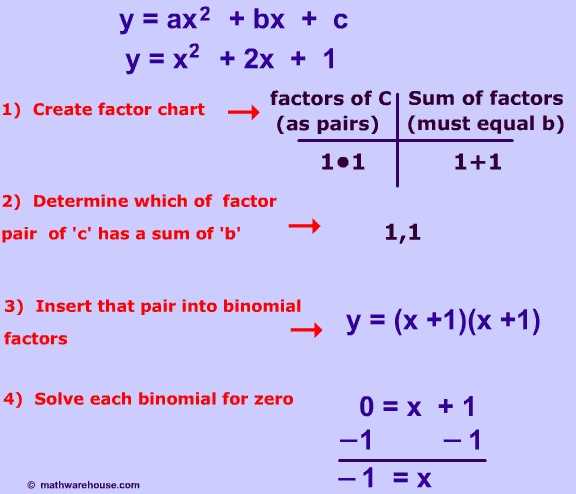
In mathematics, quadratic equations are polynomial equations with the highest power of the variable being two. They can be written in the form ax^2 + bx + c = 0, where a, b, and c are constants and x represents the variable. Solving quadratic equations involves finding the values of x that make the equation true.
Quadratic equations have various methods of solution, including factoring, completing the square, and using the quadratic formula. In the 9-3 solving quadratic equations answer key, students are provided with the solutions to the quadratic equations they have been working on.
The 9-3 solving quadratic equations answer key contains the correct values of x for each equation, allowing students to check their work and understand any errors they may have made. This answer key helps students practice and reinforce their understanding of solving quadratic equations, an essential skill in algebra and higher-level mathematics.
By using the 9-3 solving quadratic equations answer key, students can verify their solutions, compare them with the correct answers, and learn from any mistakes they may have made. This process encourages the development of problem-solving skills and helps students build confidence in their mathematical abilities.
Solving Quadratic Equations Answer Key -3
When solving quadratic equations, it is important to have a clear understanding of the steps involved in finding the solution. The answer key for solving quadratic equations -3 provides a comprehensive guide to help you solve these equations with ease.
The answer key includes step-by-step explanations for solving quadratic equations using various techniques such as factoring, completing the square, and using the quadratic formula. Each step is clearly outlined, making it easy for students to follow along and understand the process.
The answer key also includes practice problems with solutions, allowing students to apply the techniques learned to real-world examples. This helps to reinforce the concepts and improve problem-solving skills. Additionally, the answer key provides tips and tricks for solving quadratic equations more efficiently.
By utilizing the -3 solving quadratic equations answer key, students can gain confidence in their ability to solve these types of equations. They can also develop a deeper understanding of the underlying concepts and techniques involved. Whether preparing for an exam or looking to improve overall math skills, the answer key serves as a valuable resource.
Understanding Quadratic Equations
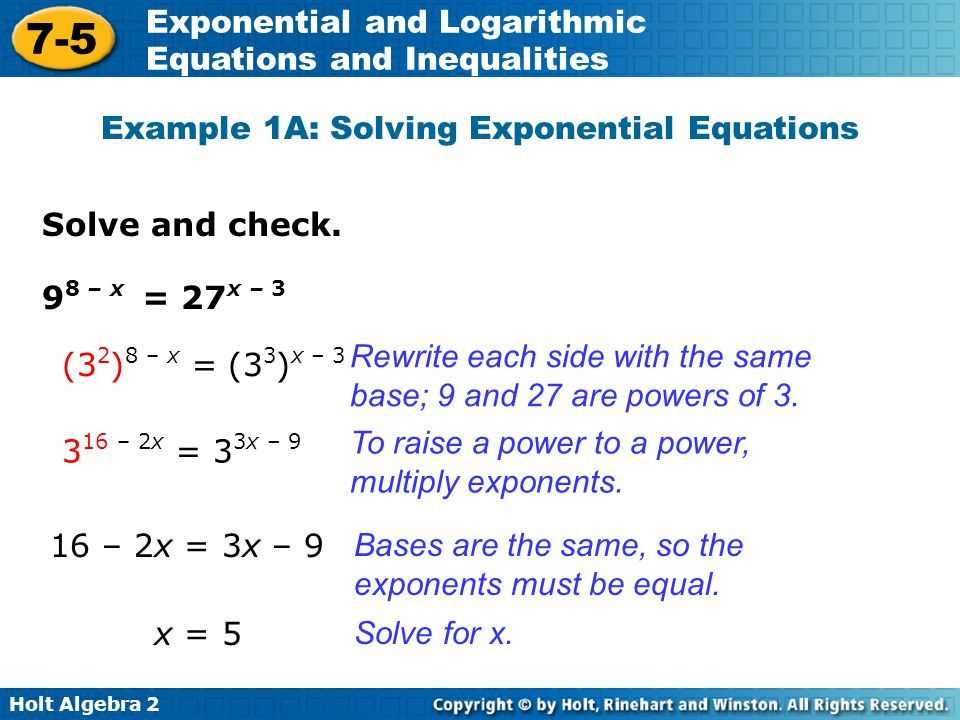
A quadratic equation is a polynomial equation of degree 2, which means that the highest power of the variable is 2. It can be written in the form ax^2 + bx + c = 0, where a, b, and c are constants. Solving quadratic equations involves finding the values of x that make the equation true.
Quadratic equations can have two, one, or no real solutions. This depends on the discriminant, which is the part of the equation under the square root (√) symbol. If the discriminant is positive, the equation has two real solutions; if it is zero, the equation has one real solution; and if it is negative, the equation has no real solutions.
There are various methods for solving quadratic equations, such as factoring, completing the square, or using the quadratic formula. Factoring involves finding two binomials whose product is equal to the quadratic equation. Completing the square involves manipulating the equation to create a perfect square trinomial. The quadratic formula is a formula that gives the solutions to any quadratic equation.
Once the solutions to a quadratic equation are found, they can be used to solve real-world problems involving quadratic relationships, such as finding the maximum or minimum value of a quadratic function, determining the height of an object thrown into the air, or finding the time it takes for two objects to collide.
Understanding quadratic equations is essential in many fields of study, including mathematics, physics, engineering, and computer science. They provide a powerful tool for modeling and analyzing various phenomena and can be used to solve a wide range of problems.
Solving Quadratic Equations by Factoring
Solving quadratic equations can often be done by factoring the quadratic expression and setting each factor equal to zero. This method is especially useful when the quadratic equation has two distinct solutions.
To start, we need to express the quadratic equation in the form of ax^2 + bx + c = 0, where a, b, and c are coefficients. Once we have the expression in this form, we can look for two factors of the constant term, c, that add up to the coefficient of the linear term, b.
For example, consider the quadratic equation 2x^2 + 5x – 3 = 0. We can rewrite this equation as (2x – 1)(x + 3) = 0. By setting each factor equal to zero, we get two separate linear equations: 2x – 1 = 0 and x + 3 = 0. Solving these equations, we find x = 1/2 and x = -3 as the two solutions to the quadratic equation.
It’s important to note that not all quadratic equations can be factored easily. In some cases, the quadratic expression may not factor into two linear terms, or the factors may not be easily determined. In these situations, other methods such as the quadratic formula or completing the square may be necessary to solve the equation.
Solving Quadratic Equations using the Quadratic Formula
When faced with a quadratic equation in the form of ax^2 + bx + c = 0, the quadratic formula is a powerful tool that can be used to find the values of x that satisfy the equation. The quadratic formula is derived from completing the square technique and it allows us to find the roots of the equation, which are the values of x that make the equation true.
The quadratic formula is given by the equation:
x = (-b ± √(b^2 – 4ac)) / 2a
To use the quadratic formula, we need to identify the values of a, b, and c in the given equation. Once we have those values, we can substitute them into the quadratic formula and simplify the equation. The term inside the square root, known as the discriminant, b^2 – 4ac, determines the nature of the roots.
If the discriminant is positive, the equation will have two distinct real roots. If the discriminant is zero, the equation will have one real root. If the discriminant is negative, the equation will have two complex roots.
By solving quadratic equations using the quadratic formula, we can find the values of x that satisfy the equation and understand the geometric interpretation of those solutions.
Using Completing the Square Method
The Completing the Square method is a technique used to solve quadratic equations. It involves rearranging a quadratic equation in order to create a perfect square trinomial and then solving for the unknown variable.
Here is the step-by-step process for solving a quadratic equation using Completing the Square:
- Start with a standard quadratic equation in the form of ax^2 + bx + c = 0.
- If the coefficient a is not equal to 1, divide the entire equation by a to make it equal to 1.
- Identify the values for b and c in the equation.
- Take half of the coefficient b and square it to get the term needed to complete the square.
- Add or subtract this term from both sides of the equation to create a perfect square trinomial on the left side of the equation.
- Factor the perfect square trinomial and write it in the form (x + p)^2 where p represents half of the coefficient b.
- Take the square root of both sides of the equation to isolate the variable x.
- Solve for x by setting the equation equal to both the positive and negative square root.
- Verify the solution by substituting the values of x back into the original equation.
Completing the Square method can be useful when factoring or using the quadratic formula is not an option. It is important to be familiar with this method as it is a fundamental technique in solving quadratic equations.
Graphing quadratic equations is an important skill in algebra and calculus. A quadratic equation is a polynomial equation of degree 2, which means it has a highest power of x squared. The general form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants.
To graph a quadratic equation, it is helpful to understand the properties of its graph. The graph of a quadratic equation is called a parabola, which is a U-shaped curve. The direction of the parabola depends on the value of a. If a is positive, the parabola opens upward, and if a is negative, the parabola opens downward.
One way to graph a quadratic equation is to create a table of values. Choose a few x-values and calculate the corresponding y-values using the equation. Plot the points on a coordinate plane and connect them to form the parabola. This method gives a good approximation of the graph.
Another method to graph a quadratic equation is to use the vertex form. The vertex form of a quadratic equation is y = a(x – h)^2 + k, where (h, k) is the vertex of the parabola. The vertex form gives information about the vertex, axis of symmetry, and direction of opening of the parabola.
Graphing quadratic equations is an essential skill for solving various real-life problems. Quadratic equations can model many situations, such as projectile motion, profit and revenue functions, and optimization problems. Being able to interpret and analyze graphs of quadratic equations allows us to understand the behavior and relationships in these situations.
Real-Life Applications of Quadratic Equations
In real-life situations, quadratic equations can be used to model various phenomena and solve problems. Here are some common applications:
Physics
Quadratic equations are used extensively in physics to describe the motion of objects. The equation used to describe the motion of a freely falling object near the surface of the Earth is a quadratic equation. By solving this equation, scientists can determine the object’s position, velocity, and acceleration at any given time.
Engineering

In engineering, quadratic equations are often used to model the behavior of physical systems. Engineers use these equations to predict the behavior and performance of structures, machines, and electronic circuits. For example, the trajectory of a projectile can be modeled using quadratic equations in order to determine its range, maximum height, and time of flight.
Economics
Quadratic equations are also used in economics to analyze and predict consumer behavior, market trends, and profit maximization. For instance, businesses can use quadratic equations to determine the optimal price for a product that maximizes profits based on demand and cost functions.
Computer Graphics
In computer graphics, quadratic equations are used to model various shapes and animations. Quadratic equations can be used to create smooth curves and realistic motion within computer-generated images. For example, the equations for conic sections, such as circles and ellipses, are quadratic equations.
Overall, quadratic equations have numerous real-life applications in various fields such as physics, engineering, economics, and computer graphics. They provide a powerful tool for modeling and solving problems in these disciplines, allowing scientists, engineers, and analysts to understand and predict the behavior of physical systems and phenomena.