
Focus on mastering key concepts such as linear equations, systems of equations, and quadratic functions. These areas will make up a significant portion of the exam. Be sure to practice solving equations, simplifying expressions, and graphing. Understanding how to apply the properties of exponents and radicals is critical for handling more complex problems.
Next, review how to factor polynomials and solve word problems. These skills will help you approach real-world scenarios and abstract questions with confidence. Don’t overlook practice problems involving slope-intercept form, as these questions are common in the exam. Review both basic and advanced techniques for solving inequalities and working with rational expressions.
Finally, reinforce your skills by working through practice exams and timed exercises. This will help you manage your time during the test. Pay close attention to questions that involve multiple steps, as these can often lead to mistakes if not handled carefully. Consistency in practice will build your confidence and readiness for the final exam.
Understanding Key Algebraic Concepts
Mastering algebra requires a strong grasp of foundational concepts. Start by focusing on these key areas:
- Variables: Represent unknown values, typically using letters like x, y, or z. Recognize that variables can take any value within a defined set, depending on the problem.
- Expressions: Combine variables, constants, and operators (addition, subtraction, multiplication, division) to form expressions. Practice simplifying these to make problem-solving easier.
- Equations: Solve for the value of a variable by isolating it on one side. Understand how to apply operations to both sides of the equation without changing the equality.
- Linear Functions: Represent straight-line relationships, typically in the form y = mx + b. Recognize how the slope (m) and y-intercept (b) influence the graph of the function.
- Factoring: Break down expressions like quadratic equations into simpler forms. This skill helps solve equations more efficiently.
- Exponent Rules: Use the laws of exponents to simplify expressions involving powers. These rules include multiplying, dividing, and raising powers to powers.
Work through problems that involve these concepts regularly. The more practice you get, the faster you’ll identify patterns and simplify solutions. Mastering these key topics will help you approach any algebra problem with confidence.
Step-by-Step Solutions to Practice Problems
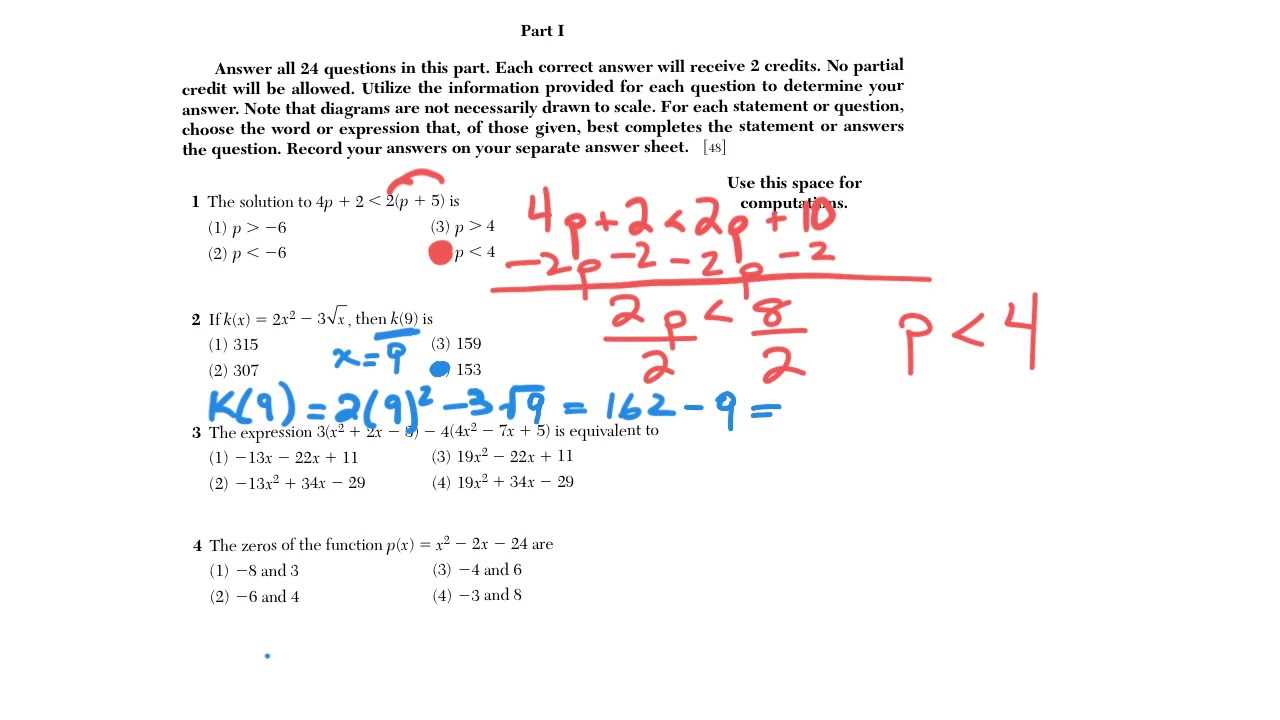
Focus on the core operations for solving equations. Begin by isolating the variable. If the equation involves addition or subtraction, eliminate constants first. For example, in the equation x + 5 = 12, subtract 5 from both sides to get x = 7.
For multiplication or division equations, reverse the operation. Consider 3x = 12. Divide both sides by 3 to find x = 4.
When working with expressions that involve fractions, find the least common denominator to simplify the equation. For instance, with 1/2x + 1/3x = 1, multiply both terms by 6 (the LCD) to eliminate fractions, resulting in 3x + 2x = 6, which simplifies to 5x = 6. Then divide both sides by 5 to solve for x = 6/5.
For quadratic equations, factor when possible. Take x² – 5x + 6 = 0 as an example. Factor the left side into (x – 2)(x – 3) = 0. Set each factor equal to 0: x – 2 = 0 or x – 3 = 0. Solve for x = 2 or x = 3.
In problems involving exponents, apply the laws of exponents. For x² * x³, add the exponents to get x⁵. In division, subtract the exponents. For example, x⁶ ÷ x² equals x⁴.
In inequalities, treat the variable just like an equation. When multiplying or dividing by a negative number, flip the inequality sign. For -2x > 6, divide by -2 to get x < -3, remembering to flip the inequality sign.
Common Mistakes to Avoid During the Exam
Rushing through calculations is a common mistake. Double-check your work before moving on to the next question. It’s easy to make minor errors when hurrying. Take a moment to confirm your results, especially for arithmetic and sign changes.
Misreading the question can lead to wrong answers. Always read the question carefully before solving. Look for key phrases or terms that indicate the required method, such as “solve for x” or “simplify the expression.”
Skipping steps may seem like a time-saver but often causes confusion later. Show every step, even if you’re confident in the answer. This ensures you don’t overlook any important details and helps you spot mistakes faster.
Not managing time effectively is a frequent issue. Keep track of the time for each section. Avoid spending too long on a single problem. If you’re stuck, move on and come back later to avoid losing valuable time.
Confusing negative signs with subtraction can lead to significant errors. Always distinguish between negative numbers and subtraction signs. Pay attention to parentheses to ensure proper handling of negative values.
Forgetting to check for extraneous solutions is crucial when solving equations, especially rational or square root equations. After solving, substitute the solutions back into the original equation to ensure they are valid.
Neglecting units when dealing with word problems or real-world applications can lead to incorrect answers. Always include the appropriate units in your final answer and ensure consistency throughout the problem.
Ignoring order of operations is another common mistake. Follow PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) carefully to avoid incorrect answers in multi-step problems.