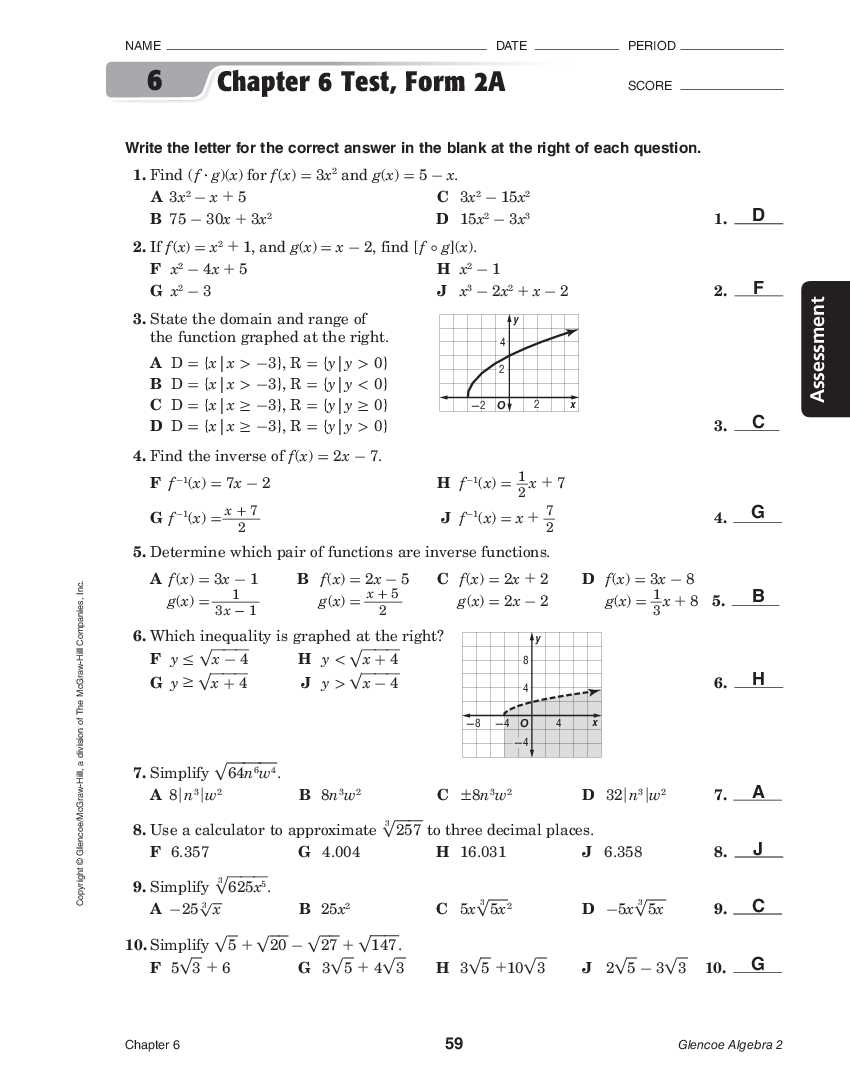
If you’re currently studying Algebra 2, then you’re most likely familiar with the challenges and complexities that come with this subject. Chapter 4 is no exception, as it deals with various topics such as exponential functions, logarithmic functions, properties of logarithms, and solving exponential and logarithmic equations. At the end of the chapter, students are often required to take a test to assess their understanding of these concepts. In this article, we will provide you with the answers to the Algebra 2 Chapter 4 test, helping you to check your work or prepare for a similar exam.
Exponential functions play a significant role in this chapter, and understanding their properties is crucial. You will encounter questions that ask you to identify the domain and range, sketch the graph of an exponential function, or find the equation of a given graph. Additionally, logarithmic functions and their corresponding properties will be tested. This includes evaluating logarithmic expressions, rewriting exponential equations in logarithmic form, and applying the properties of logarithms to simplify complex expressions.
Another important aspect of this chapter is solving exponential and logarithmic equations. You will be required to solve equations involving exponential and logarithmic functions, including those with multiple variables and ones that require the use of logarithmic properties to simplify. These types of problems often involve careful manipulation of equations and applying the laws of exponents and logarithms.
By providing the correct answers to the Algebra 2 Chapter 4 test, this article aims to assist you in your preparation and strengthen your understanding of the concepts covered in this chapter. It is essential to note that practicing more problems, reviewing the material, and seeking additional help from your instructor or peers will enhance your comprehension and improve your performance on future exams.
Overview of Algebra 2 Chapter 4 Test Answers
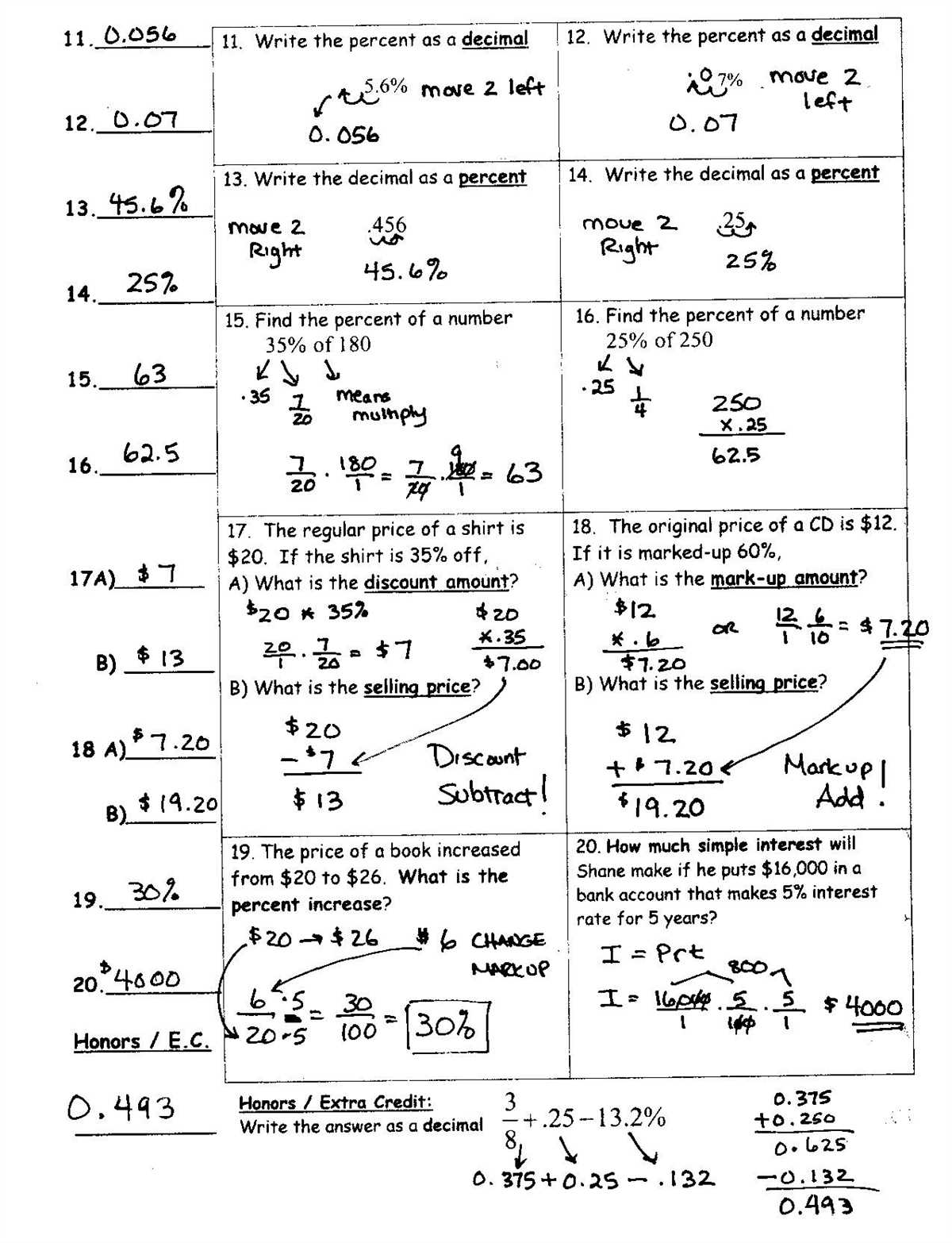
The Algebra 2 Chapter 4 test covers various topics related to functions, equations, and graphs. This chapter focuses on understanding and analyzing polynomial functions, including quadratic, cubic, and higher degree functions. Students are expected to demonstrate their knowledge of finding zeros and intercepts, determining the end behavior of polynomial functions, and using factoring and synthetic division to solve polynomial equations.
One of the main concepts covered in this chapter is the relationship between the degree of a polynomial function and its end behavior. Students are required to determine whether a polynomial function has an even or odd degree and identify the leading coefficient to determine the end behavior. They also need to plot the graph of polynomial functions using key points and transformations.
Another important topic covered in this chapter is factoring polynomial equations. Students need to be proficient in factoring quadratic, cubic, and higher degree polynomials and using the zero product property to find the solutions. They also need to know how to use synthetic division to divide polynomials and find the remainders.
Overall, the Algebra 2 Chapter 4 test assesses students’ understanding and application of polynomial functions, including finding zeros and intercepts, determining end behavior, graphing polynomials, and factoring polynomial equations. It is important for students to practice solving various types of polynomial equations and understanding the behavior of polynomial functions in order to successfully complete this chapter test.
Understanding Quadratic Equations and Functions
In algebra, a quadratic equation is a polynomial equation of the second degree. It can be written in the form ax^2 + bx + c = 0, where a, b, and c are constants, and a is not equal to zero. Quadratic equations can have two solutions, one solution, or no solutions at all. The solutions, also known as the roots, can be found using various methods such as factoring, completing the square, or using the quadratic formula.
Quadratic equations are often used to model real-life situations, particularly in physics and engineering. They help us understand and analyze the behavior of various phenomena, such as the trajectory of a projectile, the shape of a parabolic mirror, or the growth of populations. By solving quadratic equations, we can determine critical points, maximum or minimum values, and make predictions about the behavior of the system.
A quadratic function, on the other hand, is a function that can be expressed as f(x) = ax^2 + bx + c, where a, b, and c are constants. Quadratic functions have a U-shaped graph called a parabola. The vertex of the parabola represents the highest or lowest point of the function, depending on the value of a. The axis of symmetry is a vertical line passing through the vertex.
The graph of a quadratic function can provide us with valuable information about its behavior. We can determine the x-intercepts, or the points where the graph intersects the x-axis, by solving the equation f(x) = 0. The y-intercept is the point where the graph intersects the y-axis, which can be found by evaluating f(0). By analyzing the vertex and the shape of the graph, we can determine the maximum or minimum values of the function and make predictions about its behavior.
Overall, understanding quadratic equations and functions is essential in algebra and provides a foundation for solving various problems and analyzing real-life situations. By mastering the concepts and techniques related to quadratics, we can better comprehend the world around us and make informed decisions based on mathematical analysis.
Solving Quadratic Equations
In Algebra 2, one of the fundamental topics is solving quadratic equations. A quadratic equation is an equation of the form ax^2 + bx + c = 0, where a, b, and c are coefficients and x is the variable. The goal is to find the values of x that satisfy the equation.
There are several methods for solving quadratic equations. One of the most common methods is factoring. Factoring involves finding two binomials whose product is equal to the quadratic equation. By setting each binomial equal to zero and solving for x, we can find the solutions to the equation.
Another method for solving quadratic equations is using the quadratic formula. The quadratic formula is derived from completing the square and can be used to find the solutions to any quadratic equation. The formula is x = (-b ± √(b^2 – 4ac)) / (2a), where ± indicates the two possible solutions.
Additionally, quadratic equations can also be solved graphically by plotting the equation on a coordinate plane and finding the x-intercepts, which correspond to the solutions of the equation. This method can be useful for visualizing the solutions and understanding the behavior of the equation.
Overall, solving quadratic equations is a crucial skill in Algebra 2 and has many real-world applications. Whether using factoring, the quadratic formula, or graphing, the goal is to find the values of x that satisfy the equation and understand the relationship between the coefficients and the solutions. Practice and understanding of these methods are key to successfully solving quadratic equations.
Graphing Quadratic Functions

A quadratic function is a function that can be written in the form f(x) = ax^2 + bx + c, where a, b, and c are constants and a is not equal to 0. Graphing quadratic functions can help us understand their behavior and find key features such as the vertex, axis of symmetry, and intercepts.
To graph a quadratic function, we can start by plotting a few key points and then connect them to create a smooth curve. One way to find these key points is by using the vertex formula, -b/2a. The vertex is the highest or lowest point on the graph and represents the axis of symmetry. Once we have the vertex, we can find the y-intercept by substituting x = 0 into the function.
We can also find the x-intercepts by setting the function equal to zero and solving for x. These x-intercepts are also known as the roots or zeros of the quadratic function. The number of x-intercepts can be determined by the discriminant, b^2 – 4ac. If the discriminant is positive, there are two distinct x-intercepts. If the discriminant is zero, there is only one x-intercept (also the vertex). If the discriminant is negative, there are no real x-intercepts.
When graphing quadratic functions, it’s important to also consider the axis of symmetry, which is a vertical line that passes through the vertex. The graph will be symmetric about this line. Additionally, the shape of the graph can be determined by the sign of the coefficient a. If a is positive, the graph opens upwards and has a minimum value at the vertex. If a is negative, the graph opens downwards and has a maximum value at the vertex.
In conclusion, graphing quadratic functions involves finding key points such as the vertex, intercepts, and axis of symmetry. This allows us to visualize the behavior and characteristics of the function. By understanding how to graph quadratic functions, we can solve real-world problems and make predictions based on the given data.
Analyzing the Key Characteristics of Quadratic Functions
Quadratic functions play a crucial role in algebra and have several key characteristics that can be analyzed to gain a better understanding of their behavior. These functions can be represented by the equation y = ax^2 + bx + c, where a, b, and c are constants. One of the primary characteristics of a quadratic function is its vertex, which represents the highest or lowest point on the graph. The vertex can be determined using the formula x = -b/(2a) and plugging the value into the equation to find the corresponding y value.
Another important characteristic is the axis of symmetry, which is a vertical line that passes through the vertex. The equation of the axis of symmetry can be found using x = -b/(2a). Understanding the axis of symmetry helps in determining the symmetry of the graph. Quadratic functions can be symmetrical about the y-axis or have no symmetry at all.
Additionally, the value of a determines whether the graph of the quadratic function opens upwards or downwards. If a is positive, the graph opens upwards, and if a is negative, it opens downwards. The coefficient a also affects the steepness of the graph. A larger absolute value of a makes the graph steeper, while a smaller absolute value makes it flatter.
The roots, or solutions, of a quadratic function can be found by setting y equal to zero and solving the resulting quadratic equation. The number of roots and their nature (real or complex) provides information about the intersections of the graph with the x-axis. The discriminant, b^2 – 4ac, can be used to determine the nature of the roots.
By analyzing these key characteristics, one can gain insights into the behavior and properties of quadratic functions. Understanding these characteristics enables mathematicians to solve equations, graph functions, and model real-life situations.
Applying the Quadratic Formula
The quadratic formula is a powerful tool in solving quadratic equations. It provides a method for finding the solutions, or roots, of a quadratic equation of the form ax^2 + bx + c = 0. This formula is derived from completing the square and can be applied to any quadratic equation, regardless of the values of a, b, and c.
The quadratic formula is expressed as:
x = (-b ± √(b^2 – 4ac)) / 2a
This formula allows us to determine the values of x that satisfy the given quadratic equation. The term inside the square root, known as the discriminant, determines the nature of the solutions. If the discriminant is positive, the equation will have two distinct real solutions. If the discriminant is zero, the equation will have one real solution, and if the discriminant is negative, the equation will have two complex solutions.
When applying the quadratic formula, it is essential to follow the proper order of operations and simplify the expression before determining the values of x. It is also crucial to pay attention to the signs in the formula to correctly identify whether the solutions are positive or negative. In some cases, the equation may have no real solutions, indicating that the graph of the quadratic function does not intersect the x-axis.
The quadratic formula is a valuable tool in algebra and is used extensively in various fields of mathematics and science. It allows us to solve quadratic equations accurately and efficiently, providing us with the solution to various real-world problems that involve quadratic functions.
Using Factoring to Solve Quadratic Equations

Factoring is a powerful technique in algebra that allows us to solve quadratic equations. By factoring a quadratic expression, we can rewrite it as a product of two binomials and then set each factor equal to zero to find the solutions. This method is especially useful when the quadratic equation cannot be easily solved by other methods, such as the quadratic formula.
To solve a quadratic equation using factoring, follow these steps:
- First, set the equation equal to zero, so that you have a quadratic expression in the form ax^2 + bx + c = 0.
- Look for common factors among the terms. If there are common factors, factor them out.
- Write the quadratic expression as a product of two binomials. If the quadratic expression cannot be factored into two binomials, then it cannot be factored using the factoring method.
- Set each factor equal to zero and solve for the variable.
- Once you have found the solutions for the variable, check your answers by substituting them back into the original equation.
Factoring can be used to solve a variety of quadratic equations, whether they are in standard form, vertex form, or factored form. It is important to practice factoring and become familiar with the different methods and techniques in order to effectively solve quadratic equations.
By mastering the skill of factoring, you can confidently solve quadratic equations and apply this knowledge to other areas of mathematics and real-world problems. Factoring is a fundamental algebraic technique that opens up new possibilities for understanding and manipulating quadratic expressions. With practice and experience, you will become proficient in factoring and solving quadratic equations using this method.
Q&A:
What is factoring?
Factoring is the process of breaking down an expression or equation into its factors. In the context of quadratic equations, factoring involves finding two binomials that multiply together to give the quadratic equation.
Why is factoring important in solving quadratic equations?
Factoring is important in solving quadratic equations because it allows us to find the values of the unknown variable, or the solutions, by setting each factor equal to zero and solving for the variable. This helps in finding the x-intercepts or the points where the graph of the quadratic equation intersects the x-axis.
How do you factor a quadratic equation?
To factor a quadratic equation, first set it equal to zero. Then, try to find two binomials that multiply together to give the quadratic expression. This can be done by factoring out the greatest common factor, using the difference of squares or the perfect square trinomial patterns, or by trial and error. Once you have factored the quadratic equation, set each factor equal to zero and solve for the variable.
Can all quadratic equations be factored?
No, not all quadratic equations can be factored. Some quadratic equations have no real solutions, meaning they do not intersect the x-axis at any point. These equations cannot be factored using real numbers. However, they can be factored using complex numbers.
What are the advantages of factoring quadratic equations?
The advantages of factoring quadratic equations are that it provides a systematic method for solving these equations, helps in finding the solutions or x-intercepts graphically, and allows for simplification and interpretation of the equation. Factoring also provides insight into the relationship between the factors and the equation itself.