
Success on advanced math exams requires both understanding and practice. To achieve a high score, it’s essential to engage with exercises that mirror the format and complexity of the actual test. By tackling a variety of problems, you’ll strengthen your skills and boost your confidence for the big day.
Focus on Core Concepts
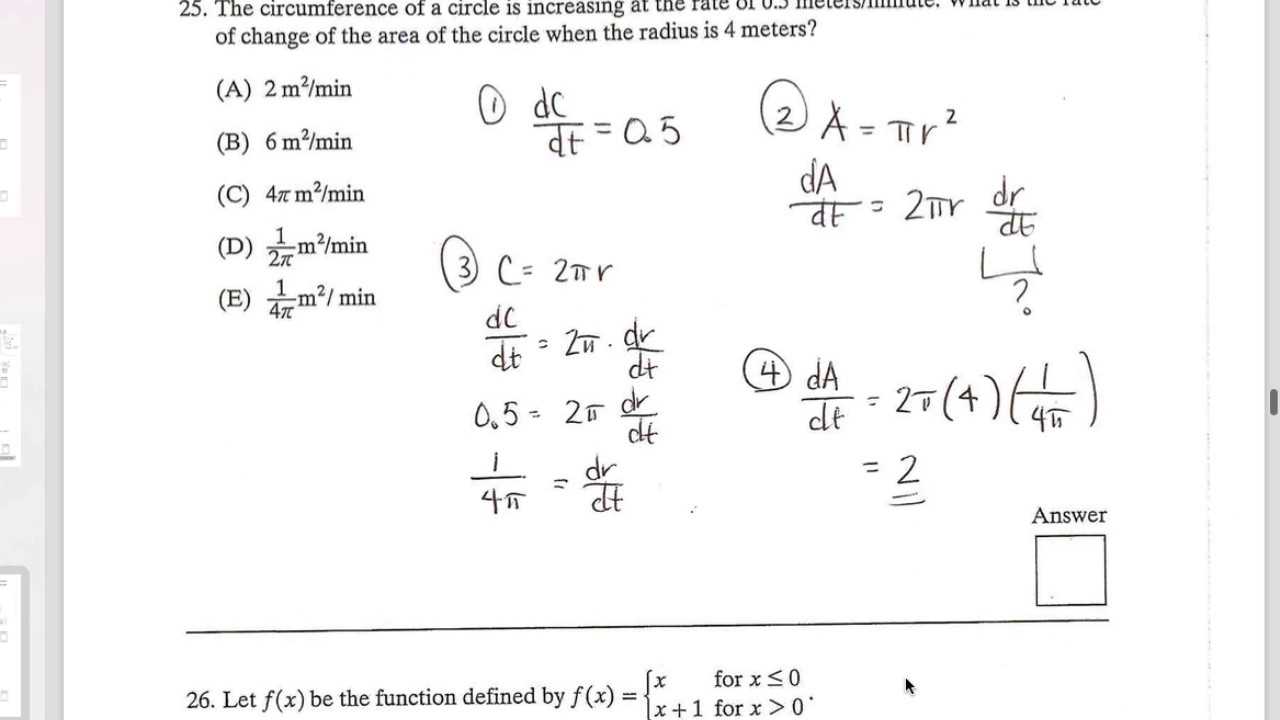
Building a solid foundation in essential mathematical principles is crucial. Focus on the most commonly tested topics, such as functions, graph analysis, and algebraic manipulation. Strengthening your knowledge in these areas will help you solve complex questions with ease.
Key Topics to Master:
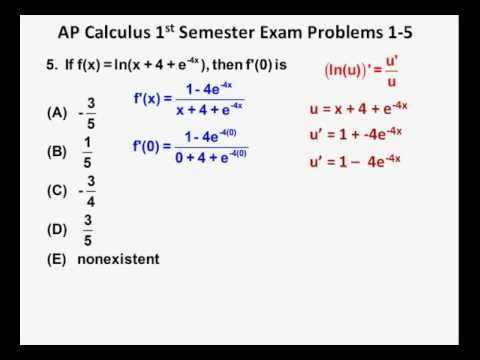
- Function transformations
- Graph interpretation
- Equations and inequalities
- Trigonometry and its applications
Simulate Real Exam Conditions
Recreating the exam environment during your preparation allows you to better manage time and reduce stress. Set aside a quiet space, limit distractions, and follow a strict time frame for each session. This approach prepares you mentally and physically for the actual challenge.
Tips for Simulation:
- Use a timer to track progress
- Set a goal for the number of problems to solve within the given time
- Avoid looking at solutions until the exercise is complete
Review Your Performance
Once you’ve completed a set of exercises, thoroughly review your results. This step is essential for identifying areas that need improvement. Take the time to analyze mistakes and understand the correct methods for solving each problem.
Steps for Effective Review:
- Highlight where you went wrong and why
- Revisit the relevant concepts to deepen your understanding
- Reattempt similar questions to reinforce your skills
Final Thoughts
Regular practice, along with careful review and strategy, is key to success. Approach your preparation with focus, and don’t hesitate to seek help when needed. With consistent effort, you’ll be ready to tackle even the most challenging questions on exam day.
Effective Strategies for Success in Advanced Math Exams
Mastering complex mathematical concepts requires more than just theoretical knowledge. To truly excel, you need to adopt a systematic approach to studying, refine your problem-solving skills, and actively engage with the material. This section outlines key strategies for optimizing your preparation, helping you to approach your exam with confidence and clarity.
Grasping Core Mathematical Concepts
At the heart of any successful exam strategy is a deep understanding of core principles. Focus on the fundamentals–such as functions, graphs, and algebraic techniques–that are frequently tested. By internalizing these concepts, you’ll be able to recognize patterns and solve problems more effectively, even when faced with unfamiliar challenges.
How Simulated Exams Enhance Performance
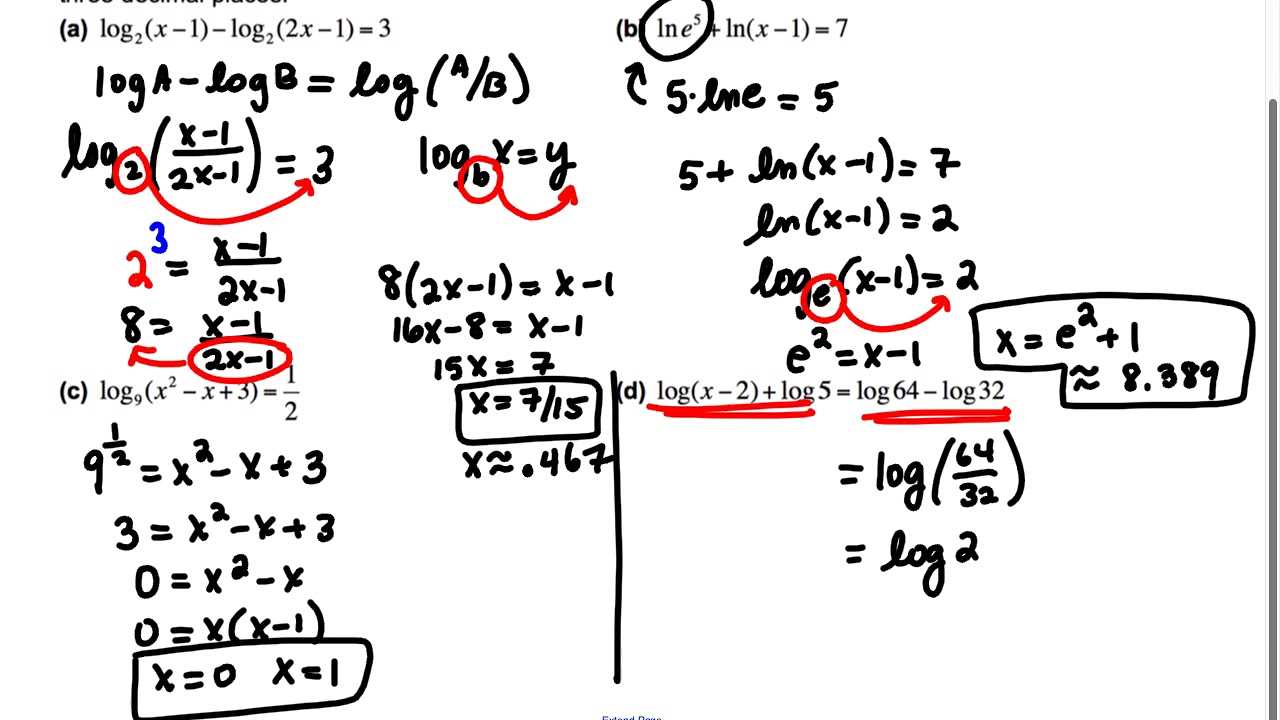
Recreating real exam conditions during your preparation is a powerful technique for improving performance. These simulated sessions allow you to develop time-management skills, reduce anxiety, and familiarize yourself with the structure of the questions. Additionally, they highlight areas where you may need to refine your knowledge.
Avoiding Common Mistakes
Many students struggle with certain types of errors that can negatively impact their performance. Common pitfalls include misinterpreting questions, overlooking key details, and rushing through problems. Developing a habit of carefully reading instructions and double-checking your work will help prevent these mistakes and ensure more accurate results.
Step-by-Step Solutions to Problem Solving
When approaching complex problems, break them down into smaller, manageable steps. By applying a logical progression to each question, you can solve even the most difficult tasks with greater efficiency. Focus on the methodology rather than just the answer to gain a deeper understanding of the process involved.
Maximizing Exam Performance
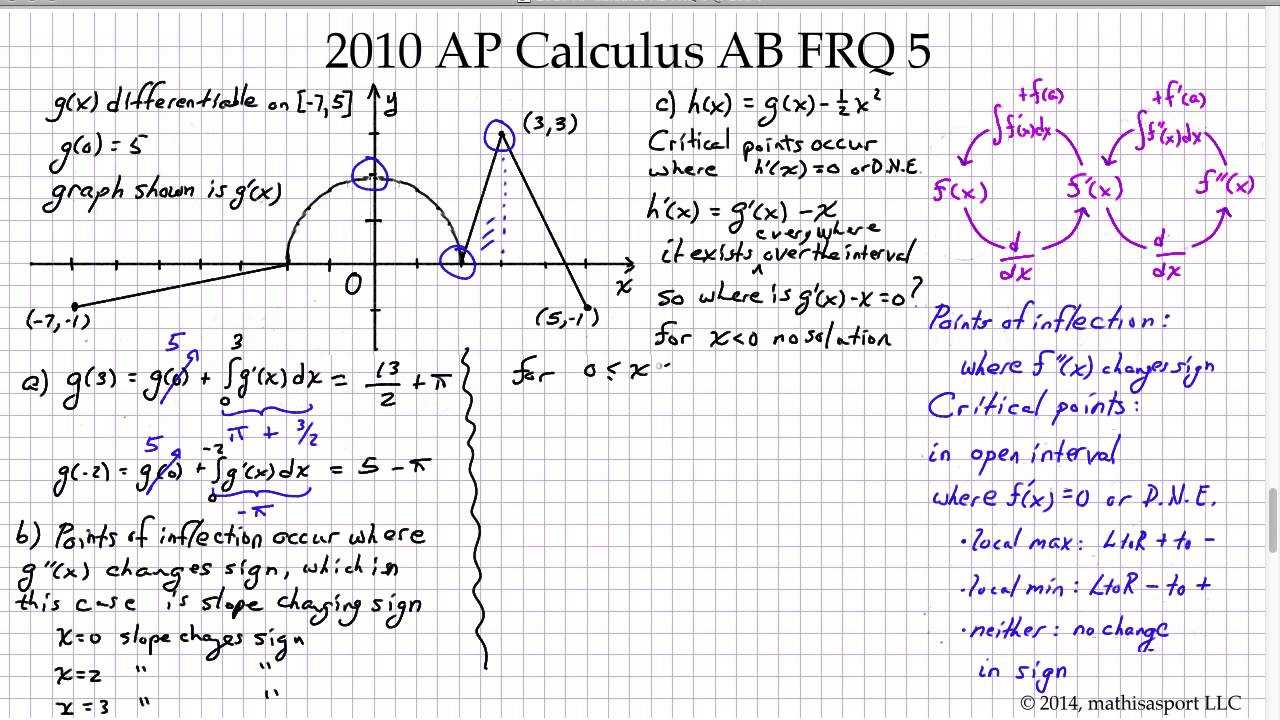
To truly excel, it’s essential to focus on both knowledge and strategy. Stay consistent with your study schedule, take regular breaks to maintain focus, and refine your approach through continuous practice. By following these techniques, you’ll be able to approach each problem with the necessary confidence and skill to achieve your best results.